Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.
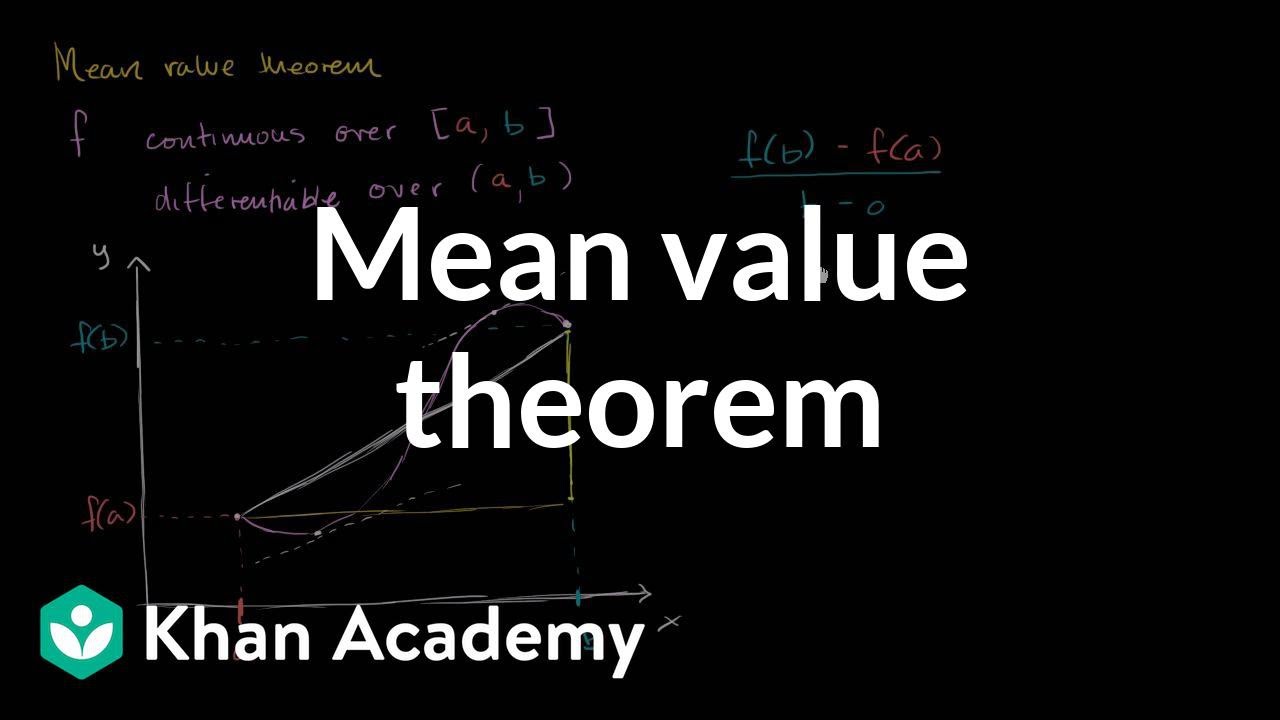
TLDRThe video script provides an engaging explanation of Rolle's Theorem and its relation to the Mean Value Theorem. Rolle's Theorem states that if a function is continuous on a closed interval [a, b], differentiable on the open interval (a, b), and crosses the x-axis at some point within this interval, there must exist at least one point 'c' where the derivative of the function (f'(c)) is zero. This is visualized as a horizontal tangent line on the graph of the function. The Mean Value Theorem, a broader concept, states that for a differentiable function on an interval, there exists a point where the derivative equals the average rate of change (slope) between any two points on the interval. The script simplifies these mathematical concepts by using the analogy of a secant line's slope and emphasizes the verification process for these theorems, which involves setting the first derivative equal to zero and ensuring the solution lies within the given interval.
Takeaways
- 📍 Rolle's Theorem states that if a function is continuous and crosses the x-axis at two points, there must be at least one point between them where the slope is zero.
- 🔍 The Mean Value Theorem is a corollary of Rolle's Theorem, which applies when the function is not only continuous but also differentiable between two points.
- 📈 If a function is differentiable on an interval, there exists at least one point where the derivative (slope of the tangent) is equal to the average rate of change (slope of the secant line) between any two points in that interval.
- 🔢 To verify Rolle's Theorem, one must find the first derivative of the function, set it equal to zero, and solve for x, ensuring that the solution lies within the given interval.
- 📐 The Mean Value Theorem can be visualized by drawing a secant line between two points on the curve of a differentiable function, and there will be at least one point where the slope of the tangent equals the slope of the secant.
- 🤔 Rolle's Theorem is a special case of the Mean Value Theorem where the secant line is horizontal, implying the slope is zero.
- 💡 The slope of the tangent line at a point on the curve can be found by evaluating the first derivative of the function at that point.
- 📉 For a function to satisfy Rolle's Theorem, it must be continuous and differentiable on the interval, with at least one point where the derivative is zero.
- 🔑 The Mean Value Theorem is a powerful tool in calculus that can be used to find the average rate of change and the existence of a tangent with a specific slope.
- 📋 To apply the Mean Value Theorem, one must ensure the function meets the criteria of being continuous and differentiable on the given interval.
- 🔗 The concepts of Rolle's Theorem and the Mean Value Theorem are fundamental in understanding the behavior of functions and their derivatives in calculus.
Q & A
What is Rolle's Theorem?
-Rolle's Theorem states that if a function is continuous on a closed interval [a, b], differentiable on the open interval (a, b), and f(a) = f(b), then there exists at least one point c in the interval (a, b) where the derivative of the function f'(c) is equal to zero.
What is a condition for Rolle's Theorem to apply?
-Rolle's Theorem applies if the function crosses the x-axis at two points within an interval, is continuous on that interval, and is differentiable on the open interval between the two points.
What does the Mean Value Theorem state?
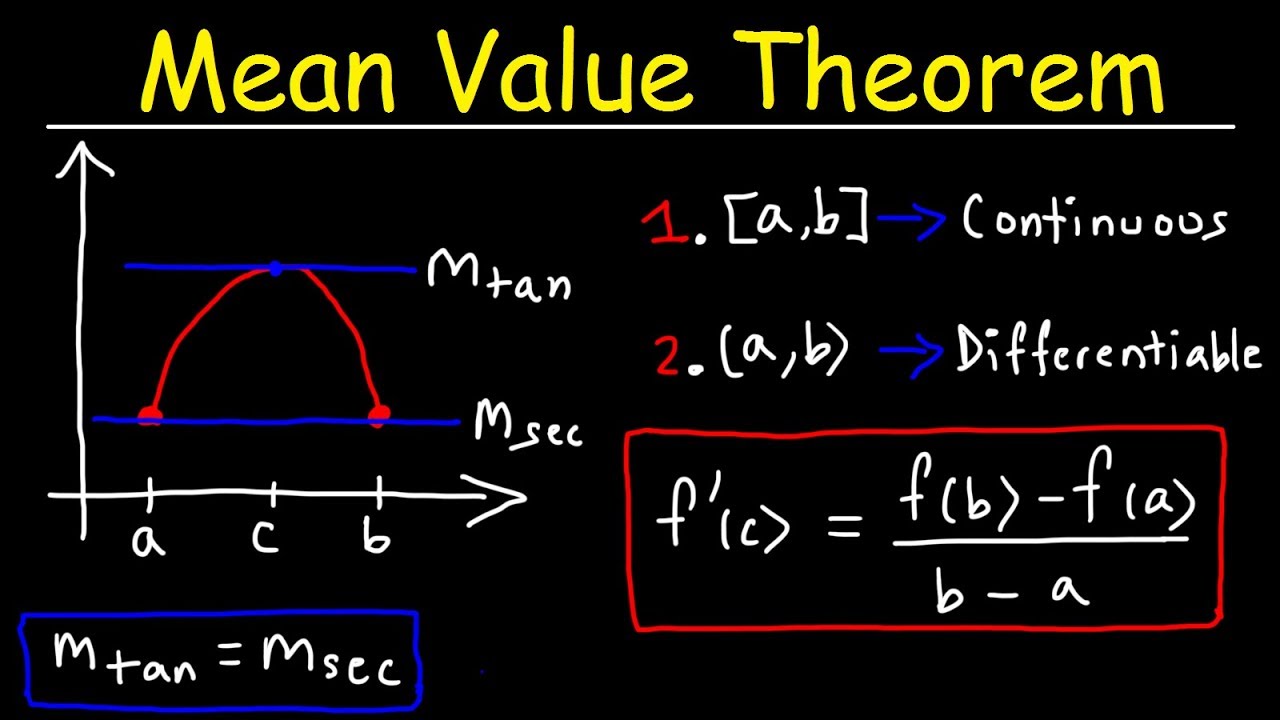
-The Mean Value Theorem states that if a function is continuous on a closed interval [a, b] and differentiable on the open interval (a, b), then there exists at least one point c in the interval (a, b) such that the derivative of the function at that point is equal to the average rate of change of the function over the interval.
How does the Mean Value Theorem relate to Rolle's Theorem?
-The Mean Value Theorem is a more general case of Rolle's Theorem. While Rolle's Theorem requires the function to have the same value at the endpoints, the Mean Value Theorem does not, making it applicable to a wider range of functions.
What does it mean for a function to be differentiable at a point?
-A function is differentiable at a point if it has a derivative at that point, which means the function's graph has a well-defined slope at that location and there is no abrupt change in direction (like a sharp corner).
How can you verify that a function satisfies the conditions of Rolle's Theorem?
-To verify that a function satisfies Rolle's Theorem, you would check that the function is continuous on the interval [a, b], differentiable on the interval (a, b), and that f(a) = f(b). Then, you would find the first derivative of the function, set it equal to zero, and solve for x to find the point(s) where the slope is zero.
What is the significance of a horizontal tangent in the context of Rolle's Theorem?
-A horizontal tangent signifies that the slope of the function at that point is zero. According to Rolle's Theorem, if a function crosses the x-axis at two points and is differentiable on the interval between them, there must be at least one point with a horizontal tangent.
What is the average rate of change of a function over an interval?
-The average rate of change of a function over an interval [a, b] is the difference in the function's values at the endpoints divided by the difference in the x-values, which is (f(b) - f(a)) / (b - a).
How does the slope of the secant line between two points relate to the Mean Value Theorem?
-According to the Mean Value Theorem, if a function is differentiable on an interval (a, b), there exists at least one point c in the interval (a, b) where the derivative of the function at that point is equal to the slope of the secant line between the endpoints a and b.
What is the slope of the secant line between two points (x1, y1) and (x2, y2)?
-The slope of the secant line between two points (x1, y1) and (x2, y2) is calculated as (y2 - y1) / (x2 - x1), provided that x1 is not equal to x2.
Why is it necessary for a function to be continuous and differentiable for Rolle's Theorem and the Mean Value Theorem to apply?
-Continuity ensures the function doesn't have any breaks or jumps in the interval, and differentiability ensures the function has a well-defined slope at each point within the interval. These conditions are necessary to guarantee the existence of a point where the derivative meets the specified criteria of the theorems.
How can you find a point where the derivative of a function equals the slope of the secant line between two points?
-To find such a point, you would first calculate the slope of the secant line between the two points. Then, you would take the first derivative of the function and set it equal to the secant line's slope. Solving for x would give you the point(s) where the derivative equals the secant line's slope.
Outlines
📚 Rolle's Theorem and Mean Value Theorem Explained
This paragraph introduces Rolle's Theorem, which states that if a function is continuous on a closed interval [a, b], differentiable on the open interval (a, b), and crosses the x-axis at some point within this interval, there must exist at least one point c in (a, b) where the derivative (slope) of the function is zero. The video script also connects Rolle's Theorem to the Mean Value Theorem, which asserts that for a differentiable function on an interval, there exists a point where the derivative equals the average rate of change (slope) between any two points on the curve. The explanation is provided in a simplified manner, making it accessible to the audience.
🔍 Verification Process for Rolle's and Mean Value Theorems
The second paragraph delves into the verification process for both Rolle's and Mean Value Theorems. For the Mean Value Theorem, one must find the first derivative, set it equal to zero to find an x-value, and verify that this x-value lies between a and b. For Rolle's Theorem, the process is slightly more complex, as it involves finding the slope between two points on the curve, setting the first derivative equal to this slope, solving for x, and then confirming that the resulting x-value is within the interval. The paragraph emphasizes that these steps are crucial for verifying the theorems' conditions and their applicability to a given function.
Mindmap
Keywords
💡Rolle's Theorem
💡Differentiable
💡Continuous
💡Slope
💡X-axis
💡Mean Value Theorem
💡Secant Line
💡Derivative
💡Horizontal Tangent
💡Verification
💡First Derivative
Highlights
Rolle's Theorem states that if a function crosses the x-axis at two points and is continuous between those points, there must be at least one point between them where the slope is zero.
The theorem is applicable when the function is differentiable between the two points where it crosses the x-axis.
A corollary of Rolle's Theorem is the Mean Value Theorem, which extends the concept to secant lines between two points on a differentiable function.
The Mean Value Theorem implies that there exists a point where the derivative of the function equals the slope of the secant line between any two points.
For verification, one must find the first derivative, set it equal to zero, and solve for x to find the point where the slope is zero.
It is necessary to ensure that the x value obtained is between the two points a and b for Rolle's Theorem to apply.
The slope of the tangent at any point on the curve will be equal to the slope of the secant line between two points if the function is differentiable on that interval.
If the function is differentiable on an interval, the first derivative at some point on that interval must equal the slope between two endpoints of the interval.
The process of verifying Rolle's Theorem involves finding the slope between two points and setting the first derivative equal to that slope.
To apply the Mean Value Theorem, one must check the differentiability of the function on the interval and find the point where the derivative equals the secant's slope.
The theorem guarantees the existence of a horizontal tangent for a function that crosses the x-axis and is differentiable at some point.
The slope of the function at a point will equal the slope of the secant line between two points if the function is continuous and differentiable on that interval.
The verification process for the Mean Value Theorem involves setting the first derivative equal to the slope of the secant and solving for x.
The Mean Value Theorem is a generalization of Rolle's Theorem, applicable to any differentiable function on a closed interval.
The theorems are fundamental in calculus, providing insights into the behavior of differentiable functions and their slopes.
Understanding Rolle's Theorem and the Mean Value Theorem is crucial for analyzing the local behavior of functions and their derivatives.
These theorems are applied in various fields, including physics and engineering, to model and analyze phenomena involving change and motion.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: