2011 Calculus AB free response #4d | AP Calculus AB | Khan Academy
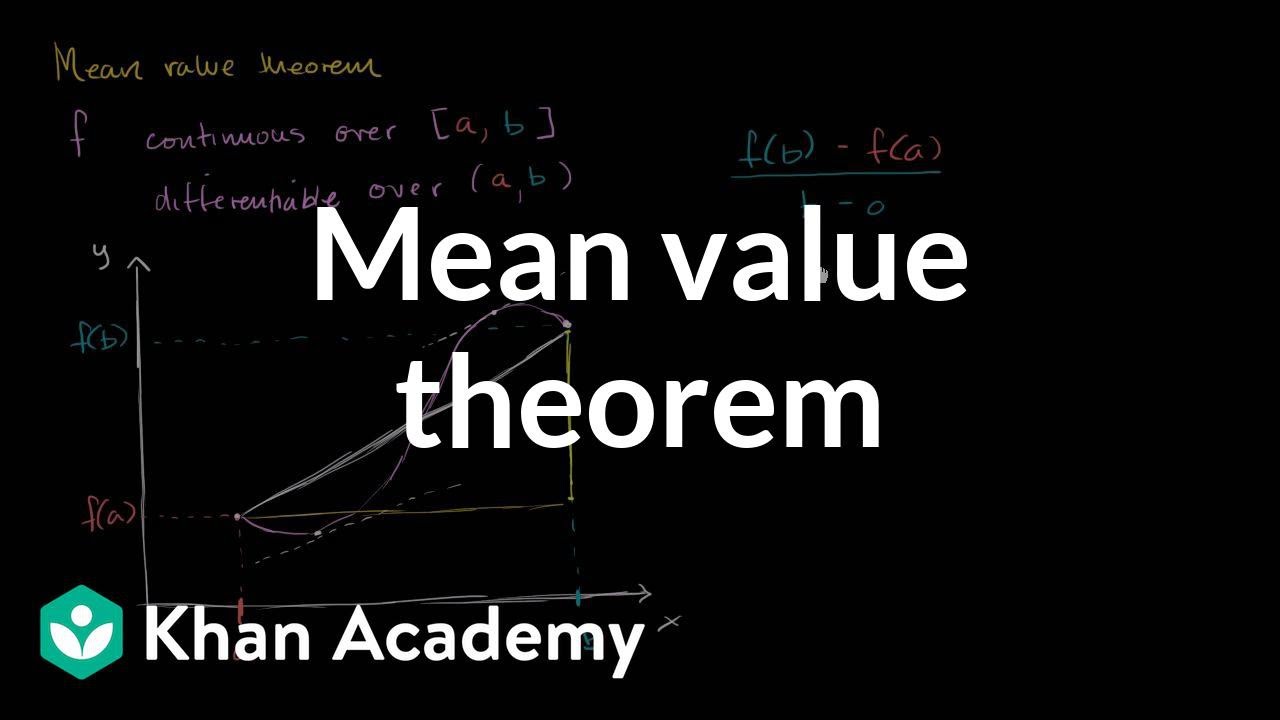
TLDRThe video script discusses the concept of the average rate of change of a function over an interval and its relation to the Mean Value Theorem. It explains that the average rate of change is the slope of the line connecting the end points of the interval, calculated as the change in y over the change in x. The script then clarifies that the Mean Value Theorem, which states there is at least one point in an interval where the derivative equals the average rate of change, does not apply when the function is not differentiable over the entire interval. The example given shows a function that is not differentiable at x=0, hence there is no point where the derivative equals the average rate of change.
Takeaways
- 📈 The average rate of change of a function on an interval is the slope of the line connecting the end points of that interval.
- 🔢 To calculate the average rate of change, you find the change in y (∆y) over the change in x (∆x) between the interval's endpoints.
- 🤔 The given interval is from x ≥ -4 to x ≤ 3, and the average rate of change is calculated as (f(3) - f(-4))/ (3 - (-4)) = -2/7.
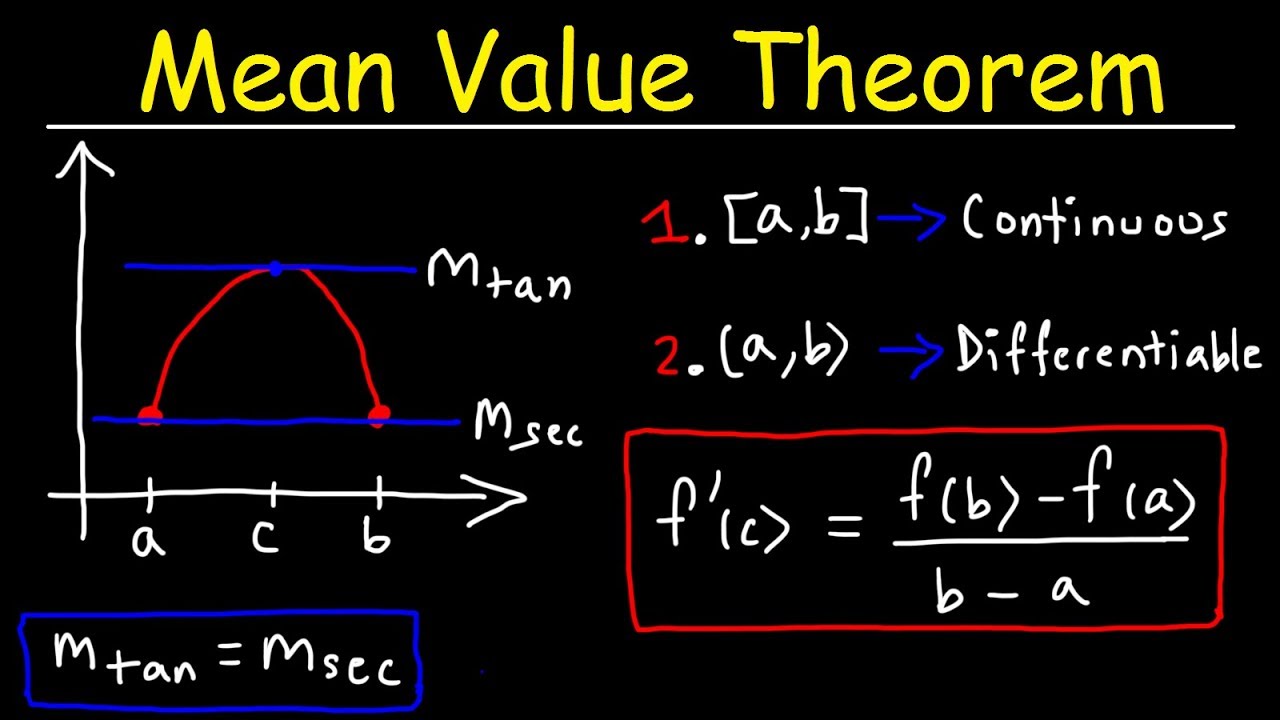
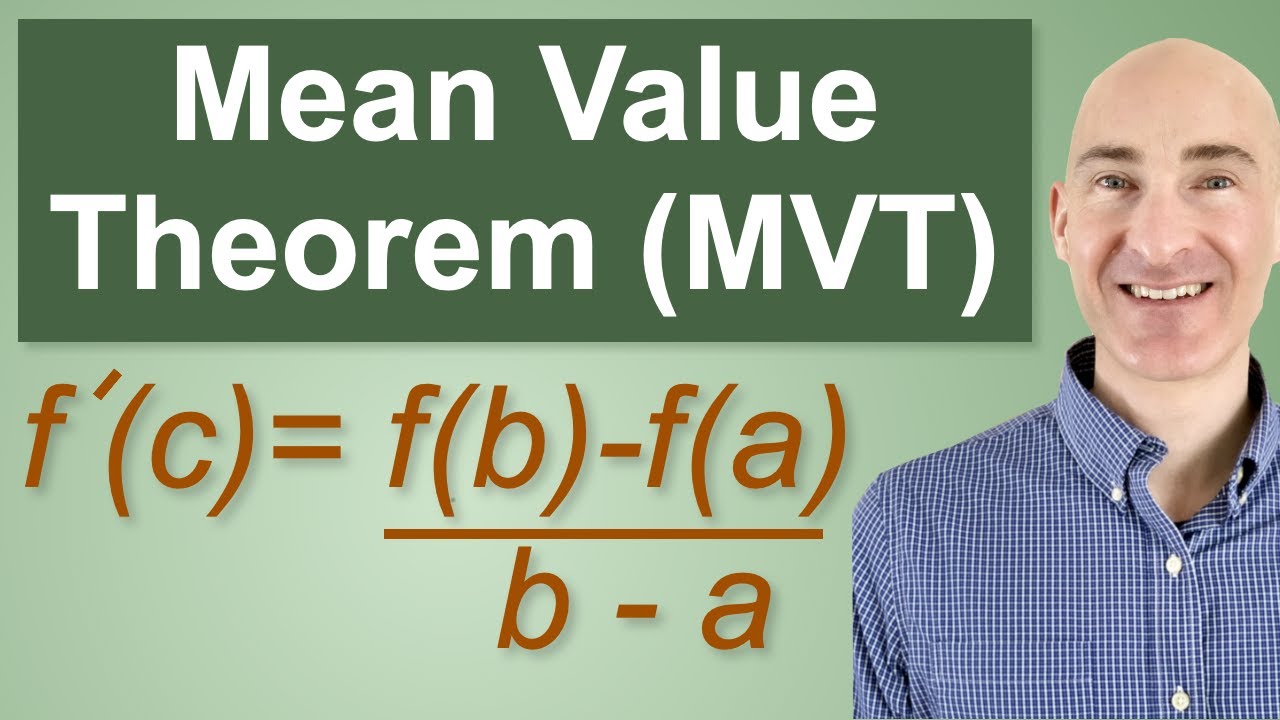
- 🏆 The Mean Value Theorem states that for a differentiable function on an interval, there exists at least one point where the derivative equals the average rate of change.
- 🚫 The function in question is not differentiable at x = 0, which is why there is no point 'c' in the interval where f'(c) equals the average rate of change.
- 🌀 The derivative of the function jumps from a positive value to a negative one at x = 0, without passing through the average rate of change value.
- 🧠 Understanding the concept of differentiability is crucial for applying the Mean Value Theorem and identifying where it may not be applicable.
- 📚 The Mean Value Theorem is a fundamental result in calculus that links the average rate of change of a function on an interval to the behavior of its derivative.
- 🔄 The function's behavior from -4 to 0 and from 0 to 3 demonstrates a discontinuity in the derivative, which is the key to understanding the absence of a point 'c'.
- 📈 The slope of the line connecting the interval's endpoints is a straightforward way to visualize and calculate the average rate of change.
- 🌟 The absence of a point 'c' where the derivative equals the average rate of change highlights the importance of differentiability in the application of calculus theorems.
Q & A
What is the average rate of change of a function on a given interval?
-The average rate of change of a function on a given interval is the slope of the line that connects the end points of the interval. It is calculated by taking the change in y (the difference in function values at the endpoints) and dividing it by the change in x (the difference in the x-values of the endpoints).
How do you calculate the change in x and change in y for the interval from x ≤ -4 to x ≤ 3?
-The change in x is calculated by subtracting the left endpoint's x-value from the right endpoint's x-value, which is 3 - (-4) = 7. The change in y is determined by the difference in function values at the endpoints, which in this case is -3 - (-1) = -2.
What is the slope of the line connecting the endpoints for the interval from x ≤ -4 to x ≤ 3?
-The slope, or the average rate of change, for the interval from x ≤ -4 to x ≤ 3 is the change in y over the change in x, which is -2/7.
What does the Mean Value Theorem state?
-The Mean Value Theorem states that for a differentiable function on a closed interval, there exists at least one point c within the interval where the derivative of the function at c is equal to the average rate of change of the function over that interval.
Why does the statement that there is no point c between -4 and 3 for which f'(c) equals the average rate of change not contradict the Mean Value Theorem?
-The statement does not contradict the Mean Value Theorem because the function is not differentiable at x = 0, which means the theorem does not apply. The derivative is not continuous over the interval, with the slope changing abruptly rather than continuously.
How does the function's behavior on the interval from -4 to 0 differ from its behavior from 0 to 3?
-From -4 to 0, the function has a positive slope, indicating an increase in function values as x increases. However, from 0 to 3, the slope becomes negative, indicating a decrease in function values as x increases. The function's behavior changes abruptly at x = 0, which is why it is not differentiable at that point.
What would need to change about the function for the Mean Value Theorem to apply?
-For the Mean Value Theorem to apply, the function would need to be differentiable, and its derivative would need to be continuous over the entire interval from -4 to 3. This would ensure that there is at least one point within the interval where the derivative equals the average rate of change.
What is the significance of a function being differentiable over an interval?
-If a function is differentiable over an interval, it means that the function has a well-defined derivative at every point in the interval, indicating a smooth and continuous change in slope. This allows for the application of various calculus theorems, such as the Mean Value Theorem, which provide important insights into the function's behavior.
How does the concept of a continuous derivative relate to the Mean Value Theorem?
-A continuous derivative is crucial for the Mean Value Theorem to hold true. The theorem relies on the existence of a point where the derivative, which represents the instantaneous rate of change, is equal to the average rate of change over an interval. If the derivative is discontinuous, as in the case of a jump, this point may not exist, and the theorem does not apply.
What is the implication of the function having a jump in its slope at x = 0?
-The jump in the slope at x = 0 indicates that the function is not smooth at that point, and there is an abrupt change in the rate of change of the function. This discontinuity in the derivative means that the function does not satisfy the conditions required for the Mean Value Theorem to apply, and thus there is no point where the derivative equals the average rate of change over the entire interval.
How can you determine if a function is differentiable at a particular point?
-To determine if a function is differentiable at a particular point, you would check if the limit of the function's derivative exists at that point. If the limit exists and is finite, the function is differentiable at that point. Additionally, the function's graph should not have any sharp corners, vertical tangents, or breaks at that point.
Outlines
📈 Calculating Average Rate of Change and Understanding Mean Value Theorem
This paragraph discusses the concept of the average rate of change of a function over a given interval and its relation to the Mean Value Theorem. The average rate of change is defined as the slope of the line connecting the end points of the interval, which in this case is calculated as the change in y over the change in x (-2/7). It is also explained that the average rate of change is equivalent to the integral of the rate of change (f'(x)) over the interval divided by the change in x. The Mean Value Theorem is introduced, stating that for a differentiable function over an interval, there exists at least one point where the derivative equals the average rate of change. However, it is clarified that the given function is not differentiable at x=0, resulting in a discontinuity in the derivative and thus the Mean Value Theorem does not apply. The absence of a point where the derivative equals the average rate of change is attributed to this non-differentiability.
🔄 Implication of Differentiability on the Mean Value Theorem
This paragraph delves into the hypothetical scenario where the function is differentiable and has a continuous derivative over the interval. It suggests that if the function were differentiable, one would expect to find a point where the slope of the tangent line matches the average rate of change. The discussion emphasizes the importance of differentiability for the Mean Value Theorem to hold true. The function's graph is used to illustrate that if it had a smooth transition, there would likely be multiple points where the derivative equals the average rate of change. The conclusion is that the absence of such a point in the previous example is due to the function's non-differentiability over the entire interval, as the derivative jumps from a positive value to a negative one without passing through the average rate of change.
Mindmap
Keywords
💡average rate of change
💡interval
💡slope
💡endpoints
💡Mean Value Theorem
💡derivative
💡differentiable
💡continuous derivative
💡slope of the line
💡change in x
💡change in y
Highlights
The average rate of change of a function on an interval is the slope of the line connecting the end points of the interval.
The change in x for the interval from -4 to 3 is 7, calculated by 3 - (-4) or counting the points on a number line.
The change in y for the interval from -4 to 3 is -2, as the function goes from -1 to -3.
The slope of the line connecting the end points is calculated as the change in y over the change in x, resulting in a slope of -2/7.
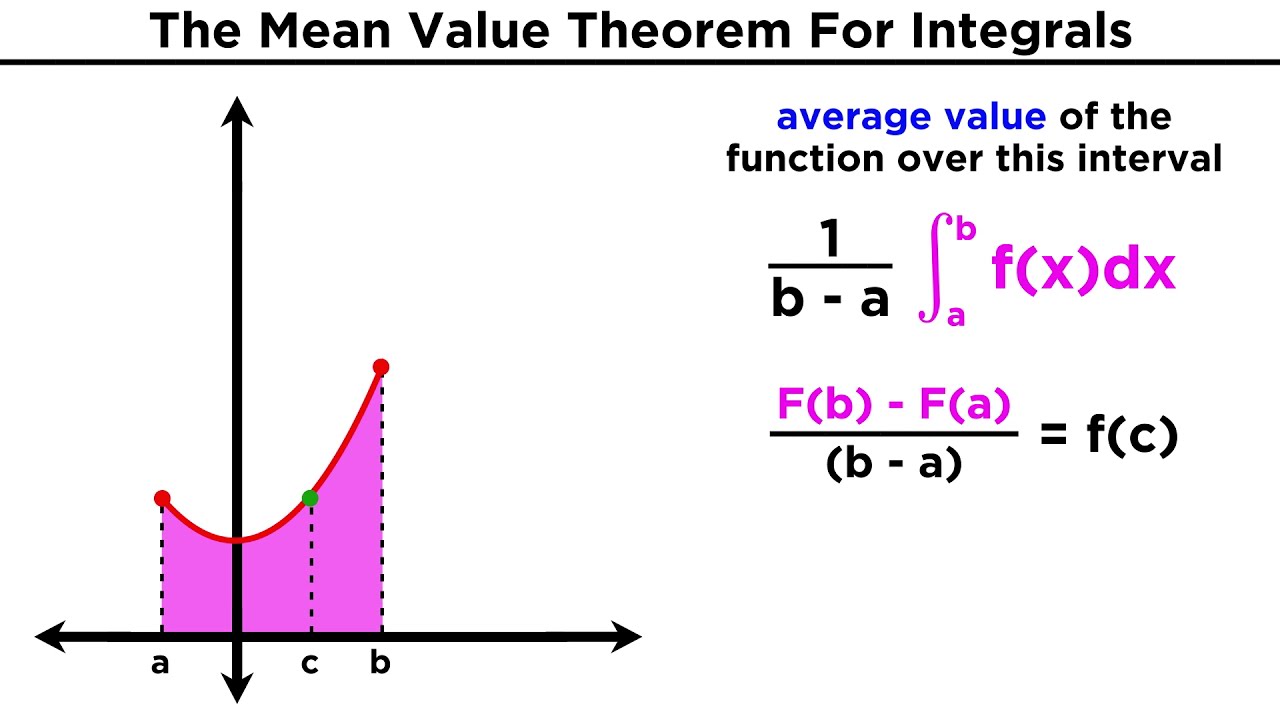
The concept of the average rate of change can also be understood by integrating the rate of change function, f'(x), over the interval and dividing by the change in x.
The Mean Value Theorem states that for a differentiable function on an interval, there exists at least one point where the derivative equals the average rate of change.
The function in the given interval is not differentiable at x=0, which is why the Mean Value Theorem does not apply.
The derivative of the function jumps from a positive value to a negative value at x=0, without being continuous.
If the function were differentiable with a continuous derivative, there would be a point where the slope equals the average rate of change.
The absence of a point where the derivative equals the average rate of change is due to the function's non-differentiability at x=0.
The function's derivative does not go through all values between the positive and negative slopes continuously, which is why there is no point with a derivative equal to the average rate of change.
The Mean Value Theorem is a fundamental concept in calculus that helps in understanding the behavior of differentiable functions on intervals.
The average rate of change is a useful concept for determining the slope of a line connecting two points on the graph of a function.
The process of integrating the rate of change function to find the average rate of change involves understanding the fundamental theorem of calculus.
The example provided illustrates the importance of differentiability in the application of the Mean Value Theorem.
The concept of the slope of a line being equal to the rise over run is a basic principle in geometry and is applied here to understand the average rate of change.
Transcripts
Browse More Related Video

Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.
Mean value theorem | Existence theorems | AP Calculus AB | Khan Academy
The Mean Value Theorem For Integrals: Average Value of a Function
Mean Value Theorem
Mean Value Theorem with Example
Mean value theorem for integrals | AP Calculus AB | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: