12 - Factoring Trinomials & Quadratic Polynomials in Algebra, Part 1 (Learn How to Factor)
TLDRThis algebra lesson focuses on factoring general trinomials, also known as quadratic polynomials. The instructor emphasizes the importance of understanding the process due to its complexity and the need for trial and error. The method involves identifying two binomials whose product equals the given trinomial, considering the multiplication of the first and last terms to determine possible factors, and choosing the correct signs to match the middle term. The lesson aims to build intuition and problem-solving skills, highlighting the necessity of practice to master this fundamental algebraic technique.
Takeaways
- 📚 Factoring trinomials, also known as quadratic polynomials, is a fundamental topic in algebra that requires practice and understanding.
- 🔍 Factoring is the process of finding expressions that multiply together to give the original expression, and it often involves some trial and error.
- 📐 The 'FOIL' method (First, Outer, Inner, Last) is crucial for understanding how to multiply binomials and is essential for factoring trinomials.
- 🔑 When factoring a trinomial, the highest power term (like x^2) is created by multiplying the first terms of the binomials, and the constant term is from the last terms.
- 🤔 Factoring trinomials can be challenging because it's one of the first times in math where the answer isn't always straightforward and requires creative problem-solving.
- 💡 It's important to recognize that there's only one correct pair of binomials that can multiply to give the original trinomial, despite multiple combinations of numbers.
- 📉 The middle term of the trinomial is derived from the addition or subtraction of the inner and outer terms of the binomials, which guides the selection of appropriate numbers for factoring.
- 🚫 Avoid wasting time on combinations that cannot possibly result in the correct middle term, such as 5 and 4 for a middle term of 12, as they cannot be added or subtracted to achieve the desired result.
- 🤓 With practice, you'll develop an intuition for choosing the right numbers and signs for factoring, reducing the need for trial and error.
- 📈 The complexity of factoring increases with larger numbers in the trinomial, offering more combinations but also more opportunities for incorrect choices.
- 🎯 The goal of practice is to become proficient in factoring trinomials, learning to make educated guesses and quickly eliminate impossible options to find the correct factorization.
Q & A
What is the main topic of the algebra lesson in the transcript?
-The main topic of the algebra lesson is factoring trinomials, specifically general trinomials, which are also known as quadratic polynomials.
What is the significance of the term 'FOIL' in the context of this lesson?
-FOIL is an acronym used to remember the process of multiplying binomials. It stands for First, Outer, Inner, Last, and it helps in understanding the distribution of terms when multiplying two binomials, which is essential for factoring trinomials.
Why might students initially struggle with factoring trinomials according to the transcript?
-Students might struggle with factoring trinomials because it often requires a bit of creativity and trial and error, unlike many other math problems where there is a straightforward method to find the answer.
What is the general form of a trinomial that can be factored into two binomials?
-A general trinomial that can be factored into two binomials has the form of ax^2 + bx + c, where a, b, and c are constants, and x is the variable.
Why is it important to understand that the first term of the trinomial comes from the multiplication of the first terms of the binomials?
-It is important because knowing that the first term is derived from the multiplication of the first terms helps in correctly setting up the binomials when factoring the trinomial.
How does the constant term in the trinomial relate to the last terms of the binomials?
-The constant term in the trinomial is the result of the multiplication of the last terms of the two binomials.
What is the purpose of trying different combinations of numbers for the last term of the trinomial?
-Trying different combinations of numbers for the last term helps in finding the correct pair that, when multiplied, gives the constant term of the trinomial and can also be added or subtracted to give the linear coefficient.
What is the role of signs when choosing the terms for the binomials in the factored form of a trinomial?
-The signs determine whether the middle term of the trinomial will be positive or negative. The correct signs must be chosen so that when the binomials are multiplied, they yield the original trinomial.
Why is it suggested to abandon certain number combinations early in the factoring process?
-It is suggested to abandon certain number combinations early because they cannot possibly add or subtract to give the middle term of the trinomial, thus saving time and avoiding unnecessary calculations.
What is the final step in verifying the factored form of a trinomial?
-The final step is to multiply the binomials to see if they yield the original trinomial, ensuring that the first terms give the squared term, the outer and inner terms give the linear term, and the last terms give the constant term.
Outlines
📚 Introduction to Factoring Trinomials
This paragraph introduces the concept of factoring trinomials, which are general quadratic polynomials. The speaker emphasizes the importance of understanding the process, as it is a central topic in algebra. It explains that factoring involves finding expressions that multiply to give the original expression. The paragraph also highlights the challenges students face with this topic due to its non-linear problem-solving nature, contrasting it with more straightforward mathematical procedures. The speaker reassures students that it's normal to require trial and error and that this approach is common in higher mathematics as well.
🔍 Understanding the FOIL Method and Trinomial Factoring
The speaker reviews the FOIL (First, Outer, Inner, Last) method for multiplying binomials, which is essential for understanding trinomial factoring. It explains how to distribute terms when multiplying binomials to achieve a trinomial result. The paragraph then transitions into the reverse process, factoring trinomials, which involves finding two binomials that, when multiplied, yield the original trinomial. The importance of recognizing the relationship between the terms of the trinomial and the binomials is highlighted, with a focus on the signs and the middle terms of the binomials.
📉 Factoring Trinomials with Positive Middle Terms
This section delves into the process of factoring trinomials with a positive middle term. The speaker uses the example of 'x squared plus 3x plus 2' and guides through the thought process of selecting the correct numbers for the binomials that will multiply to the given trinomial. It discusses the trial and error involved in choosing the correct signs for the binomials and confirms the correct factored form through multiplication.
📈 Factoring Trinomials with Negative Middle Terms
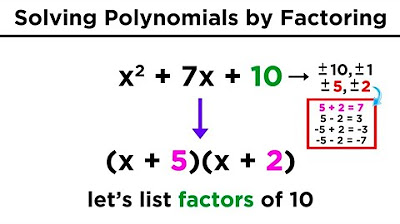
The paragraph explores factoring trinomials that have a negative middle term, using 'x squared minus 9x plus 14' as an example. It demonstrates the process of determining the correct pair of numbers that, when multiplied, give the constant term and, when added or subtracted, yield the middle term. The speaker illustrates the trial and error process, showing how incorrect sign choices lead to wrong results and emphasizes the importance of choosing signs that align with the original trinomial's terms.
🔎 The Thought Process in Factoring Trinomials
Here, the speaker elaborates on the thought process required for factoring trinomials, especially when the numbers involved are larger, leading to more potential combinations. The importance of choosing numbers that not only multiply to the constant term but also can be added or subtracted to achieve the middle term is stressed. The speaker uses examples to show how to eliminate impossible combinations and focus on those that have a chance of being correct.
📘 Final Thoughts on Factoring General Trinomials
The final paragraph wraps up the lesson by summarizing the key points of factoring general trinomials. It reiterates the process of identifying the correct numbers for the binomials and choosing the appropriate signs. The speaker encourages practice and developing an intuition for making educated guesses to avoid wasting time on incorrect paths. The paragraph concludes with an invitation to continue learning through further lessons, promising more practice with increasingly complex trinomials.
Mindmap
Keywords
💡Factoring
💡Trinomials
💡Quadratic Polynomials
💡FOIL
💡Special Trinomials
💡Trial and Error
💡Polynomials
💡Binomials
💡Algebra
💡Practice
Highlights
Introduction to factoring trinomials, also known as quadratic polynomials, as a central topic in algebra.
Explanation of factoring as finding expressions that multiply to the original expression.
Discussion on the challenges of factoring trinomials compared to more straightforward math problems.
Emphasis on the need for creativity and trial-and-error in factoring trinomials.
Importance of understanding FOIL (First, Outer, Inner, Last) for multiplying binomials as a foundation for factoring.
The process of reversing FOIL to factor a trinomial into two binomials.
Identifying the first terms of the binomials from the squared term in the trinomial.
Determining the last terms of the binomials by finding factors of the constant term in the trinomial.
The necessity of choosing the correct signs between the terms to achieve the correct middle term in the trinomial.
Demonstration of factoring the trinomial x^2 + 3x + 2 and the thought process behind it.
Preview of the upcoming lessons focusing on increasing the complexity of factoring trinomials.
The concept that factoring trinomials requires both choosing the right numbers and the right signs.
Illustration of the process with the trinomial x^2 + 9x + 14, including the trial-and-error aspect.
Mental exercise to quickly assess the feasibility of certain numbers for the middle term without full calculations.
The importance of choosing numbers that can potentially add up to the middle term of the trinomial.
Example of factoring the trinomial R^2 - 12R + 20, showcasing the thought process and sign choices.
Final emphasis on practicing factoring to develop intuition and avoid wasting time on incorrect guesses.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: