Algebra Introduction - Basic Overview - Online Crash Course Review Video Tutorial Lessons

The Organic Chemistry Tutor

12 Aug 201678:13

EducationalLearning

32 Likes 10 Comments

TLDRThis video script offers an in-depth exploration of algebraic concepts, focusing on the manipulation of like terms, polynomials, and exponents. It delves into the addition and subtraction of polynomials, the multiplication of monomials and trinomials, and the use of FOIL for binomials. The script also addresses properties of exponents, including multiplication, division, and raising to a power. Further, it covers solving equations, factoring, and graphing linear equations, as well as deriving equations of lines given points and slopes. The comprehensive guide aims to enhance the viewer's understanding and problem-solving skills in algebra.

Takeaways

📚 The concept of like terms in algebra allows for the addition and subtraction of terms with the same variable part, such as 5x and 4x combining to form 9x.
🔢 When dealing with polynomials, addition and subtraction of terms can be performed by combining like terms, for example, 3x and 5x can be added to result in 8x, while non-like terms like 3x and 4y cannot be combined.
🛠️ The multiplication of monomials by polynomials involves distributing the monomial across each term of the polynomial, as shown with 7x multiplying (x^2 + 2x + 3) resulting in (7x^3 + 14x^2 + 21x).
📈 The process of multiplying binomials, known as FOIL (First, Outer, Inner, Last), combines each term of the first binomial with each term of the second, such as (3x - 4)(x + 7) becoming (3x^2 + 21x - 4x - 28).
🔄 Understanding and applying the properties of exponents is crucial in algebra, including the rules for multiplying (adding exponents), dividing (subtracting exponents), and raising a power to a power (multiplying exponents).
🌟 Solving equations often involves isolating the variable, using operations such as addition, subtraction, multiplication, and division to transform the equation until the variable is alone, as in 2x - 5 = 11 where x is found by dividing both sides by 2, resulting in x = 8.
📊 Factoring is a method to break down a polynomial into simpler expressions, which can be used to solve equations, such as factoring x^2 - 5x + 6 into (x - 2)(x - 3) to find the solutions x = 2 and x = 3.
📐 The graphing of linear equations can be done using the slope-intercept form (y = mx + b), where m is the slope and b is the y-intercept, allowing for a visual representation of the equation on a coordinate plane.
🔍 Converting between different forms of a line equation, such as point-slope, slope-intercept, and standard form, is essential for various applications, including finding parallel or perpendicular lines and graphing.
🌐 When graphing, finding the x and y-intercepts can quickly provide points to plot on the coordinate plane, which, along with the slope, helps in drawing the line representing the equation.
🔧 Solving cubic and quadratic equations can be done through factoring, the quadratic formula, or by applying techniques like the difference of squares, offering multiple methods to find the value(s) of x.

Q & A

What are like terms and when can they be combined?
-Like terms are terms that have the same variables raised to the same power. They can be combined by adding their coefficients when they appear in a polynomial expression.
How do you combine like terms?
-To combine like terms, you add the coefficients of the terms while keeping the variable and its exponent unchanged. For example, 5x + 4x combines to 9x.
What is the rule for combining terms with different exponents?
-Terms with different exponents cannot be combined directly as they are not like terms. You can only combine terms with the same exponent by adding their coefficients.
Can you give an example of combining terms with the same radical expression?
-Yes, for example, 3√2 + 5√7 + 8√2 + 3√7 can be combined to 11√2 + 8√7 since √2 and √7 are distinct radical expressions and can be combined separately.
How do you multiply a monomial by a trinomial?
-To multiply a monomial by a trinomial, you distribute the monomial across each term inside the trinomial. For example, 7x * (x^2 + 6x + 5) requires multiplying 7x by each term within the parentheses.
What is the process called when you multiply a binomial by another binomial?
-The process is called FOIL, which stands for First, Outer, Inner, Last. It involves multiplying each term in the first binomial by each term in the second binomial to get the expanded form of the product.
What is a polynomial?
-A polynomial is a mathematical expression consisting of multiple terms, which can include variables and coefficients. It can represent algebraic relationships between these terms.
How do you simplify an expression with negative exponents?
-Negative exponents can be simplified by converting them to positive exponents and moving the variable to the denominator. For example, x^(-3) becomes 1/x^3.
What is the rule for multiplying powers with the same base?
-When multiplying powers with the same base, you add the exponents. For example, x^3 * x^4 equals x^(3+4), which simplifies to x^7.
How do you solve a linear equation with one variable?
-To solve a linear equation with one variable, you isolate the variable by applying inverse operations (such as subtraction, addition, multiplication, or division) to both sides of the equation until you find the value of the variable.

Outlines

00:00

📘 Introduction to Algebra Concepts

This paragraph introduces several fundamental concepts in algebra, such as combining like terms, understanding polynomials, and basic rules for adding and subtracting polynomial expressions. The explanation includes examples of combining terms with the same variable and exponent, as well as the importance of recognizing like terms in order to perform valid arithmetic operations.

05:01

📘 Polynomials and Monomials

This section delves deeper into the definition of polynomials, which are expressions consisting of multiple terms. It differentiates between monomials, which are single-term expressions, and binomials and trinomials, which contain two and three terms respectively. The paragraph also explains how to multiply a monomial by a trinomial, providing a step-by-step breakdown of the process.

10:05

📘 Multiplying Polynomials

The focus of this paragraph is on the multiplication of polynomials, specifically binomials and trinomials. It introduces the method of FOIL (First, Outer, Inner, Last) for multiplying binomials and demonstrates how to apply this technique through examples. Additionally, it covers the multiplication of a binomial by a trinomial and the importance of combining like terms after each multiplication step.

15:06

📘 Exponents and Their Properties

This section discusses the properties of exponents, including the rules for multiplying and dividing powers with the same base, as well as raising a power to another power. It clarifies the concept of negative exponents and their equivalence to positive exponents when the base is moved to the denominator. The explanation is supplemented with examples to illustrate these rules and their applications.

20:06

📘 Solving Equations

This paragraph is centered around solving algebraic equations, both linear and quadratic. It begins with simple linear equations, explaining how to isolate the variable 'x' by performing inverse operations. The explanation extends to quadratic equations, introducing factoring as a method to solve them, as well as the quadratic formula for cases where factoring is not straightforward.

25:07

📘 Factoring and Solving Trinomials

The content in this paragraph is dedicated to factoring and solving trinomials, particularly those that are not factorable by inspection. It explains how to use the factoring by grouping method, applying it to various examples, and how to solve for 'x' by setting each factor equal to zero. The paragraph also touches on the quadratic equation and its application in finding solutions.

30:09

📘 Cubic Functions and Graphing

This section introduces cubic functions and their solutions, discussing how to factor by grouping for expressions with a common factor and those that resemble a difference of squares. It also covers the method of graphing linear equations in slope-intercept form, explaining the steps to plot the y-intercept and use the slope to find additional points for drawing the graph.

35:11

📘 Standard Form Equations and Intercepts

This paragraph focuses on graphing equations in standard form (ax + by = c) by finding the x and y intercepts. It explains the process of converting the equation to slope-intercept form to find the y-intercept and setting y to zero to find the x-intercept. The explanation also briefly touches on converting equations to point-slope form and back to standard form.

40:11

📘 Writing Equations of Lines

The final paragraph discusses various methods for writing the equations of lines, including point-slope form, slope-intercept form, and standard form. It explains how to derive the equation of a line given a point and a slope, how to convert between different forms, and how to find the equation of a line parallel or perpendicular to another line through a given point.

Mindmap

Keywords

💡Algebra

Algebra is a branch of mathematics that focuses on the study of mathematical symbols and the rules for manipulating these symbols. In the context of the video, algebra is used to solve problems involving variables, coefficients, and expressions, such as finding the value of 'x' in an equation.

💡Like Terms

Like terms are terms in a mathematical expression that have the same variables raised to the same powers. They can be combined by adding or subtracting their coefficients. In the video, combining like terms is demonstrated with examples like 5x + 4x = 9x.

💡Polynomials

Polynomials are mathematical expressions consisting of variables and coefficients, involving only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. The video explains how to add, subtract, and multiply polynomials, and how to identify monomials, binomials, and trinomials within them.

💡Exponents

Exponents are a mathematical notation that indicate the number of times a base is multiplied by itself. The video discusses the properties of exponents, such as how to multiply and divide powers with the same base, and how to raise a power to another power.

💡FOIL

FOIL is a method for multiplying two binomials (expressions with two terms). It stands for First, Outer, Inner, and Last, which refers to the four terms that are created when two binomials are multiplied. The video demonstrates how to use FOIL to find the product of binomials, such as (3x - 4)(x + 2).

💡Graphing

Graphing is the process of plotting points on a coordinate plane to visualize the relationship between two variables. In the video, graphing is used to visually represent linear equations, such as y = 2x - 1, by finding the y-intercept and using the slope to determine additional points on the line.

💡Solving Equations

Solving equations involves finding the values of the unknowns (variables) that make the equation true. The video covers various techniques for solving equations, such as isolating the variable, using inverse operations, and factoring.

💡Quadratic Equations

Quadratic equations are second-degree polynomial equations of the form ax^2 + bx + c = 0. The video discusses solving these equations by factoring, using the quadratic formula, or finding the square roots of the solutions.

💡Slope-Intercept Form

The slope-intercept form of a linear equation is y = mx + b, where m is the slope of the line and b is the y-intercept, the point where the line crosses the y-axis. The video explains how to convert this form to standard form and how to use it for graphing.

💡Standard Form

The standard form of a linear equation is Ax + By = C, where A, B, and C are constants. This form is useful for comparing equations and finding intercepts. The video shows how to convert equations from slope-intercept form to standard form.

💡Complex Fractions

Complex fractions are expressions that involve a fraction within another fraction. They are simplified by multiplying the numerator and denominator by the least common denominator to eliminate the inner fractions. The video demonstrates how to simplify complex fractions through this process.

💡Difference of Squares

The difference of squares is a factoring technique used for quadratic expressions of the form a^2 - b^2, which can be factored into (a + b)(a - b). The video explains how to use this method to solve equations and factor expressions, particularly when the expression is a difference of two squares.

Highlights

Introduction to common algebra concepts.

Explanation of like terms and combining coefficients.

Addition of unlike terms with examples.

Combining like terms in complex expressions.

Addition of radical expressions with like radicals.

Multiplication of monomials by polynomials.

Explanation of polynomials and their structure.

FOIL method for multiplying binomials.

Simplification of expressions using exponent rules.

Division of monomials and polynomials.

Solving linear equations by isolating the variable.

Use of the quadratic formula for solving equations.

Factoring quadratic expressions using the difference of squares.

Solving cubic equations by factoring.

Graphing linear equations in slope-intercept form.

Conversion between different forms of linear equations.

Writing equations of lines given points and slopes.

Graphing equations from standard form.

Creating equations of parallel and perpendicular lines.

Transcripts

Browse More Related Video

College Algebra Introduction Review - Basic Overview, Study Guide, Examples & Practice Problems

Ch. 1.3 Algebraic Expressions

Algebra Final Exam Review

Math Videos: How To Learn Basic Arithmetic Fast - Online Tutorial Lessons

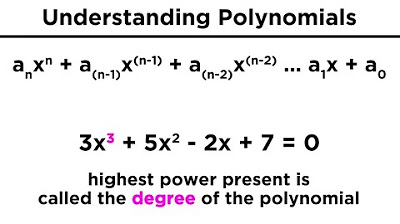
Introduction to Polynomials

12 - Factoring Trinomials & Quadratic Polynomials in Algebra, Part 1 (Learn How to Factor)

Related Tags

Algebra Basics Math Education Equation Solving Graphing Techniques Polynomials Exponents Linear Equations Quadratic Formula Factoring Math Tutorial