Factoring Review

Stacy Colaiacovo

7 Feb 202331:13

EducationalLearning

32 Likes 10 Comments

TLDRThe video lesson focuses on the mathematical skill of factoring, which is essential for solving various types of equations, including new ones in trigonometry. The instructor begins by defining factoring as the process of rewriting a polynomial as a product of two or more polynomials, which is the reverse of multiplication. The lesson emphasizes the importance of having terms in descending order, or standard form, before factoring. It outlines the first step in factoring, which is to factor out the greatest common factor (GCF) if possible. The instructor then discusses different factoring techniques based on the number of terms in the expression: grouping for four terms, the 'Bottoms Up' method for three terms, and recognizing patterns like difference of squares or sum/difference of cubes for two terms. The 'Bottoms Up' method is explained in detail for factoring trinomials, which involves multiplying the first and last coefficients, finding factors of the product that add up to the middle coefficient, dividing by the first coefficient, reducing fractions, and reading the factors from the bottom up. The video also covers factoring differences and sums of squares and cubes, and concludes by noting that if no factoring is possible, the polynomial is considered prime. The lesson serves as a comprehensive review of factoring techniques, preparing students to apply these skills to trigonometric expressions.

Takeaways

📚 Factoring is a fundamental skill used across various math classes, including those involving trigonometric equations.
🔍 Factoring involves rewriting a polynomial as a product of two or more polynomials, which is the reverse process of multiplying them out.
📉 Before factoring, it's important to ensure that terms are in descending order, also known as standard form for polynomials.
🧮 The first step in factoring is to factor out the greatest common factor (GCF) if possible, which simplifies the expression.
🤔 The method of factoring continues based on the number of terms in the expression: grouping for four terms, Bottoms Up for three terms, and looking for differences of squares or sums/differences of cubes for two terms.
🔢 In the Bottoms Up method for factoring trinomials, you multiply the first and last coefficients, find factors of the product that add up to the middle coefficient, then divide by the first coefficient and reduce fractions.
🔴 When factoring a difference of squares, you use conjugate binomials, which are binomials that differ only by the sign between them.
🟢 For sums of squares, no factoring is possible, and such expressions are considered prime.
📈 The process for factoring cubes is similar to that for squares, but you work with cube roots and the formula is adjusted for the cube terms.
✅ Factoring can be checked by multiplying the factors back out to see if they yield the original polynomial.
🚫 If no factoring is possible, the polynomial is prime, which means it cannot be broken down into simpler polynomial factors.

Q & A

What is the primary purpose of factoring in the context of this lesson?
-The primary purpose of factoring in this lesson is to review the process and prepare for solving new types of equations, specifically trigonometric equations, which will require the use of factoring with trigonometric identities.
What is the reverse process of factoring called?
-The reverse process of factoring is called multiplying. It involves taking two or more polynomials and combining them to form a single polynomial.
What should be the order of terms in a polynomial before factoring?
-Before factoring, the terms in a polynomial should be in descending order, also known as standard form, with the highest degree terms first and the constant term last.
What is the first step in the factoring process?
-The first step in the factoring process is to factor out the greatest common factor (GCF) if possible.
What is the term used for the process of factoring when there are four terms in the expression?
-When there are four terms in the expression, the process of factoring is referred to as 'grouping'.
How many different methods are mentioned for factoring trinomials with three terms?
-The instructor mentions about a dozen different methods that are taught for factoring trinomials with three terms.
What is the name of the process used for factoring trinomials with three terms as described in the script?
-The process used for factoring trinomials with three terms, as described in the script, is called 'Bottoms Up'.
What is a 'difference of squares' and how is it factored?
-A 'difference of squares' is an expression where two squared terms are subtracted from each other, like A^2 - B^2. It can be factored into the product of two binomials, (A + B)(A - B), which are known as conjugate binomials.
What is the condition for a polynomial to be considered prime in the context of factoring?
-A polynomial is considered prime if it cannot be factored into a product of two or more polynomials with integer coefficients.
What happens when you try to factor a sum of squares?
-A sum of squares cannot be factored using the same methods as a difference of squares. It remains prime because there is no combination of factors that can result in a sum of squares without producing additional terms in the expression.
How does the degree of a polynomial affect the factoring process?
-The degree of a polynomial affects the factoring process because it determines the structure of the binomials used in the factoring. For example, if the polynomial has an even degree, it can be split evenly between the binomials, but if it has an odd degree, the factoring process may not accommodate it in the same way.

Outlines

00:00

📚 Introduction to Factoring and Trigonometric Equations

The video begins with an introduction to factoring as a fundamental skill in mathematics, essential for solving various types of equations, including the upcoming focus on trigonometric equations. Factoring is described as the process of rewriting a polynomial as a product of two or more polynomials, which is the reverse of multiplication. The importance of having terms in descending order (standard form) is emphasized, and the first step in factoring is to identify and factor out the greatest common factor (GCF) if possible. The video also mentions that after GCF, the factoring process will depend on the number of terms in the expression.

05:01

🔢 Factoring Techniques: GCF, Grouping, and Bottoms Up

The video continues by explaining different factoring techniques based on the number of terms in the expression. For expressions with four terms, the grouping method is introduced, where terms are grouped and GCFs are factored out from each group. For three terms, the video presenter prefers a method called 'Bottoms Up,' which involves multiplying the first and last terms, finding factors of the product that add up to the middle term, and then using these factors to form binomials. The process is demonstrated with examples, emphasizing the need to reduce fractions when possible and read the factors from the bottom up.

10:02

🔍 Factoring Trinomials and Polynomials with Two Terms

The presenter discusses factoring trinomials using the 'Bottoms Up' method in detail, providing a step-by-step guide on how to find the correct factors and reduce fractions. The video also covers factoring polynomials with two terms, which can be factored if there's a GCF or if the terms represent a difference of squares or a sum/difference of cubes. The concept of conjugate binomials is introduced for factoring differences of squares, and the importance of having a negative product to maintain the middle terms is highlighted.

15:02

📉 Factoring Cubes and the Formula Approach

The video moves on to factoring expressions involving cubes, using both sum and difference formulas. The presenter explains the process of finding the cube roots of the coefficients and using them as the bases for the binomial factors. The formula for factoring cubes is demonstrated, and it's noted that the process is similar to factoring squares, but with cubes. The video emphasizes the need to accommodate the degree of the polynomial in the binomial factors and checks the work by multiplying the factors to ensure they yield the original polynomial.

20:03

🔐 Prime Polynomials and the Limitations of Factoring

The final paragraph addresses the scenario where a polynomial cannot be factored using the previously discussed methods. In such cases, the polynomial is termed 'prime.' The video provides examples of prime polynomials, including trinomials and expressions that are sums of squares, which cannot be factored over the integers. The presenter reassures viewers that while some factoring processes might be challenging, the foundation laid in the video will be crucial for future work with trigonometric expressions.

25:03

🚀 Conclusion and Application to Trigonometric Expressions

In conclusion, the video summarizes the factoring processes covered and reassures viewers that these techniques will be applied to trigonometric expressions in subsequent lessons. The importance of understanding factoring as a foundational skill is reiterated, and viewers are encouraged to reach out for extra help if needed. The video ends with a reminder that the next lesson will involve applying these factoring methods to trig expressions, emphasizing the continuity of mathematical concepts.

Mindmap

Keywords

💡Factoring

Factoring in mathematics refers to the process of breaking down an expression, such as a polynomial, into a product of simpler expressions, or factors, that when multiplied together result in the original expression. In the context of the video, factoring is described as the reverse process of multiplication, essential for simplifying and solving equations, especially when dealing with trigonometric identities and expressions. The instructor emphasizes the foundational importance of factoring across different mathematical areas, preparing the students to apply it to more complex trigonometric equations.

💡Trigonometric Equations

Trigonometric equations involve variables within trigonometric functions like sine, cosine, and tangent. The video script introduces solving trigonometric equations as a key application area for the reviewed factoring techniques. By mastering factoring, students can more effectively manipulate and solve these types of equations, which are common in advanced mathematics and applications involving periodic phenomena.

💡Polynomial

A polynomial is a mathematical expression consisting of variables (also called indeterminates) and coefficients, that involves only the operations of addition, subtraction, multiplication, and non-negative integer exponentiation of variables. In the video, polynomials are central to the discussion on factoring, as the presenter describes how to decompose a polynomial into products of polynomials of lower degrees, thereby simplifying the original expression.

💡Greatest Common Factor (GCF)

The Greatest Common Factor, or GCF, is the largest factor that divides two or more numbers or terms. In the context of the video, the concept of the GCF is crucial for the initial step in factoring expressions. The presenter uses examples to show how identifying and factoring out the GCF can simplify the process of breaking down an expression into more manageable parts.

💡Difference of Squares

A difference of squares is a specific type of algebraic expression that takes the form a^2 - b^2 and can be factored into (a + b)(a - b). The video highlights this as a common pattern to recognize when factoring polynomials, especially after a GCF has been factored out, simplifying the equation into products of linear factors.

💡Grouping

Grouping is a factoring technique used to rearrange and divide a polynomial into groups that can each be factored separately. This method is typically used when a polynomial consists of four or more terms, as explained in the video. The instructor demonstrates how grouping helps in extracting common factors from different groups, thereby breaking the polynomial into simpler binomial products.

💡Trinomials

Trinomials are polynomials with three terms. The video script discusses various methods for factoring trinomials, like 'Bottoms Up', a method the presenter prefers due to its efficiency. Factoring trinomials is portrayed as a critical skill for simplifying and solving equations, particularly in preparing to work with trigonometric functions.

💡Conjugate Binomials

Conjugate binomials refer to a pair of two-term polynomials that are identical except for the opposite signs between their terms, typically in the form (a + b) and (a - b). These are used to factor expressions like the difference of squares, as they eliminate the middle term when multiplied. The video uses this concept to explain how to factor and simplify expressions containing squares.

💡Standard Form

In algebra, standard form refers to arranging the terms of a polynomial in descending order of their degrees. The video emphasizes the importance of starting the factoring process with polynomials in standard form to simplify and correctly apply factoring techniques. This arrangement helps in systematically reducing complex expressions to simpler factors.

💡Binomials

Binomials are polynomials that contain only two terms. The video discusses factoring expressions into binomials using various methods, including the difference of squares and grouping. Understanding how to manipulate binomials is essential for algebraic simplification and solving equations, providing a foundation for more complex mathematical operations involving polynomials.

Highlights

Factoring is a fundamental skill used across various math classes, including computational math and trigonometry.

Factoring involves rewriting a polynomial as a product of two or more polynomials, which is the reverse of the multiplication process.

When factoring, terms should be arranged in descending order, or standard form, starting with the highest degree terms.

The first step in factoring is to factor out the greatest common factor (GCF) if possible.

After factoring out the GCF, sometimes the process ends, continues with further factoring, or proceeds without a GCF.

Grouping is a factoring process used for expressions with four terms, involving separating terms and factoring out GCFs.

The 'Bottoms Up' method is introduced for factoring trinomials, focusing on multiplying the first and last terms and finding factors that add up to the middle term.

For trinomials with an even degree, the degree can be split between the two binomial factors to accommodate the higher degree.

A difference of squares can always be factored into conjugate binomials, which are binomials with the same bases and opposite signs.

A sum of squares is always a prime polynomial and cannot be factored further.

When factoring cubes, similar to squares, the process involves identifying the bases of the cubes and applying a formula for factoring.

Prime polynomials are those that cannot be factored any further using the available methods.

The ability to factor expressions is crucial for solving more complex equations, including trigonometric equations.

The process of factoring can be checked by multiplying the factors back together to ensure they yield the original polynomial.

Factoring techniques will be applied to trigonometric expressions in subsequent lessons, building on the foundation reviewed.

The 'Bottoms Up' method provides an efficient way to factor trinomials by creating binomial factors from reduced fractions.

Understanding the process of factoring is essential for recognizing when a polynomial is prime and cannot be factored further.

Transcripts

Browse More Related Video

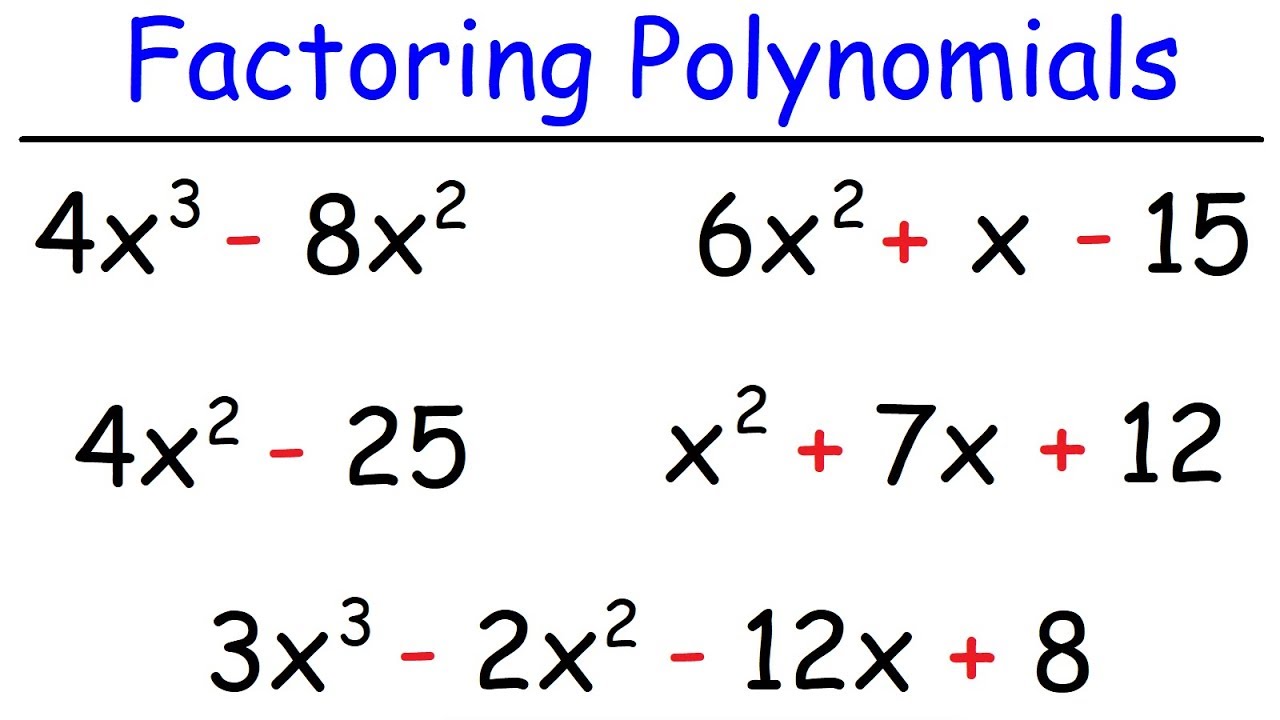
How To Factor Polynomials The Easy Way!

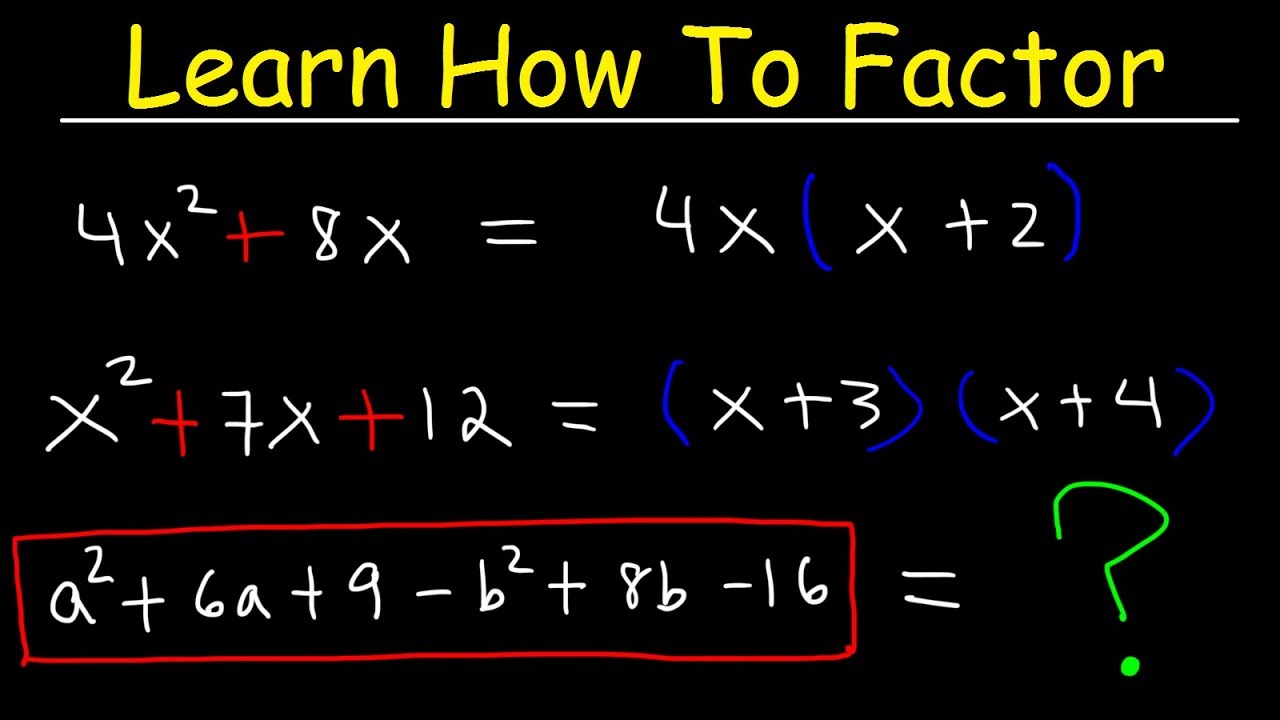
Factoring Trinomials & Polynomials, Basic Introduction - Algebra

Factoring Polynomials - By GCF, AC Method, Grouping, Substitution, Sum & Difference of Cubes

factoring trinomials with "a" greater than 1

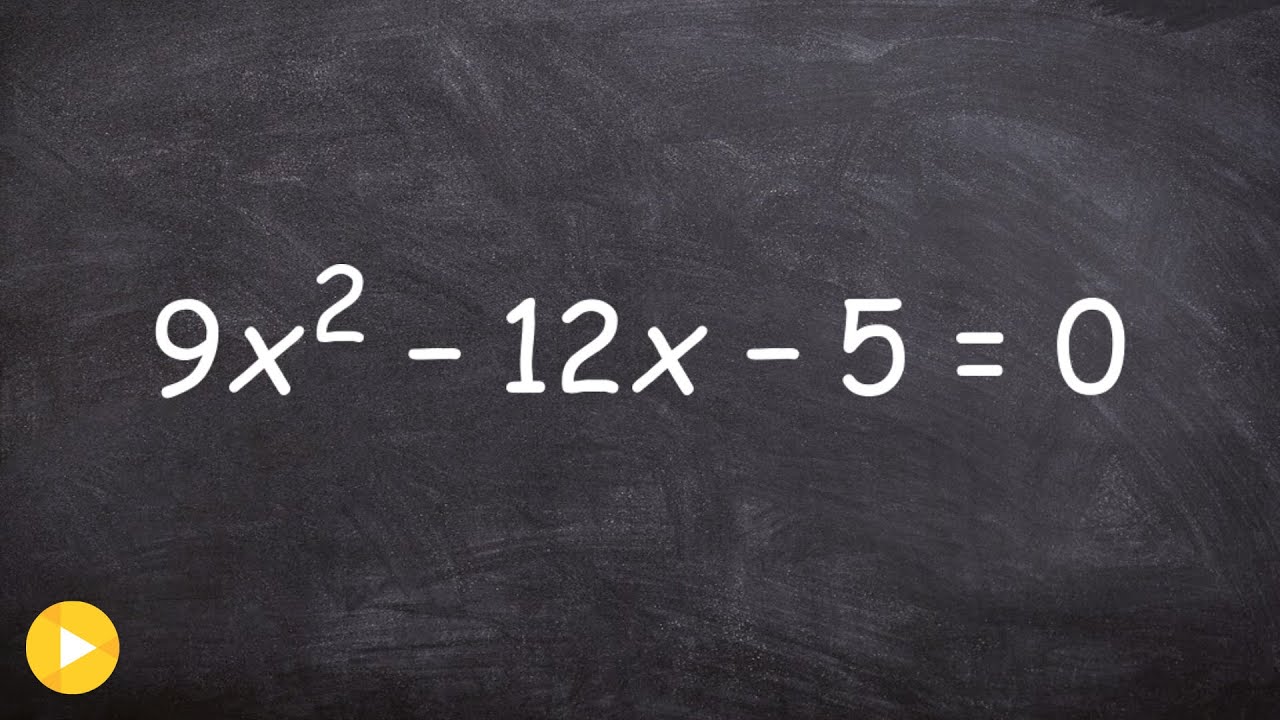
Learn the ac method for factoring and solving a quadratic equation

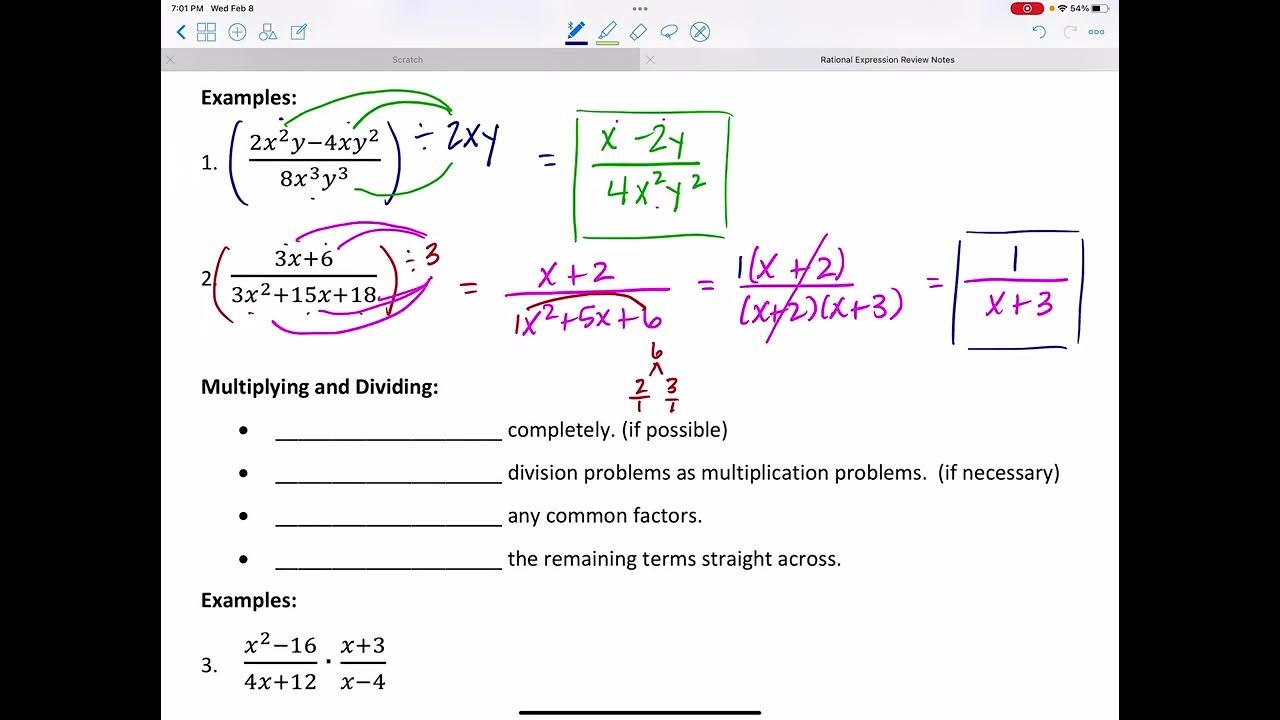
Rational Expressions Review

Related Tags

Factoring Techniques Trigonometric Equations Mathematical Review Polynomial Equations Trig Identities Educational Content Greatest Common Factor Grouping Method Bottoms Up Approach Difference of Squares Prime Polynomials