conical pendulums and banking explained
TLDRThis educational video delves into complex circular motion scenarios, exploring the physics behind a conical pendulum, banking in aircraft, and banked roads for vehicles. It explains how centripetal force, tension, and gravity interact, providing mathematical relationships and formulas to calculate velocity and tension. The video also touches on the effects of friction and how it influences the ideal velocity for circular paths, offering insights into real-world applications of these principles.
Takeaways
- 🔄 Circular motion can be more complex when involving different planes and angles.
- 🎡 The conical pendulum, similar to a merry-go-round, involves an object spinning in a circular path with two forces: tension and weight.
- ✈️ When a plane banks, it turns in a circle at an angle, contributing to centripetal force through lift and weight.
- 🚗 A car on a banked road experiences increased centripetal force, allowing it to travel faster around a bend without sliding.
- 📐 In a conical pendulum, the centripetal force is the sum of tension and weight, resulting in a net force towards the center.
- ⚖️ The formula for the velocity of a conical pendulum is V = sqrt(g * tan(theta)).
- 🧮 For a banking plane, the centripetal force is due to the combination of lift and weight forces.
- 🚦 On a flat road, the centripetal force for a car is provided by the frictional force between the tires and the road.
- 🛤️ In the case of a frictionless banked road, the ideal velocity of a car is V = sqrt(g * R * tan(theta)).
- 🌡️ The mass of the object in circular motion scenarios often cancels out, indicating that the mass is not a factor in determining the velocity.
Q & A
What is the main topic of the video?
-The main topic of the video is circular motion in high school physics, with a focus on more complex examples involving centripetal forces.
What are the three examples discussed in the video?
-The three examples discussed are the conical pendulum, a banking plane, and a road surface banked towards the center of a circle.
What is a conical pendulum?
-A conical pendulum is a simple system where an object is attached to a string and spun around in a circular path, creating a cone shape.
What are the two forces acting on an object in a conical pendulum?
-The two forces acting on an object in a conical pendulum are the tension along the string and the weight of the object acting downwards.
How is the tension in a conical pendulum related to the weight of the object?
-The tension in a conical pendulum is equal to the weight of the object (mg) divided by the cosine of the angle (theta) from the vertical.
What is the formula for the velocity of an object in a conical pendulum?
-The formula for the velocity of an object in a conical pendulum is V = √(g * R * tan(theta)), where g is the acceleration due to gravity, R is the radius of the circular path, and theta is the angle from the vertical.
What does it mean for a road to be banked?
-A road is banked when it is inclined towards the center of the circle, which helps increase the centripetal force and allows a car to travel faster around a bend.
What is the ideal velocity for a car to travel on a banked road without friction?
-The ideal velocity for a car to travel on a banked road without friction is given by V = √(g * R * tan(theta)), where theta is the angle of the banked road.
How does friction affect the motion of a car on a banked road?
-Friction affects the motion of a car on a banked road by providing an additional force that helps maintain the centripetal force needed for circular motion. If friction is absent, the car may slide up or down the banked road.
What is the relationship between the period of a pendulum and the conical pendulum when the angle is small?
-When the angle is small, the period of a conical pendulum is similar to that of a simple pendulum, given by T = 2π * √(L/g), where L is the length of the pendulum and g is the acceleration due to gravity.
How can the formula for the period of a conical pendulum be derived from the given script?
-The formula for the period of a conical pendulum can be derived by equating the velocity formula (V = √(g * R * tan(theta))) with the circumference over time (V = 2πR/T), leading to T = 2π * √(R/g * tan(theta)).
Outlines
🔁 Introduction to Complex Circular Motion
The script begins with an introduction to the topic of circular motion, building upon a previous discussion about uniform circular motion in a horizontal plane. The host outlines three more complex examples of circular motion involving centripetal forces: the conical pendulum, a banking plane, and a banked road surface. These examples are intended to deepen the understanding of concepts such as velocity, centripetal force, and their applications in real-world scenarios. The host also promises to provide timestamps in the description for viewers to navigate through different sections of the video.
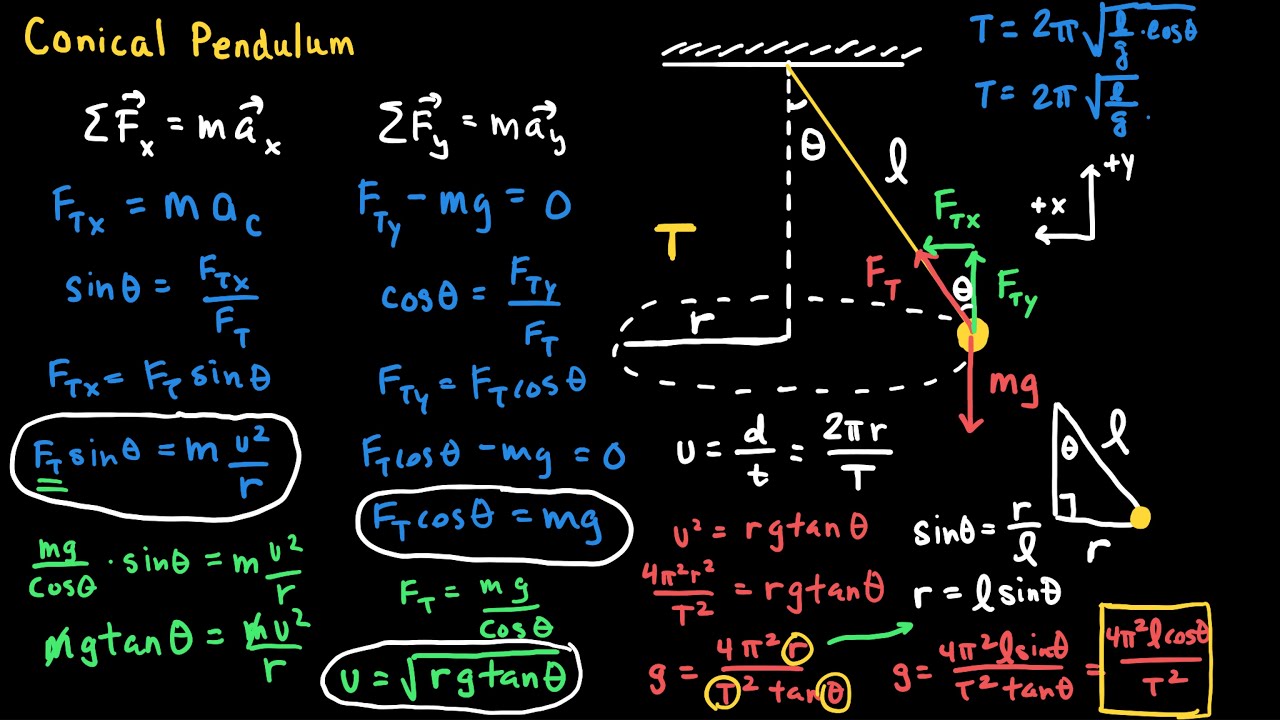
🌗 The Conical Pendulum and Its Dynamics
This paragraph delves into the physics of the conical pendulum, a classic example of circular motion. The object is spinning in a circle with a fixed radius, influenced by two main forces: tension along the string and the weight of the object. The net force creates the centripetal force necessary for circular motion, which is mathematically described as MV^2/R. By analyzing the forces in terms of their components and using trigonometric relationships, the script derives formulas to calculate the tension in the string and the velocity of the pendulum. The host provides an example with specific values to demonstrate how to apply these formulas.
✈️ Banking Planes and Circular Turn Dynamics
The script moves on to discuss the dynamics of a banking plane, which is an aircraft turning in a circle. The forces involved are the weight of the plane and the lift force, which combine to provide the centripetal force for the turn. Although the mathematical treatment is not detailed, it is noted to be similar to that of the conical pendulum. The host emphasizes the importance of understanding the components of force and how they contribute to the circular motion of the plane.
🚗 Banked Roads and Vehicle Stability
This section examines the physics of a car traveling on a banked road, focusing on the forces that allow the car to maintain its circular path. The normal force and the weight of the car combine to produce the centripetal force. The script explores the scenario both with and without friction, highlighting the 'ideal velocity' at which a car can travel without slipping. An example calculation is provided for a car on an icy, banked track, demonstrating how to determine the necessary speed to maintain a stable path.
🔄 Incorporating Friction into Circular Motion
The script concludes with a more complex analysis that includes friction in the circular motion equations. The forces acting on the car are broken down into vertical and horizontal components, with the net horizontal force equating to the centripetal force. The vertical forces must sum to zero since there is no vertical acceleration. The host derives a formula that incorporates friction into the calculation of the car's velocity, showing how the velocity increases with greater road friction. The script acknowledges the complexity of this scenario and encourages viewers to continue learning about the role of frictional forces in circular motion.
⏱ Deriving the Period Formula for Circular Motion
In an additional exploration, the script derives a formula for the period of circular motion, relating it to the velocity, radius, and the angle of inclination. The host shows that, for small angles, the period of a conical pendulum approaches that of a simple pendulum, providing a deeper understanding of the relationship between pendulum motion and circular motion. The script encourages viewers to appreciate the interconnectedness of physical concepts and to explore these connections further.
Mindmap
Keywords
💡Circular Motion
💡Centrifugal Force
💡Centripetal Force
💡Conical Pendulum
💡Tension
💡Banked Road
💡Friction
💡Plane Banking
💡Velocity
💡Trigonometry
💡Mass
💡Acceleration Due to Gravity
Highlights
Introduction to circular motion concepts beyond basic horizontal plane rotation.
Explanation of the conical pendulum and its force dynamics involving tension and weight.
Derivation of the formula for centripetal force in a conical pendulum scenario.
Analysis of the relationship between tension, weight, and centripetal force in circular motion.
Calculation of velocity in a conical pendulum using gravitational force and angle.
Application of the derived formula to calculate the tension and speed in a real-world example.
Discussion on the banking of a plane and its relation to centripetal force during turns.
Introduction to the concept of a banked road and its impact on vehicle speed and stability.
Explanation of the ideal velocity for a car on a banked road without friction.
Calculation of the ideal speed for a car on a banked track with a given radius and angle.
Introduction to the effects of friction on the dynamics of circular motion in vehicles.
Detailed breakdown of forces acting on a car in a frictionless and banked scenario.
Derivation of the formula incorporating friction into the circular motion of a car.
Analysis of the impact of friction on the maximum achievable velocity of a car on a banked road.
Extension of the circular motion discussion to include the period of motion and pendulum formulas.
Derivation of the period formula for a conical pendulum and its relation to a simple pendulum.
Conclusion summarizing the understanding of complex circular motion problems and their practical applications.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: