Dynamics of Uniform Circular Motion
TLDRThis script delves into the dynamics of uniform circular motion, explaining how an object moves at a constant speed along a circular path. It clarifies that while speed remains constant, velocity changes due to direction alteration. The period of rotation, speed calculation, and centripetal acceleration and force are discussed, with examples like a tire balancing machine and a model airplane. The lecture also covers scenarios including a car on a banked curve, a satellite orbiting Earth, and a Space Station creating artificial gravity, illustrating how centripetal force, derived from various sources like tension, gravity, or friction, is pivotal in maintaining circular motion.
Takeaways
- 🔁 Uniform circular motion involves an object moving at a constant speed along a circular path, with a constant speed but changing velocity direction.
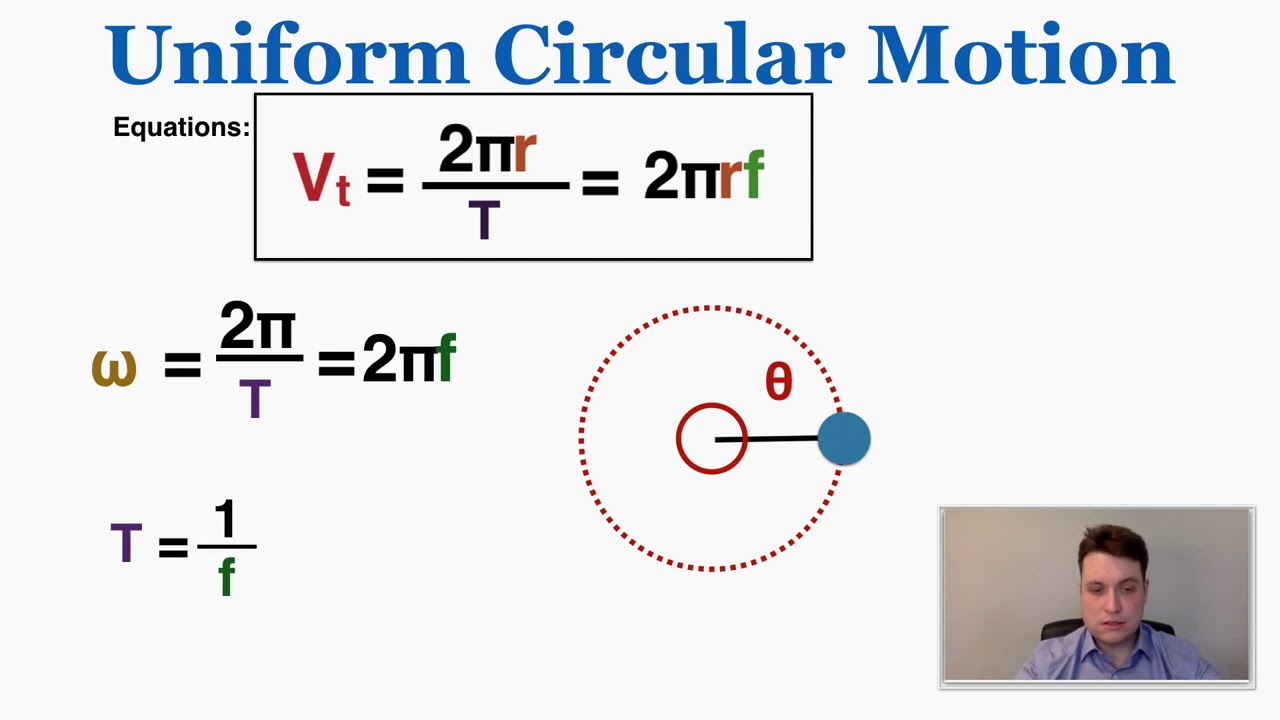
- ⏱ The period of rotation (T) is the time taken for one complete revolution in uniform circular motion, and speed can be calculated using \( v = \frac{2\pi R}{T} \).
- 📐 Centripetal acceleration is always directed towards the center of the circle, and its presence causes the change in velocity direction, not magnitude.
- 🔗 Newton's second law relates the centripetal force to mass, acceleration, and the net force acting on an object undergoing circular motion.
- 🌐 Centripetal force is the net force required to keep an object in circular motion, and it continually changes direction as the object moves.
- 🛫 In the case of a model airplane tied to a string, the centripetal force is provided by the tension in the string.
- 🚀 For a satellite orbiting Earth, the centripetal force is the gravitational force exerted by Earth.
- 🚗 When a car takes a turn, static friction provides the centripetal force necessary for uniform circular motion.
- 🎢 On a banked curve, the centripetal force is provided by the normal force component, which is a function of the road's banking angle and the car's speed.
- 🌌 The speed of a satellite in uniform circular motion around Earth is determined by the gravitational constant, Earth's mass, and the radius of the orbit, independent of the satellite's mass.
- 🛰 The Hubble Space Telescope's speed in orbit can be calculated using the derived formula, demonstrating how satellite speed is related to its orbital radius.
Q & A
What is uniform circular motion?
-Uniform circular motion is the motion of an object traveling at a constant speed on a circular path. The speed (magnitude of velocity) is constant, but the direction of the velocity changes continuously.
What is the period of rotation in uniform circular motion?
-The period of rotation (T) is the time it takes for an object in uniform circular motion to complete one full revolution around its trajectory.
How can you calculate the speed of rotation for an object in uniform circular motion?
-The speed of rotation (V) can be calculated using the formula V = 2πR/T, where R is the radius of the circular path and T is the period of rotation.
What is the relationship between the speed of rotation and the period of rotation in uniform circular motion?
-The speed of rotation is directly proportional to the circumference of the circle (2πR) and inversely proportional to the period of rotation (T). If the period is constant, as in uniform circular motion, then the speed is also constant.
Can you provide an example of calculating the speed of rotation from the script?
-Yes, the script provides an example of a car wheel with a radius of 0.29 meters rotating at 830 revolutions per minute on a tire balancing machine. The speed at the outer edge of the wheel is calculated to be 25 meters per second.
What is centripetal acceleration and why is it important in uniform circular motion?
-Centripetal acceleration is the acceleration that acts towards the center of the circle, causing an object to move in a circular path. It's important because it's responsible for the continuous change in the direction of the velocity, even though the speed remains constant.
What is the direction of centripetal acceleration in uniform circular motion?
-The direction of centripetal acceleration is always towards the center of the circular path.
How is centripetal acceleration related to the speed of an object in uniform circular motion?
-Centripetal acceleration (a_c) is equal to the square of the speed (V) divided by the radius (R) of the circular path, expressed as a_c = V^2/R.
What is the centripetal force and how does it relate to Newton's second law?
-Centripetal force is the net force required to keep an object moving in a circular path. According to Newton's second law, this force is equal to the mass of the object (M) times the centripetal acceleration (a_c), or FC = M * a_c.
Can you give an example of different forces that can act as centripetal force in various scenarios?
-Yes, different forces can act as centripetal force depending on the scenario: tension in a string for a model airplane, gravitational force for a satellite orbiting Earth, and static friction for a car taking a turn.
How does the tension in the guideline of a model airplane relate to its speed?
-The tension in the guideline is directly proportional to the square of the speed of the airplane. If the speed increases, the tension increases, and if the speed decreases, the tension decreases.
What is the significance of the banking of a curve in terms of the centripetal force?
-A banked curve changes the source of the centripetal force from friction to the normal force component from the road surface. The angle of the bank allows the normal force to provide the necessary centripetal force to keep the car from slipping.
Can you explain how the speed of a satellite in orbit is determined?
-The speed of a satellite in orbit is determined by the gravitational force acting between the satellite and Earth. The speed (V) is the square root of the product of the gravitational constant (G), the mass of Earth (Me), and the radius of the orbit (R), expressed as V = √(G * Me / R).
What is the relationship between the period of rotation and the radius of a satellite's orbit?
-The period of rotation (T) of a satellite is related to the radius of its orbit (R) by the formula T = 2π * R^(3/2) / √(G * Me), where G is the gravitational constant and Me is the mass of Earth.
How can the concept of uniform circular motion be applied to create artificial gravity?
-Artificial gravity can be created by rotating a space station about its central axis. The centripetal force experienced by an astronaut must equal their weight on Earth, which can be achieved by setting the appropriate speed of rotation.
What are the four characteristic positions of forces acting on a motorcyclist going around a vertical loop?
-The four positions are: 1) At the bottom, where the centripetal force is the difference between the normal force and the weight of the motorcyclist; 2) At the top, where the centripetal force is the normal force; 3) On the downward slope, where the centripetal force is the sum of the normal force and the weight; and 4) On the upward slope, where the centripetal force is the normal force pointing towards the center of the loop.
Outlines
🔁 Dynamics of Uniform Circular Motion
This paragraph introduces the concept of uniform circular motion, where an object moves at a constant speed along a circular path. The script uses the example of an airplane tied to a string to illustrate that while the speed (magnitude of velocity) remains constant, the direction of velocity changes continuously, necessitating a centripetal force. It explains the period of rotation (T) and how to calculate the speed of rotation using the formula V = 2πR/T, where R is the radius of the circle. An example problem involving a tire balancing machine is presented to demonstrate the calculation of speed for a wheel rotating at a given number of revolutions per minute.
🚀 Centripetal Acceleration and Force in Circular Motion
The paragraph delves into the reasons behind circular motion, highlighting the role of centripetal acceleration, which is always directed towards the center of the circular path. It contrasts this with linear motion, where acceleration increases the speed of an object. The relationship between centripetal acceleration (a_c), speed (V), and radius (R) is established as a_c = V^2/R. The paragraph also connects Newton's second law to centripetal force (F_c), demonstrating that F_c = M*a_c, and further explains that F_c can be expressed as M*V^2/R. Various examples of centripetal forces in different scenarios, such as tension in a string, gravitational force on a satellite, and static friction in a turning car, are provided to illustrate the concept.
📐 Centripetal Force in Uniform Circular Motion: Examples and Calculations
This section uses an example involving a model airplane to calculate the tension in a guideline required to maintain uniform circular motion. It reinforces the concept that the centripetal force is directed towards the center of the circle and is responsible for the change in direction of the velocity, not its magnitude. The calculation shows that an increase in speed would result in a higher tension in the guideline, which could potentially break it. The consequences of such an event are discussed, applying Newton's first law to predict the airplane's trajectory if the guideline were to break.
🛣️ Banked Turns and Centripetal Force in Driving
The paragraph explores the dynamics of a car taking a banked turn, explaining how the centripetal force is provided by the normal force in the absence of friction. It introduces the concept of a banked curve and describes the forces acting on a car, including gravity and the normal force. The relationship between these forces is used to derive an expression for the safe speed to drive on a banked road without slipping. An example using the Daytona International Speedway illustrates how to calculate the speed at which cars can safely navigate banked turns.
🌌 Satellite Orbits and Uniform Circular Motion
This section discusses the uniform circular motion of satellites orbiting Earth, focusing on the gravitational force as the centripetal force that keeps the satellite in orbit. The formula for the speed of a satellite in uniform circular motion is derived, showing that it depends on the radius of the orbit and not on the satellite's mass. The Hubble Space Telescope is used as an example to calculate its orbital speed. Additionally, the period of rotation for a satellite is derived, and the formula is applied to various celestial bodies.
🌐 Artificial Gravity and Vertical Circular Motion
The final paragraph examines the concept of artificial gravity, specifically the speed at which a Space Station must rotate to create a centripetal force equal to an astronaut's weight on Earth. The calculation involves the radius of the Space Station and Earth's gravity, resulting in a specific speed of rotation. The paragraph also touches on the forces experienced by a motorcyclist going around a vertical loop, detailing how the centripetal force changes at different points in the loop.
🏍️ Forces in Vertical Circular Motion: The Motorcycle Loop
This concluding section of the script describes the forces acting on a motorcyclist as they navigate a vertical loop. It outlines the four characteristic positions a motorcyclist encounters while completing the loop, detailing how the centripetal force is determined at each point. The forces involved include the normal force exerted by the loop and the weight of the motorcyclist. The description provides a clear understanding of how the centripetal force varies as the motorcyclist moves through different stages of the vertical circular motion.
Mindmap
Keywords
💡Uniform Circular Motion
💡Velocity
💡Acceleration
💡Centripetal Acceleration
💡Centripetal Force
💡Tire Balancing Machine
💡Newton's Second Law
💡Static Friction
💡Banked Curve
💡Satellite Orbit
💡Artificial Gravity
Highlights
Uniform circular motion involves an object traveling at a constant speed on a circular path.
In uniform circular motion, the speed is constant but the direction of velocity changes due to the circular trajectory.
The period of rotation (T) is the time taken for one full revolution in uniform circular motion.
Speed of rotation can be calculated using the formula: speed = (2π * radius) / period.
An example problem illustrates calculating the speed of a car wheel on a tire balancing machine.
Centripetal acceleration is the acceleration directed towards the center of the circular path.
Centripetal acceleration is perpendicular to the velocity, which is why speed remains constant during circular motion.
The relationship between centripetal acceleration, speed, and radius is given by the formula: centripetal acceleration = (speed^2) / radius.
Newton's second law is applied to find the centripetal force acting on an object in uniform circular motion.
Centripetal force is the net force required to keep an object moving in a circular path and always points towards the center.
Examples of centripetal forces include tension in a string, gravitational force on a satellite, and static friction in a turning car.
An example calculates the tension in a guideline holding a model airplane in uniform circular motion.
If the guideline breaks, the airplane will continue in a straight line due to Newton's first law.
Banked curves allow vehicles to turn without slipping due to the normal force providing the centripetal force.
The formula for calculating the safe speed to drive on a banked curve is derived from the normal force components.
Satellites orbiting Earth maintain a uniform circular motion due to gravitational force acting as the centripetal force.
The speed of a satellite in orbit is independent of its mass and depends solely on the radius of the orbit.
The Hubble Space Telescope's orbiting speed is calculated using the gravitational constant and Earth's mass.
The period of rotation for a satellite can be determined using its speed and the gravitational constant.
Artificial gravity can be created by rotating a space station at a specific speed to counteract an astronaut's weight.
Forces and subjective feelings change as a motorcyclist moves through different positions in a vertical loop.
Transcripts
Browse More Related Video

Uniform Circular Motion Free Body Diagrams
Uniform Circular Motion - IB Physics
Changing Velocity | Forces & Motion | Physics | FuseSchool
Introduction to Centripetal Acceleration - Period, Frequency, & Linear Speed - Physics Problems

Uniform Circular Motion and Centripetal Force

Centripetal force and acceleration intuition | Physics | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: