Circular Motion - Part 5 - Conical Pendulums
TLDRThis video script explores the concept of horizontal circular motion, exemplified by the conical pendulum. It explains the forces at play, including tension and weight, and how they contribute to the centripetal force necessary for circular motion. The script guides viewers through resolving forces vertically and horizontally to solve for tension and linear velocity, using trigonometry and physics principles. It also introduces the double string pendulum, emphasizing its similarity to the single string pendulum in terms of force resolution. The video concludes with a summary of key principles for understanding conical pendulums.
Takeaways
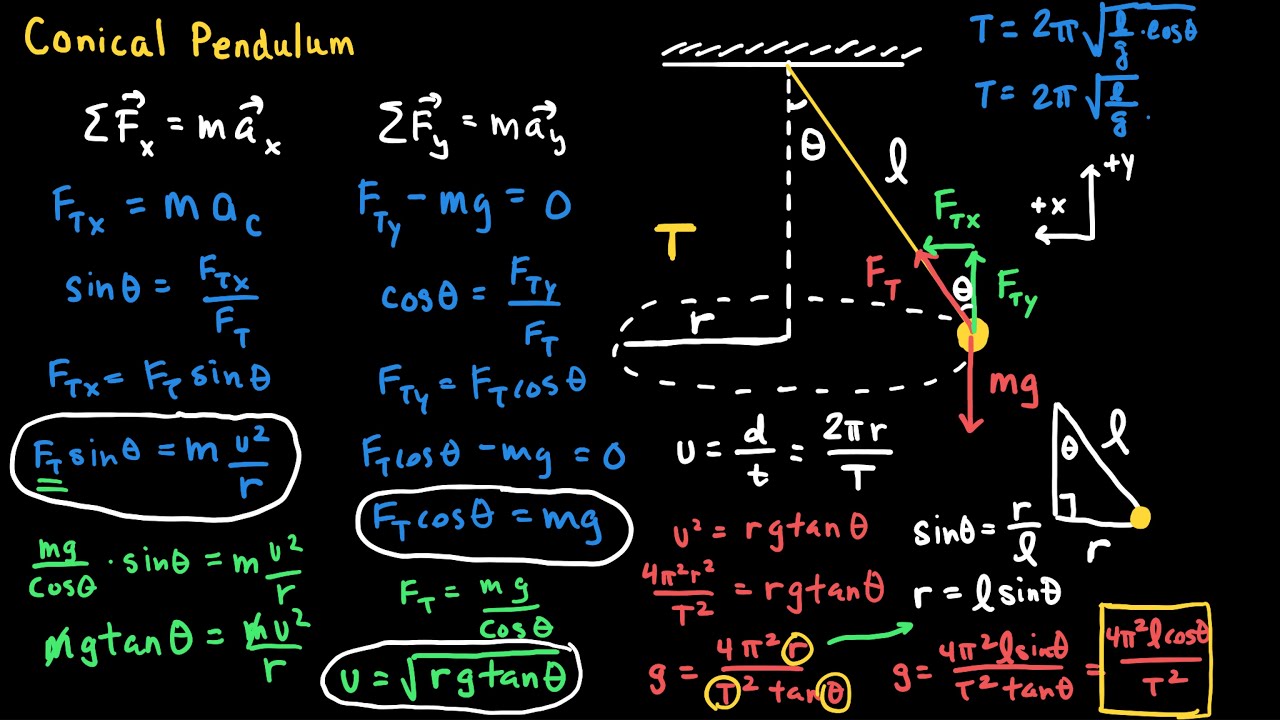
- 📚 The conical pendulum is an example of horizontal circular motion where the resultant force provides the centripetal force.
- 📐 The forces acting on the pendulum are resolved into vertical and horizontal components, with the vertical component being zero due to no vertical displacement.
- 🔍 The vertical component of the tension is equal to the weight of the object, which can be expressed as T sin Theta = mg.
- 📈 The horizontal component of the tension (T cos Theta) is the centripetal force needed to maintain circular motion and is equal to mv^2 / R.
- 🔢 To solve problems involving the conical pendulum, one must understand the relationship between tension, mass, velocity, and radius.
- 🧭 The conical pendulum gets its name from the conical volume of revolution it creates as it moves in a circular path.
- 📉 The vertical resolution of forces is crucial for finding the tension in the pendulum, which is essential for further calculations.
- 📈 The horizontal resolution is key to finding the linear velocity of the pendulum, as the horizontal component of the resultant force is the centripetal force.
- 🔄 The double string pendulum operates on the same principles as the single string, requiring vertical and horizontal force resolution.
- 🔑 Understanding the trigonometric relationships in the pendulum's motion is fundamental to calculating variables such as tension and velocity.
- 🔗 The principles of the conical pendulum can be applied to various circular motion scenarios, emphasizing the importance of resultant force components.
Q & A
What is a conical pendulum?
-A conical pendulum is an example of horizontal circular motion where an object is attached to a string and moves in a circular path, constrained by the resultant force of tension and gravity, creating a cone-shaped volume of revolution.
What are the forces acting on an object in a conical pendulum?
-The forces acting on the object in a conical pendulum are the tension in the string and the weight of the object due to gravity.
Why is it called a conical pendulum?
-It is called a conical pendulum because the path traced by the bob as it swings in a circle forms a cone when viewed in three dimensions.
How do you resolve forces in a conical pendulum setup?
-Forces in a conical pendulum are resolved into vertical and horizontal components. The vertical components must sum to zero since there is no vertical displacement, and the horizontal component of tension provides the centripetal force necessary for circular motion.
What is the relationship between the vertical component of tension and the weight of the object in a conical pendulum?
-The vertical component of tension (T sin Theta) must be equal to the weight of the object (mg), as the net vertical force is zero due to no vertical displacement.
How can you calculate the tension (T) in a conical pendulum given the angle and the weight?
-You can calculate the tension by using the equation T sin Theta = mg, where Theta is the angle between the string and the vertical, m is the mass, and g is the acceleration due to gravity. Solving for T gives T = mg / sin Theta.
What is the horizontal component of the resultant force in a conical pendulum?
-The horizontal component of the resultant force is the horizontal component of the tension (T cos Theta), which provides the centripetal force necessary to keep the object in circular motion.
How can you find the linear velocity (v) of the object in a conical pendulum?
-The linear velocity can be found by using the relationship between the horizontal component of the tension and the centripetal force, T cos Theta = mv^2 / R, where R is the radius of the circular path. Solving for v gives v = sqrt(T cos Theta * R / m).
What is a double string pendulum and how does it differ from a single string pendulum?
-A double string pendulum consists of two pendulums connected end-to-end, and it operates on the same principles as a single string pendulum. The main difference is that you have to resolve forces for both pendulums and account for the interactions between them.
How do you calculate the velocity in a double string pendulum scenario?
-In a double string pendulum, you first calculate the individual tensions and then use the horizontal component of the resultant force (which is the sum of the horizontal components of the tensions) to find the velocity using the equation mv^2 / R = T1 cos Theta1 + T2 cos Theta2.
What are the basic principles to remember when dealing with conical pendulums?
-The basic principles include: 1) The vertical component of the resultant force is zero due to no vertical displacement. 2) The horizontal component of the resultant force is T cos Theta, which provides the centripetal force. 3) For double string pendulums, resolve forces vertically and horizontally for each pendulum and apply the same principles as for single string pendulums.
Outlines
🔍 Understanding the Conical Pendulum
The first paragraph introduces the concept of the conical pendulum, which exemplifies horizontal circular motion. It explains the forces acting on the pendulum's bob, emphasizing the resultant force from tension and weight that provides the centripetal force necessary for circular motion. The paragraph delves into resolving forces both vertically and horizontally, highlighting the significance of the vertical component being zero due to no vertical displacement. It also provides an example calculation to find the tension in the pendulum using trigonometric relationships and the given mass and gravitational acceleration. The paragraph concludes with a discussion on resolving horizontal forces to determine the centripetal force, which is key to solving problems related to the conical pendulum's motion.
📚 Advanced Concepts with Double String Pendulums
The second paragraph extends the discussion to the double string pendulum, which operates on similar principles as the single string conical pendulum. It outlines the process of calculating variables and resolving forces in both vertical and horizontal directions. The paragraph provides a step-by-step calculation to determine the tension in the first string (T1) using the vertical force balance and trigonometric relationships. It then relates this tension to the centripetal force required for circular motion, offering an example calculation to find the linear velocity of the pendulum. The summary concludes with a brief overview of the fundamental principles when dealing with conical pendulums, including the zero vertical resultant force and the relationship between tension, angle, and centripetal force. The paragraph ends with an invitation to subscribe for more content on circular motion.
Mindmap
Keywords
💡Horizontal Circular Motion
💡Conical Pendulum
💡Resultant Force
💡Centripetal Force
💡Tension
💡Weight
💡Trigonometry
💡Vertical Component
💡Horizontal Component
💡Double String Pendulum
💡Velocity
Highlights
A conical pendulum demonstrates horizontal circular motion.
The resultant force in a conical pendulum provides the centripetal force.
Forces in a conical pendulum are resolved both vertically and horizontally.
The vertical component of the resultant force in a conical pendulum is zero due to no vertical displacement.
The vertical component of tension equals the weight in a conical pendulum.
A simple question is presented to calculate tension in a conical pendulum.
The horizontal component of tension provides the centripetal force for circular motion.
An equation relates mass, linear speed, and radius to tension and angle in a conical pendulum.
A method to find the linear velocity in a conical pendulum is explained.
The double string pendulum operates on the same principles as the single string pendulum.
Calculating variables for a double string pendulum involves resolving forces vertically and horizontally.
The resultant force in a double string pendulum is calculated to find tension.
The centripetal force in a double string pendulum is related to the horizontal component of the resultant force.
A method to find the velocity in a double string pendulum is provided.
Basic principles of dealing with conical pendulums are summarized.
The vertical and horizontal components of the resultant force in conical pendulums are equated to mg and mv^2/R, respectively.
The video concludes with an invitation to subscribe for more on circular motion.
Transcripts
Browse More Related Video
The Conical Pendulum

conical pendulums and banking explained

AP Physics Workbook 6.J SMall Angles, Tensions, and Pendulum Period

Centripetal force problem solving | Centripetal force and gravitation | Physics | Khan Academy
Centripetal Acceleration & Force - Circular Motion, Banked Curves, Static Friction, Physics Problems

Uniform Circular Motion Free Body Diagrams
5.0 / 5 (0 votes)
Thanks for rating: