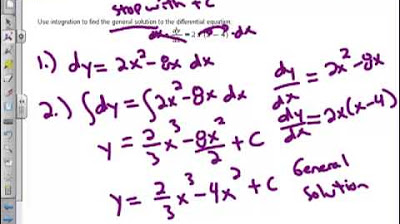Differential Equations: Lecture 1.1-1.2 Definitions and Terminology and Initial Value Problems
TLDRThis lecture delves into the world of differential equations (DEs), starting with the basics and progressing to more complex concepts. The instructor explains what DEs are, their types (ordinary and partial), and the importance of their applications in various scientific fields. The lecture covers how to determine the order of a DE and distinguishes between linear and nonlinear equations, providing examples and exercises to solidify understanding. It also explores different types of solutions, including one-parameter and two-parameter families, particular solutions, and the concept of singular solutions. The session concludes with initial value problems, emphasizing the process of finding particular solutions and the interval of definition, which is crucial for understanding the scope of DE solutions.
Takeaways
- 📚 A differential equation (DE) is fundamentally an equation involving an unknown function and its derivatives.
- 🌌 The solution to a DE can describe phenomena like waves or vibrating springs, which are modeled by functions derived from the DE.
- 🔍 There is a distinction between ordinary differential equations (ODEs), which involve ordinary derivatives, and partial differential equations (PDEs), involving partial derivatives.
- 📈 The order of a DE is determined by the highest derivative present in the equation, which is a key concept for solving DEs.
- 📉 Linear DEs are those where the unknown function and its derivatives appear to the first power with coefficients that are pure functions of the independent variable.
- 🧩 Nonlinear DEs can be more complex, as they do not meet the criteria for linearity, often involving products or powers of the unknown function or its derivatives.
- 🔑 The general solution to a DE includes arbitrary constants (like C in y = c1 cos(x) + c2 sin(x)), representing a family of solutions.
- 🔑🔑 Specific initial conditions can be used to determine the particular solution from the general solution, effectively 'picking' a specific path that satisfies the condition.
- 🔍 The interval of definition refers to the set of values for the independent variable for which the solution is defined and makes sense within the context of the problem.
- 📝 The script also covers the concept of singular solutions, which are solutions to a DE that cannot be obtained by varying the arbitrary constants in the general solution.
Q & A
What is a differential equation?
-A differential equation is an equation that involves an unknown function and one or more of its derivatives. It is used to describe the relationship between a function and its rates of change.
Can you provide an example of a differential equation?
-An example of a differential equation is y double prime (the second derivative of y with respect to x) plus y equals zero: y'' + y = 0.
What is the general solution to the differential equation y'' + y = 0?
-The general solution to the differential equation y'' + y = 0 is y(x) = C1 * cos(x) + C2 * sin(x), where C1 and C2 are arbitrary constants.
How are differential equations used in real-world applications?
-Differential equations are used to model phenomena in various fields such as physics, chemistry, biology, and finance. For example, the Black-Scholes equation is used to model the price of stock options.
What is the difference between an ordinary differential equation (ODE) and a partial differential equation (PDE)?
-An ordinary differential equation (ODE) involves ordinary derivatives, while a partial differential equation (PDE) involves partial derivatives. ODEs deal with functions of a single variable and their derivatives, whereas PDEs deal with functions of multiple variables and their partial derivatives.
What is the order of a differential equation?
-The order of a differential equation is the order of the highest derivative present in the equation. For example, an equation with a second derivative is of the second order.
How is the order of a differential equation determined?
-The order of a differential equation is determined by identifying the highest derivative of the unknown function present in the equation. The highest power of the derivative indicates the order.
What is the difference between a linear and a nonlinear differential equation?
-A linear differential equation can be written in a form where the unknown function and all its derivatives are to the first power and multiplied by functions of the independent variable. A nonlinear differential equation does not satisfy this condition and often involves products or powers of the unknown function or its derivatives.
What is a singular solution in the context of differential equations?
-A singular solution is a solution to a differential equation that cannot be obtained by varying the arbitrary constants in the general solution. It is an exception and does not belong to the family of solutions parameterized by arbitrary constants.
What is an initial value problem in the context of differential equations?
-An initial value problem (IVP) is a differential equation together with an initial condition, which is a specific value of the unknown function at a given point. The initial condition allows for the determination of the particular solution that passes through the specified point.
What is the interval of definition for a solution to a differential equation?
-The interval of definition for a solution to a differential equation is the largest interval over which the solution is defined. It is the set of all points for which the solution exists and is unique.
Outlines
📚 Introduction to Differential Equations
The script begins with an introduction to differential equations (DEs), explaining that they are equations involving an unknown function and its derivatives. The presenter illustrates this with the example of a simple DE, y'' + y = 0, and its solution in the form of a linear combination of cosine and sine functions. The context of DEs in various scientific fields, including physics, chemistry, biology, and finance, is briefly touched upon, with a mention of the famous Black-Scholes equation. The importance of DEs is emphasized, leading into a discussion about the types of DEs: ordinary differential equations (ODEs) and partial differential equations (PDEs), with an example given for each type.
🔍 Exploring the Order of Differential Equations
The second paragraph delves into the concept of the order of a DE, which is defined as the highest derivative present in the equation. The presenter uses several examples to clarify this, such as first-order DEs with first derivatives and higher-order DEs with second or even fourth derivatives. The explanation is accompanied by interactive questioning with the audience, aiming to solidify their understanding of how to identify the order of a DE.
📉 Linear Versus Nonlinear Differential Equations
This section introduces the distinction between linear and nonlinear DEs. The presenter provides a formal definition of a linear DE in the form of an equation where the function y and its derivatives appear to the first power with pure functions of x as coefficients. The explanation is supplemented with examples to demonstrate how to distinguish between linear and nonlinear DEs, emphasizing the complexity and difficulty in solving nonlinear DEs compared to their linear counterparts.
🔑 Types of Solutions to Differential Equations
The script discusses various types of solutions to DEs, such as one-parameter and two-parameter families of solutions, which are sets of solutions containing arbitrary constants resulting from integration. The concept of a particular solution is introduced as a specific member of the family of solutions, determined by specific values of the arbitrary constants. The trivial solution, where y equals zero, is highlighted as a special case. The importance of understanding these different types of solutions is underscored for solving DEs effectively.
📘 Explicit and Implicit Solutions in Differential Equations
The explanation continues with the difference between explicit and implicit solutions. Explicit solutions are those where y is expressed directly in terms of x, while implicit solutions are defined by an equation where y is not isolated. The presenter provides examples to illustrate both types, emphasizing the importance of recognizing whether a solution is explicit or implicit for further analysis and application.
📌 Interval of Definition and Singular Solutions
The concept of the interval of definition is introduced as the largest interval over which a solution to a DE is defined. The presenter demonstrates how to determine this interval using a function and its graph. Additionally, singular solutions are discussed as solutions that cannot be obtained by selecting values for arbitrary constants within a family of solutions. The presenter uses examples to highlight how singular solutions can be overlooked and their importance in solving DEs.
🎯 Initial Value Problems in Differential Equations
This section focuses on initial value problems (IVPs), which are DEs accompanied by a condition that specifies the value of the solution at a particular point. The presenter explains how IVPs are used to select a particular solution from a family of solutions, using the initial condition to determine the value of any arbitrary constants involved. The graphical interpretation of IVPs is also discussed, showing how the condition helps in identifying the specific solution curve that passes through the given point.
📝 Solving Initial Value Problems with Examples
The script provides a step-by-step walkthrough of solving an IVP, including finding the particular solution and determining the interval of definition. The presenter uses a nonlinear DE and an initial condition to demonstrate the process of plugging in values to find the specific solution that fits the condition. The importance of understanding the interval of definition for the initial condition is also emphasized, with examples showing how to choose the correct interval based on the given condition.
🤔 Dealing with No Solution Scenarios in Initial Value Problems
The final paragraph addresses the challenging scenario where an IVP does not yield a solution when the initial condition cannot be satisfied by the given family of solutions. The presenter explains that despite not finding a particular solution that fits the condition, it is still necessary to determine the interval of definition. The concept of singular solutions is revisited, and the presenter demonstrates how to identify and work with such solutions, especially when they are the only solutions that satisfy the DE and the initial condition.
Mindmap
Keywords
💡Differential Equation (DE)
💡Derivative
💡Ordinary Differential Equation (ODE)
💡Partial Differential Equation (PDE)
💡Linear Differential Equation
💡Nonlinear Differential Equation
💡Order of a Differential Equation
💡Solution to a Differential Equation
💡Initial Value Problem (IVP)
💡Interval of Definition
Highlights
A differential equation is an equation involving an unknown function and its derivatives.
Example given: y double prime + y = 0, which is a second-order differential equation.
The solution to the example DE is y = C1*cos(x) + C2*sin(x), representing wave behavior over time.
Differential equations are used to model phenomena in physics, chemistry, biology, and finance, such as the Black-Scholes equation for stock options pricing.
There are two main types of DEs: ordinary differential equations (ODEs) and partial differential equations (PDEs).
An ODE involves ordinary derivatives, while a PDE involves partial derivatives.
The order of a DE is determined by the highest derivative present in the equation.
Linear DEs are contrasted with nonlinear DEs, with linear DEs being more straightforward to solve.
A linear DE can be written with the function and its derivatives to the first power and pure functions of x in front of them.
Nonlinear DEs do not fit the pattern of linear DEs and are generally more complex to solve.
The concept of the general solution of a DE includes all possible solutions obtained by varying the parameters.
A singular solution is a solution that cannot be obtained by varying the parameters of the general solution.
An initial value problem (IVP) is a DE with an additional condition that specifies the value of the solution at a particular point.
The interval of definition is the largest interval over which a solution to a DE is defined.
The process of finding a particular solution from a family of solutions using an initial condition is demonstrated.
The importance of understanding the interval of definition in relation to the initial condition is highlighted.
The concept of a trivial solution, where y = 0 for all x, is introduced as a special case in the context of DEs.
The lecture concludes with a discussion on the challenges and strategies for solving DEs, including recognizing singular solutions and dealing with initial value problems.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: