Differential Equations BC Calculus
TLDRThis video script is an educational lecture on differential equations, focusing on two main questions: whether a derivative can be used to find the original function and how to approximate the original function using its derivative. The instructor outlines steps to solve differential equations, including separating variables, integrating both sides, and adding a constant of integration. Examples of finding both general and particular solutions are provided, explaining the process of handling initial conditions to solve for specific functions. The lecture also touches on verifying solutions to differential equations, preparing students for an upcoming quiz.
Takeaways
- 📚 The chapter focuses on two main topics: finding the original function from a given derivative and using the derivative to approximate the original function around certain areas.
- 🔍 The process involves working with differential equations to either find the general or particular solution based on the derivative provided.
- 📐 The first step in solving differential equations is to separate variables, ensuring that x's and y's are on opposite sides and dx and dy are in the numerator.
- ✅ After separation of variables, the next step is to integrate both sides of the equation, leading to the general solution which includes an added constant 'C'.
- 🔑 The 'C' in the general solution represents an unknown constant that cannot be determined without additional information.
- 🔍 To find a particular solution, one must use an initial condition, which is a known point on the curve, to solve for the constant 'C'.
- 📉 The script provides examples of solving differential equations, including the process of integrating and adding the constant 'C' to the solution.
- 📝 The process of checking work by differentiating the derived general solution to ensure it matches the original differential equation is emphasized.
- 📌 The script mentions an upcoming quiz on differential equations, indicating that the material covered is directly relevant to an assessment due Monday.
- 📚 The examples given in the script illustrate the method of solving for both general and particular solutions to differential equations.
- 📉 The final part of the script involves verifying which of the given functions is a solution to a specific differential equation by substituting and simplifying.
Q & A
What is the main focus of the chapter discussed in the transcript?
-The chapter is focused on two main topics: finding the original function from its derivative and using the derivative to approximate the original function in a certain area.
What is a differential equation in the context of this transcript?
-A differential equation in this context is a mathematical equation that involves a function and its derivative, where the goal is to find the original function from the given derivative.
What is the first step in solving a differential equation as described in the transcript?
-The first step is to separate the variables by cross-multiplying or dividing, ensuring that the x's and y's are on opposite sides and the dx and dy are always in the numerator.
Why is it necessary to add a constant 'C' when integrating both sides of a differential equation?
-The constant 'C' is added because when taking the derivative of a function, the derivative of a constant is zero, and there's no way to know if there was a constant in the original function.
What is the difference between a general solution and a particular solution in the context of differential equations?
-A general solution includes a constant 'C' and represents a family of solutions, whereas a particular solution specifies the exact equation by using an initial condition to solve for 'C'.
How can you find a particular solution to a differential equation?
-To find a particular solution, you use an initial condition, which is a known point on the curve, to solve for the constant 'C' in the general solution.
What is the purpose of checking the work by differentiating the found solution?
-Checking the work by differentiating the solution ensures that the derived function indeed gives the original differential equation, confirming the correctness of the solution.
What does the transcript suggest about the importance of the upcoming quiz on differential equations?
-The transcript suggests that the quiz on differential equations is significant as it mentions that the examples provided are similar to what students will encounter on the quiz due Monday.
How does the transcript explain the process of solving for a particular solution using an initial condition?
-The transcript explains that you substitute the initial condition (known point on the curve) into the general solution to solve for the constant 'C', thus obtaining the particular solution.
What is the significance of the term 'separation of variables' in solving differential equations?
-Separation of variables is a method used in solving differential equations where the variables are manipulated to be on opposite sides of the equation, allowing for integration to find the solution.
Outlines
📚 Introduction to Derivatives and Differential Equations
This paragraph introduces the concept of derivatives and their relationship with original functions. It sets the stage for the exploration of differential equations, emphasizing the two main questions: whether a derivative can be used to find the original function and if it can be used to approximate the original function. The speaker outlines the steps for solving differential equations, which include separating variables, integrating both sides, and adding a constant of integration. The paragraph also mentions an upcoming quiz on differential equations, indicating that the content is relevant to the audience's immediate academic needs.
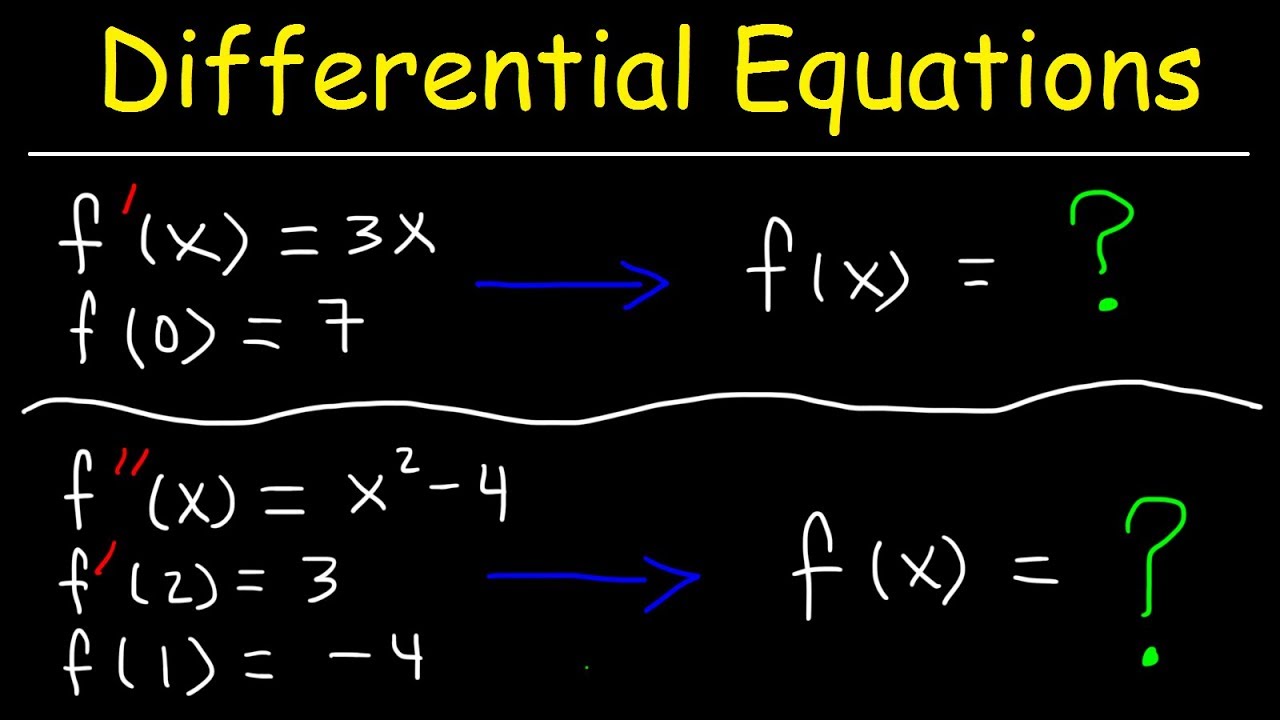
🔍 Finding General and Particular Solutions to Differential Equations
This paragraph delves into the process of finding both general and particular solutions to differential equations. The speaker explains the method of separating variables and integrating to find the general solution, which includes an undetermined constant 'C'. The paragraph then contrasts this with finding a particular solution, which requires knowledge of a specific point on the curve to determine the value of 'C'. Examples are provided to illustrate the steps, including integrating given differential equations and applying initial conditions to solve for 'C'. The speaker also emphasizes the importance of checking work by differentiating the solution to ensure it matches the original equation.
📘 Verifying Solutions to Differential Equations
The final paragraph focuses on the verification of solutions to differential equations. It discusses the process of plugging the derived function back into the original equation to check for correctness. The speaker provides an example where a given function is tested against a differential equation to confirm it is indeed a solution. The paragraph also addresses why certain incorrect options do not satisfy the equation, highlighting the importance of algebraic manipulation and understanding of derivatives in solving and verifying solutions to differential equations.
Mindmap
Keywords
💡Derivative
💡Differential Equation
💡Separation of Variables
💡Integration
💡General Solution
💡Particular Solution
💡Initial Condition
💡Constant of Integration
💡Variable
💡Approximation
Highlights
Introduction to the concept of finding the original function from its derivative.
Exploration of the possibility of using derivatives to approximate the original function.
Explanation of the process for finding the particular or general solution from a differential equation.
The importance of separating variables in differential equations.
Instructions on integrating both sides of a differential equation.
The significance of the constant 'C' in the integration process.
Demonstration of finding the general solution with an example.
Verification of the general solution by differentiating it back.
Introduction to the concept of a particular solution in differential equations.
How to handle initial conditions to find a particular solution.
Example of solving for a particular solution with a given point on the curve.
The process of solving for 'C' in a particular solution.
Cross-multiplying technique in separating variables.
Integration of both sides to solve for 'y' in an equation.
Explanation of why a general solution is called 'general'.
Identification of a correct solution to a given differential equation.
The process of verifying a solution by plugging it into the differential equation.
Preview of a similar question that may appear on an upcoming quiz.
Transcripts
Browse More Related Video

Separation of Variables
Finding Particular Solutions of Differential Equations Given Initial Conditions

Separable differential equations introduction | First order differential equations | Khan Academy

Calculus AB Homework 7.2 Slope Fields

Solving Basic Differential Equations with Integration (Differential Equations 6)
Calculus Chapter 3 Lecture 17 Indefinite Integrals
5.0 / 5 (0 votes)
Thanks for rating: