Business Calculus - Math 1329 - Section 1.5 (Part 1) - Limits and Continuity

Doug Ray

7 Jul 202033:32

EducationalLearning

32 Likes 10 Comments

TLDRThe video script introduces the concept of limits in calculus, which is a fundamental aspect of the subject. It explains the idea of one-sided and two-sided limits, and how they are used to understand the behavior of functions as they approach certain values. The script uses examples to illustrate how to calculate limits algebraically and graphically, emphasizing the importance of evaluating the function at the target point, and if that's not possible due to division by zero, then factoring and canceling can be used. The video clarifies the difference between a limit that does not exist and a function that is undefined, noting that a limit approaches a value or infinity, not that it is undefined. It also touches on the concept of continuity in relation to limits and how they are interconnected in calculus. The script provides a comprehensive foundation for learners to grasp the intricacies of limits, which are essential for further study in calculus.

Takeaways

📚 **Introduction to Calculus**: The video begins with an introduction to limits and continuity, marking the start of the calculus portion of the course.
🔢 **Defining Limits**: Limits are defined, including one-sided and two-sided limits, which are used to understand the behavior of functions as the input approaches a certain value.
🚫 **Undefined Function Values**: The function f(x) is not defined at x = 9 in the given example because it results in a division by zero.
📈 **Approaching Values**: Even when a function is undefined at a point, it's possible to determine the values the function approaches as input gets closer to that point, which is the concept of a limit.
🔍 **Table of Values**: A table of values is used to demonstrate how function values approach a certain number, showing patterns that lead to the understanding of limits.
🔄 **One-Sided Limits**: The left limit and right limit of a function at a certain point are explained, highlighting how they can differ if the function behaves differently on either side of the point.
↔️ **Two-Sided Limits**: A function has a two-sided limit if both the left and right limits exist and are equal, which is a prerequisite for the existence of the limit.
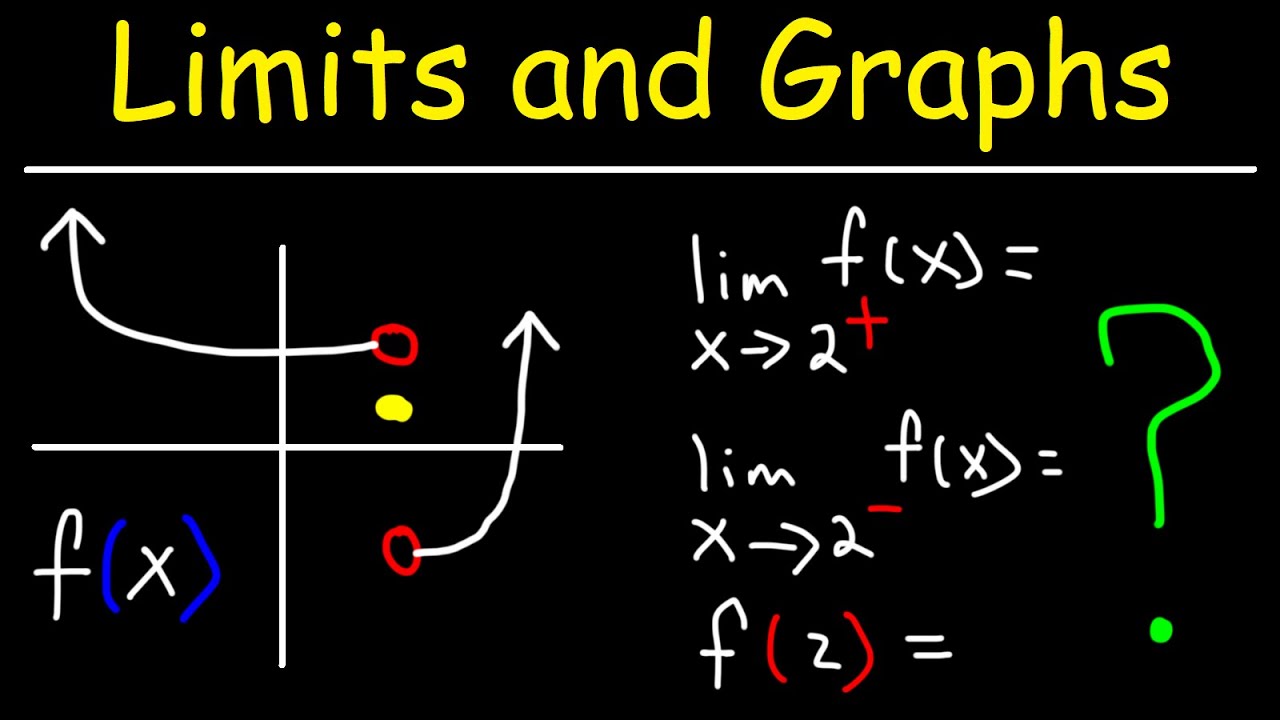
📉 **Graph Analysis**: The video uses graph analysis to compute limits, showing how to find the value approached by the function as x approaches a certain value from the left or right.
🛑 **Non-Existent Limits**: The concept of a limit not existing (denoted as 'dne' for 'does not exist') is introduced when the one-sided limits are not equal.
∞ **Infinity as a Limit**: The behavior of limits approaching infinity is discussed, showing how it is represented on a graph and understood in the context of limits.
📖 **Algebraic Limit Finding**: Techniques for finding limits algebraically are presented, including evaluation, factoring, and canceling out terms, especially when dealing with 'zero over zero' indeterminate forms.

Q & A

What is the main topic of the lesson?
-The main topic of the lesson is limits and continuity in the context of calculus.
What are the different types of limits discussed in the script?
-The script discusses one-sided limits, two-sided limits, and the concept of limits at infinity.
How is the concept of a limit used to evaluate a function at a point where the function is undefined?
-The concept of a limit is used to determine what the function values are approaching as the input values get closer and closer to a certain point, even if the function is undefined at that point.
What is the difference between a limit and a function value?
-A limit considers what the function values are approaching as the input values get closer to certain numbers, while a function value is the actual output of the function for a specific input. The limit can exist even if the function value is undefined.
What is the correct notation for a left limit as X approaches a certain value C?
-The correct notation for a left limit is (lim_{x -> C^-} f(x)), which indicates the limit is taken as X approaches C from the left side.
What is the process of finding limits using algebra?
-The process of finding limits using algebra involves first trying to evaluate the function at the target number. If this is not possible due to division by zero, one can try factoring and canceling before re-evaluating.
What does it mean when a limit results in '0/0'?
-A result of '0/0' indicates an indeterminate form, which means that further algebraic manipulation, such as factoring and canceling, is needed to find the limit.
How is the existence of a two-sided limit determined?
-A two-sided limit exists if and only if both the left and right one-sided limits exist and have the same value.
What does it mean when a limit is said to be 'infinity'?
-When a limit is said to be 'infinity', it means that the function values are increasing without bound as the input values approach a certain point, and the limit does not exist as a finite number.
Why are solid dots used on a graph to denote function values?
-Solid dots are used on a graph to denote points where the function is defined and has a specific value, as opposed to areas where the function is undefined or where limits are being considered.
How does the script demonstrate the concept of continuity in relation to limits?
-The script implies that continuity is related to limits by showing how limits can be used to understand the behavior of a function at points where it may not be defined, thus providing a bridge to the concept of continuity, which is a key topic in calculus.

Outlines

00:00

😀 Introduction to Limits and Continuity

The video begins with an introduction to limits and continuity within the calculus portion of a course. It discusses the need to define limits, including one-sided and two-sided limits, and how to find limit values using tables, functions, algebra, and graphs. The concept of limits is illustrated by examining a function where the denominator becomes zero, which is undefined. The video also explores how to determine what happens to the function's output (Y values) as the input (X values) approaches a certain number, even if the function is not defined at that point.

05:04

🔢 Evaluating Limits with Tables and Values

The script continues with an exploration of how to evaluate limits by using a table of values. It demonstrates how to input different X values into a calculator to find corresponding Y values. The video shows that as X approaches a certain value, the Y values may approach a limit, even if the function is not defined at that X value. The concept of one-sided limits (left and right) is introduced, and the notation for these limits is explained. The video emphasizes that the limit is about the behavior of the function values as they approach a certain point, rather than the value of the function at that point.

10:06

📈 Using Graphs to Understand Limits

The video script explains how to use graphs to compute limits. It describes how to find the Y values approached by the function as X approaches a certain value from the left and right, which are the one-sided limits. The concept of a two-sided limit is introduced, which only exists if both one-sided limits exist and are equal. The video also addresses the difference between function values and limit values, noting that they do not necessarily have to be connected. It concludes with an example of how to determine limits using a graph, including cases where the limit does not exist.

15:06

🤔 Limits at Infinity and Undefined Function Values

The script delves into limits as X approaches infinity or negative infinity, discussing the behavior of function values in these scenarios. It explains that limits can describe the behavior of a function as it approaches a certain value, even if the function is undefined at that point. The video clarifies the difference between saying a function is undefined and saying a limit does not exist. It also provides examples of how to compute limits using graphs, emphasizing the importance of understanding the behavior of the graph, not just the function values at specific points.

20:08

📝 Techniques for Finding Limits Algebraically

The video outlines methods for finding limits algebraically. It suggests first trying to evaluate the function directly at the target number. If this is not possible due to an undefined expression (such as division by zero), the video recommends factoring and canceling before re-evaluating. Common mistakes, such as incorrectly assuming the limit is one or zero in indeterminate forms like 0/0, are highlighted. The process is demonstrated through examples, emphasizing the importance of correctly applying algebraic techniques to find limits.

25:09

🏁 Concluding Remarks on Limit Notation and Factoring

The video concludes with a discussion on when to use and when to drop the limit notation in mathematical expressions. It stresses the importance of maintaining clarity in mathematical communication by keeping equal signs at every step and starting with the original problem before solving. The video also addresses the process of factoring to resolve indeterminate forms like 0/0, showing how to identify and cancel out common factors that lead to such expressions. It concludes with an example that demonstrates the process of finding a limit by factoring and canceling, leading to a final evaluation.

Mindmap

Keywords

💡Limit

A limit in calculus is a value that a function or sequence approaches as the input (or index) approaches some value. In the video, the concept of limits is central to understanding how function values behave as they approach certain points, even if the function is not defined at that point. For example, the function f(x) = (x - 18) / sqrt(x - 3) is not defined at x = 9 because it results in division by zero, but the limit can be determined by looking at the behavior of the function as x approaches 9 from both the left and right sides.

💡Continuity

Continuity in calculus refers to a function being defined and having a derivative at a certain point without any breaks, jumps, or asymptotes. The video discusses how continuity works with limits, emphasizing that for a function to be continuous at a point, the limit as x approaches that point from both sides must exist and be equal to the function's value at that point.

💡One-sided Limits

One-sided limits are limits approached from either the left or the right side of a point where the function may not be defined. The video illustrates this with the function f(x) = (2x - 18) / sqrt(x - 3), showing that as x approaches 9 from the left, the limit is 12, and from the right, the limit is also 12. When both one-sided limits are equal, they define the two-sided limit at that point.

💡Two-sided Limits

A two-sided limit is the value that a function approaches as the input approaches a certain point from both directions (left and right). The video explains that a two-sided limit exists if both one-sided limits exist and are equal. This is demonstrated with the function f(x) = (2x - 18) / sqrt(x - 3), where the left and right limits as x approaches 9 are both 12, thus the two-sided limit exists and is 12.

💡Indeterminate Forms

Indeterminate forms, often resulting in expressions like 0/0 or ∞/∞, occur when direct substitution of a limit results in an undefined expression. The video clarifies that an indeterminate form does not mean the limit does not exist; rather, it indicates that further algebraic manipulation, such as factoring, may be required to find the limit. An example given is the limit as x approaches 7 of (x^2 - 49) / (x - 7), which initially results in 0/0 but simplifies to a determinate limit of 14 after factoring.

💡Factoring and Canceling

Factoring and canceling is a technique used in calculus to simplify expressions, especially when dealing with indeterminate forms. The video demonstrates this method by factoring the numerator and denominator of a limit expression and then canceling out common factors, which allows for the evaluation of the limit. This technique is applied to the limit as x approaches 7 of (x^2 - 49) / (x - 7), simplifying the expression to x + 7 and thus finding the limit to be 14.

💡Undefined Function

A function is said to be undefined at a point where it does not have a value or where it is not valid, such as at points of discontinuity or where the denominator is zero. In the video, the function f(x) = (x - 18) / sqrt(x - 3) is undefined at x = 9 because the denominator becomes zero, which would result in division by zero. The concept is important because it distinguishes between points where the function has a value and those where it does not.

💡Domain of a Function

The domain of a function is the set of all possible input values (x-values) for which the function is defined. The video touches on this concept when discussing why f(9) is undefined for the function f(x) = (x - 18) / sqrt(x - 3), as the domain excludes values that would make the denominator zero. Understanding the domain is crucial for determining where a function's output is valid.

💡Graph of a Function

The graph of a function is a visual representation of the function where the x-values are plotted on the horizontal axis and the corresponding y-values (function outputs) on the vertical axis. The video uses graphs to illustrate the behavior of limits, such as how function values approach a certain point even if the function is not defined at that point. For instance, the graph helps visualize that the limit as x approaches 3 exists but the function value at x = 3 does not necessarily equal the limit.

💡Vertical Asymptote

A vertical asymptote is a vertical line that the graph of a function approaches but never crosses. It occurs where the denominator of a rational function is zero, making the function undefined. The video mentions vertical asymptotes when discussing the limit as x approaches 2 of a function, noting that the function values increase without bound as x approaches 2 from the left, indicating a vertical asymptote at x = 2.

💡Horizontal Asymptote

A horizontal asymptote is a horizontal line that the graph of a function approaches as the input (x-value) increases or decreases without bound. The video discusses this concept when considering the behavior of a function as x approaches positive or negative infinity. For example, it is mentioned that as x approaches negative infinity, the graph of the function levels off at y = 0, indicating a horizontal asymptote at 0.

Highlights

Introduction to limits and continuity in calculus, defining one-sided and two-sided limits.

Demonstration of how to determine if a function is defined at a specific point using an example function f(x) = (x - 18) / sqrt(x - 3).

Explaining that f(9) is undefined due to a zero in the denominator, which is consistent with domain determination.

Investigating the behavior of the function as x approaches 9 by examining a table of values.

Observation that the Y values approach 12 as X approaches 9 from the left, introducing the concept of left limit.

Similarly, the right limit is introduced, showing Y values also approach 12 as X approaches 9 from the right.

The concept of a two-sided limit is introduced, existing only if both one-sided limits are equal.

Graphical representation of limits using a graph to compute the limit as X approaches 3 from the left and right.

Illustration of how function values and limit values can be distinct, emphasizing the difference between limit and function value.

Procedure for finding limits using algebra, including evaluation, factoring, and canceling if direct evaluation is not possible.

Handling of indeterminate forms like 0/0 by factoring and canceling to find a determinate limit.

Emphasis on the importance of using equal signs at every level and starting with the original problem for clear communication.

Example of finding the limit as W approaches -1 using factoring and canceling to solve an indeterminate form.

Explanation of the process of taking a limit as the action of evaluating the function for a given X.

Highlighting the incorrect common mistakes to avoid when dealing with limits, such as assuming a limit of zero or one.

Approach to finding limits when the function is undefined at a point, but the limit exists.

Use of limit notation as part of the problem-solving process, to be dropped once the limit is performed.

Example of a limit that does not exist due to a zero in the denominator with no corresponding zero in the numerator.

Transcripts

Browse More Related Video

1.1 - Numerical and Graphical Limits

BusCalc 02 Limits and Continuity

1.2 - Algebraic Limits and Continuity

Limits via Graphs!

How To Evaluate Limits From a Graph

Math 11 - Sections 1.1-1.2

Related Tags

Calculus Limits Continuity Mathematics Education One-Sided Limits Two-Sided Limits Academic Learning Math Concepts Function Behavior Educational Content