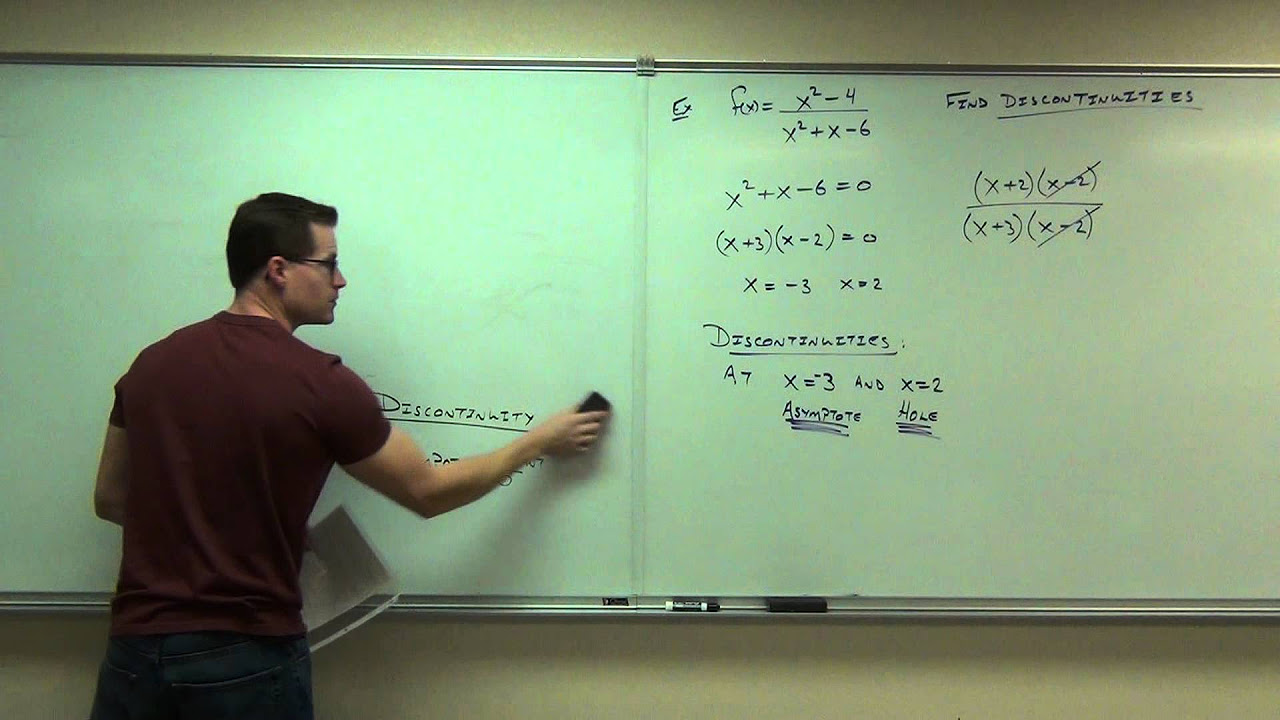1.2 - Algebraic Limits and Continuity
TLDRThe video script delves into the concept of limits in calculus, illustrating how they can be evaluated algebraically, graphically, and numerically. It emphasizes that the limit is the value a function's output approaches as the input (x values) nears a certain point. The script clarifies that for rational functions, if a number is within the domain, the limit as x approaches that number is equivalent to the function's value at that point. However, if the function has discontinuities, such as vertical asymptotes, the direct substitution is not possible, and other techniques must be employed. The transcript also discusses the importance of using multiple strategies to evaluate limits and to verify work. It provides several examples to demonstrate how to find limits for polynomial, rational, and irrational functions, and how to handle cases where direct substitution results in an undefined value due to division by zero. The script concludes with a discussion on continuity, explaining how to determine if a function is continuous at a point or over an interval by ensuring the function is defined, the limit exists, and the function value matches the limit. This comprehensive overview equips viewers with a solid understanding of limits and continuity in calculus.
Takeaways
- 📚 A limit is a mathematical concept that describes the behavior of a function as the input approaches a certain value.
- 📈 Limits can be evaluated algebraically, graphically, or numerically, providing different perspectives and methods to understand the function's behavior.
- 🔢 For rational functions, if a number is within the domain, the limit as x approaches that number can often be found by direct substitution into the function.
- 🚫 If direct substitution results in an undefined expression (like division by zero), the limit may still exist but requires alternative methods such as algebraic manipulation or numerical approaches.
- 🔍 Graphical analysis of limits can provide intuition about the function's behavior and help verify algebraic and numerical findings.
- 📊 Numerical techniques, such as evaluating the function at points increasingly close to the value in question, can approximate the limit.
- 🤔 Discontinuities in a function can occur at points where the function is not defined, or where the left and right limits do not match the function value.
- 🔗 The concept of continuity is closely tied to limits; a function is continuous at a point if it is defined, its limit exists, and the function value equals the limit.
- 🔁 To check continuity at a point, ensure the function is defined, the limit exists, and the function value equals the limit at that point.
- ⛔ Discontinuities can be identified by points where the function is undefined, where the left and right limits do not exist, or do not match the function value.
- 🧩 Polynomial functions are generally continuous over the entire real number line, as they can be drawn without lifting the pen, indicating no holes or breaks in the graph.
Q & A
What are the different ways to evaluate a limit?
-A limit can be evaluated algebraically, graphically, and numerically. Algebraic evaluation involves simplifying the function and substituting the value of x that the limit approaches. Graphical evaluation uses the graph of the function to determine the behavior of the function around the point of interest. Numerical evaluation involves calculating the function's value for points that are very close to the point of interest.
How does the domain of a function affect the evaluation of a limit?
-The domain of a function determines which x-values are valid inputs for the function. If the value x approaches is within the domain of the function, the limit can often be evaluated by direct substitution. However, if the value is not in the domain, such as at a vertical asymptote, direct substitution is not possible, and one must use algebraic manipulation, graphical, or numerical methods to find the limit.
What is a common mistake when substituting a value into a function to evaluate a limit?
-A common mistake is to substitute a value into a function without considering whether that value is within the domain of the function. If the value results in division by zero or an undefined expression, the limit cannot be evaluated by direct substitution, and alternative methods must be used.
What is the significance of a limit approaching a common value from both sides?
-If a limit approaches the same value from both the left and the right sides, this indicates that the function is continuous at that point. If the values from the left and right do not meet at a common value, the limit does not exist at that point, indicating a discontinuity in the function.
What is a rational function and how does it relate to evaluating limits?
-A rational function is a function that can be expressed as the ratio of two polynomials. When evaluating limits of rational functions, one must consider the domain of the function, as the function may be undefined at certain points (like at vertical asymptotes). Rational functions can often be simplified algebraically before evaluating limits, which can make the process easier.
How can you verify a limit that you found algebraically?
-A limit found algebraically can be verified using numerical techniques or by graphing the function. Numerical techniques involve calculating the function's value at points very close to the point of interest. Graphing the function allows you to visualize the behavior of the function around the point and confirm if the limit makes sense based on the graph.
What is the relationship between the existence of a limit and the continuity of a function?
-The existence of a limit is a necessary condition for the continuity of a function at a point. If a limit exists as x approaches a certain value, and the function value at that point matches the limit, the function is continuous at that point. If the limit does not exist or the function value differs from the limit, the function is discontinuous at that point.
What are the conditions for a function to be considered continuous at a point?
-A function is considered continuous at a point if three conditions are met: 1) The function value at that point exists, 2) The limit as x approaches that point exists, and 3) The function value and the limit are equal.
How does a piecewise function affect the continuity of a function?
-A piecewise function can affect continuity at the points where the rules of the function change. Even if each individual rule represents a continuous function over its domain, the point of transition between the rules can introduce a discontinuity if the function values or limits on either side of the transition point do not match.
What is a vertical asymptote and how does it affect the limit of a function?
-A vertical asymptote is a vertical line on the graph of a function where the function is undefined (often due to division by zero in a rational function). At a vertical asymptote, the function approaches infinity or negative infinity from both sides, so the limit at the point of the asymptote does not exist.
Why is it important to have multiple strategies for evaluating limits?
-Having multiple strategies for evaluating limits is important because different functions and limit scenarios may require different approaches. Algebraic evaluation is often the quickest but not always possible. Numerical and graphical evaluations serve as backups and can also be used to check the work done through algebraic methods, ensuring the accuracy of the results.
Outlines
😀 Understanding Limits Algebraically
The video begins by discussing the concept of limits in calculus. It explains how limits can be evaluated algebraically, graphically, and numerically. The importance of the behavior of x and y values as x approaches a certain number is emphasized. The video also covers how to find limits for rational functions when the function is defined at a given number, and the case when the function has discontinuities or holes in the graph is discussed. It concludes with the idea that while algebraic evaluation of limits can save time, it's essential to have multiple strategies for finding limits, including numerical and graphical methods.
📚 Evaluating Limits of Polynomial Functions
The second paragraph focuses on evaluating the limit of a polynomial function as x approaches 2. It demonstrates the process of substituting the value of x into the function and simplifying to find the limit. The video also shows how to verify this limit using a numerical technique by observing the behavior of the function's values as x gets closer to 2 from both sides. The concept of left-hand and right-hand limits is introduced, and it's shown that for the limit to exist, both must approach the same value.
🔍 Examining Limits of Irrational Functions
This part of the video explores the limit of an irrational function as x approaches 0. It discusses the potential for limits to exist even for irrational functions, provided the value is within the domain of the function. The video shows how to approximate the square root of 2 and verify the limit numerically by observing the function's behavior around x equals 0. The importance of checking the function's behavior from both sides of the point of interest is highlighted.
🚫 Dealing with Undefined Limits in Rational Functions
The video addresses the challenge of evaluating limits for rational functions when the denominator becomes zero, which leads to an undefined expression. It uses the example of a rational function and shows that plugging in the value of x that causes division by zero does not yield a valid limit. Instead, the video suggests using graphical or numerical methods to find the limit. The concept of a hole in the graph, representing an undefined function value, is introduced.
🔢 Numerical Verification of Limits
The paragraph discusses the numerical verification of limits by changing table settings on a graphing calculator to observe the behavior of function values as x approaches a certain value. It demonstrates how to input specific x values to see if the function values approach a consistent limit from both sides. The video shows that even when an algebraic approach fails, numerical techniques can confirm the behavior of the function near points of interest.
🔁 Factoring to Simplify Limits Calculation
The video explains how factoring can help simplify the process of finding limits, especially when direct substitution results in an undefined expression. It walks through the factorization of a quadratic trinomial in both the numerator and the denominator, showing how a common factor can be canceled out. This simplification can sometimes allow for an algebraic evaluation of the limit where it was previously not possible.
📉 Discontinuities and Their Algebraic, Graphical, and Numerical Analysis
This section delves into the concept of discontinuities in functions. It explains how to identify points of discontinuity by evaluating the function and its limit at specific points. The importance of function values and limits matching at a point for the function to be continuous is emphasized. The video also discusses how to handle situations where the function's expression becomes undefined or where the limit does not exist due to the function's behavior from different sides of the point in question.
🔍 Investigating Continuity Over Intervals
The final paragraph discusses the concept of continuity over intervals. It explains that a function is continuous over an interval if it is continuous at every point within that interval. The video provides a method to determine continuity by checking if the function is defined, if the limit exists, and if the function value matches the limit at every point in the interval. It concludes by encouraging the use of algebraic, numerical, and graphical techniques to evaluate limits and check for continuity.
Mindmap
Keywords
💡Limit
💡Continuity
💡Domain
💡Asymptote
💡Polynomial Function
💡Rational Function
💡Direct Substitution
💡Factoring
💡Discontinuity
💡Graphical and Numerical Techniques
💡Piecewise Function
Highlights
The video discusses methods to evaluate limits algebraically, graphically, and numerically.
A limit is defined as the behavior of y-values as x approaches a certain number.
For rational functions, if a number is in the domain, the limit as x approaches that number is the function value at that number.
Discontinuities in functions, such as vertical asymptotes or holes, prevent direct evaluation of limits.
Algebraic manipulation can sometimes resolve issues with evaluating limits at points of discontinuity.
Graphical and numerical techniques are alternative methods to find limits when algebraic approaches are not feasible.
The video provides a detailed example of evaluating the limit of a polynomial function as x approaches 2.
Verification of limit results using numerical techniques and graphing is important to ensure accuracy.
The concept of left-hand and right-hand limits is introduced to verify the behavior from both sides of a discontinuity.
An irrational function example demonstrates that limits can sometimes be evaluated directly even for non-rational functions.
The video explains that continuity can be assessed by evaluating function values and limits at specific points.
A function is considered continuous at a point if the function value exists, the limit exists, and both are equal.
Discontinuities can occur due to holes in the graph, jumps between function rules, or undefined function values.
The video uses piecewise functions to illustrate different types of discontinuities and how to analyze them.
A function is continuous over an interval if it is continuous at every point within that interval.
The importance of using multiple strategies (algebraic, numerical, and graphical) to evaluate limits and assess continuity is emphasized.
The video concludes with a comprehensive example that ties together the concepts of limits, continuity, and discontinuity.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: