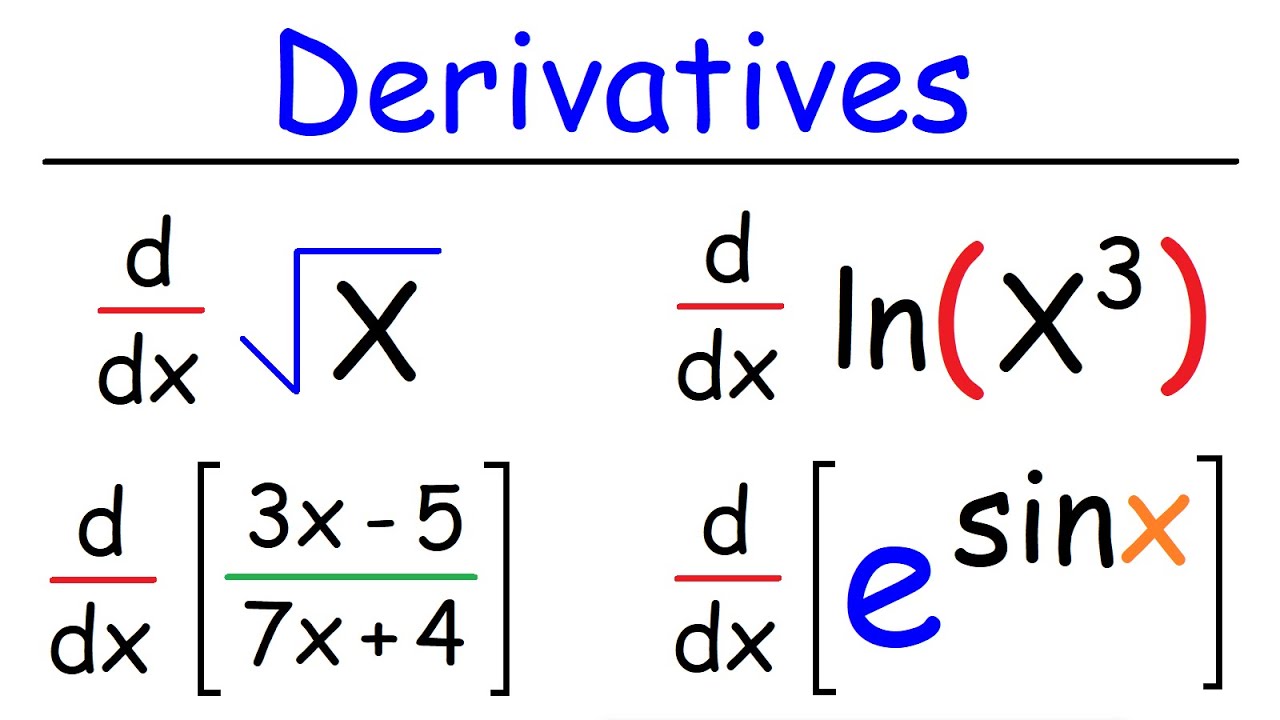Basic Differentiation Rules For Derivatives
TLDRThis video script offers a comprehensive guide to understanding derivatives, covering basic differentiation rules such as the power rule for functions like x^n, and extending to more complex topics like the derivatives of polynomial, radical, rational, and trigonometric functions. It also explains the derivatives of exponential and logarithmic functions with base e, and introduces the product rule for multiplying functions. The script is a valuable resource for anyone seeking to strengthen their calculus skills.
Takeaways
- 📚 The power rule states that the derivative of x raised to the n is n times x to the power of n-1.
- 🔢 For constants, the derivative is always zero, moving the constant to the front of the derivative of the variable.
- 🌟 The derivative of x to the first power is 1, as anything raised to the zero power is 1.
- 📈 The derivative of a polynomial function is found by differentiating each term separately.
- 🤔 For radical functions, rewrite the expression to expose the exponent and then apply the power rule.
- 🏠 The derivative of the square root of x is 1/(2*sqrt(x)), which simplifies to 1/(2*x^(1/2)) or 1/(2*x).
- 📊 Trigonometric functions follow specific derivative patterns: sin'(x) = cos(x), cos'(x) = -sin(x), tan'(x) = sec^2(x), etc.
- 📝 The derivative of exponential functions with base e is found using the formula: d/dx[e^u] = e^u * u', where u is a function of x.
- 🌲 The natural logarithm function's derivative is given by: d/dx[ln(u)] = u'/u, where u is a function of x.
- 🔧 The product rule is used for functions that are the product of two other functions, expressed as (uv)' = u'v + uv'.
- 🚀 For more advanced topics like the quotient rule and chain rule, additional resources are available through linked videos.
Q & A
What is the power rule for differentiation and how is it applied?
-The power rule states that the derivative of x raised to the power of n is n times x to the power of n minus one. It is applied by taking the exponent n, multiplying it by the base x, and then decreasing the exponent by one.
How do you find the derivative of x squared?
-The derivative of x squared (n=2) is found by applying the power rule: 2 times x to the power of (2-1), which simplifies to 2x.
What is the derivative of x cubed and x raised to the fourth power?
-The derivative of x cubed (n=3) is 3 times x squared, and the derivative of x raised to the fourth power (n=4) is 4 times x cubed.
What is the derivative of a constant and why?
-The derivative of any constant is zero because a constant does not change; its rate of change with respect to the variable is zero.
How do you differentiate a polynomial function?
-To differentiate a polynomial function, you apply the power rule to each term separately and then combine the results. For example, the derivative of 4x^5 + 7x^3 - 9x + 5 involves finding the derivative of each term and then adding them together.
What is the process for finding the derivative of a radical function?
-To find the derivative of a radical function, you first rewrite the function with the radical in the form of a power (e.g., square root as x^(1/2)). Then, you apply the power rule with the new exponent, adjusting for negative exponents by flipping the fraction and changing the radical back.
What are the derivatives of the basic trigonometric functions?
-The derivative of sine x is cosine x, the derivative of cosine x is negative sine x, the derivative of tangent x is secant squared, and the derivatives of cotangent, secant, and cosecant x follow a pattern where they are negative and involve the related trigonometric function.
How do you find the derivative of a function involving e to the power of a function?
-The derivative of e to the power of a function u(x) is e to the power of u times the derivative of u, which is u prime. For example, the derivative of e^(x^2) is e^(x^2) times the derivative of x^2, which is 2x.
What is the formula for the derivative of the natural logarithm of a function?
-The derivative of the natural logarithm of a function u(x) is u prime divided by u. For example, the derivative of ln(x^2 + 5) is (2x) / (x^2 + 5).
What is the product rule for differentiation and when is it used?
-The product rule states that the derivative of a product of two functions, u and v, is the derivative of u times v plus u times the derivative of v. It is used when differentiating a function that is the product of two other functions.
Can you provide an example of using the product rule to differentiate a function?
-An example of using the product rule is differentiating x^2 times sine x. The derivative is 2x times sine x plus x^2 times cosine x.
Where can one find more resources on differentiation topics such as logarithmic functions, exponential functions, and implicit differentiation?
-Additional resources on differentiation topics can be found in the description section below the video, where links to more video resources are provided for further study.
Outlines
📚 Introduction to Derivatives and Basic Differentiation Rules
This paragraph introduces the concept of derivatives and explains the fundamental differentiation rules required for calculating them. The power rule is introduced and demonstrated with examples, showing how to find the derivative of functions like x squared, x cubed, and x raised to the fourth power. It also explains how to handle constants and variables in derivatives, emphasizing that the derivative of any constant is zero. The paragraph sets the foundation for understanding how to use the power rule to find the derivative of a function.
🌟 Derivatives of Radical and Rational Functions
This section delves into finding the derivatives of radical and rational functions. It starts by explaining how to differentiate the square root of x using the power rule and introduces the concept of negative exponents and their handling. The paragraph then moves on to cover the derivatives of higher root functions, like the seventh root of x to the fourth power. It also explains the process of differentiating rational functions, such as the derivative of one divided by x raised to the fifth power. The key takeaway is learning how to apply the power rule to various types of functions and understanding the transformations necessary for dealing with radicals and rational expressions.
📈 Trigonometric and Exponential Functions Derivatives
This part of the script focuses on the derivatives of trigonometric and exponential functions. It provides the derivatives of basic trigonometric functions like sine, cosine, tangent, cotangent, and secant, highlighting the patterns and relationships between them. The script also explains the derivative formula for exponential functions with base e, using it to find the derivatives of various exponential expressions. The main points include understanding the relationship between trigonometric functions and their derivatives, as well as the constant nature of the base e in exponential functions and how it affects differentiation.
🧮 Derivatives of Logarithmic Functions and the Product Rule
This paragraph covers the derivatives of natural logarithmic functions and introduces the product rule for differentiation. The derivative of ln x is derived and explained, with an example of differentiating a function that includes a natural log. The product rule is then defined and demonstrated through examples, showing how to differentiate the product of two functions. The summary emphasizes the importance of understanding the relationship between the product rule and the derivatives of multiplications of functions, as well as the specific rules for differentiating logarithmic functions.
🔗 Additional Resources and Conclusion
In the final paragraph, the speaker directs the audience to additional resources for learning more about derivatives, including links to other videos covering related topics like the quotient rule and chain rule. The speaker also encourages viewers to subscribe to the channel for more content. The main takeaway is that the video serves as an introduction and there are further resources available for those interested in deepening their understanding of derivatives and related mathematical concepts.
Mindmap
Keywords
💡Derivative
💡Power Rule
💡Polynomial Function
💡Trigonometric Functions
💡Exponential Functions
💡Logarithmic Functions
💡Product Rule
💡Chain Rule
💡Quotient Rule
💡Constant
💡Negative Exponent
Highlights
The video discusses the concept of derivatives and how to find them using basic differentiation rules.
The power rule for differentiation is introduced, which states that the derivative of x raised to the power of n is n times x to the power of n-1.
The derivative of x squared is calculated as 2x, using the power rule with n equal to 2.
The derivative of x cubed and x raised to the fourth power are calculated using the power rule, resulting in 3x squared and 4x cubed respectively.
The derivative of x is found to be 1, since it is x raised to the first power with n equal to 1.
A constant multiplied by a variable in a term does not change the derivative of the variable; the constant is factored out.
The derivative of any constant is zero, which can be remembered by writing the constant as a term with x to the zero power.
Derivatives of polynomial functions are calculated by differentiating each term separately, as demonstrated with the function 4x to the fifth plus 7x cubed minus 9x plus 5.
The derivative of the square root of x, or x to the power of 1/2, is found to be 1/(2x) by rewriting the function and applying the power rule.
Derivatives of radical functions, such as the seventh root of x to the fourth power, are calculated by adjusting the exponent and changing the sign for negative exponents.
The derivative of a rational function, such as the derivative of 1 divided by x raised to the fifth power, is found by inverting the exponent and adjusting for the negative sign.
Trigonometric function derivatives are discussed, with the derivative of sine x being cosine x, and the derivative of cosine x being negative sine x.
Derivatives of functions involving the base e, such as e to the x, are found by multiplying the function by its derivative, which is 1 for e to the x.
The natural log function's derivative is found by dividing the derivative of the function inside the log by the function itself.
The product rule for differentiation is introduced for functions that are the product of two other functions, with an example given for x squared times sine x.
The video provides additional resources for learning more about derivatives, including links to other videos on exponential functions, logarithmic functions, implicit differentiation, and more.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: