Derivatives for Beginners - Basic Introduction
TLDRThis comprehensive video tutorial delves into the intricacies of finding derivatives, covering a variety of mathematical rules and scenarios. It begins with foundational concepts such as the derivative of constants and the power rule, then progresses to more complex topics like differentiating polynomials, rational functions, and radical functions. The video also explores the derivatives of trigonometric functions, exponential functions, and logarithmic functions, including both natural and regular logs. It introduces the product, quotient, and chain rules, as well as implicit differentiation and related rates. The tutorial is designed to equip viewers with the necessary tools to tackle a wide range of calculus problems, providing clear examples and explanations to aid understanding.
Takeaways
- 📌 The derivative of any constant is always zero, e.g., the derivative of 7, -4, π, and π^e is zero.
- 📈 The power rule states that the derivative of x raised to the nth power is n times x to the (n-1)th power, e.g., d/dx(x^n) = n * x^(n-1).
- 🔢 For a polynomial function, the derivative is found by applying the power rule to each term and summing the results, e.g., d/dx(4x^3 + 7x^2 - 9x + 5) = 12x^2 + 14x - 9.
- 📂 The constant multiple rule is used when a constant is multiplied by a function; the derivative is the constant times the derivative of the function, e.g., d/dx(6x^8) = 48x^7.
- 🔄 The derivative of a rational function is found by applying the power rule after rewriting the expression, e.g., d/dx(1/x^2) = -2x^(-3).
- 🌐 The derivative of a radical function involves rewriting the function in exponential form and then applying the power rule, e.g., d/dx(√x) = 1/(2√x).
- 📊 Trigonometric functions have specific derivative formulas, e.g., d/dx(sin(x)) = cos(x), d/dx(cos(x)) = -sin(x), and so on.
- 🌀 The chain rule is used for composite functions, where the derivative of the outer function times the derivative of the inner function gives the overall derivative, e.g., d/dx(f(g(x))) = f'(g(x)) * g'(x).
- 📝 Implicit differentiation involves differentiating both sides of an equation with respect to x to solve for dy/dx, e.g., if x^3 + 4xy + y^2 = 9, then dy/dx = -3x^2 + 4y / (4x + 2y).
- 📚 Logarithmic differentiation is performed by setting y equal to the function, taking the natural log of both sides, and then differentiating with respect to x, e.g., the derivative of x^x is x^x * (1 + ln(x)).
- 🔧 The product and quotient rules are used when differentiating functions that are products or quotients of other functions, e.g., d/dx(u*v) = u'*v + u*v' and d/dx(u/v) = (v*u' - u*v') / v^2.
Q & A
What is the derivative of a constant?
-The derivative of any constant is always zero. For example, the derivative of 7, -4, π, and π^e are all zero since there is no variable involved.
What is the power rule for derivatives?
-The power rule states that if you have a variable raised to a constant, such as x^n, the derivative is n times x raised to the power n minus one (n * x^(n-1)).
How do you find the derivative of a polynomial function?
-To find the derivative of a polynomial function, such as f(x) = 4x^3 + 7x^2 - 9x + 5, you take the derivative of each term separately and then combine them. For example, the derivative of 4x^3 is 12x^2, of 7x^2 is 14x, of -9x is -9, and the derivative of a constant is 0.
What is the derivative of a rational function like 1/x^2?
-To find the derivative of a rational function like 1/x^2, you first rewrite the expression to have the variable in the numerator with a positive exponent, then apply the power rule. The derivative of 1/x^2 is -2x^(-3) or -2/x^3 after simplifying.
How do you differentiate a radical function?
-To differentiate a radical function, such as the square root of x, you rewrite the expression as an exponent (x^(1/2)) and then apply the power rule. The derivative of the square root of x is (1/2)x^(-1/2) or 1/(2*sqrt(x)) after simplifying.
What is the derivative of the natural log function ln(u)?
-The derivative of the natural log function ln(u), where u is a function of x, is (1/u) * u' (u prime). For ln(x), the derivative is 1/x since u is x and its derivative is 1.
What is the product rule for derivatives?
-The product rule states that the derivative of a product of two functions (u * v) is u' * v + u * v'. You differentiate each function separately and then combine the results following this rule.
How do you use the quotient rule to find derivatives?
-The quotient rule for derivatives states that the derivative of a quotient (u/v) is (v * u' - u * v') / v^2. You differentiate both the numerator (u) and the denominator (v) and then apply the formula.
What is the chain rule for derivatives?
-The chain rule is used for composite functions, like f(g(x)). The derivative is the derivative of the outer function (f') times the derivative of the inner function (g'(x)), keeping the inner function (g(x)) unchanged.
How do you perform implicit differentiation?
-Implicit differentiation involves differentiating both sides of an equation with respect to x to find dy/dx. You treat y as a function of x and include dy/dx when differentiating terms involving y with respect to x.
What is the derivative of a variable raised to another variable, like x^x?
-To find the derivative of a variable raised to another variable, such as x^x, you use logarithmic differentiation. Set y = x^x, take the natural log of both sides, differentiate both sides with respect to x, and then solve for dy/dx, which results in y * (1 + ln(x)) where y is back-substituted with x^x.
Outlines
📚 Introduction to Derivatives
This paragraph introduces the concept of derivatives, focusing on the derivative of constants and the power rule. It explains that the derivative of any constant is zero and demonstrates the power rule through examples, showing how to find the derivative of a variable raised to a constant power.
📈 Derivatives of Polynomial and Rational Functions
The paragraph delves into finding the derivatives of polynomial and rational functions. It covers the derivative of linear terms, the power rule for higher powers, and the constant multiple rule. The explanation includes examples of deriving polynomial functions and rational functions, illustrating the process of differentiating expressions like 1/x squared and 1/x cubed.
🌟 Derivatives of Radical Functions
This section discusses the process of finding the derivatives of radical functions. It explains how to differentiate expressions involving square roots and higher order roots by rewriting them as exponents and applying the power rule. The paragraph provides examples of deriving the cube root and the fifth power of x, demonstrating the steps involved in simplifying the expressions.
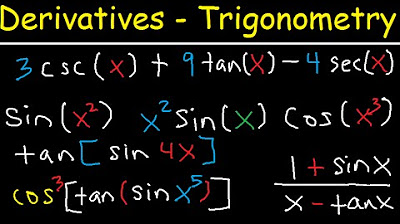
📊 Derivatives of Trigonometric Functions
The paragraph covers the derivatives of trigonometric functions, outlining the rules for differentiating sine, cosine, tangent, cotangent, secant, and cosecant. It emphasizes the patterns and relationships between the derivatives of these functions, providing examples to illustrate the application of these rules in practice.
🔍 Derivatives of Logarithmic Functions
This section explains the derivatives of natural logarithm functions and logarithmic functions with different bases. It details the process of differentiating ln u and log base a of u, with examples that show how to find the derivative of natural logs of x raised to various powers and the natural log of tangent x.
🚀 Derivatives of Exponential Functions
The paragraph focuses on the derivatives of exponential functions, explaining the rule for differentiating a constant raised to a variable. It provides examples of deriving e to the power of x, e to the power of 2x, and other exponential expressions, highlighting the importance of the base e in calculus and its special properties.
🔧 Product and Quotient Rules
This section introduces the product and quotient rules for differentiation. It explains how to differentiate a product of two functions and a quotient of two functions, providing examples and demonstrating the application of these rules in practice. The paragraph also touches on the concept of factoring out common terms for simplification.
🔄 Chain Rule and Implicit Differentiation
The paragraph discusses the chain rule for dealing with composite functions and the process of implicit differentiation. It provides examples of using the chain rule to differentiate functions like sine of x to the fifth power and tangent of sine x cubed. The section also covers implicit differentiation, showing how to find the derivative of y with respect to x in an equation involving x and y.
🌐 Logarithmic Differentiation
The final paragraph of the script focuses on logarithmic differentiation, specifically differentiating a variable raised to another variable. It outlines the steps of setting the function equal to the variable raised to the variable, taking the natural log of both sides, and differentiating with respect to x. The paragraph concludes with an example of finding the derivative of x raised to the x using logarithmic differentiation.
Mindmap
Keywords
💡Derivative
💡Constant
💡Power Rule
💡Constant Multiple Rule
💡Polynomial Function
💡Rational Function
💡Chain Rule
💡Product Rule
💡Quotient Rule
💡Implicit Differentiation
💡Logarithmic Differentiation
Highlights
The derivative of any constant is always zero.
The power rule states that the derivative of x raised to the nth power is n times x to the (n-1)th power.
The constant multiple rule is used to find the derivative of a constant multiplied by a function.
The derivative of a polynomial function is found by applying the power rule to each term and summing the results.
The derivative of a rational function is found by using the power rule and simplifying the resulting expression.
The derivative of a radical function is found by rewriting the expression and applying the power rule.
The derivative of the square root of x is 1/2 times the square root of x.
Trigonometric function derivatives are found by applying specific rules for sine, cosine, tangent, cotangent, secant, and cosecant.
The derivative of a natural log function is found by using the formula u prime divided by u.
The derivative of a regular logarithmic function is found using the formula u prime divided by u times the natural log of the base a.
The derivative of an exponential function with base e is found by multiplying the function by the derivative of the variable.
The product rule states that the derivative of a function multiplied by another function is the derivative of the first times the second plus the first times the derivative of the second.
The quotient rule is used to find the derivative of a function divided by another function by using the formula v*u prime - u*v prime over v squared.
The chain rule is used for composite functions and involves differentiating the outer function and then multiplying by the derivative of the inner function.
Implicit differentiation involves differentiating both sides of an equation with respect to x to find the derivative of one variable with respect to another.
Related rates involve differentiating with respect to time, not x, and are used to find the rate of change of related variables over time.
Logarithmic differentiation is a method for finding the derivative of a function where the variable is raised to the power of another variable.
The derivative of x raised to the power of x is found by setting y equal to x to the power of x, taking the natural log of both sides, and differentiating with respect to x.
Transcripts
Browse More Related Video
Basic Differentiation Rules For Derivatives
Basic Derivative Rules - Part 2: Combining the Rules

AP Calculus AB and BC Unit 3 Review [Differentiation: Composite, Implicit, and Inverse Functions]
Calculus 1 - Derivatives
Derivatives of Composite Functions - Chain Rule, Product & Quotient Rule
Derivatives of Trigonometric Functions - Product Rule Quotient & Chain Rule - Calculus Tutorial
5.0 / 5 (0 votes)
Thanks for rating: