DERIVATIVES that keep getting HARDER 🤯 | jensenmath.ca
TLDRThe video script presents a mathematical journey through the calculation of eleven progressively challenging derivatives. Starting with a basic polynomial function, the video delves into the application of various differentiation rules, including the power rule, product rule, quotient rule, and chain rule. It explores derivatives of functions involving exponential, logarithmic, trigonometric, and inverse hyperbolic functions. The script also tackles composite functions and the use of implicit differentiation. Each derivative calculation is meticulously explained, with an emphasis on simplifying expressions and recognizing mathematical identities. The video concludes with a complex derivative involving an inverse tangent function and an inverse hyperbolic sine function, encouraging viewers to test their mathematical skills and share their experiences in the comments.
Takeaways
- 📚 Start with simple polynomial functions: The video begins with the differentiation of a basic polynomial, emphasizing the use of the power rule for each term.
- 📈 Rewrite expressions for easier differentiation: The presenter suggests rewriting terms to make the application of the power rule more straightforward.
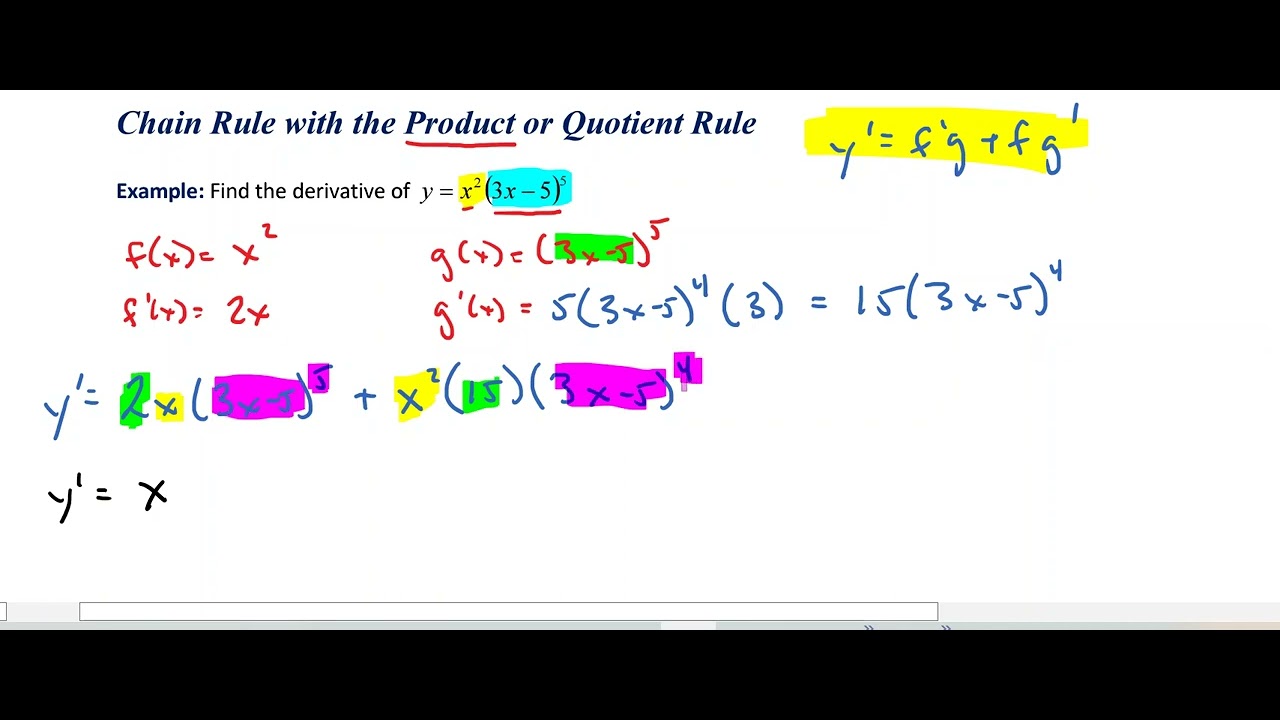
- 🔢 Apply the product rule for multiplying functions: When two functions are multiplied, the product rule is used, combining the derivatives of each function.
- 🔄 Use the quotient rule for dividing functions: For a quotient of two functions, the quotient rule is applied, involving the derivatives of both the numerator and the denominator.
- 🔶 Recognize and apply trigonometric identities: The Pythagorean identity is used to simplify expressions involving trigonometric functions.
- 🏗️ Chain rule for composite functions: When differentiating composite functions, the chain rule is applied, which involves differentiating the outer function and then multiplying by the derivative of the inner function.
- 🤝 Factoring to simplify derivatives: Factoring common terms in expressions can simplify the process of differentiation and the final expression.
- 🧮 Implicit differentiation for undefined relationships: When y is not explicitly defined in terms of x, implicit differentiation is used, taking the derivative of both sides of an equation with respect to x.
- 📐 Differentiate logarithmic and exponential functions: Understanding the derivatives of logarithmic and exponential functions is crucial, with the latter involving the natural logarithm and the function inside the exponent.
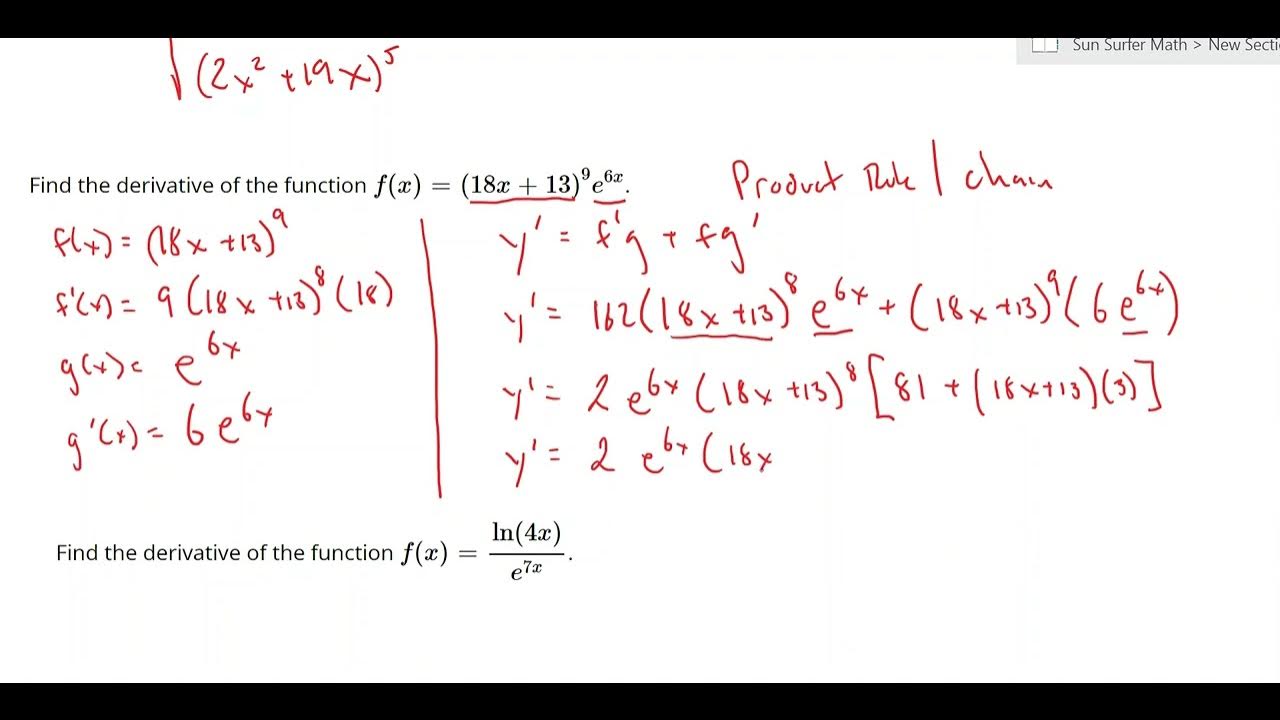
- 🤔 Combine differentiation rules for complex expressions: For more complex expressions, multiple differentiation rules (e.g., product, chain, and quotient rules) are combined to find the derivative.
- 🧲 Use identities and properties of functions: Properties of functions, such as the Pythagorean identity for trigonometric functions, are used to simplify derivatives and make them easier to compute.
Q & A
What is the power rule used for differentiation?
-The power rule is used to differentiate functions of the form x^n, where you can take the exponent, multiply it by the coefficient, and then reduce the exponent by 1.
How does the derivative of a constant compare to the derivative of a variable?
-The derivative of a constant is zero, whereas the derivative of a variable, such as x, is 1.
What is the product rule of differentiation?
-The product rule states that the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function.
How do you differentiate a quotient of two functions?
-The quotient rule states that the derivative of a quotient is the derivative of the top function times the bottom function minus the top function times the derivative of the bottom function, all divided by the bottom function squared.
What is the Pythagorean identity mentioned in the script?
-The Pythagorean identity mentioned in the script is sin^2(x) + cos^2(x) = 1, which is used to simplify trigonometric expressions.
How is the chain rule applied when differentiating composite functions?
-The chain rule states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function. It is used when the base of the power is a function of x itself.
What is the derivative of sin^3(x) with respect to x?
-The derivative of sin^3(x) with respect to x is found using the chain rule, resulting in 3sin^2(x)cos(x).
How do you differentiate e^(x) when x is a function of another variable?
-When differentiating e^(x) and x is a function of another variable, you multiply e^(x) by the derivative of x with respect to that variable.
What is the derivative of the square root of e^x?
-The derivative of the square root of e^x is half of e^(x/2) times the derivative of x/2, which simplifies to e^(x/2)/2√e^x.
How do you approach differentiating a function using implicit differentiation?
-Implicit differentiation is used when y is not explicitly defined in terms of x. You differentiate all terms with respect to x and then solve for dy/dx, often resulting in an equation that you can rearrange to isolate dy/dx.
What is the derivative of arctan(x^x)?
-The derivative of arctan(x^x) involves using the chain rule and the derivative of arctan, which is 1/(1 + x^(2x)) times the derivative of x^x, which is x^x * ln(x) + x.
How do you differentiate the inverse hyperbolic sine function with respect to x?
-The derivative of the inverse hyperbolic sine function with respect to x uses the chain rule and is found to be 1/√(tanh^2(x) + 1) times the derivative of tanh(x), which is sech^2(x).
Outlines
😀 Derivative Calculations: Polynomials to Trigonometry
The video begins with the presenter introducing a series of 11 derivative calculations, each increasing in difficulty. The audience is encouraged to comment on their progress and which derivatives they find challenging. The first derivative involves a simple polynomial function, where each term is differentiated using the power rule. The second derivative is a more complex expression involving terms with fractional and radical expressions, which are simplified using the power rule. The third derivative uses the product rule for two functions multiplied together, and the process involves expanding and collecting like terms. The fourth derivative showcases the quotient rule with trigonometric functions, and the presenter simplifies the expression using the Pythagorean identity. The fifth derivative involves a product of two functions and the use of the product rule, including a composite function requiring the chain rule.
🤔 Advanced Derivatives: Chain Rule and Quotient Rule
The sixth derivative involves a power of a function where the base is a quotient of two functions, necessitating the use of both the chain rule and the quotient rule. The seventh derivative is more complex, with three terms that are differentiated independently, including the use of the chain rule for a trigonometric function cubed. The eighth derivative requires rewriting terms and applying the exponent rule, while the ninth derivative involves a product rule for a function of x times another function of x, including the use of trigonometric identities. The tenth derivative is the first to use implicit differentiation, as y is not explicitly defined in terms of x, and involves logarithmic differentiation and the use of product rule for powers of e.
🧐 Complex Derivatives: Implicit Differentiation and Exponential Functions
The eleventh and most difficult derivative involves an inverse tangent function, an inverse hyperbolic sine function, and an x to the power of x term. The presenter starts by finding the derivative of x to the power of x by rewriting it as an exponential function and applying the product rule. The derivative of the inverse tangent function is found using the derivative of arctan(x), and the inverse hyperbolic sine function's derivative is found by applying the chain rule and using the derivative of tan(x). The final expression is simplified by using trigonometric identities and the properties of absolute values, resulting in a complex but complete derivative.
Mindmap
Keywords
💡Derivative
💡Power Rule
💡Product Rule
💡Quotient Rule
💡Chain Rule
💡Trigonometric Functions
💡Rational Exponents
💡Implicit Differentiation
💡Inverse Functions
💡Exponential Functions
💡Logarithmic Functions
Highlights
Calculation of 11 derivatives with increasing complexity.
Use of power rule for differentiating polynomial functions.
Derivative of a function with rational exponents, rewritten for easier application of the power rule.
Application of the product rule in differentiation, including the handling of composite functions.
Utilization of the quotient rule and trigonometric function derivatives.
Derivation of a product of two functions using the product rule and chain rule for composite functions.
Differentiation of a power of a quotient using both the chain rule and quotient rule.
Independent differentiation of multiple terms, including the use of trigonometric identities.
Exponentiation of e to a function and application of the exponent rule.
Differentiation of a logarithmic function using the properties of logarithms and the quotient rule.
Implicit differentiation applied to an equation where y is not explicitly defined in terms of x.
Differentiation of a function involving an inverse trigonometric function and an inverse hyperbolic function.
Rewriting x to the power of x as an exponential function to facilitate differentiation.
Use of the chain rule to differentiate composite functions involving trigonometric and hyperbolic functions.
Derivation of a logarithmic function with argument involving the inverse tangent function.
Final derivative calculation of a complex function involving multiple rules and substitutions.
Engagement with the audience by asking for their experience and what series they would like to see next.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: