Basic Derivative Rules - Part 2: Combining the Rules
TLDRThe video transcript outlines a comprehensive tutorial on advanced derivative rules in calculus. It begins with a continuation of basic derivative rules, moving on to more complex problems that involve combining several rules such as the power rule, sum and difference rules, constant multiple rule, and the derivative of a constant rule. The speaker demonstrates how to apply these rules term by term, particularly focusing on the manipulation of terms involving fractions and radicals. The process involves algebraic rewriting of functions to isolate variables in the numerator and express them with rational exponents before differentiating. The video also covers the conversion between radical and rational exponent forms, and the handling of negative exponents. The speaker provides step-by-step instructions for finding derivatives, emphasizing the importance of understanding the underlying algebraic principles. Additionally, the transcript touches on the derivatives of exponential functions (e^x) and logarithmic functions (log x), which are fundamental in calculus. The tutorial is designed to equip viewers with the skills to tackle more complex derivative problems, encouraging them to practice the methods presented and compare their work with the solutions provided.
Takeaways
- 🔢 The video provides an advanced tutorial on applying various derivative rules such as the power rule, sum and difference rules, constant multiple rule, and the derivative of a constant.
- 📚 Each derivative rule is demonstrated through detailed examples, showing step-by-step how to combine these rules to solve more complex calculus problems.
- 🧮 Negative and rational exponents are extensively handled, showing how to manipulate these in both the numerator and denominator to simplify expressions before differentiating.
- ✍️ The process of rewriting functions with radicals and rational exponents into more manageable forms for differentiation is clearly outlined.
- 🔍 Examples include converting negative exponents and handling functions expressed with fractions to prepare for applying derivative rules.
- 📐 Specific derivative calculations are demonstrated, including adjusting the power of x and managing constants effectively.
- 🧑🏫 The educator suggests practical tips, like pausing the video to attempt solving problems independently before proceeding with the tutorial.
- 📉 Each derivative problem is followed by a detailed explanation of how to rewrite the derivative in a form that matches the original function, emphasizing the importance of maintaining the expression's structure.
- 🧐 Complex derivative problems involving decimals and negative exponents are addressed, providing solutions that incorporate the use of calculators for simplification.
- 👩🎓 The video concludes with a segment on applying derivative rules to exponential and logarithmic functions, highlighting the simplicity of these derivatives compared to polynomial functions.
Q & A
What is the main focus of the video?
-The video focuses on solving more complicated derivative problems by combining various basic derivative rules such as the power rule, sum and difference rules, constant multiple rule, and the derivative of a constant rule.
What is the power rule used for finding derivatives?
-The power rule is used to find the derivative of a function in the form of f(x) = x^n, where n is a constant. It states that the derivative f'(x) = n * x^(n-1).
How does the sum and difference rule apply to derivatives?
-The sum and difference rule states that the derivative of a sum or difference of functions is the sum or difference of their derivatives, i.e., (f(x) ± g(x))' = f'(x) ± g'(x).
What is the constant multiple rule in the context of derivatives?
-The constant multiple rule states that the derivative of a constant times a function is the constant times the derivative of the function, i.e., (c * f(x))' = c * f'(x), where c is a constant.
What is the derivative of a constant?
-The derivative of a constant is always zero because a constant does not change with respect to the variable.
How does the video approach the problem with fractions?
-The video approaches problems with fractions by combining the rules term by term and applying the power rule to each term, taking care to handle the exponents and constants appropriately.
What is the process for rewriting a function with radicals in the denominator?
-The process involves moving the variables from the denominator to the numerator, ensuring they are raised to a power, and then applying the derivative rules. After finding the derivative, the result may be rewritten back into radical form to match the original problem.
What is the derivative of an exponential function e^x?
-The derivative of an exponential function e^x is e^x, as the exponential function is its own derivative.
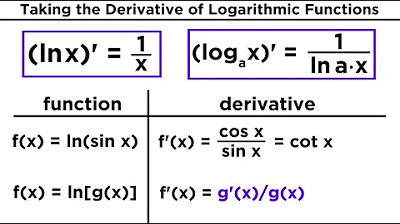
What is the derivative of the logarithmic function log(x)?
-The derivative of the logarithmic function log(x) is 1/x.
How does the video handle negative exponents in derivatives?
-The video handles negative exponents by rewriting the function to have the variables in the numerator with positive exponents, then applying the derivative rules, and finally rewriting the derivative in a form that matches the original problem, which may involve converting back to negative exponents or radical form.
What does the video suggest for solving problems with decimal exponents?
-The video suggests using a calculator, such as Desmos or a smartphone calculator, to handle decimal exponents in derivative problems.
What is the final advice given in the video for those who find the concepts confusing?
-The video advises viewers who find the concepts confusing to watch additional videos on exponent rules and the switching between radical and rational exponent forms for better understanding.
Outlines
📚 Introduction to Advanced Derivative Rules
This paragraph introduces the continuation of a series of videos on basic derivative rules, focusing on more complex problems. It outlines the use of several rules, such as the power rule, sum and difference rules, constant multiple rule, and the derivative of a constant rule, in a single problem. The process involves applying these rules term by term. The example provided demonstrates the application of these rules to a function involving negative exponents and constants, resulting in a simplified derivative form.
🔢 Dealing with Fractions and Radicals in Derivatives
The second paragraph deals with the process of finding derivatives of functions that include fractions and radicals. It emphasizes the need to rewrite the function to remove radicals and variables from the denominator. The process involves several algebraic steps, including rewriting the function with variables in the numerator and applying the power rule to each term. The paragraph concludes with the derivative of the function, expressed in radical form, to match the original problem's format.
🧮 Negative Exponents and Decimals in Derivatives
This paragraph discusses the handling of negative exponents and decimals in derivative problems. It suggests using a calculator for some of the computations due to their complexity. The process involves multiplying coefficients and applying the power rule, taking care with subtraction to avoid errors. The final answers are left in the form of negative exponents, as they were originally presented in the problem.
🔄 Rewriting Functions and Their Derivatives
The fourth paragraph focuses on rewriting functions and their derivatives to match the original problem's form. It involves converting negative exponents to positive and moving them to the numerator. The power rule and other derivative rules are then applied to find the derivative. The paragraph also emphasizes the need to rewrite the derivative in the same form as the original function, which may include expressing terms with radicals.
📈 New Derivative Rules for Exponential and Logarithmic Functions
The final paragraph introduces new derivative rules for exponential and logarithmic functions. It states that the derivative of e to the power of x is e to the power of x, and the derivative of the logarithmic function log of x is 1 over x. These rules are applied to example problems, resulting in simplified derivatives. The paragraph concludes with a note on upcoming applications of derivatives, which will be covered in a subsequent video.
Mindmap
Keywords
💡Derivative
💡Power Rule
💡Sum and Difference Rule
💡Constant Multiple Rule
💡Derivative of a Constant
💡Rational Exponents
💡Rewriting Functions
💡Negative Exponents
💡Logarithmic Function
💡Exponential Function
💡Desmos
Highlights
Combining basic derivative rules to solve more complex problems.
Using the power rule, sum and difference rules, constant multiple rule, and derivative of a constant rule in a single problem.
Applying the sum and difference rule term by term to find derivatives.
Power rule application for the term -9x^3, resulting in -27x^2.
Combining the constant multiple rule with the power rule for terms involving constants times x.
Derivative of a constant is zero, simplifying the process.
Handling fractions by applying rules term by term and bringing down exponents.
Rewriting functions to eliminate radicals and variables in the denominator.
Techniques for rewriting derivatives back into the original radical form.
Dealing with negative exponents and converting them to positive exponents in the numerator.
Using algebraic manipulation to simplify derivatives into a more understandable form.
Derivative of exponential functions e^x is e^x itself.
Derivative of logarithmic functions log(x) is 1/x.
Practical applications of derivatives in various problems, which will be covered in another video.
Using calculators or software like Desmos for complex decimal and negative exponent calculations.
The importance of understanding the rules of exponents and switching between radical and rational exponent forms.
The process of rewriting derivatives to match the form of the original problem for clarity and consistency.
Introduction of new derivative rules for exponential and logarithmic functions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: