How To Make a Frequency Polygon

The Organic Chemistry Tutor
14 Jan 201910:23
EducationalLearning
32 Likes 10 Comments

TLDRThe video explains how to construct a frequency polygon for a statistics class. It first walks through creating a frequency distribution table by determining income ranges and tallying the frequencies for each range. It then calculates the midpoints for each income range to plot on the x-axis. Finally, it introduces an additional low and high midpoint, plots the frequencies on the y-axis, connects the points, and explains the importance of starting and ending the line on the x-axis when graphing a frequency polygon.

Takeaways
  • 😀 The first step is to construct a frequency distribution table with income ranges and corresponding frequencies
  • 😊 To find the midpoint of an income range, add the lower and upper bounds then divide by 2
  • 😏 Introduce a midpoint below the lowest actual midpoint and one above the highest midpoint
  • 🤓 On the y-axis plot the frequencies, on the x-axis plot the midpoint values
  • 😮 Give the extra midpoint values frequencies of 0 since they start from the x-axis
  • 👍 Draw a straight line connecting all the plotted points
  • 📈 Other statistical plots that may be covered include stem, leaf, dot, histograms and quantiles
  • 🧐 Additional video topics beyond statistics like chemistry, physics, algebra and calculus
  • 🔍 Videos are organized into playlists by subject for easy search
  • 👋 Thanks for watching and subscribing for more stats video content
Q & A

  • What is a frequency polygon and its purpose in statistics?

    -A frequency polygon is a graphical representation used in statistics to show the distribution of a dataset. It is created by plotting the frequencies of data points against their corresponding midpoints and then connecting these points with straight lines. Its purpose is to provide a visual understanding of the distribution's shape and to identify patterns, trends, or outliers within the data.

  • How do you define the intervals for a frequency polygon?

    -Intervals for a frequency polygon are defined based on the range of data. In the provided script, intervals are set with a width of 20,000 (20k), starting from 20k since there's no income below 20k. Each interval includes the lower bound but not the upper bound, so a value exactly at the interval's upper limit falls into the next interval.

  • Why are midpoints calculated for constructing a frequency polygon?

    -Midpoints are calculated for constructing a frequency polygon to accurately represent the center of each interval on the graph. They are used as the x-axis values where the corresponding frequencies (y-axis values) are plotted. This approach helps in visualizing the distribution's trends and patterns more clearly.

  • How is the midpoint of an interval calculated?

    -The midpoint of an interval is calculated by adding the lower bound and upper bound of the interval and then dividing by two. This calculation finds the central value of the interval, which is used as the x-coordinate for plotting the frequency of that interval in a frequency polygon.

  • Why are additional points added at the beginning and end of a frequency polygon?

    -Additional points are added at the beginning and end of a frequency polygon, with frequencies set to zero, to visually ground the polygon to the x-axis. These points are calculated by extending the midpoint sequence beyond the actual data range. This practice ensures that the polygon starts and ends on the x-axis, making the distribution's shape clearer and more comprehensive.

  • How do you handle values that fall exactly on the boundary of an interval range?

    -Values that fall exactly on the boundary of an interval range are included in the upper interval. For instance, if an interval is from 20k to 40k (not including 40k), and the next is from 40k to 60k, a value of 40k would fall into the second interval. This convention ensures that each data point is uniquely assigned to a single interval without overlap.

  • What is the significance of plotting frequency values on the y-axis and midpoint values on the x-axis?

    -Plotting frequency values on the y-axis and midpoint values on the x-axis is significant because it allows for a clear representation of how frequently data points occur within each interval across the distribution. This graphical representation helps in visualizing the data's distribution pattern, making it easier to analyze and interpret statistical information.

  • How do you interpret the peaks and valleys in a frequency polygon?

    -Peaks in a frequency polygon represent intervals with higher frequencies, indicating a higher concentration of data points within those ranges. Valleys, on the other hand, represent intervals with lower frequencies, indicating fewer data points. Interpreting these peaks and valleys helps identify the most and least common data ranges, showing the distribution's central tendency and variability.

  • What additional resources does the video creator suggest for viewers interested in statistics?

    -The video creator suggests that viewers interested in furthering their statistics knowledge explore their channel for additional videos on topics such as stem-and-leaf plots, dot plots, histograms, percentiles, quartiles, and calculating the interquartile range. They also mention offering videos on related subjects like general and organic chemistry, physics, and various mathematics topics.

  • Why is it recommended to subscribe and turn on notifications for the video channel?

    -It is recommended to subscribe and turn on notifications for the video channel to stay updated on new video posts related to statistics and other educational content. This ensures viewers do not miss out on future videos that could help them with their studies or interests in statistics and other academic subjects.

Outlines
00:00
📊 Constructing a Frequency Table

The first step is to construct a frequency distribution table. The table has two columns - one for income ranges and one for frequency values. The ranges start at 20k and increase by 20k up to 120k. The frequencies are counted based on how many data values fall within each range.

05:01
📈 Calculating Midpoints and Plotting the Polygon

The next step is to calculate the midpoint for each income range by averaging the lower and upper bounds. Two additional midpoints below the lowest and above the highest are added. The frequencies are plotted on the y-axis and midpoints on the x-axis. Finally, the points are connected with straight lines to create the frequency polygon.

10:02
💡Tips for Creating More Stats Videos

The narrator recommends subscribing and enabling notifications to see more stats videos in the future. Other video topics like stem plots, histograms, quartiles etc. are mentioned. Videos on chemistry, physics, math are also available.

Mindmap
Keywords
💡frequency polygon
A frequency polygon is a type of graph used in statistics to visualize the distribution of data values. In the context of this video, it refers to a graphical representation of the income distribution data, with income ranges on the x-axis and frequencies (counts) on the y-axis. The polygon is obtained by connecting each midpoint on the x-axis to its corresponding frequency on the y-axis.
💡income distribution
The income distribution refers to how income is spread out within a population. The video aims to construct a visual representation (frequency polygon) of the incomes of individuals in a town. Analyzing income distribution gives insights into characteristics like inequality and income disparity in a population.
💡frequency
In statistics, frequency refers to the number of times a value occurs in a data set. In this video, it represents the number of people that fall within the defined income ranges (e.g. 5 people earn $20k to $40k). Plotting frequencies allows analyzing and visualizing the income distribution.
💡interval
Interval refers to the consecutive, non-overlapping income ranges defined in the analysis (e.g $20k-$40k, $40k-$60k etc.). Defining intervals is important for grouping the data and determining frequencies to construct the frequency distribution table.
💡class width
Class width or interval width refers to the size of each interval/income range. For example, the analysis uses a uniform interval width of $20,000 for grouping the incomes. Class width impacts how the data is aggregated and thus the shape of the distribution.
💡midpoint
The midpoint of an interval is the number that divides it into two equal parts. Midpoints represent the central value in each interval (e.g. $30k for $20k-$40k range). Plotting midpoints rather than interval limits gives a better, smoother representation of the distribution.
💡statistics
Statistics is the scientific discipline focused on collecting, analyzing and making inferences from quantitative data. This video demonstrates statistical concepts like distributions, frequencies, midpoints to visually analyze income data.
💡histogram
A histogram is another graph used to visualize distributions, similar to a frequency polygon. While polygons connect midpoints directly, histograms connect interval frequencies in bars. The video mentions it as a related distribution graph.
💡interquartile range
The interquartile range measures the spread of the middle 50% of data values. It gives information regarding variability and dispersion. The video script references it as another statistical analysis tool for income data.
💡dot plot
A dot plot visualizes the distribution of data points as dots above a number line. It gives a sense of clustering or gaps within the full range of values. The script mentions it as an alternative graphical representation tool.
Highlights

First significant highlight text

Second notable highlight text

Third innovative method introduced

Fourth theoretical contribution detailed

Fifth impact of research discussed

Transcripts

Browse More Related Video

Elementary Statistics - Chapter 2 - Exploring Data with Tables & Graphs

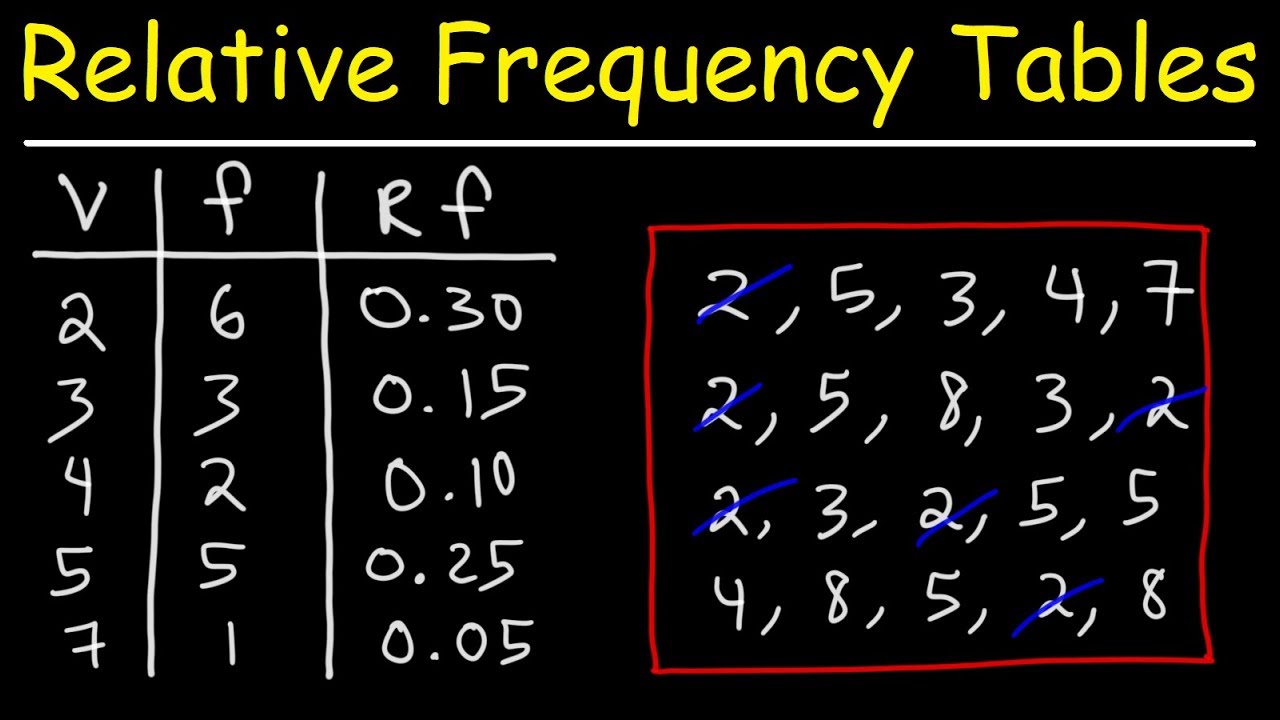
How To Make a Relative Frequency Distribution Table

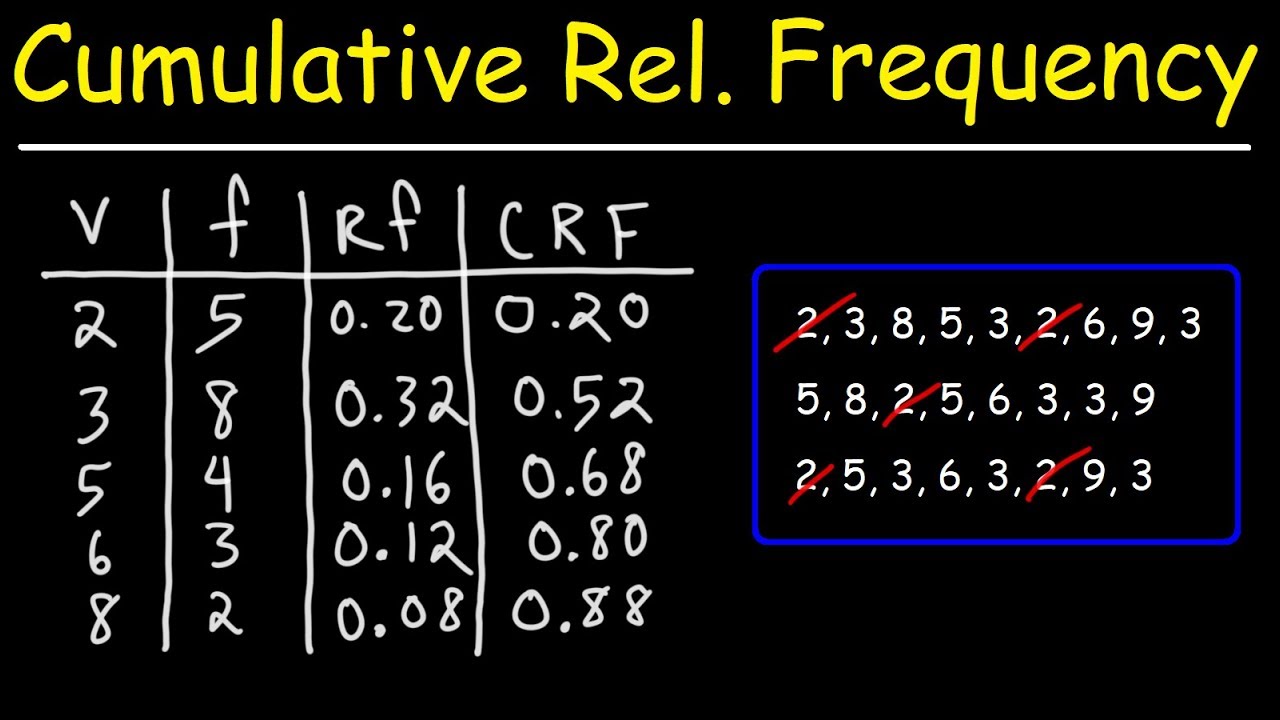
How To Make a Cumulative Relative Frequency Table

Histograms and Density Plots for Numeric Variables | Statistics Tutorial | MarinStatsLectures

Mean, Median, and Mode of Grouped Data & Frequency Distribution Tables Statistics

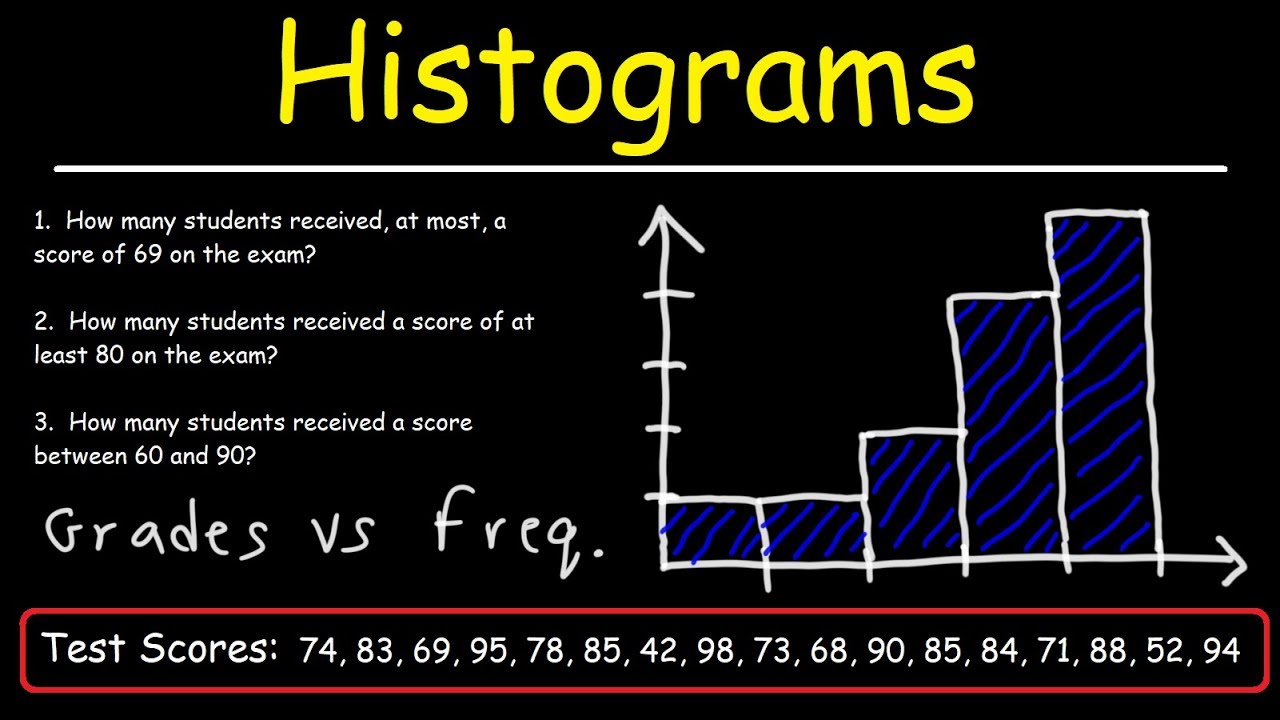
How To Make a Histogram Using a Frequency Distribution Table

Rate This

5.0 / 5 (0 votes)

Thanks for rating: