Probability of Mutually Exclusive Events With Venn Diagrams

The Organic Chemistry Tutor
29 Mar 201911:15
EducationalLearning
32 Likes 10 Comments

TLDRThis educational lesson dives into the concept of mutually exclusive events, explaining that these events cannot occur simultaneously due to the absence of shared outcomes. By using practical examples, such as rolling a six-sided die and analyzing outcomes of various events (A, B, and C), the video clarifies how to determine if events are mutually exclusive or not. It illustrates this through Venn diagrams and delves into calculating probabilities of event occurrences, both mutually exclusive and not. Through example problems involving a six-sided die and events with overlapping outcomes, the lesson elaborates on calculating probabilities of combined events, highlighting the formula for events that are not mutually exclusive and adjusting it for mutually exclusive scenarios, thereby offering a comprehensive understanding of this fundamental probability concept.

Takeaways
  • 😀 Mutually exclusive events cannot occur at the same time.
  • 😇 Events are mutually exclusive if they do not share any outcomes.
  • 📝 To determine if events are mutually exclusive, find if their intersection is ø.
  • 🧮 The probability of mutually exclusive events occurring together is 0.
  • 📊 Use Venn diagrams to represent mutually exclusive and non-mutually exclusive events.
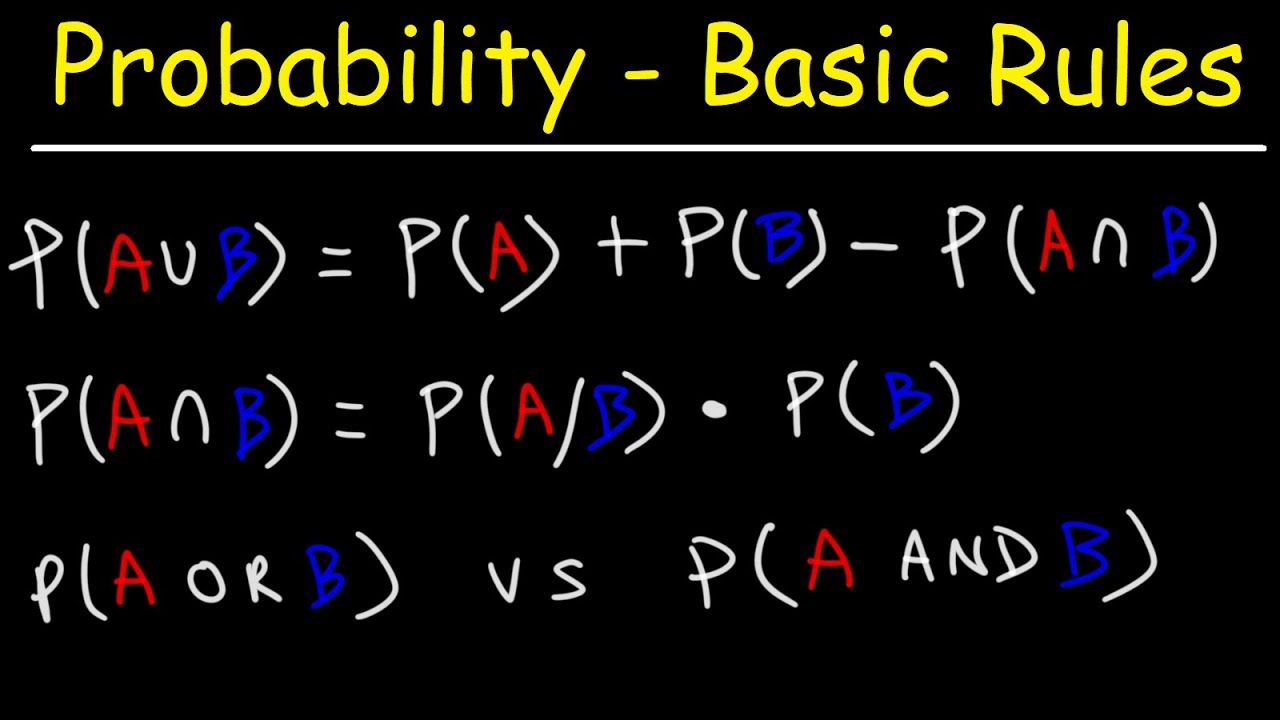
  • 🤔 Calculate P(A or B) as P(A) + P(B) - P(A∩B) for non-mutually exclusive events.
  • ⚠️ For mutually exclusive events, P(A or B) simplifies to P(A) + P(B).
  • 🎲 Applied example of calculating probability with dice outcomes.
  • ✅ Check if probability makes sense based on sample space.
  • 📋 Formula works for both mutually exclusive and non-mutually exclusive events.
Q & A

  • What defines mutually exclusive events?

    -Mutually exclusive events are events that cannot occur at the same time, meaning they do not share any outcomes.

  • How can you determine if two events are mutually exclusive?

    -To determine if two events are mutually exclusive, you need to check if their intersection is empty. If they do not share any outcomes, they are mutually exclusive.

  • What is the probability of two mutually exclusive events occurring together?

    -The probability of two mutually exclusive events occurring together is always zero.

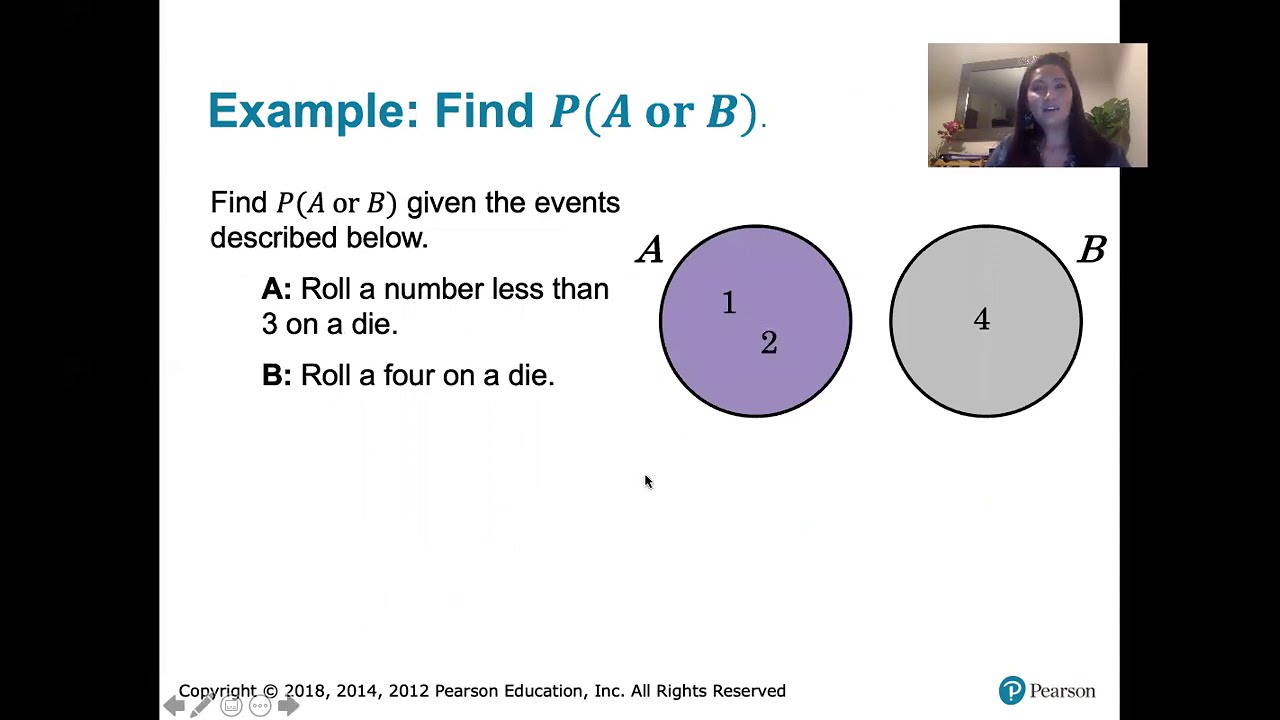
  • Using the example of rolling a six-sided die, are events A (outcomes 1, 2, 3) and B (outcomes 5, 6) mutually exclusive?

    -Yes, events A and B are mutually exclusive because they do not share any outcomes.

  • Can events A (outcomes 1, 2, 3) and C (outcomes 3, 4, 5) be considered mutually exclusive?

    -No, events A and C are not mutually exclusive because they share an outcome, which is the number 3.

  • How do you calculate the probability of event A or event B occurring if they are not mutually exclusive?

    -The probability of event A or event B occurring is calculated as the probability of A plus the probability of B minus the probability of A and B occurring together (their intersection).

  • What modification does the formula for calculating the probability of A or B undergo when A and B are mutually exclusive?

    -When A and B are mutually exclusive, the formula simplifies to the sum of the probabilities of A and B, since their intersection is zero.

  • How is the sample space of A and B defined in the context of mutually exclusive events?

    -The sample space of A and B in the context of mutually exclusive events is the set of all outcomes they can produce without any overlap, as they cannot occur together.

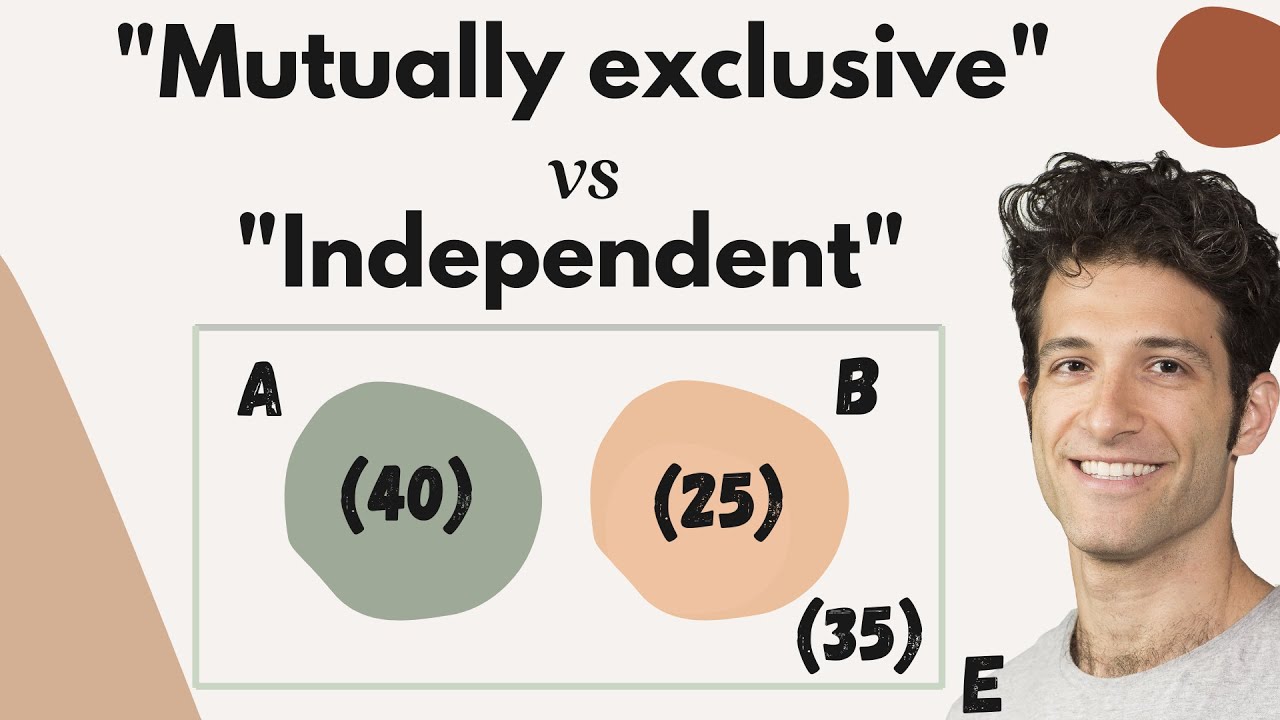
  • What is the significance of a Venn diagram in understanding mutually exclusive events?

    -A Venn diagram visually represents how events are related to each other. For mutually exclusive events, their circles do not overlap, indicating they have no outcomes in common.

  • In the example provided, what is the probability of event B or C occurring, considering event C has the outcome 6?

    -Since events B and C do not share any outcomes, they are mutually exclusive. The probability of B or C occurring is the sum of their individual probabilities, which is 4 out of 6 or 2 out of 3.

Outlines
00:00
🎲 Understanding Mutually Exclusive Events

This segment introduces the concept of mutually exclusive events in probability, defined as events that cannot occur simultaneously. Through the illustration of rolling a six-sided die, it explains that events A and B, with distinct outcomes, do not share any outcomes, making them mutually exclusive with a combined occurrence probability of zero. Conversely, events A and C share an outcome, demonstrating that they are not mutually exclusive. The importance of the intersection of events and its effect on their probability of occurring together is emphasized, providing a foundational understanding of how mutually exclusive events are identified and their implications in probability calculations.

05:00
🔢 Applying Mutually Exclusive Concepts with Venn Diagrams

The script expands on the mutually exclusive event concept by applying it to Venn diagrams and probability calculations. It describes how to calculate the probability of event A or B occurring when the events are not mutually exclusive, using the formula that subtracts the probability of their intersection from the sum of their individual probabilities. Through examples involving a six-sided die, it illustrates the calculation process and clarifies the application of the formula in different scenarios, including when events are mutually exclusive, thereby solidifying the understanding of how probabilities are computed for complex events.

10:02
📊 Exploring Probability with Mutually Exclusive and Non-Exclusive Events

This part delves deeper into the probability calculations for mutually exclusive and non-exclusive events using Venn diagrams and specific examples. It presents a scenario involving events B and C, which are mutually exclusive, demonstrating how their probability of occurring together is calculated. The explanation clarifies that for mutually exclusive events, the formula simplifies to the sum of their individual probabilities since their intersection is zero. Through practical examples, this segment reinforces the concepts of probability calculation, mutual exclusivity, and the application of these principles in solving probability problems.

Mindmap
Keywords
💡mutually exclusive events
Events that cannot occur at the same time. For example, when rolling a die, getting a 1 and getting a 6 on the same roll would be mutually exclusive outcomes. The video explains that mutually exclusive events have no shared outcomes and therefore a zero probability of occurring together.
💡sample space
The set of all possible outcomes for an experiment or event. For a 6-sided die, the sample space would be the numbers 1 through 6 representing the possible values that could be rolled. The video uses sample spaces to calculate probabilities.
💡intersection
The outcomes that two events have in common. To determine if events are mutually exclusive, you check if their intersection is empty (no shared outcomes). For example, events A and B have no intersection so they are mutually exclusive.
💡probability
A number from 0 to 1 representing the likelihood of an event occurring. Mutually exclusive events have a probability of 0 of occurring together. Non-mutually exclusive events have a non-zero probability of intersection.
💡venn diagram
A visual representation using overlapping circles to depict sets and their relationships. The video shows how venn diagrams illustrate whether events are mutually exclusive based on if their circles intersect.
💡union
The combined set of all elements in multiple sets. When finding the probability of A or B, you are taking the union of the events and calculating the probability of that overall set.
💡favorable outcomes
The outcomes from the sample space that meet the criteria for the event in question. For example, if event A is rolling 1-4, the favorable outcomes would be 1, 2, 3 and 4.
💡equation
The video teaches the equation for finding the probability of A OR B occurring for non-mutually exclusive events. This calculation uses the individual probabilities minus the intersection.
💡simplifies
For mutually exclusive events, the equation can be simplified to just the sum of the individual probabilities, since the intersection is 0.
💡makes sense
The video emphasizes conceptually thinking through the probabilities to check if the math aligns with what makes intuitive sense.
Highlights

Researchers developed a new method to rapidly sequence complete genomes from single cells.

The technique enables efficient and accurate analysis of genomic variations between individual cells.

Results showed the approach reliably detected genomic abnormalities and copy number variations down to the single cell level.

Researchers were able to sequence the genomes of over 200 single neurons from post-mortem human brain tissue.

The single neuron genomes showed somatic variation patterns related to neurodegenerative disease processes.

The method allows massively parallel single cell genome sequencing at substantially lower costs.

This enables new research into genomic heterogeneity in complex tissues like brain, cancer, and during development.

Overall, this is a major advance in single cell genomics that will enable many new insights into tissue heterogeneity.

Researchers discuss potential clinical applications for detecting mutations in circulating tumor cells.

Limitations include technical errors in single cell whole genome amplification steps.

Further improvements to genome coverage and accuracy are needed before broad clinical use.

The approach complements single cell RNA sequencing methods for multi-omics analysis.

Overall this represents a major technical leap in single cell genomics.

It enables system-wide study of genomic heterogeneity at single cell resolution.

This will lead to many new insights into tissue development, disease processes, and improved clinical diagnosis.

Transcripts

Browse More Related Video

4.2.3 Addition and Multiplication Rules - Disjoint Events and Implications for Probability

"Mutually Exclusive" and "Independent" Events (...are VERY different things!)

Chapter 4 Probability Part 2

AP Stats Test Quick Review: Probability

Elementary Stats Lesson #8

Multiplication & Addition Rule - Probability - Mutually Exclusive & Independent Events

Rate This

5.0 / 5 (0 votes)

Thanks for rating: