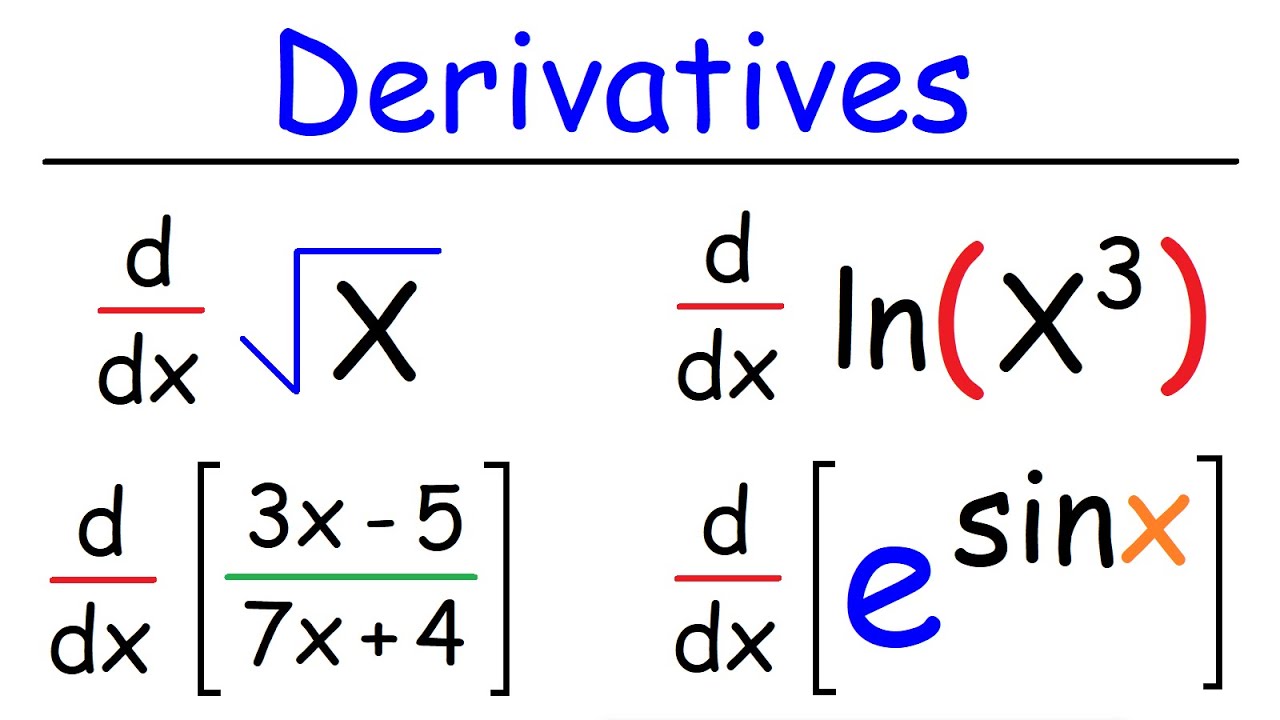Calculus 1 - Derivatives
TLDRThis comprehensive lesson delves into the concept of derivatives, explaining their role in determining the slope of a function at a specific point. It introduces the power rule for finding derivatives of monomials and the constant multiple rule for constants multiplied with a function. The lesson progresses to illustrate how to differentiate polynomial functions, rational functions, and radical expressions. It also touches on the derivatives of trigonometric functions and concludes with explanations of the product and quotient rules, providing examples to solidify understanding.
Takeaways
- 📌 The derivative of a constant is always zero.
- 📈 A derivative represents the slope of a function at a specific x-value.
- 🔢 The power rule states that the derivative of x^n is n*x^(n-1).
- 📦 The constant multiple rule is used to find the derivative of a monomial: (c*f(x))' = c*f'(x).
- 🔄 Understanding the difference between tangent and secant lines is crucial: a tangent line touches the curve at one point, while a secant line touches at two points.
- 🌐 The slope of the tangent line can be approximated using the slope of the secant line as the two points approach the point of tangency.
- 📊 To find the derivative of a polynomial, differentiate each term separately using the power rule and combine the results.
- 📂 For rational functions, rewrite the function to have the variable in the denominator and then apply the power rule.
- 📉 The derivatives of trigonometric functions are fundamental: (d/dx) sin(x) = cos(x), (d/dx) cos(x) = -sin(x), etc.
- 📌 The product rule is essential for differentiating the product of two functions: (f*g)' = f'*g + f*g'.
- 📌 The quotient rule is used for differentiating the quotient of two functions: (f/g)' = (g*f' - f*g')/g^2.
Q & A
What is the derivative of a constant?
-The derivative of any constant is equal to zero.
What does a derivative represent?
-A derivative is a function that gives you the slope at a specific x value.
What is the power rule for finding the derivative of a monomial?
-The power rule states that the derivative of a variable X raised to a constant N is equal to N times X raised to the N minus 1.
How do you find the derivative of a function that is a constant multiple of a monomial?
-You use the constant multiple rule, which states that the derivative of a constant times a function is the constant times the derivative of that function.
What is the slope of the tangent line at a point on a curve represented by the derivative?
-The derivative at a specific x value represents the slope of the tangent line at that point on the curve.
How do you find the derivative of a polynomial function?
-You differentiate each monomial separately using the power rule and then combine the results, adding or subtracting as needed based on the original polynomial.
What is the relationship between a tangent line and a secant line?
-A tangent line touches the curve at exactly one point, while a secant line touches the curve at two points.
How do you find the derivative of a radical function?
-You rewrite the radical function as a rational exponent using the rule that the nth root of X is equivalent to X to the 1/n, and then apply the power rule.
What is the product rule for derivatives?
-The product rule states that the derivative of a product of two functions is the derivative of the first function times the second, plus the first function times the derivative of the second.
What is the quotient rule for derivatives?
-The quotient rule states that the derivative of a quotient of two functions is the denominator times the derivative of the numerator minus the numerator times the derivative of the denominator, all divided by the square of the denominator.
What are the derivatives of the basic trigonometric functions sine and cosine?
-The derivative of sine X is cosine X, and the derivative of cosine X is negative sine X.
Outlines
📚 Introduction to Derivatives
This paragraph introduces the concept of derivatives, explaining that they represent the slope of a function at a particular x-value. It begins with the derivative of constants, which is zero, and progresses to the power rule for finding derivatives of monomials. The power rule states that the derivative of X to the N is N times X to the N minus 1. Examples are provided to illustrate the application of this rule for different powers, including the derivative of x squared, x cubed, and higher powers.
🔢 Applying the Power Rule and Constant Multiple Rule
This section delves deeper into the application of the power rule and the constant multiple rule for finding derivatives. It explains how to find the derivative of a monomial by applying the power rule and demonstrates this with examples of different powers. The constant multiple rule is introduced to handle the derivative of a constant times a function, which is the constant times the derivative of the function. The paragraph also provides practice examples to reinforce the understanding of these rules.
📈 Confirming Derivatives Using Limits
This part of the lesson discusses an alternative method for confirming derivatives, specifically focusing on the definition of the derivative. It explains the concept of limits and how they can be used to verify the derivative of a function, such as confirming that the derivative of x squared is 2x. The process involves taking the limit as h approaches zero of the difference quotient, which simplifies to the slope of the tangent line at a given x-value.
🤔 Understanding Tangent and Secant Lines
The paragraph explains the difference between tangent and secant lines, emphasizing that a tangent line touches the curve at a single point, while a secant line touches at two points. It describes how the slope of a secant line can approximate the slope of a tangent line by choosing points whose midpoint is the x-value of interest. The concept is illustrated with examples, showing how the slope of the tangent line at a specific x-value can be found using the derivative function.
📝 Deriving Polynomial Functions
This section focuses on finding the derivative of polynomial functions. It outlines the process of differentiating each term of a polynomial separately using the power rule and constant multiple rule. The paragraph provides examples of polynomial functions and their derivatives, demonstrating how to combine the derivatives of individual terms to find the derivative of the entire function.
📊 Calculating Slopes of Tangent Lines
The paragraph discusses how to calculate the slope of a tangent line at a specific x-value using the derivative of a function. It provides examples of functions and their derivatives, and then shows how to evaluate the derivative at a given x-value to find the slope of the tangent line. The process is demonstrated with both simple and more complex functions, reinforcing the application of derivatives in determining slopes of tangent lines.
📚 Derivatives of Rational Functions
This section introduces the process of finding derivatives of rational functions, where the function is a fraction with polynomials in the numerator and denominator. The paragraph explains how to rewrite the function in a way that allows the application of the power rule. It provides examples of rational functions and their derivatives, showing the step-by-step process of moving the variable to the denominator and simplifying the resulting expression.
🌟 Derivatives of Radical Functions
The paragraph discusses the process of finding derivatives of radical functions, which involve roots or fractional exponents. It explains how to rewrite radical expressions as rational exponents and then apply the power rule. The section includes examples of finding derivatives of square roots, cube roots, and higher root functions, demonstrating the step-by-step process and the final simplified form of the derivatives.
🔄 Solving Complex Derivative Problems
This part of the lesson tackles more complex derivative problems, including those with mixed terms and those that require the use of the product rule for multiple functions being multiplied together. The paragraph provides examples of how to apply the product rule, explaining the process of differentiating each part of the function and then combining them according to the rule. It also introduces the quotient rule for dividing one function by another.
📐 Derivatives of Trigonometric Functions
This section introduces the derivatives of basic trigonometric functions, providing the formulas for the derivatives of sine, cosine, secant, and cotangent. It also offers a mnemonic to help remember the signs of the derivatives based on the presence of the letter 'C' in the function name. The paragraph emphasizes the importance of knowing these derivatives for future applications.
📈 Advanced Derivative Calculations
The paragraph presents advanced examples of derivative calculations, including the product rule for three functions and the quotient rule for dividing one function by another. It provides a step-by-step explanation of how to apply these rules, including the need to differentiate each part of the function and combine them according to the rules. The paragraph concludes with a challenge problem that applies the product rule to a function involving three parts.
📊 Working with Fractions and Quotient Rule
This final section focuses on the quotient rule for finding the derivative of a fraction. It explains the formula for the derivative of F divided by G, which involves the numerator and denominator of the fraction, their derivatives, and the square of the denominator. The paragraph provides a detailed example of how to apply the quotient rule, including the steps of simplifying the expression and combining like terms to arrive at the final answer.
Mindmap
Keywords
💡Derivative
💡Power Rule
💡Constant Multiple Rule
💡Monomial
💡Tangent Line
💡Limit Definition of Derivative
💡Secant Line
💡Polynomial
💡Chain Rule
💡Product Rule
Highlights
Derivative of a constant is zero.
Derivative as a function that gives slope at an x value.
Derivative of x squared is 2x using the power rule.
Derivative of monomials using the power rule: nx^(n-1).
Derivative of a constant multiple: constant times the derivative of the function.
Confirmation of derivative of x^2 using the limit definition.
Derivative gives the slope of the tangent line at a specific x value.
Difference between tangent and secant lines explained.
Derivative of polynomial functions by differentiating each monomial separately.
Derivative of rational functions by rewriting the function and applying the power rule.
Derivative of radical functions by rewriting the expression as a rational exponent and applying the power rule.
Derivative of trigonometric functions: sine, cosine, secant, and cotangent derivatives provided.
Product rule for derivatives: (fG)' = f'G + fG'.
Quotient rule for derivatives: (F/G)' = (Gf' - F'G) / G^2.
Chain rule mentioned for future learning.
Example of using the product rule with three functions.
Detailed explanation of the quotient rule for derivatives with an example.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: