Limits of Rational Functions - Fractions and Square Roots
TLDRThe video script discusses the concept of limits in calculus, focusing on scenarios where direct substitution is not applicable due to undefined expressions. It demonstrates the technique of multiplying by conjugates to simplify radical expressions and rational functions. The script provides detailed examples, including the limit of square root functions and rational expressions as x approaches specific values, and emphasizes the importance of factoring and simplifying before applying direct substitution to find the limits. The examples are worked out step by step, leading to the final answers, which are presented clearly.
Takeaways
- 📚 When dealing with limits that involve undefined expressions, direct substitution may not be applicable.
- 🔢 To address undefined expressions, use the technique of multiplying by the conjugate of the radical expression.
- 🌟 For limits as x approaches a value, the expression should be simplified before direct substitution can be applied.
- 📈 When the limit involves a radical, factorization (FOIL method) is often used to simplify the radical expression.
- 🤔 In some cases, after simplifying, the limit can be evaluated by canceling out common factors between the numerator and the denominator.
- 🧠 The process of rationalizing the numerator or denominator can be necessary when dealing with square roots in the final simplified expression.
- 📊 When approaching a limit with a rational function, look for common denominators to combine fractions and simplify the expression.
- 🔄 Factor out the greatest common factor (GCF) to further simplify expressions before evaluating limits.
- 🎯 Always rewrite the limit expression with the variable approaching the point of interest to facilitate the evaluation process.
- 🛠️ Practice and repetition are key to mastering the techniques for evaluating limits, especially with complex rational functions.
- 📋 The script provides a step-by-step approach to solving limit problems, emphasizing the importance of understanding the underlying mathematical principles.
Q & A
What is the limit of (sqrt(x) - 2) / (x - 4) as x approaches 4?
-We cannot use direct substitution because plugging in x=4 results in division by zero. Instead, we multiply the numerator and denominator by the conjugate of the numerator, (sqrt(x) + 2). This results in the limit of (x - 4) / (sqrt(x) + 2) as x approaches 4. After simplification, we can directly substitute x=4 to get the final answer of 1/4.
How do you handle a limit with a radical when direct substitution results in an undefined expression?
-You should multiply both the numerator and the denominator by the conjugate of the radical expression. This process, known as rationalizing the denominator, eliminates the radical in the numerator and allows for direct substitution.
What is the conjugate of sqrt(x) - 2?
-The conjugate of sqrt(x) - 2 is sqrt(x) + 2. Conjugates are found by changing the sign of the radical part in the expression.
What is the limit of (sqrt(x) + 2) / (x - 4) as x approaches 4?
-After simplifying the expression by multiplying both the numerator and the denominator by the conjugate of the numerator, we get (x - 4) / (sqrt(x) + 2). Now, using direct substitution with x=4, the limit is 1 / (sqrt(4) + 2), which simplifies to 1/4.
What is the limit of (sqrt(x) + 2) / x as x approaches 0?
-We cannot directly substitute x=0 as it would result in division by zero. Instead, we multiply the numerator and the denominator by the conjugate of the numerator, which is (sqrt(x) - 2). After simplification and canceling terms, we can use direct substitution. With x=0, the limit is (sqrt(0) + 2) / 2, which simplifies to 2/2 or 1.
How do you rationalize the denominator of a limit expression involving a radical?
-To rationalize the denominator, you multiply both the numerator and the denominator by the conjugate of the radical in the denominator. This process eliminates the radical and allows for further simplification or direct substitution.
What is the conjugate of sqrt(x) + 2 when finding the limit as x approaches 0?
-The conjugate of sqrt(x) + 2 is sqrt(x) - 2. This is used to eliminate the square root from the denominator when finding limits that would otherwise be undefined.
What is the limit of (sqrt(x) + 2) / (x) as x approaches 0?
-By multiplying the numerator and the denominator by the conjugate of the numerator, (sqrt(x) - 2), we get a new expression to evaluate. After canceling terms and simplifying, we can substitute x=0 directly into the new expression to find the limit, which is 1.
How do you find the limit of a rational function when direct substitution leads to an undefined denominator?
-You can multiply the numerator and the denominator by the common denominator of the fractions in the function to simplify the expression. After this, you can cancel out common factors and use direct substitution to find the limit.
What is the common denominator of the fractions 1/(x-3) and 1/3?
-The common denominator is 3x. By multiplying both the numerator and the denominator of the function by this common denominator, we can simplify the expression and find the limit as x approaches 3.
What is the limit of (1/(x-3)) / (x / 3) as x approaches 3?
-By multiplying the numerator and the denominator by the common denominator 3x, we simplify the expression to 3 / x. As x approaches 3, we can use direct substitution to find the limit, which is 3 / 3, simplifying to 1.
How do you handle a limit that has a common denominator but direct substitution leads to division by zero?
-You should factor the denominator and cancel out common factors to simplify the expression. After this, you can use direct substitution to find the limit as the variable approaches the given value.
What is the limit of (1/(x+2) - 1/2) / x as x approaches 0?
-By multiplying the numerator and the denominator by the common denominator (x+2) * 2, we can simplify the expression. After canceling terms and simplifying, we can substitute x=0 directly into the new expression to find the limit, which is -1/4.
Outlines
📚 Limit Calculations with Radicals
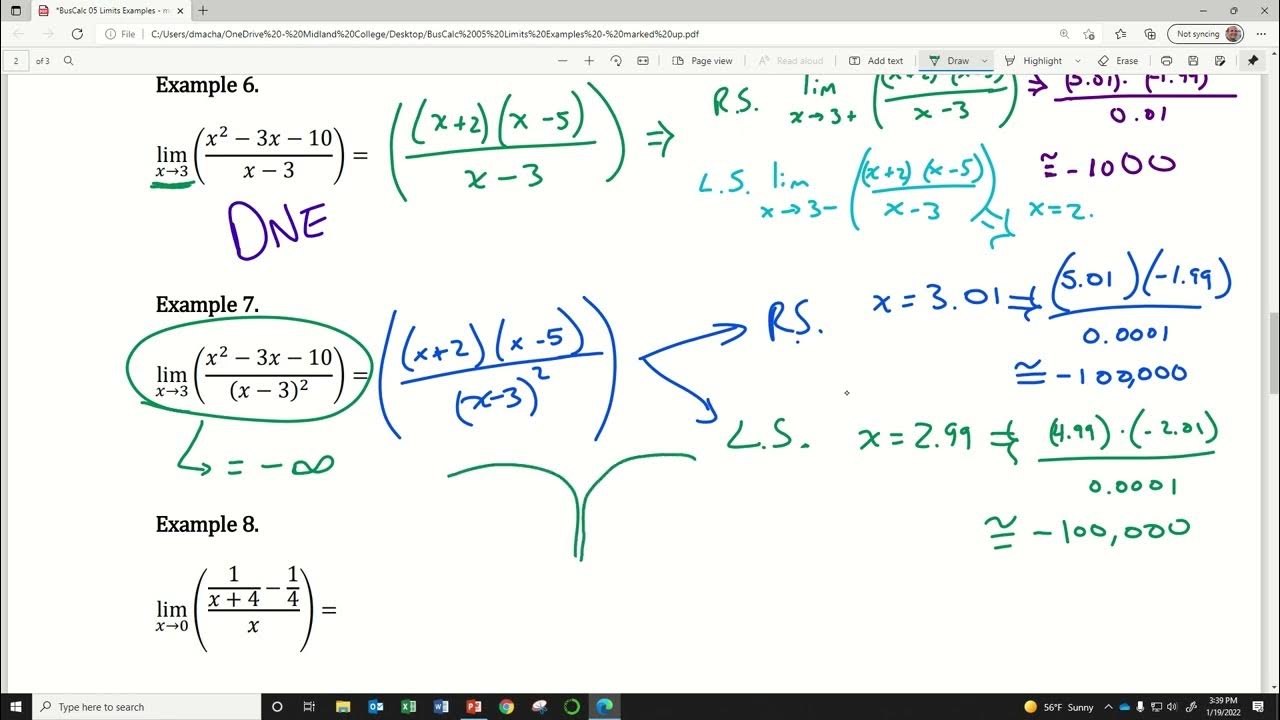
This paragraph discusses the process of calculating limits involving square roots and rational functions. It begins with a problem where the limit of (sqrt(x) - 2) / (x - 4) as x approaches 4 cannot be found using direct substitution because it would result in division by zero. Instead, the technique of multiplying by the conjugate is used to simplify the expression. The process involves 'foiling' the numerator and keeping the denominator unchanged. After simplification, the limit is found using direct substitution, resulting in the value of 1/4. The paragraph then moves on to another limit problem, illustrating the same technique for a different function, emphasizing the importance of handling radical expressions carefully to avoid undefined functions.
📘Limit Calculations with Rational Functions
This paragraph focuses on calculating limits of rational functions, particularly when direct substitution leads to an undefined expression. The first example involves finding the limit as x approaches 3 for the function (1/(x - 3)) / (x - 3). By multiplying the numerator and denominator by the common denominator 3x, the expression is simplified to 3/(3x), and the limit is found by direct substitution, resulting in the value of -1/9. The paragraph then presents a similar problem, where the limit as x approaches 0 for the function (1/(x + 2)) - (1/2) / x is calculated. The common denominator (x + 2) is used to simplify the expression, and after canceling terms, the limit is found to be -1/4. The explanation emphasizes the importance of factoring and simplifying rational functions to avoid undefined values and to find the limit.
Mindmap
Keywords
💡limit
💡direct substitution
💡conjugate
💡FOIL
💡rational functions
💡factoring
💡GCF (Greatest Common Factor)
💡indeterminate forms
💡simplification
💡cancellation
💡rationalize
Highlights
The limit of a function as x approaches a certain value is a fundamental concept in calculus.
When a function is undefined at the point of interest, direct substitution cannot be used to find the limit.
Multiplying by the conjugate is a technique to simplify radical expressions and find limits.
The process of factoring and canceling out terms is crucial for simplifying expressions and evaluating limits.
Direct substitution can be used when the simplified expression does not result in an undefined value.
The limit of a function can reveal insights into the behavior of the function near specific points.
Rational functions often require manipulation to find limits, such as multiplying by a common denominator.
The concept of limits is essential for understanding the continuity and properties of functions.
The process of rationalizing the numerator is a technique used to simplify radical expressions in limits.
The limit of a function at a point can be found by transforming the expression to cancel out the undefined term.
The use of factoring and the common factor property is vital in simplifying rational functions to find limits.
When dealing with limits, it's important to be mindful of the domain of the function and where it is undefined.
The process of simplifying and canceling terms can lead to the discovery of patterns in limit problems.
The concept of limits is not only theoretical but also has practical applications in various fields of mathematics and science.
The ability to find limits is a key skill in calculus that helps in understanding the behavior of functions at critical points.
The process of simplifying radical expressions and rational functions demonstrates the versatility of algebraic techniques in calculus.
The method of multiplying by the conjugate is a powerful tool for dealing with limits involving square roots and other radicals.
The transcript provides a comprehensive guide to finding limits using various algebraic techniques, showcasing the depth and complexity of calculus.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: