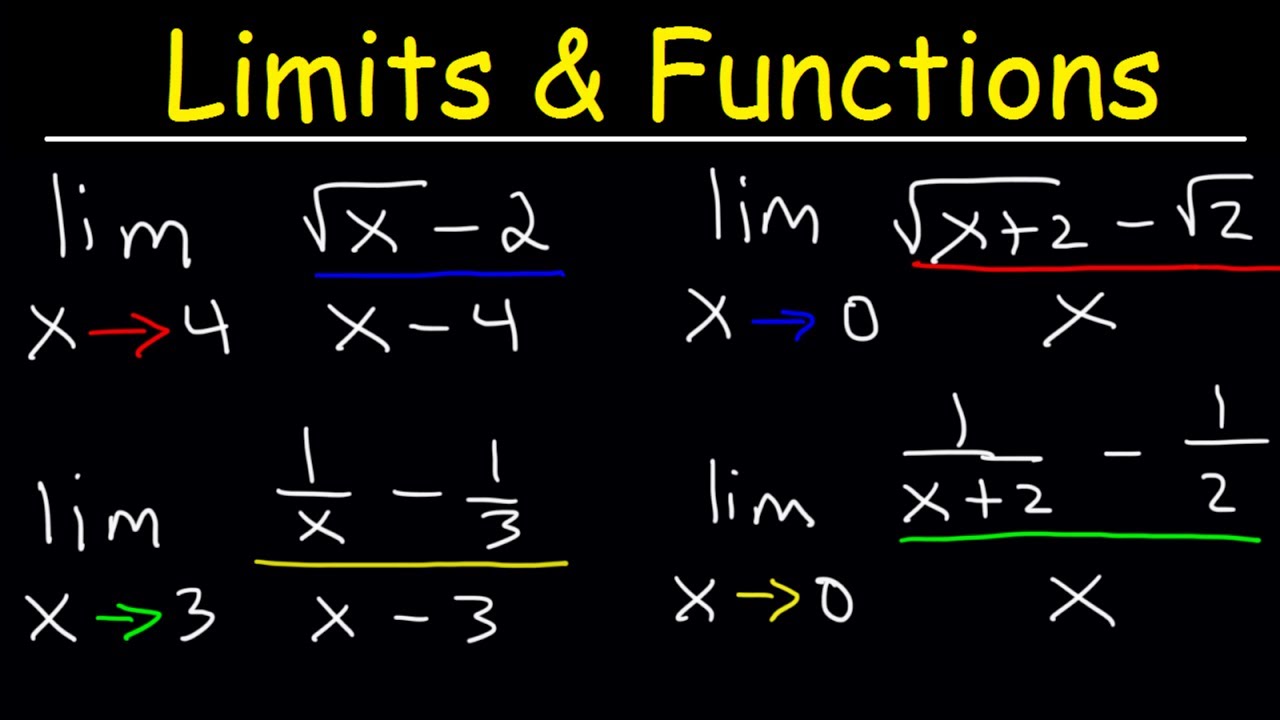
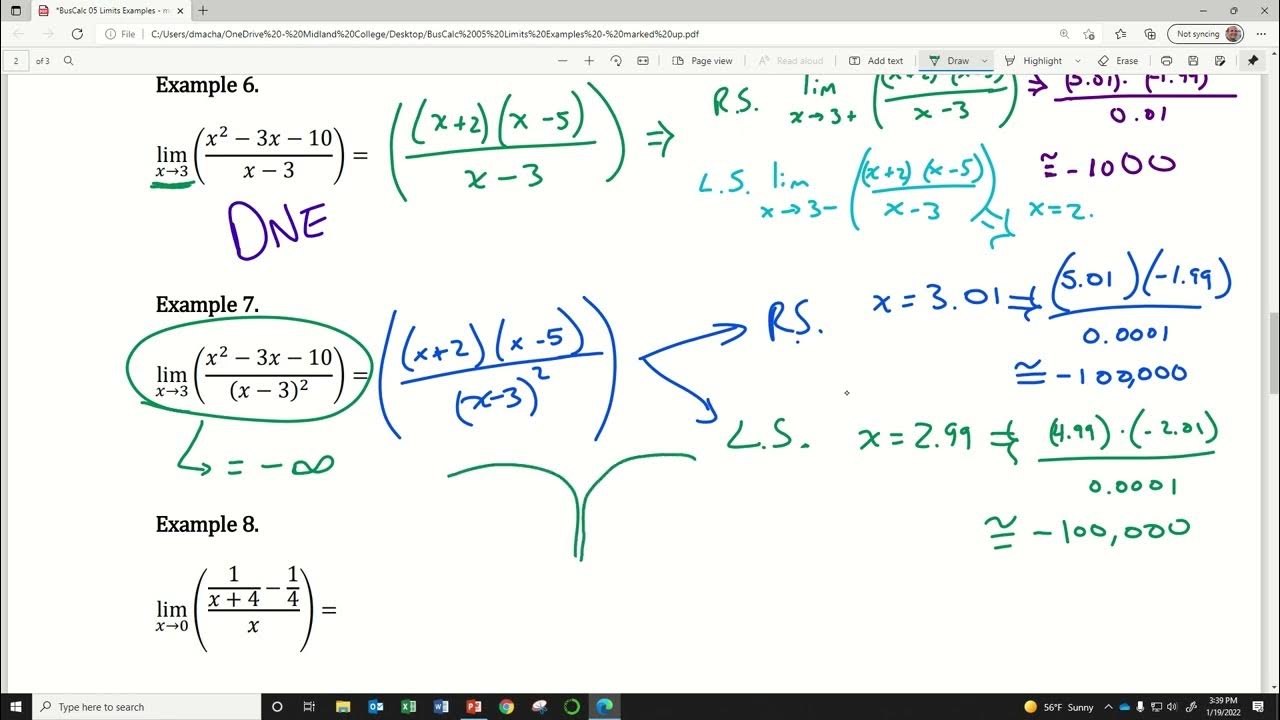
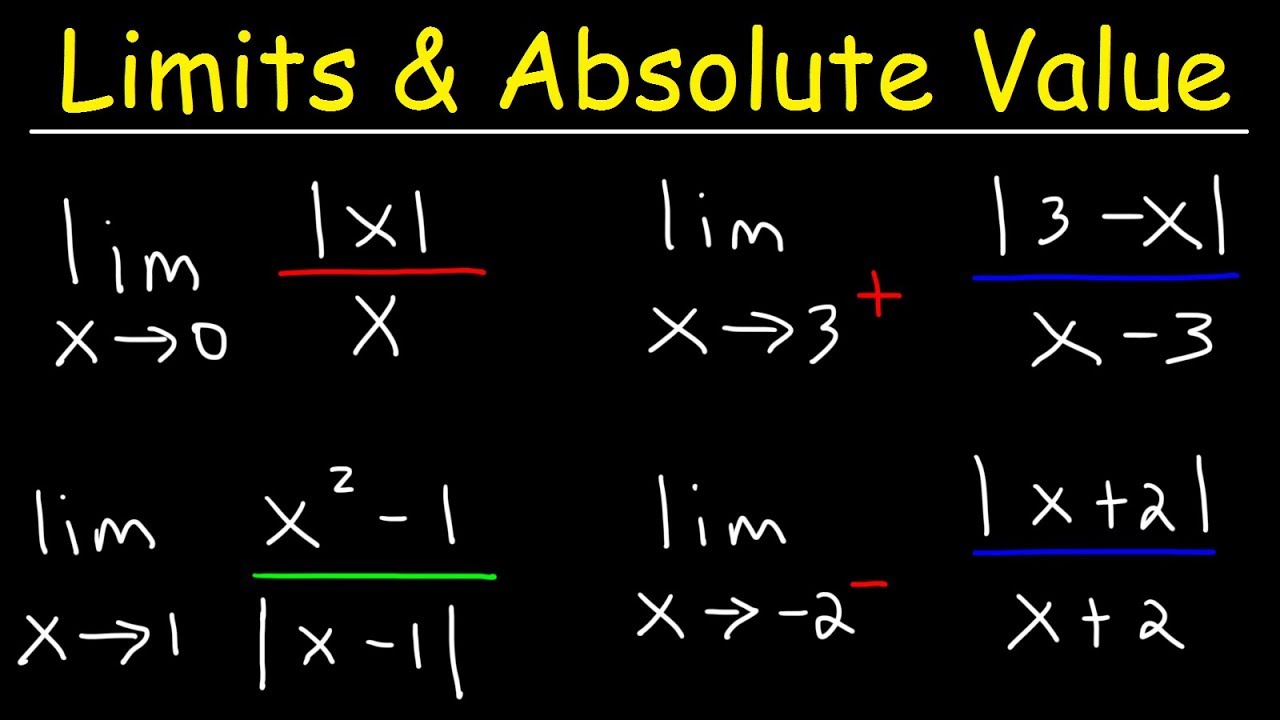Introduction to Limits

The Organic Chemistry Tutor
30 May 201711:08
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the concept of limits in mathematics, illustrating how to find limits of various functions as the input approaches specific values. It differentiates between direct substitution and scenarios where the function is not defined at a point, using examples to demonstrate how to approach and calculate limits. The examples span polynomial, rational, and trigonometric functions, highlighting the technique of direct substitution and its effectiveness in solving limit problems.

Takeaways
  • πŸ“Œ Limits are a fundamental concept in calculus that describe the behavior of a function as the input approaches a certain value.
  • πŸ” When a function is not defined at a point, such as x=2 for the function (x^2 - 4)/(x - 2), we can't use direct substitution but must find the limit by approaching the value from nearby points.
  • πŸ“ˆ By plugging in values close to the point of interest (e.g., 1.9, 1.99, 1.999), we can observe how the function behaves and determine the limit as x approaches that point.
  • 🌟 The limit of a function can be found analytically through direct substitution if it results in a determinate form, such as for the polynomial function (x^2 + 7x + 3) as x approaches 3.
  • πŸ”’ For rational functions, like (x^2 - 3x + 4)/(x + 2), direct substitution can be used to find the limit as x approaches a value that does not render the denominator zero.
  • πŸ“Š Direct substitution is a straightforward technique that can be applied to various types of functions, including polynomials, rational functions, and trigonometric functions.
  • πŸ“ To convert radians to degrees, multiply the radian measure by (180/Ο€), which is useful for evaluating trigonometric limits at specific angles.
  • πŸ“ The limit of a trigonometric function can be found using known values from the unit circle or by performing algebraic operations if necessary.
  • πŸ”‘ The concept of limits is essential for understanding continuity, derivatives, and integrals in calculus, and it is a key topic that may appear in various forms on a calculus test.
  • πŸ’‘ Understanding how to find and evaluate limits is crucial for solving problems in calculus, as it helps in determining the behavior of functions at certain points and their asymptotic behavior.
Q & A

  • What is the concept of a limit in mathematics?

    -A limit in mathematics is a fundamental concept that describes the behavior of a function when the input, or variable, approaches a particular value. It is used to understand the value that a function approaches as the input gets arbitrarily close to a certain point.

  • Why can't we directly substitute the value of x when it approaches 2 in the function (x^2 - 4) / (x - 2)?

    -Direct substitution is not possible in this case because it results in a division by zero, which is undefined. When x is exactly 2, the denominator becomes zero, leading to an indeterminate form, hence we cannot find the function's value at x=2.

  • How does the pattern of approaching values help in determining the limit?

    -By plugging in values that are very close to the point of interest (in this case, x=2 and x=3), we can observe how the function's output approaches a specific value. This pattern helps us to determine the limit of the function as x gets arbitrarily close to the given value.

  • What is the limit of the function (x^2 - 4) / (x - 2) as x approaches 2?

    -The limit of the function as x approaches 2 is 4. This is determined by observing that as x gets closer and closer to 2, the expression approaches the number 4.

  • How does direct substitution help in evaluating limits?

    -Direct substitution is a technique where we simply plug the value of the variable that the limit is approaching into the function. If the function is defined at that point and the result is not an indeterminate form like 0/0, we can evaluate the limit analytically, making it a straightforward and useful method for certain types of functions.

  • What is the limit of the function (x^3 - 27) / (x - 3) as x approaches 3?

    -The limit of the function as x approaches 3 is 27. This is found by observing that as x gets closer to 3, the function's output approaches 27.

  • How does the concept of limits apply to polynomial functions?

    -For polynomial functions, the limit as x approaches a certain value can often be found using direct substitution, as long as there are no undefined operations like division by zero. The limit will simply be the result of the polynomial evaluation at that point.

  • What is the limit of the function (x^2 + 7x + 3) as x approaches 3?

    -The limit of the function as x approaches 3 is 33. This is calculated by directly substituting the value of x (which is 3 in this case) into the polynomial function and evaluating it.

  • How do you find the limit of a rational function as x approaches a certain value?

    -To find the limit of a rational function, you first attempt direct substitution. If the result is not an indeterminate form, you can evaluate the limit analytically. If direct substitution results in an indeterminate form, you may need to use other techniques, such as factoring or L'HΓ΄pital's rule.

  • What is the limit of the function (x^2 - 3x + 4) / (x + 2) as x approaches 5?

    -The limit of the function as x approaches 5 is 2. This is found by directly substituting x with 5 in the given rational function and simplifying the result.

  • How do you evaluate the limit of a trigonometric function as x approaches a certain value?

    -To evaluate the limit of a trigonometric function, you can use the properties of trigonometric functions and their values at specific angles. For example, if the function involves sine or cosine, you can use the unit circle or known trigonometric values for standard angles to find the limit.

  • What is the limit of the function (sin x) as x approaches Ο€/4?

    -The limit of the function as x approaches Ο€/4 is the square root of 2 divided by 2, which is obtained by evaluating the sine of Ο€/4 (or 45 degrees) using the unit circle.

Outlines
00:00
πŸ“š Understanding Limits and Direct Substitution

This paragraph introduces the concept of limits in calculus, emphasizing the difference between finding the value of a function at a specific point and the limit of a function as the variable approaches a certain value. It uses the example of the function f(x) = (x^2 - 4) / (x - 2) as x approaches 2, highlighting that direct substitution results in an indeterminate form. Instead, the paragraph demonstrates the method ofι€ΌθΏ‘ (ι€ΌθΏ‘) values to show that the limit is 4. It further explains this with another example involving the function f(x) = (x^3 - 27) / (x - 3) as x approaches 3, where the limit is shown to be 27. The paragraph also discusses the use of direct substitution in evaluating limits for polynomial and rational functions, providing examples for clarity.

05:04
πŸ“ˆ Evaluating Limits of Polynomial and Rational Functions

This paragraph continues the discussion on limits, focusing on polynomial and rational functions. It explains that direct substitution is a straightforward method to find limits when there are no issues with division by zero. The paragraph provides a worked-out example for the function f(x) = x^2 + 7x + 3 as x approaches 3, showing that the limit is 33. It then moves on to rational functions, using the example of f(x) = (x^2 - 3x + 4) / (x + 2) as x approaches 5, and demonstrates that the limit is 2. The paragraph also touches on the importance of recognizing when direct substitution can be applied and when alternative methods may be necessary.

10:04
πŸ“Š Limits of Trigonometric Functions

The final paragraph of the script shifts focus to the limits of trigonometric functions. It begins by explaining how to find the limit of sin(x) as x approaches Ο€/4, converting radians to degrees and using the unit circle to determine the value. The paragraph then addresses the limit of tan(x) as x approaches Ο€/3, explaining the relationship between sine and cosine in the context of tangent. Another example is provided for the secant function, showing the limit as x approaches Ο€/6. The paragraph emphasizes the process of rationalizing the denominator and the use of trigonometric identities to simplify expressions and find the limits of these functions.

Mindmap
Keywords
πŸ’‘limit
In the context of the video, a limit refers to the value that a function approaches as the input (x-value) gets arbitrarily close to a certain point. It is a fundamental concept in calculus, used to describe the behavior of functions, particularly when the input reaches certain values. For example, the video discusses finding the limit as x approaches 2 for the function f(x) = (x^2 - 4) / (x - 2), which is shown to be 4 by substituting values close to 2 and observing the pattern that emerges.
πŸ’‘x approaches
The phrase 'x approaches' is used to describe the behavior of a variable (x) that gets closer and closer to a particular value without actually reaching it. This is a key concept in understanding limits, as it implies a process of taking values arbitrarily near to a point of interest. In the video, this concept is used to illustrate how the function's output changes as x nears specific values, such as 2 or 3, to determine the limit of the function at those points.
πŸ’‘direct substitution
Direct substitution is a technique used to evaluate a function at a specific value by simply replacing the variable with that value. In the context of the video, it is a method for finding the limit of a function when the function is defined at the point of interest. For instance, the video demonstrates direct substitution by plugging in x = 3 into the function f(x) = (x^3 - 27) / (x - 3) to find that the limit is 27, as the function output approaches this value as x gets closer to 3.
πŸ’‘indeterminate form
An indeterminate form occurs when an expression simplifies to an undefined state, such as 0/0. This is a situation where the usual rules of arithmetic do not apply, and additional techniques are needed to find a limit. In the video, the author discusses how plugging in x = 2 into the function f(x) = (x^2 - 4) / (x - 2) results in an indeterminate form of 0/0, which requires a different approach to determine the limit, such as analyzing the behavior of the function as x gets close to but not equal to 2.
πŸ’‘polynomial function
A polynomial function is a mathematical function that involves only the variables and constants, with the variable raised to whole number exponents in operations of addition, subtraction, multiplication, and non-negative integer exponents of the variable. In the video, the polynomial function f(x) = x^2 + 7x + 3 is mentioned, and the limit of this function as x approaches 3 is calculated using direct substitution, resulting in the value 33. This demonstrates that polynomial functions can be evaluated at specific points, and their limits can be found by simply plugging in the value of interest.
πŸ’‘rational function
A rational function is a function that can be expressed as the quotient or ratio of two polynomial functions. The video discusses the limit of a rational function as x approaches 5 for f(x) = (x^2 - 3x + 4) / (x + 2). By using direct substitution, the limit is found to be 2, highlighting that while rational functions can have undefined points due to division by zero, their behavior near those points or at other values of x can be analyzed to find limits.
πŸ’‘trigonometric function
Trigonometric functions, such as sine, cosine, and tangent, are mathematical functions based on the relationships between the angles and sides of a triangle. In the video, the limit of the trigonometric function sine x as x approaches Ο€/4 (45 degrees) is discussed. The limit is found to be √2/2, illustrating that trigonometric functions have specific values at particular angles, which can be determined using the unit circle or other trigonometric identities.
πŸ’‘tangent x
The tangent function, denoted as tan(x), is a trigonometric function that represents the ratio of the sine to the cosine of an angle x. In the video, the limit of the function tan(x) as x approaches Ο€/3 (60 degrees) is calculated. By using the definitions of sine and cosine for these angles, the limit is found to be √3, showing how the behavior of the tangent function can be analyzed near specific angles to find its limit.
πŸ’‘secant x
The secant function, denoted as sec(x), is a trigonometric function that represents the reciprocal of the cosine function, or 1/cos(x). In the video, the limit of the function sec(x) as x approaches Ο€/6 (30 degrees) is discussed. By substituting the value for cosine at this angle and rationalizing the resulting expression, the limit is calculated to be 2√3/3, demonstrating how trigonometric functions and their reciprocals can be analyzed to find limits at specific angles.
πŸ’‘unit circle
The unit circle is a circle with a radius of 1 centered at the origin of a coordinate system. It is a fundamental tool in trigonometry, as it allows for the definition of trigonometric functions for all angles. In the video, the unit circle is used to find the value of sine at Ο€/4 (45 degrees), which is √2/2. This illustrates how the unit circle can be used to determine the limits of trigonometric functions at specific angles.
πŸ’‘rationalize the denominator
Rationalizing the denominator is the process of eliminating the radical (root) in the denominator of a fraction, typically by multiplying both the numerator and the denominator by a suitable expression that contains the conjugate of the denominator. In the video, this process is used when calculating the limit of the secant function as x approaches Ο€/6 (30 degrees), where the denominator contains a radical. By rationalizing, the final answer is simplified to a form that does not contain radicals, which is 2√3/3.
Highlights

The concept of limits in mathematics is introduced, providing a foundational understanding for calculus.

The difference between finding the value of a function at a point and finding the limit as a variable approaches that point is explained.

An example is provided to illustrate the indeterminate form of 0/0 when directly substituting the value into a function.

A method of approaching the concept of limits through tables and numerical substitution is demonstrated.

The limit of the function (x^2 - 4)/(x - 2) as x approaches 2 is shown to be 4 through a step-by-step numerical approach.

The process of evaluating the limit of a polynomial function using direct substitution is explained.

An example demonstrates the successful use of direct substitution to find the limit as x approaches 3 for the function x^3 - 27/(x - 3), which is found to be 27.

The importance of direct substitution as a technique for evaluating limits is emphasized.

A rational function's limit as x approaches 5 is calculated using direct substitution, resulting in a limit of 2.

The concept of limits is extended to trigonometric functions, with an example showing the limit of sin(x) as x approaches Ο€/4 to be √2/2.

The limit of the tangent function as x approaches Ο€/3 is calculated, demonstrating the use of trigonometric identities.

The limit of the secant function as x approaches Ο€/6 is found using direct substitution, illustrating the application of trigonometric functions in evaluating limits.

The process of rationalizing the denominator to find the limit of a trigonometric function is explained.

The transcript provides a comprehensive overview of the concept of limits, including various methods and applications in both algebraic and trigonometric functions.

The use of a calculator is suggested for finding the values of trigonometric functions at specific angles.

The transcript emphasizes the potential for direct substitution to be a simple and effective technique for evaluating limits in certain scenarios.

Transcripts

Browse More Related Video

1.2 - Algebraic Limits and Continuity

Limits of Rational Functions - Fractions and Square Roots

BusCalc 05 Limits Examples Pt 2

Calculus 1: Limits & Derivatives (6 of 27) Finding the Limit of a Function - Example 1

Evaluating Limits With Fractions and Square Roots

Limits and Absolute Value

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusLimitsDirect SubstitutionRational FunctionsTrigonometric FunctionsSineCosineTangentMathematicsEducation