BusCalc 05 Limits Examples Pt 2
TLDRThe video script discusses the concept of limits in calculus, focusing on two-sided and one-sided limits, as well as limits at infinity. It explores rational functions and how to handle discontinuities, including point and essential discontinuities. The transcript provides detailed examples of calculating limits, such as factoring the numerator to cancel out terms and simplify expressions. It also covers the use of algebraic manipulation to evaluate limits at points where direct substitution is not possible. Additionally, the script touches on the application of limits in real-world scenarios, like approximating values using Microsoft Excel for a function as x approaches zero. The examples given range from straightforward limit calculations to more complex scenarios involving square roots and exponential functions, illustrating the importance of understanding the behavior of functions at certain points and the concept of limits in calculus.
Takeaways
- 📚 Factoring the numerator of a rational function can help simplify the expression and determine discontinuities.
- 🚫 A rational function is undefined when the denominator equals zero, which creates a point of discontinuity.
- ➗ Cancellation of common factors in the numerator and denominator can simplify rational functions and reveal their behavior as x approaches certain values.
- 🔢 Evaluating limits by direct substitution can be misleading when the function is not defined at the point of interest, such as x = 0 or x = a specific value.
- 🔍 To find the limit as x approaches a certain value, consider the behavior of the function from both the left and right sides to determine if it's a point or essential discontinuity.
- 📈📉 The left-sided and right-sided limits can have different values, leading to an essential discontinuity, which is indicated by the function approaching positive or negative infinity.
- 🧮 Algebraic manipulation can transform expressions to avoid division by zero and allow for the evaluation of limits at points where the function is not directly defined.
- 🛑 A function may not be defined at a point, but the limit as x approaches that point can still exist and be evaluated.
- 💻 Using technology like Microsoft Excel can help approximate limits by observing the convergence of values as x approaches the point of interest.
- 🔢 When approaching infinity, the powers of a number become very small fractions, leading to limits that can be determined by understanding the behavior of exponential functions.
- ➿ The concept of limits is fundamental in calculus, providing a way to understand the behavior of functions at points where they may not be defined or as they extend to infinity.
Q & A
What is the rational function in the numerator of Example 5?
-The rational function in the numerator of Example 5 is x^2 - 3x - 10, which factors into (x + 2)(x - 5).
Why is the function undefined at x = -2 in Example 5?
-The function is undefined at x = -2 because the denominator, which is (x + 2), becomes zero, resulting in division by zero.
How does the behavior of the rational function in Example 5 change when x approaches -2?
-The rational function in Example 5 has a point discontinuity at x = -2. By canceling out the (x + 2) factor from the numerator and denominator, the function behaves like the simpler linear polynomial x - 5 elsewhere, and the limit as x approaches -2 is -7.
What type of discontinuity is present in the function of Example 6 at x = 3?
-In Example 6, the function has an essential discontinuity at x = 3 because the function cannot be simplified to cancel out the (x - 3) factor, and the limit does not exist as x approaches 3 from either side.
How does the right-sided limit of Example 6 behave as x approaches 3?
-The right-sided limit of the function in Example 6 approaches negative infinity as x gets very close to, but remains greater than, 3.
What is the limit as x approaches 0 in Example 8?
-The limit as x approaches 0 in Example 8 is -1/16, which is found by algebraic manipulation and direct substitution after rearranging the expression.
What is the trick used in Example 9 to avoid the indeterminate form 0/0?
-In Example 9, the trick is to multiply the numerator and denominator by the conjugate of the numerator's radical term, which is √x + √5, to eliminate the radical and simplify the expression.
How is the limit in Example 10 approximated to five decimal places?
-The limit in Example 10 is approximated by using Microsoft Excel to create a table of values for x approaching 0 from the right side. The first time the first five decimal places of the calculated values do not change from one row to the next indicates the approximation to five decimal places.
What is the right-sided limit as x approaches 3 in Example 11?
-The right-sided limit as x approaches 3 in Example 11 is 0, because the expression simplifies to the square root of a difference that evaluates to zero.
What is the limit as x approaches positive infinity in Example 12?
-The limit as x approaches positive infinity in Example 12 is 1, because any number raised to the power of 0 is 1.
What is the limit as x approaches negative infinity in Example 13?
-The limit as x approaches negative infinity in Example 13 is 0, because as the exponent becomes increasingly negative, the value of the expression approaches zero.
Outlines
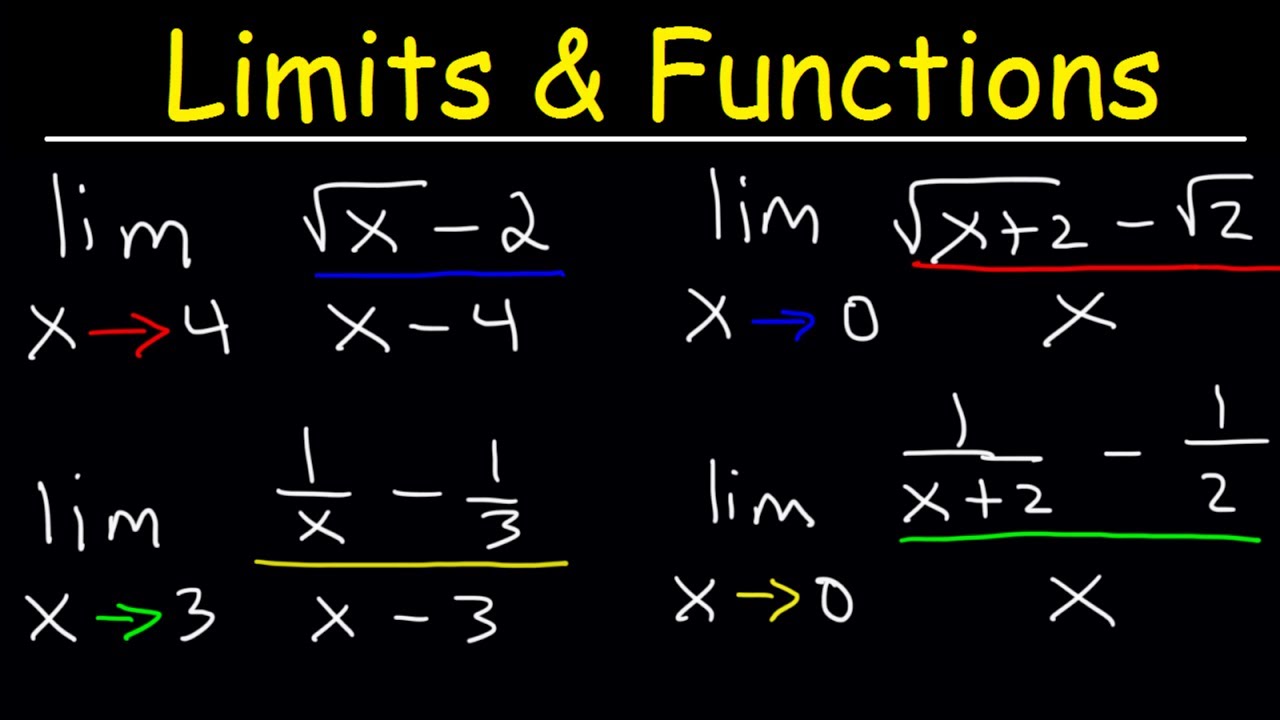
📚 Evaluating Limits of Rational Functions
The paragraph discusses the process of finding the limit of a rational function as x approaches negative two. The function given is (x^2 - 3x - 10) / (x + 2). The speaker suggests factoring the numerator to simplify the expression and then cancels out the common factor with the denominator. They note that the function is undefined at x = -2 due to division by zero but can be simplified to a linear polynomial (x - 5) elsewhere. The limit as x approaches -2 of the simplified function is then evaluated, resulting in -7, indicating a point discontinuity.
🔍 Analyzing Discontinuities in Rational Functions
This section of the script examines a similar rational function to the previous one, but with a different denominator, (x - 3), and focuses on the limit as x approaches 3. The speaker points out that this function has an essential discontinuity at x = 3, as the function cannot be simplified by factoring and the denominator becomes zero at this point. By testing values just above and below 3, the speaker shows that the function approaches negative infinity on the right side and positive infinity on the left side of the discontinuity, confirming that the two-sided limit does not exist.
🧮 Algebraic Manipulation to Find Limits
The speaker approaches a limit problem by algebraically manipulating the expression to avoid division by zero. The limit as x approaches 0 of a function with x in the denominator is considered. By multiplying by an equivalent form of 1, the speaker combines terms over a common denominator, allowing the cancellation of terms. The resulting expression simplifies to a function that can be evaluated at x = 0, yielding a limit of -1/16.
🔢 Approximating Limits Numerically with Excel
The paragraph describes an example where the limit as x approaches 0 of (1 + x)^(1/x) is to be approximated to five decimal places using Microsoft Excel. The speaker sets up a table with decreasing values of x, approaching zero, and calculates the corresponding function values. By observing when the first five decimal places stabilize across consecutive rows, the speaker approximates the limit to be 2.71828, noting the limitations of numerical approximations due to rounding errors in computational math.
🚦 Understanding One-Sided Limits and Imaginary Numbers
The speaker explains a right-sided limit as x approaches 3 for the function √(x^2 - 9). They clarify that a right-sided limit is considered because for x > 3, the function yields real numbers, whereas for x < 3, the function would involve the square root of a negative number, resulting in imaginary numbers. The limit is evaluated directly to be 0, as the expression simplifies to √(0) when x = 3.
∞ Limits at Infinity and Approaching Zero
The paragraph explores the concept of limits as x approaches positive and negative infinity. For positive infinity, the speaker considers the limit of 2^(1/x) and explains that as x grows larger, 1/x approaches zero, and thus 2 to any small power approaches 1. For negative infinity, the sequence of 3 to the power of negative integers is examined, showing that as the exponent becomes more negative, the values approach zero. The speaker concludes that the limit of 3^x as x approaches negative infinity is 0.
Mindmap
Keywords
💡Limit
💡Rational Function
💡Factoring
💡Quadratic Polynomial
💡Point Discontinuity
💡Essential Discontinuity
💡Algebraic Manipulation
💡Microsoft Excel
💡Indeterminate Form
💡Right-Sided Limit
💡Positive Infinity
Highlights
Factoring the numerator of a rational function can simplify the process of finding limits.
A rational function is undefined when the denominator equals zero.
Cancellation of factors in a rational function can lead to a simpler form for limit evaluation.
A point of discontinuity in a function can be identified by the behavior of the function as it approaches that point.
The limit of a function as x approaches a certain value can be evaluated by substitution if the function is defined at that point.
For essential discontinuities, the function's behavior on either side of the point of interest can lead to different outcomes.
The right-sided and left-sided limits of a function can be evaluated separately to understand the function's behavior as it approaches a point from different directions.
Microsoft Excel can be used to approximate the value of a limit by observing when consecutive approximations stabilize.
The concept of a right-sided limit is important when dealing with functions that are not defined for all real numbers.
The limit of a function as x approaches positive infinity can be understood by considering the behavior of the function as x becomes very large.
When x approaches negative infinity, the behavior of functions with negative exponents can be predicted by the increasing size of the denominator.
The limit of an expression involving roots and powers can be found by multiplying by an appropriate form of 1 to simplify the expression.
Computer calculations can become imprecise with very small or large numbers due to the rounding off of decimal places in each operation.
The limit of a rational function can be found even if the function is undefined at a particular point by using algebraic manipulation.
The limit of a function as it approaches a certain point can be understood by evaluating the function at values infinitesimally close to that point.
For limits involving exponential functions, the behavior of the exponent as it approaches zero can simplify the evaluation process.
Understanding the properties of limits, such as the behavior of 1 over x as x approaches infinity, is crucial for evaluating complex limits.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: