Lesson 17 - Improper Integrals (Calculus 1 Tutor)

Math and Science
5 Mar 201604:00
EducationalLearning
32 Likes 10 Comments

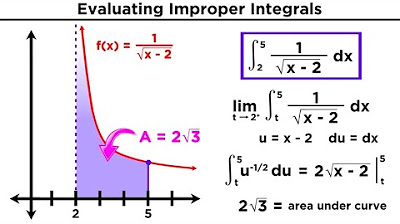
TLDRThis educational video delves into the concept of improper integrals, which involve integrating functions to infinity. It clarifies that while such integrals might seem to result in infinity, certain functions actually converge to a finite value. The video uses the integral from 2 to infinity of 1 over (x + 3)^(1/2) as an example and demonstrates how to solve it through substitution, ultimately highlighting the application of the fundamental theorem of calculus to find the convergent value.

Takeaways
  • 📚 Improper integrals are a type of integral where the limits of integration involve infinity.
  • 🤔 The concept might initially seem counterintuitive, as integrating a function to infinity would naively suggest an infinite result.
  • 🔄 However, if a function decreases rapidly enough as it approaches infinity, the integral can converge to a finite value.
  • 🌟 An example given in the script is the integral from 2 to infinity of 1 over X plus 3 to the power of 1/2 with respect to X.
  • 🧠 Understanding improper integrals is essential for grasping certain mathematical concepts and their applications.
  • 📈 The process of solving an improper integral often involves techniques like substitution and manipulation of the integral expression.
  • 🔢 The derivative of the integrand in the example is utilized to perform the substitution, changing the integral into a more manageable form.
  • 🎓 When evaluating the integral, it's crucial to consider the limits in terms of the original variable, which in this case are 2 and infinity.
  • 🌐 The conversion from X to U is necessary to apply the limits of integration correctly, which involves solving for U when X equals 2 and infinity.
  • 📊 After performing the integration, the fundamental theorem of calculus is applied to evaluate the expression at the given limits.
  • 📱 The final result of the integral is expressed as 2 times U to the one-half evaluated from 5 to infinity, demonstrating the convergence of the improper integral.
Q & A

  • What are improper integrals?

    -Improper integrals are integrals where the limits of integration are infinite, such as from a number to infinity or from negative infinity to infinity.

  • Why does integrating a function to infinity not always result in infinity?

    -Integrating a function to infinity does not always result in infinity because if the function approaches zero as it goes to infinity, the areas calculated by the integral become smaller and may converge to a finite value.

  • What is the integral example given in the transcript?

    -The integral example given in the transcript is the integral from 2 to infinity of 1 over X plus 3 to the 1/2 with respect to X (∫ from 2 to ∞ of 1/(x + 3)^(1/2) dx).

  • How is the integral example approached in the transcript?

    -The integral example is approached by using substitution. The substitution used is X plus 3, and the derivative of this substitution is 1, which allows the integral to be rewritten in terms of a new variable U.

  • What is the role of the fundamental theorem of calculus in evaluating the integral example?

    -The fundamental theorem of calculus is used to evaluate the integral by plugging in the upper and lower limits of integration (5 and infinity, respectively) to find the value of the integral.

  • What is the final result of the integral example given in the transcript?

    -The final result of the integral example is 2 times U to the one-half evaluated from 5 to infinity, which simplifies to 2 times infinity to the one-half minus 2 times 5 to the 1/2.

  • How does the concept of convergence apply to improper integrals?

    -Improper integrals can converge if the function being integrated approaches zero as the limit of integration approaches infinity, resulting in a finite value rather than infinity.

  • What is the significance of the substitution method in solving improper integrals?

    -The substitution method simplifies the integral by transforming it into a more manageable form, often allowing for easier evaluation and determination of convergence or divergence.

  • What happens if the function being integrated does not approach zero as it goes to infinity?

    -If the function being integrated does not approach zero as it goes to infinity, the improper integral will diverge, meaning it does not converge to a finite value.

  • How can one determine if an improper integral converges or diverges?

    -To determine if an improper integral converges or diverges, one must evaluate the limit of the integral as it approaches the infinite or negative infinite limits of integration. If the limit is a finite number, the integral converges; if the limit is infinite, the integral diverges.

  • What is the main takeaway from the transcript regarding improper integrals?

    -The main takeaway is that improper integrals can sometimes converge to a finite value despite having infinite limits of integration, depending on whether the function approaches zero as it goes to infinity.

Outlines
00:00
📚 Introduction to Improper Integrals

This paragraph introduces the concept of improper integrals, which are a type of integral where the limits of integration involve infinity. The explanation begins with an example of the integral from 2 to infinity of 1 over X plus 3 to the 1/2 DX, highlighting the challenge of calculating the area as the integration extends to infinity. It is emphasized that although one might expect an infinite result, certain functions may decrease towards zero and thus converge to a finite value despite the infinite range of integration. The paragraph concludes with a brief mention of solving such integrals using substitution, specifically by changing the variable to U, which simplifies the integral for easier evaluation.

Mindmap
Keywords
💡Improper Integrals
Improper integrals are a type of integral calculation where the limits of integration are infinite, such as from a number to infinity. Unlike conventional integrals that calculate the area under a curve between two finite points, improper integrals deal with the concept of infinity, which can lead to non-convergent results. However, if the function being integrated decreases rapidly enough as it approaches infinity, the integral may converge to a finite value. In the video, the integral from 2 to infinity of 1 over x plus 3 to the 1/2 is an example of an improper integral.
💡Integration
Integration is a fundamental concept in calculus that involves finding the area under a curve or between two points. It is the reverse process of differentiation. In the context of the video, integration is used to calculate the area under a function's curve as it extends to infinity, which is a key aspect of discussing improper integrals.
💡Limits of Integration
The limits of integration are the boundaries or values between which the integration is performed. In standard integrals, these limits are finite numbers. However, in the case of improper integrals, one or both of the limits may be infinite. The video discusses how these infinite limits affect the interpretation and computation of the integral.
💡Convergence
Convergence in the context of improper integrals refers to the situation where the integral of a function from a starting point to infinity results in a finite value. This occurs when the function being integrated approaches zero rapidly enough as x approaches infinity, allowing the area calculated to be finite despite the infinite limit.
💡Substitution
Substitution is a technique used in calculus to simplify integrals by replacing the variable of integration with another variable, often denoted as 'u'. This method is particularly useful when dealing with complicated integrands or when the integral involves expressions that can be simplified through algebraic manipulation. In the video, substitution is used to transform the improper integral into a more manageable form.
💡Derivative
The derivative of a function is a measure of how the function changes as its input changes. It is a fundamental concept in calculus and is used to analyze the rate of change or slope of a function. In the context of the video, the derivative is used as part of the substitution process to find the differential 'du' in terms of 'dx'.
💡Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus is a key result that connects differentiation and integration. It states that if a function is continuous on the interval [a, b] and F is an antiderivative of the function on that interval, then the definite integral of the function from 'a' to 'b' is equal to F(b) - F(a). In the video, this theorem is used to evaluate the improper integral by substituting the limits of integration into the antiderivative.
💡Exponent
An exponent is a mathematical notation that indicates the number of times a base is multiplied by itself. In the context of the video, the exponent 1/2 (or 0.5) is used in the function that is being integrated, affecting how the function behaves as the variable 'x' approaches infinity and thus impacts the convergence of the integral.
💡Area under a curve
The area under a curve refers to the region enclosed by the graph of a function and the x-axis on a Cartesian coordinate system. Integration is the process used to calculate this area. In the video, the concept is extended to calculate the area under a curve as it extends to infinity, which is a central theme when discussing improper integrals.
💡Antiderivative
An antiderivative, also known as an indefinite integral, is a function whose derivative is equal to the original function. In the context of the video, finding the antiderivative of the function being integrated is a crucial step in evaluating the improper integral using the Fundamental Theorem of Calculus.
Highlights

The discussion of improper integrals and their definition involving infinite limits.

The example provided of the integral from 2 to infinity of 1 over X plus 3 to the 1/2 DX.

The explanation of how the area under a curve increases indefinitely when integrating to infinity.

The clarification that integrating to infinity does not always result in infinity.

The scenario where a function starts high and decreases, approaching zero as it goes to infinity.

The concept that integrating an ever-decreasing function can result in a convergent answer.

The method of solving the integral by substitution, changing X to X plus 3.

The derivative calculation and the resulting simplification to 1DX.

The step-by-step integration process, including the change of variables from X to U.

The application of the fundamental theorem of calculus to evaluate the integral at the limits.

The calculation of the final answer, 2 times U to the one-half evaluated from 5 to infinity.

The method of handling the lower limit by substituting the value of X as 2 into the new variable U.

The method of handling the upper limit by considering infinity as a larger version of itself.

The final expression of the integral result, 2 times infinity to the 1/2 minus 2 times 5 to the 1/2.

The demonstration of how improper integrals can yield finite answers despite integrating to infinity.

Transcripts

Browse More Related Video

Introduction to improper integrals | AP Calculus BC | Khan Academy

Evaluating Improper Integrals

Improper Integrals - Infinite Bounds

Improper Integrals - Convergence and Divergence - Calculus 2

Improper Integrals BC Calc

Improper Integrals - Convergent or Divergent (Made Easy)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsCalculusIntegrationInfinityConvergenceEducationLearningSubstitutionFundamental Theorem