Formal definition of limits Part 1: intuition review | AP Calculus AB | Khan Academy

Khan Academy
11 Jan 201303:36
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the concept of limits in mathematics, aiming to enhance the viewer's intuition about this fundamental topic. It begins by visually representing the axes and a generic function that is undefined at a certain point, denoted as 'c'. The discussion then focuses on what happens as the input 'x' approaches 'c', illustrating that the function 'f(x)' seems to get closer to a particular value, termed 'L'. This value 'L' is defined as the limit. The script emphasizes the importance of understanding limits, even when a function is not defined at certain points, and sets the stage for a more mathematically rigorous definition of limits in subsequent videos.

Takeaways
  • 📈 The concept of a limit in mathematics is introduced as a fundamental idea for understanding functions.
  • 📊 The script begins by visually representing limits through a graph, using axes and a hypothetical function.
  • 🚫 The function in the example is not defined at a particular point, denoted as 'c', which adds complexity to the limit concept.
  • 🎯 As 'x' approaches 'c', the value of 'f(x)' seems to get closer to a certain value, illustrating the intuitive idea of a limit.
  • 🠔 The script highlights the importance of considering 'x' approaching 'c' from both the left (x < c) and the right (x > c).
  • 🎨 The visual representation shows that 'f(x)' appears to approach the same value, 'L', from both directions, if it exists.
  • 📝 The limit is formally denoted as 'lim (f(x)) as x approaches c equals L', which is a way to express the behavior of a function near a certain point.
  • 🤔 The script emphasizes that while this is a useful conceptual understanding, it is not a mathematically rigorous definition.
  • 📚 The video promises that future content will provide a more rigorous and formal definition of limits, allowing for proofs and deeper analysis.
  • 🔍 The discussion of limits is foundational for further study in calculus and its applications.
  • 🌟 Understanding limits is crucial for grasping why they are relevant, especially in cases where a function is undefined at certain points.
Q & A

  • What is the basic concept of a limit in calculus?

    -The basic concept of a limit in calculus is understanding what value a function (f(x)) approaches as the input (x) approaches a certain point (c). This is crucial in cases where the function may not be defined at the point c but has a predictable behavior as it gets close to c.

  • In the script, why was a function chosen that is not defined at a point c?

    -A function not defined at a point c was chosen to illustrate the significance of limits in understanding the behavior of functions at points where they may not be explicitly defined. This helps in grasping why limits are relevant and useful in calculus.

  • How does the function's behavior as x approaches c from the left differ from approaching from the right?

    -As x approaches c from the left (values of x less than c), the function approaches a certain value. The script indicates a similar behavior when x approaches c from the right (values of x greater than c). The consistency in approaching the same value from both sides is key in defining the limit at c.

  • What is the symbol 'L' used for in the context of limits?

    -'L' represents the value that the function f(x) approaches as x gets closer to c. It symbolizes the limit of the function at that specific point.

  • Why does the script emphasize that the initial explanation of limits isn't mathematically rigorous?

    -The script highlights this to differentiate between an intuitive, conceptual understanding of limits and a more precise, mathematically rigorous definition. While the intuitive understanding is important for grasping the concept, a rigorous definition is necessary for formal proofs and deeper studies in calculus.

  • What is the importance of having a mathematically rigorous definition of limits?

    -A mathematically rigorous definition of limits is crucial for accurately proving theorems in calculus, ensuring precise calculations, and providing a solid foundation for more advanced topics in mathematics. It eliminates ambiguities and allows for a consistent application of limits in various mathematical problems.

  • How does approaching a limit differ when a function is defined at the point c versus when it is not?

    -When a function is defined at point c, the limit as x approaches c is typically the function's value at c. However, when it's not defined at c, the limit concerns the value the function approaches as x gets near c, which may be different from any actual function value at c.

  • Why is it said that understanding limits will 'take you pretty far' in calculus?

    -Understanding limits is fundamental in calculus because they form the basis for defining derivatives and integrals, which are core concepts in calculus. Grasping limits enables one to delve into these more complex topics and solve a wide range of mathematical problems.

  • What might be covered in the 'next few videos' following this script?

    -The next few videos are likely to introduce a more mathematically rigorous definition of limits, delve into the formal methodologies for calculating and proving limits, and possibly explore their applications in different calculus concepts like derivatives and continuity.

  • Why is the limit concept more interesting or relevant when the function is not defined at a point?

    -The concept of limits becomes more interesting and relevant when a function is not defined at a point because it allows us to understand the behavior of the function near that point. This can lead to insights about the function's continuity, asymptotic behavior, or how it behaves around singularities or points of discontinuity.

Outlines
00:00
📚 Introduction to the Concept of Limits

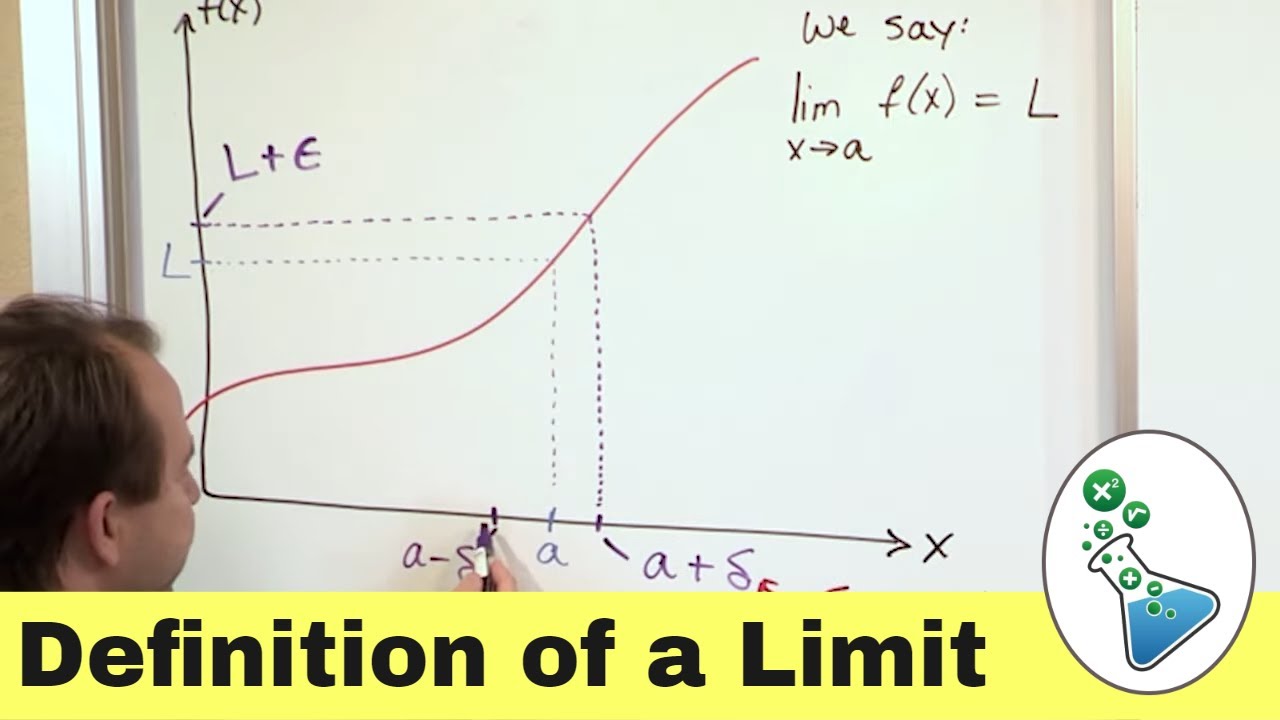
The paragraph introduces the fundamental concept of limits in mathematics, using a graphical approach to illustrate the idea. It begins by setting up a coordinate system with defined x and y axes, focusing on the first quadrant. The speaker then draws a generic function, y = f(x), and discusses the concept of a limit by examining what happens as x approaches a specific value 'c', where the function is not defined. The explanation delves into how the value of the function (f of x) changes as x gets closer to 'c' from both the left and right sides. It highlights the observation that the function seems to approach a specific value, denoted as 'L', as x gets arbitrarily close to 'c'. This value 'L' is referred to as the limit of the function as x approaches 'c'. The paragraph emphasizes that while this is a useful conceptual understanding, it is not mathematically rigorous, and future discussions will introduce a more formal definition of limits that allows for proof and deeper analysis.

Mindmap
Keywords
💡limit
In the context of the video, a 'limit' refers to the value that a function approaches as the input (x) gets arbitrarily close to a certain point (c). It is a fundamental concept in calculus and mathematical analysis, used to describe the behavior of functions, particularly at points where the function may not be defined. The video illustrates this by showing how the function f(x) seems to get closer to a value as x approaches c from both sides.
💡intuition
The term 'intuition' in the video refers to the initial, non-rigorous understanding of a concept, which in this case is the concept of limits. It is the informal grasp of how something works or behaves, which serves as a foundation for later, more formal and mathematically rigorous definitions and proofs.
💡axes
The 'axes' mentioned in the script are the coordinate lines on a graph that help plot functions and analyze their behavior. The x-axis represents the horizontal direction, while the y-axis is vertical. They are essential tools in visualizing and understanding the relationship between variables in a function.
💡function
A 'function' is a mathematical relation that pairs each element from one set (the domain) to exactly one element of another set (the range). In the video, the function is represented by f(x), and the discussion revolves around the behavior of this function as x approaches a certain value, c.
💡approaches
In the context of the video, 'approaches' is used to describe the process of getting arbitrarily close to a value without necessarily reaching it. This term is crucial in understanding limits, as it conveys the idea that the input (x) can get as near as desired to the point c, but does not have to actually equal c.
💡value
A 'value' in the script refers to the specific output that the function f(x) is getting closer to as x approaches the point c. This value, denoted as L, is the limit of the function at c and is the central focus of the discussion on limits.
💡defined
The term 'defined' in the video relates to whether a function has a specified output for a given input. The script mentions a scenario where the function is not defined at a point c, which means there is no output value associated with the input c in the function f(x).
💡first quadrant
The 'first quadrant' is the region of the coordinate plane where both x and y have positive values. The video mentions focusing on this area, although it's not strictly necessary for understanding limits, it provides a simple context for visualizing the function and its behavior.
💡mathematically-rigorous
A 'mathematically-rigorous' definition or explanation is one that is precise, formal, and based on a solid foundation of mathematical principles. The video script indicates that the initial explanation of limits is conceptual and not rigorous, and promises a more rigorous definition in subsequent videos.
💡denote
In the context of the video, 'denote' refers to the act of representing or symbolizing a concept or value with a specific symbol or expression. The limit of a function as x approaches c is denoted by the symbol L, and the mathematical notation for this limit is written as 'the limit of f(x) as x approaches c is equal to L'.
💡prove
The term 'prove' in the video refers to the process of demonstrating the truth or validity of a mathematical statement through logical reasoning and the use of established theorems and axioms. The script mentions that future videos will introduce a rigorous definition of limits that will allow for proving statements about them, such as the equality of a limit to a specific value L.
Highlights

Intuition of what a limit is in mathematics is being reviewed.

The concept is being explained with the help of a graphical representation on axes.

A function y = f(x) is introduced with an undefined point.

The limit is described as the value f(x) approaches when x gets closer to a certain point c.

The function's behavior on both sides of point c is discussed to understand the limit.

The value L is introduced as the limit of the function as x approaches c.

The notation for representing the limit of a function is explained.

The conceptual understanding of limits is acknowledged as a solid foundation for further study.

The current explanation is noted as not being mathematically rigorous.

The importance of a mathematically rigorous definition of limits is mentioned for future discussions.

The process of visually tracking the function's value as it approaches the point of discontinuity is described.

The concept of limits is highlighted as crucial for deeper understanding in calculus.

The transcript outlines the necessity of a formal definition to prove the existence of a limit.

The role of limits in analyzing functions and their behavior is emphasized.

The session sets the stage for a more detailed exploration of limits in upcoming videos.

The importance of understanding the approach of x to c from both directions is discussed.

The session aims to transition from conceptual understanding to mathematical proof in the study of limits.

Transcripts

Browse More Related Video

Formal definition of limits Part 2: building the idea | AP Calculus AB | Khan Academy

Introduction to limits 2 | Limits | Precalculus | Khan Academy

Limits in Calculus: Definition & Meaning. What is a Limit?

Introduction to Limits (NancyPi)

1.2 - Algebraic Limits and Continuity

Squeeze Theorem

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusMathematicsConceptual UnderstandingLimitsFunction BehaviorEducational ContentMath TheoryLearning MathAsymptotic AnalysisMath Intuition