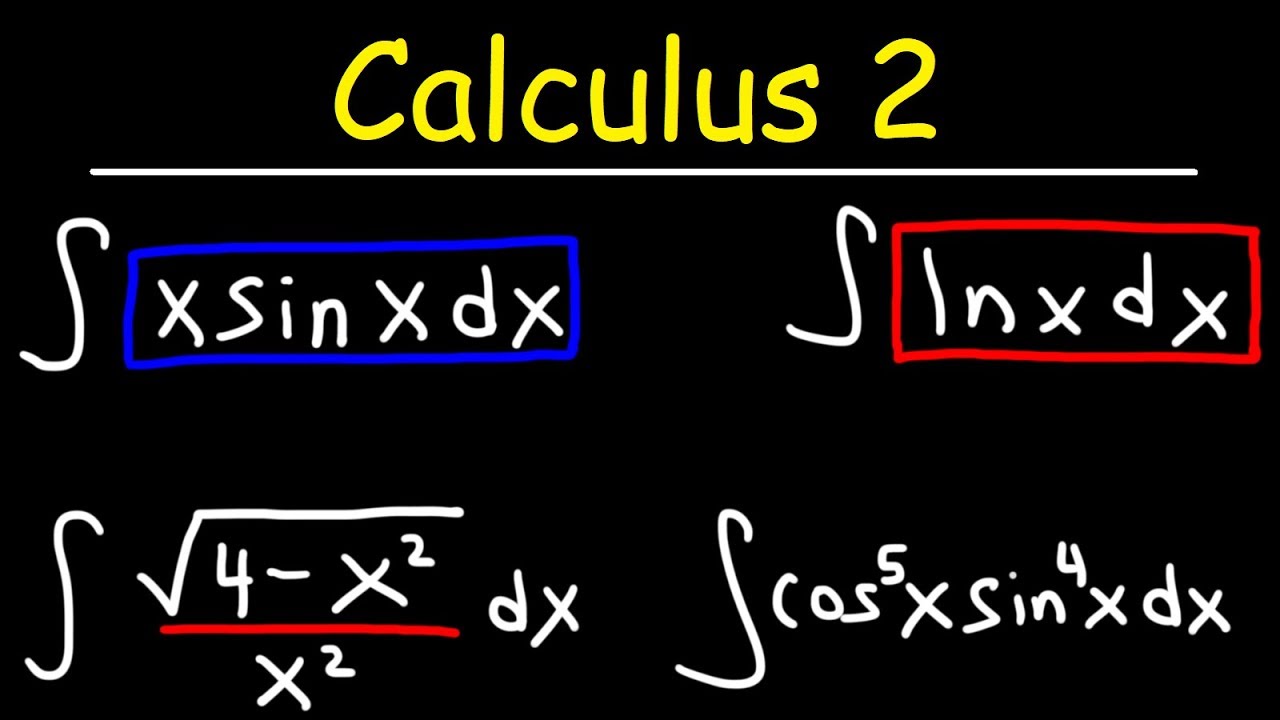
Advanced Strategy for Integration in Calculus
TLDRThis is a calculus video lecture discussing techniques for evaluating indefinite integrals. It emphasizes that integration often requires developing a strategy based on available techniques like u-substitution, integration by parts, and trig substitution. Broadly, the steps are to first seek basic integrands or simplification strategies, then try u-substitution for composite functions, and finally use integration by parts for products of functions without obvious substitutions. The video walks through examples demonstrating when to employ certain methods and that sometimes multiple techniques must be combined. It stresses that with enough practice evaluating diverse integrals, you can master this creative problem solving process.
Takeaways
- 😀 Integration requires strategy and practice, unlike differentiation which follows set rules
- 👨🏫 Always check substitution first when integrating, then parts if needed
- 📚 Memorize common integration formulas and techniques
- 🔍 Multiple strategies may work, don't get stuck on just one
- 🤔 Evaluate and simplify terms first before integrating
- ✏️ Substitute to eliminate composite functions and enable other techniques
- 🧮 Sometimes substitute more than once to reach an integrable form
- ⚖️ If stuck, try manipulating the integrand to enable substitution
- ⚡ Be clever - use trig identities, foil denominators, etc. if needed
- 💪 Practice frequently with unfamiliar integrals to improve strategy
Q & A
What makes integration more difficult than differentiation?
-Integration does not have a rigid algorithm to follow like differentiation does. We sometimes have to look at an integrand and come up with a strategy or technique to integrate it.
What are some integration techniques we can use?
-Some integration techniques are: substitution, integration by parts, trigonometric substitution, simplifying the integrand, and manipulating the integrand.
When using substitution, what should we check first before trying other techniques?
-When using substitution, we should first check if one of the functions in the integrand is the derivative of the other. If so, substitution will likely be the easiest technique.
What should we do if substitution does not work initially?
-If substitution does not work right away, try solving for dx instead of the derivative. Or try substituting something else. If substitution still does not work, try integration by parts or a different technique.
When does integration by parts work best?
-Integration by parts works best when the integrand is a product of functions where one function is a power of x or polynomial and the other is a transcendental function like a trig, exponential or logarithmic function.
How can we manipulate a complicated integrand?
-We can manipulate a complicated integrand by simplifying a sum or difference in the denominator using conjugates, splitting the fraction into simpler pieces, or using trig identities.
What should we do if none of the integration techniques seem to apply?
-If none of the standard integration techniques seem to apply, try to be creative and manipulate or substitute the integrand in a way that allows you to recognize one of the special integration formulas.
Why substitute twice sometimes?
-Sometimes we need to substitute twice when the first substitution results in something that requires another substitution or integration by parts to evaluate.
How can you check your integration work?
-You can check your integrated solution by taking its derivative and verifying the result matches the original integrand.
How can you get better at integration?
-You can get better at integration through practice over time, learning more techniques and formulas, and applying ingenuity to figure out strategies for unfamiliar integrals.
Outlines
🧑🏫 Overview of integration strategy and available techniques
Professor Dave provides an overview of various integration techniques like substitution, integration by parts, and trigonometric substitution. He notes that there is no rigid algorithm for integration and we sometimes need to analyze the integrand and determine the best strategy, which requires practice over time.
💡 Try substitution first before other techniques
Professor Dave works through an example integral using substitution, noting that this is often the easiest technique to try first. He emphasizes carefully checking if the integrand contains a function matching the derivative of another function, allowing direct substitution.
😕 Keep trying when substitution doesn't work initially
When substitution does not initially provide a straightforward solution, Professor Dave shows how further steps like integrating by parts may be required. He encourages perseverance using different tricks when needed.
🧠 General tips and concluding remarks
Professor Dave summarizes key learnings like trying substitution first and using integration by parts for products containing polynomials/trig functions. He notes that repeated substitution or integration by parts may be needed, requiring practice over time.
Mindmap
Keywords
💡differentiation
💡integration
💡substitution
💡integration by parts
💡trigonometric substitution
💡strategy
💡techniques
💡manipulate
💡conjugate
💡ingenuity
Highlights
Integration doesn't work like differentiation; there is no rigid algorithm to follow.
With integration, we sometimes have to look at an integrand and come up with a strategy, which could involve substitution, integration by parts, trig substitution, or something else.
Memorize common integration formulas and techniques like substitution, integration by parts, and trig substitution.
If the integrand matches a common formula, integration will be easy, but we may need to substitute to get it into that form.
Try direct substitution first before other techniques - it's usually easiest if it works.
If multiple strategies could work, try the simplest first rather than most complex.
For integration by parts, assign u and dv so du makes the integral easy to evaluate.
If substitution doesn't work directly, try solving for dx instead of du - terms may cancel out.
Substitution may lead to a form suitable for integration by parts instead of direct evaluation.
With practice, you'll become better at assessing integration strategies for unfamiliar expressions.
Check your integral by taking the derivative - if you get the original function, you integrated correctly.
Sometimes manipulate the integrand to enable substitution or parts instead of applying directly.
Trig identities can simplify denominators to assist integration.
Don't get lost in complex steps - box each stage to keep track of substitutions and technique changes.
Bring back substituted variables and distribute constants when finished with a technique.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: