Integration By Parts
TLDRProfessor Dave teaches integration by parts, a technique used to evaluate integrals of products of functions. He first derives the formula from the product rule of differentiation, allowing integration of products not integrable through substitution. He shows it works by choosing one function that simplifies when differentiated, making the new integral easier to solve. Through examples like integrating x sin(x) and ln(x), he demonstrates assigning functions as u and dv, then plugging them into the formula. He notes that while integration by parts can simplify difficult integrals, sometimes multiple applications are needed. Overall, it's a useful trick, complementing substitution, to evaluate challenging integrals.
Takeaways
- 😀 Integration by parts is a technique to evaluate integrals of products of functions
- 👍🏻 It is based on the product rule for differentiation but reversed
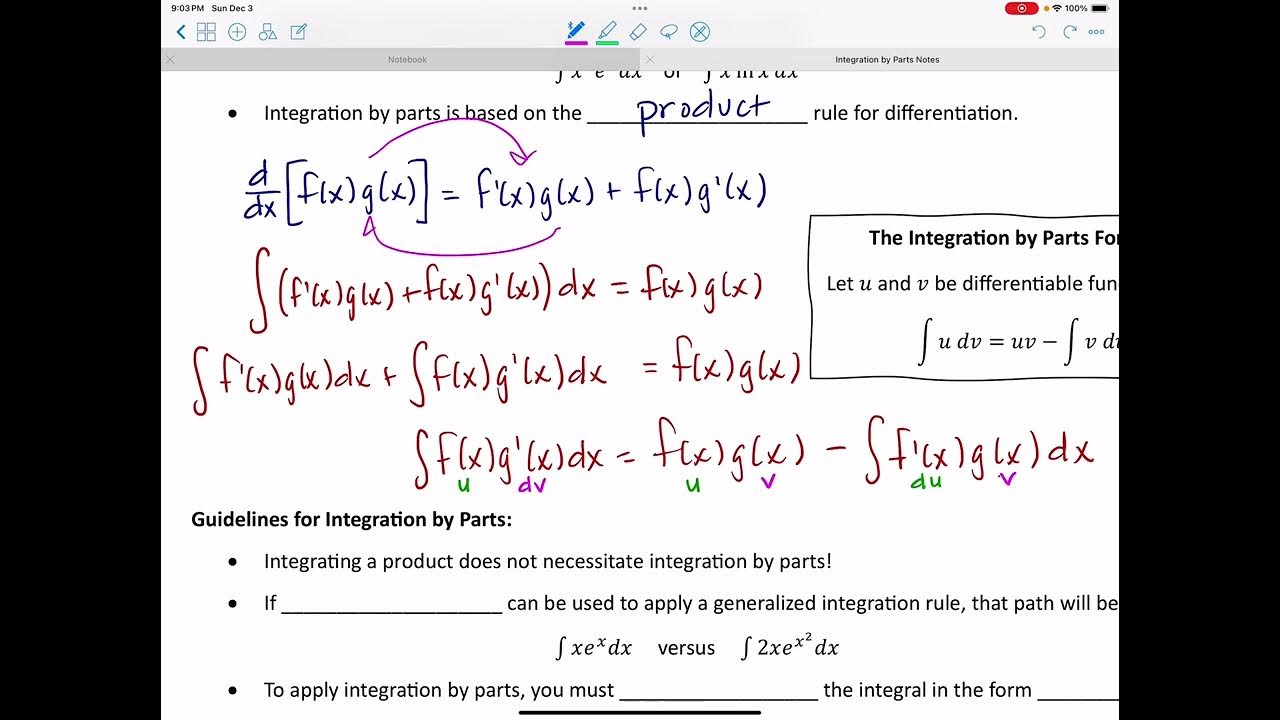
- 📝 It works by splitting the integral into two parts, one of which hopefully becomes simpler to evaluate after differentiation
- 💡 Choosing the right functions to differentiate is key - pick the one that simplifies the most upon differentiation
- 🧠 Memorize: ∫u dv = uv - ∫v du
- 🤔 It may require applying integration by parts twice if the new integral also needs it
- 😣 It won't always work - sometimes neither of the new integrals gets simpler
- 📈 Examples showed integrating x sinx, ln x, and (ln x)2 using this method
- 🔁 The substitution method works when one factor is the derivative of the other
- 🎓 Integration by parts works when the factors are unrelated but one simplifies upon differentiation
Q & A
What is the product rule for differentiation?
-The product rule states that the derivative of a product of two functions is equal to the first function times the derivative of the second function plus the derivative of the first function times the second function.
How is integration by parts related to the product rule for differentiation?
-Integration by parts is derived from the product rule. It allows us to integrate products of functions when one function becomes simpler upon differentiation.
What is the formula for integration by parts?
-The formula is: ∫u dv = uv - ∫v du, where u and v are functions and du and dv are their respective derivatives.
Why can integration by parts make an integral easier to solve?
-By properly selecting u and dv, the second integral ∫v du can become easier to evaluate than the original integral ∫u dv. This makes the technique useful.
In the first example integral, why was x chosen as f(x)?
-x was chosen as f(x) because its derivative is 1, which is very simple. This makes the second integral in the integration by parts formula easier to evaluate.
What are u, dv, du and v in the context of integration by parts?
-u and v are functions. dv and du are the derivatives of v and u respectively.
Why did the second example with ln(x) require applying integration by parts twice?
-The new integral generated after the first integration by parts was still a natural log function, which cannot be integrated normally. So integration by parts had to be applied again to evaluate it.
When is integration by parts useful to apply?
-Integration by parts is useful when the integrand is a product of functions that are not related by one being the derivative of the other. It works by generating a new integral that can sometimes be evaluated more easily.
What are the limitations of integration by parts?
-Integration by parts does not always make an integral easier to solve. Sometimes the new integral generated is just as difficult or even more difficult. In that case, the technique is not very useful.
How is integration by parts different from the substitution rule?
-The substitution rule works when one factor in the integrand is the derivative of the other factor. Integration by parts works when the factors are unrelated functions.
Outlines
😊 Learning Integration by Parts
This paragraph introduces integration by parts, explaining that it is a technique corresponding to the product rule of differentiation that allows integrating a product of two functions. The formula is derived and explained using u, v, du, and dv notation.
😃 Choosing u and dv for Integration by Parts
This paragraph provides guidance on choosing u and dv when applying integration by parts. The key is to choose u so its derivative du is as simple as possible, making the second integral easier to evaluate.
🤓 Repeating Integration by Parts
This paragraph explains that sometimes integration by parts results in another integral that must itself be integrated by parts. While tedious, this repeating of the technique may be necessary to reach the final solution.
Mindmap
Keywords
💡integrate
💡substitution rule
💡integration by parts
💡indefinite integrals
💡antiderivative
💡tricks
💡product rule
💡chain rule
💡calculus
💡evaluate
Highlights
Integration by parts works well when the two functions are not related at all, but one of them becomes much simpler when differentiated.
The key is to choose the function that becomes simpler when differentiated to be f(x), because f'(x) will remain in the integral and we want to evaluate it easily.
If applying integration by parts does not make the integral simpler, then the technique is not useful in that case.
Using u and dv notation makes applying integration by parts formula simpler by allowing us to plug functions back in according to our assignments.
Integration by parts can be applied to integrals that do not appear to be products, like the natural log example.
If applying integration by parts results in another integral we can't evaluate, we may need to apply the method again to the new integral.
The substitution rule works well when the integrand is a product of a function and its derivative.
Integration by parts produces a new integral, which could be simpler or more complex than the original.
Choosing the function that simplifies when differentiated as f(x) makes the second integral easier to evaluate.
The product rule for differentiation corresponds to the integration by parts technique.
Integration by parts allows integrating products of functions when substitution can't be applied.
Integration by parts works by turning one integral into another, hopefully simpler one.
If the new integral from integration by parts must also be integrated by parts, we just repeat the process.
Integration by parts, like substitution, gives us another technique to evaluate tricky integrals.
The key to applying integration by parts is assigning u, dv, du and v correctly based on which function simplifies.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: