Formal definition of limits Part 2: building the idea | AP Calculus AB | Khan Academy

Khan Academy

11 Jan 201306:05

EducationalLearning

32 Likes 10 Comments

TLDRThe video script delves into the mathematical concept of limits, specifically focusing on the limit of a function f(x) as x approaches a value c, denoted as L. It explains the intuitive idea that f(x) can be made arbitrarily close to L by choosing x sufficiently close to c, despite potential undefined points or kinks in the function. The script sets the stage for the epsilon-delta definition of limits, emphasizing the necessity to satisfy this condition for any given range around L to prove the limit's validity.

Takeaways

📚 The concept of a limit in mathematics is defined as the value that a function f(x) approaches as x gets arbitrarily close to a certain point c.
🎯 The statement 'the limit of f(x) as x approaches c is L' can be interpreted as being able to get f(x) as close to L as desired, by making x sufficiently close to c.
🔍 To illustrate this, one can think of it as a game where a skeptic asks for f(x) to be within a certain range of L, and the prover must find a corresponding range for x around c that ensures f(x) meets the skeptic's requirement.
📈 The video script uses a graphical representation to demonstrate the idea of a limit, showing how the function's values huddle around the limit value L as x approaches c.
🤔 The process of convincing a skeptic about a limit involves defining ranges around both the point c and the limit value L, and showing that within the specified range around c, the function's values stay within the desired range around L.
🔧 The concept is not limited to functions that are defined at c; even if the function has a 'hole' or is undefined at c, the limit can still be discussed.
🌟 The script introduces the idea of 'delta-epsilon' definitions of limits, which is a formal way to express the concept using precise mathematical language.
📊 The graphical representation helps to visualize the limit and understand the relationship between the proximity of x to c and the proximity of f(x) to L.
🔎 The process of proving a limit involves showing that for any given range around L (no matter how small), there exists a corresponding range around c such that all f(x) values within that range are within the desired proximity to L.
🚀 The video script sets the stage for a deeper exploration of the epsilon-delta definition in subsequent content, which is a cornerstone in the rigorous study of limits in calculus.

Q & A

What does it mean for the limit of a function f(x) to be equal to L as x approaches c?
-The limit of a function f(x) as x approaches c being equal to L means that as x gets arbitrarily close to c, the function values f(x) get as close as desired to L. This is a formal way of expressing the intuitive idea that the output of the function approaches the value L when the input approaches the value c.
How can you express the idea of getting f(x) as close as desired to L?
-You can express the idea of getting f(x) as close as desired to L by stating that for any given positive number (however small), it is possible to find a corresponding value of x that is sufficiently close to c such that f(x) will be within that small range around L.
What is the significance of the phrase 'as close as you want' in the context of limits?
-The phrase 'as close as you want' refers to the precision or proximity that the function values f(x) can reach to the limit L. It emphasizes that no matter how stringent the desired proximity to L is, if the limit exists, it is always possible to find an x sufficiently close to c to achieve that level of proximity.
What is the role of the value epsilon (ε) in the definition of a limit?
-Epsilon (ε) is a positive number that represents the desired proximity to the limit L. It is used to specify how close the function values f(x) must be to L in order to say that the limit of f(x) as x approaches c is L.
What is the significance of the value c in the context of limits?
-The value c is the point to which the input variable x is approaching in the definition of a limit. It is the 'limiting' value that defines the context in which the limit of the function is being considered.
What does it mean for a function to be undefined at a point c?
-A function being undefined at a point c means that there is no specified value for f(x) when x equals c. The function may have a 'hole' or a break at that point, and it does not have a value that can be computed or evaluated.
How does the concept of 'sufficiently close to c' relate to the value delta (δ)?
-The value delta (δ) represents the range of values around c that x can take such that the function values f(x) will be within the specified proximity (defined by epsilon) to the limit L. If x is within this delta range, then f(x) is guaranteed to be as close to L as desired.
What is the epsilon-delta definition of limits?
-The epsilon-delta definition of limits is a formal way to state that the limit of a function f(x) is L as x approaches c. It says that for any positive epsilon (however small), there exists a corresponding positive delta such that if 0 < |x - c| < δ, then |f(x) - L| < ε. This means that you can make f(x) as close to L as desired by choosing x sufficiently close to c.
How does the process of finding a range around c relate to proving a limit?
-The process of finding a range around c is part of the proof that the limit of a function is a certain value L. By demonstrating that for any given small range around L (defined by epsilon), there exists a corresponding small range around c (defined by delta) that ensures f(x) falls within the epsilon range, one can prove that the limit is indeed L as x approaches c.
What is the relationship between the concepts of epsilon and delta in the epsilon-delta definition of limits?
-In the epsilon-delta definition of limits, epsilon and delta are related in the sense that delta is the range of values for x that ensures f(x) falls within the range defined by epsilon around the limit L. The smaller the epsilon, the smaller the corresponding delta must be to ensure the proximity of f(x) to L.
How does the concept of limits relate to the behavior of a function near a certain point?
-The concept of limits describes the behavior of a function near a certain point c. It provides a way to analyze and understand how the function values approach a specific value L as the input x gets arbitrarily close to c, even if the function is not defined at c or has some irregularities at that point.

Outlines

00:00

📚 Introduction to the Concept of Limits

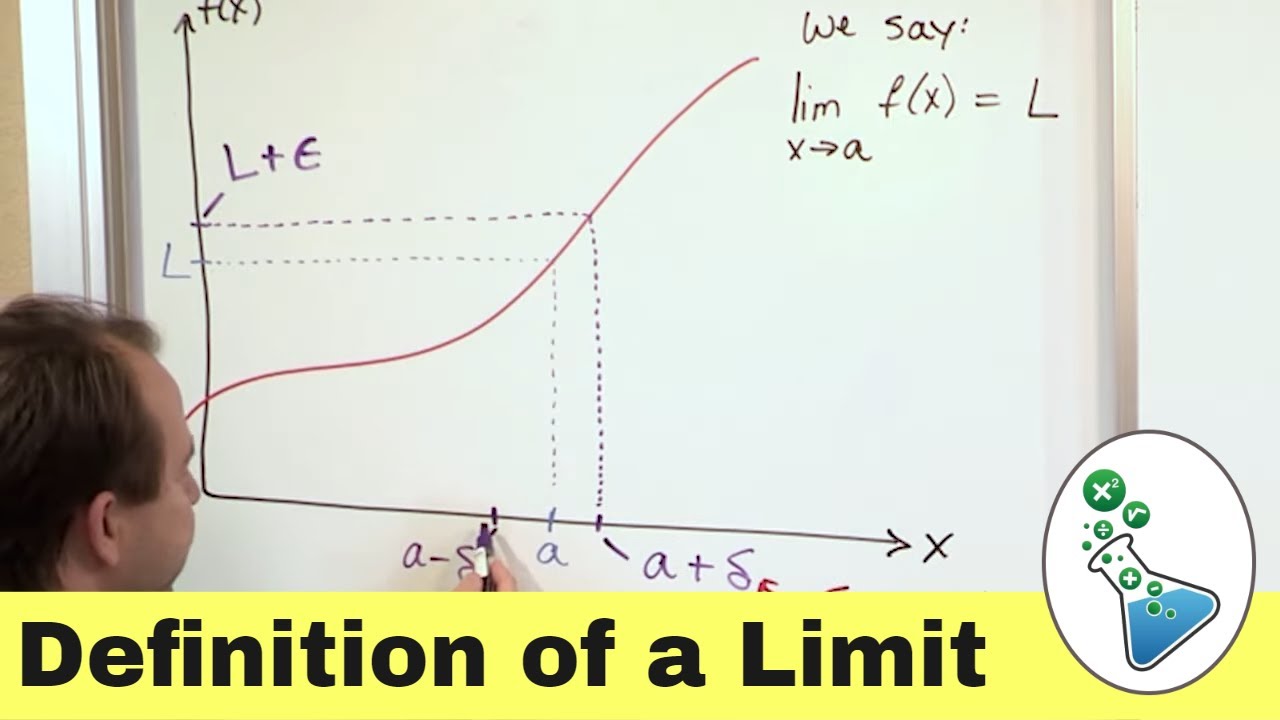
The paragraph introduces the mathematical concept of limits, specifically focusing on the limit of a function f(x) as x approaches a value c, denoted as L. It discusses the idea that f(x) can be made arbitrarily close to L by choosing x sufficiently close to c. The explanation involves a visual representation, with a hypothetical function graphed around the points c and L. The paragraph emphasizes the need for a rigorous definition, acknowledging the potential looseness in the intuitive understanding of 'closeness'. It sets the stage for a deeper exploration of limits and their mathematical treatment.

05:00

🔍 Refining the Concept through a 'Game' of Precision

This paragraph delves deeper into the operational definition of limits by framing it as a 'game'. It describes a scenario where a skeptic challenges the claim that the limit of f(x) as x approaches c is L. The paragraph outlines a process where the skeptic specifies a range around L (say, L ± 0.5) and the proponent of the limit must identify a corresponding range around c such that any x within this range will yield an f(x) value within the skeptic's specified range. The process is then repeated with increasingly tighter ranges (e.g., L ± 0.05), demonstrating that for any desired precision, there exists a corresponding level of x proximity to c that ensures the precision is met. This exercise illustrates the foundational principle leading to the formal epsilon-delta definition of limits.

Mindmap

Keywords

💡limit

In the context of the video, 'limit' refers to a mathematical concept that describes the behavior of a function when its input, or 'x', approaches a certain value, denoted as 'c'. The limit is the value that the function 'f of x' approaches, and it is used to understand the long-term behavior of functions. For example, the video discusses the limit of f of x as x approaches c is equal to L, which means as x gets arbitrarily close to c, the function values get arbitrarily close to L.

💡epsilon (L plus 0.5 and L minus 0.5)

Epsilon, often denoted by the Greek letter ε, is a term used in mathematics to describe a very small positive number. In the video, the concept of epsilon is introduced to illustrate how close the function values can get to the limit L. The video uses the example of L plus 0.5 and L minus 0.5 to show that if the limit as x approaches c is L, then the function values should be within 0.5 units of L whenever x is sufficiently close to c.

💡delta (c plus 0.25 and c minus 0.25)

Delta, often denoted by the Greek letter δ, is a term used in the definition of a limit to describe a range or tolerance level for the input variable x. In the video, the concept of delta is used to establish a relationship between how close x must be to c and how close f(x) will be to L. For instance, the video explains that if x is within 0.25 of c, then f(x) will fall within the desired range around L.

💡function

A function in mathematics is a relation that assigns a single output value to each input value. In the video, the function is denoted as 'f of x', and the discussion revolves around understanding the behavior of this function as the input x approaches a certain value 'c'. The function's graph is used as a visual tool to illustrate the concept of limits.

💡approaches

The term 'approaches' in the context of the video refers to the process of getting arbitrarily close to a certain value. When discussing limits, it means that as the input x gets closer and closer to the value c, the function values get closer and closer to the limit L. This concept is crucial for understanding the if-then statement structure of limit definitions.

💡sufficiently close

In the video, 'sufficiently close' is used to describe the proximity of the input value x to the critical value c, and the function values to the limit L. It implies that there is a certain tolerance level within which the function values will align with the limit. For example, the video explains that if x is sufficiently close to c, then f(x) will be as close to L as desired.

💡y-axis

The y-axis is a vertical line in a two-dimensional coordinate system that serves as a reference for the vertical position of points. In the video, the y-axis is used to represent the range of output values for the function 'f of x'. The discussion of limits involves the behavior of these output values as x moves along the x-axis towards the value c.

💡graph

A graph is a visual representation of the relationship between two variables, where one variable's values are plotted on the x-axis and the other's on the y-axis. In the video, the graph of 'y is equal to f of x' is used to visually demonstrate the concept of limits, particularly how the function behaves as x approaches the value c.

💡undefined

In mathematics, a function is said to be 'undefined' at a point if it does not have a value at that point. In the video, the function is described as being undefined at x equals c, which is a common scenario when discussing limits. The video uses this to illustrate that even if a function has a 'hole' or is not defined at a certain point, limits can still be calculated.

💡kink

A 'kink' in the video refers to a sharp change or discontinuity in the graph of a function. This term is used metaphorically to describe a point where the function may not be smooth, even if it is still approachable for the purposes of calculating limits. The video uses the concept of a kink to show that the limit can still be defined even if the function has abrupt changes or is not continuous at the point of interest.

💡epsilon-delta definition

The epsilon-delta definition is a formal way to describe the concept of a limit in mathematics. It provides a precise criterion for when a limit exists. In the video, the discussion builds up to this definition by exploring the idea that for any given small range around L (epsilon), there must exist a corresponding small range around c (delta) such that all x values within that range will produce function values within the epsilon range of L. This will be the focus of the next video.

Highlights

The introduction of a mathematically rigorous definition for the limit of a function as it approaches a certain value.

The concept of getting the function value as close as desired to the limit L by making x sufficiently close to c.

The clarification that 'as close as you want' is a bit vague and requires a more precise mathematical description.

The illustration of the concept using a visual representation, with a focus on the areas around c and L.

The idea that even if a function is undefined at c, a limit can still be found.

The introduction of a hypothetical scenario where someone challenges the limit and asks to get the function value within 0.5 of L.

The explanation that if the limit is true, one should be able to find a range around c that ensures the function value falls within the desired range around L.

The demonstration of how to find a suitable range around c when given a specific range around L.

The example of choosing a range around c (c - 0.25 to c + 0.25) to ensure the function value stays within the desired range around L.

The challenge of proving the limit for any given range around L, not just for one specific range.

The concept that to prove the limit, one must be able to find a suitable range around c for any range given around L.

The mention of the next step, which is generalizing the concept to the famous epsilon-delta definition of limits.

The use of a visual aid to clarify the abstract concept of limits in mathematics.

The application of the concept to a hypothetical function with a kink at the point of interest.

The emphasis on the importance of being able to reproduce the result for any desired range, not just one.

The explanation that the limit of a function is true if it can be made arbitrarily close to the limit value for values of the function's argument that are sufficiently close to the point of interest.

The introduction of the concept of 'certain range' which is a crucial part of the definition of a limit.

The use of a game-like scenario to illustrate the process of proving a limit, making the concept more relatable and understandable.

Transcripts

Browse More Related Video

Formal definition of limits Part 4: using the definition | AP Calculus AB | Khan Academy

Formal definition of limits Part 1: intuition review | AP Calculus AB | Khan Academy

A Tale of Three Functions | Intro to Limits Part II

Epsilon-delta definition of limits

Limits in Calculus: Definition & Meaning. What is a Limit?

Squeeze Theorem

Formal definition of limits Part 2: building the idea | AP Calculus AB | Khan Academy

Takeaways

Q & A

What does it mean for the limit of a function f(x) to be equal to L as x approaches c?

How can you express the idea of getting f(x) as close as desired to L?

What is the significance of the phrase 'as close as you want' in the context of limits?

What is the role of the value epsilon (ε) in the definition of a limit?

What is the significance of the value c in the context of limits?

What does it mean for a function to be undefined at a point c?

How does the concept of 'sufficiently close to c' relate to the value delta (δ)?

What is the epsilon-delta definition of limits?

How does the process of finding a range around c relate to proving a limit?

What is the relationship between the concepts of epsilon and delta in the epsilon-delta definition of limits?

How does the concept of limits relate to the behavior of a function near a certain point?