Limits in Calculus: Definition & Meaning. What is a Limit?
TLDRThis educational transcript delves into the concept of limits in calculus, explaining how a function's limit as it approaches a certain value is defined. The script begins with a layman's explanation, emphasizing the function's approach to a value without reaching it. It then transitions to a more mathematically rigorous approach, introducing symbols like Delta and Epsilon to illustrate the infinitesimal distances involved. The explanation uses visual aids to clarify how as 'x' gets infinitely close to 'a', the function 'f(x)' approaches the limit 'L'. The script also breaks down the formal definition of a limit found in textbooks, making it accessible by relating it to the graphical representation and the practical implications for calculating limits in various scenarios.
Takeaways
- 📚 A limit is a fundamental concept in calculus that describes the behavior of a function as its input approaches a certain value.
- 📉 In layman's terms, if a function is smooth and continuous, you can find the limit by simply substituting the value of 'a' into the function.
- 📈 Mathematically, a limit is defined more rigorously using the concepts of getting infinitely close to a value 'a' without actually reaching it.
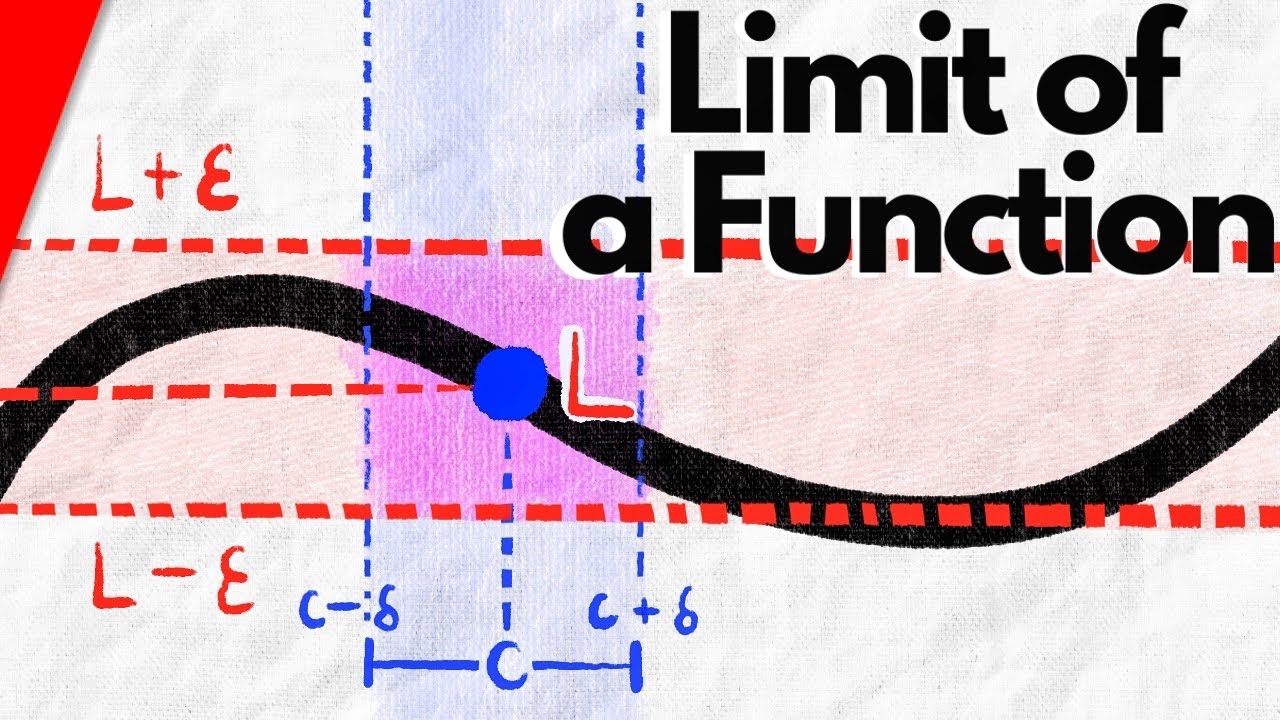
- 📊 The graphical representation of a limit involves drawing the function and marking the value 'a' on the x-axis and its corresponding function value 'L' on the y-axis.
- 🔍 The formal definition of a limit introduces the Greek letters Delta (a small positive number) and Epsilon (another small positive number) to quantify the closeness to 'a' and 'L', respectively.
- 📐 Delta represents the small distance around the point 'a' from which the function can be approached, either from the left (a - Delta) or the right (a + Delta).
- 📉 Epsilon is used to denote the closeness of the function's value to the limit 'L', where f(x) approaches L plus or minus a small Epsilon.
- 🔢 As Delta becomes infinitely small, Epsilon also becomes very small, indicating that f(x) gets infinitely close to the limit L.
- 📖 The textbook definition of a limit involves the formal mathematical framework that for every small Epsilon, there exists a corresponding Delta such that if the distance between x and a is less than Delta, then the distance between f(x) and L is less than Epsilon.
- 🤓 Understanding the formal definition of a limit is crucial for grasping more complex theorems and concepts in calculus, as it provides the foundational framework for limit analysis.
Q & A
What is the basic concept of a limit in mathematics?
-The basic concept of a limit is that as the variable x approaches a certain value a, the function f(x) approaches a value L. This is represented mathematically as lim (x→a) f(x) = L.
What does it mean for a function to be smooth and continuous?
-A smooth and continuous function is one that has no breaks, jumps, or discontinuities. It can be drawn without lifting the pen from the paper, indicating a constant flow of values.
How does the concept of a limit relate to the idea of a function getting infinitely close to a value?
-The concept of a limit is about the function values getting arbitrarily close to a certain value L as the input x approaches another value a, without actually reaching a.
What is the purpose of the mathematical definition of a limit?
-The mathematical definition of a limit provides a rigorous framework to prove the existence of limits for any function under certain conditions, which is essential for mathematical proofs and analysis.
What are 'Delta' and 'Epsilon' in the context of limits?
-In the context of limits, 'Delta' (δ) and 'Epsilon' (ε) are small, positive numbers used to define the closeness of x to a and f(x) to L, respectively. Delta is used to measure how close x can be to a without being equal to it, and Epsilon measures how close f(x) can be to the limit L.
How does the definition of a limit account for approaching a value from both sides?
-The definition of a limit uses the absolute value to ensure that it does not matter whether x approaches a from the left or the right. This is represented by the conditions |x - a| < Δ and |f(x) - L| < ε, which hold true for both directions of approach.
What is meant by 'for every positive Epsilon, there exists a Delta such that...' in the limit definition?
-This phrase means that for any small distance (Epsilon) you want the function values to be from the limit L, there exists a corresponding small distance (Delta) from the point a such that whenever x is within this Delta distance from a, the function values will be within Epsilon distance from L.
How does the graphical representation of a limit help in understanding the concept?
-The graphical representation visually shows how as x values get closer and closer to a, the corresponding f(x) values get closer to L. It provides a clear, intuitive understanding of the limit concept before delving into the formal mathematical definition.
What is the significance of the limit definition in calculus?
-The limit definition is fundamental to calculus as it underpins the concepts of continuity, derivatives, and integrals. It provides the necessary framework to analyze the behavior of functions and their rates of change at specific points.
Why are limits important in understanding the behavior of functions at points where they may not be defined?
-Limits are important because they allow us to discuss the behavior of functions near points where they may not be defined or where they have discontinuities. This is crucial for understanding the overall behavior and properties of functions in calculus.
Outlines
📚 Introduction to Limits in Functions
This paragraph introduces the concept of limits in calculus. It explains that for a continuous function, the limit as x approaches a certain value 'a' is simply substituting 'a' into the function to get the limit 'l'. The paragraph emphasizes the need for a rigorous mathematical approach, involving getting infinitely close to the value 'a' without actually reaching it. It sets the stage for a graphical representation and a more formal definition that will be introduced later in the script.
📈 Graphical Representation of Limits
The paragraph delves into a graphical explanation of limits. It describes drawing a smooth and continuous function and identifying a point 'a' where x approaches. The function's value at point 'a' is denoted as 'L'. The concept of approaching 'a' from both sides is introduced with the use of 'a plus Delta' and 'a minus Delta', where Delta is a small number representing proximity to 'a'. The paragraph also introduces 'Epsilon' to represent how close the function's value is to the limit 'L' as x gets infinitely close to 'a'. The graphical explanation aims to provide an intuitive understanding before moving on to the mathematical definition.
🔍 Deep Dive into the Mathematical Definition of Limits
This section breaks down the formal mathematical definition of a limit. It clarifies that a function 'f(x)' is defined on an interval containing a point 'a', possibly excluding 'a' itself. The limit 'L' of 'f(x)' as x approaches 'a' is established by the relationship between two small numbers, Delta and Epsilon. For every positive Epsilon, there exists a corresponding Delta such that if the difference between x and 'a' is less than Delta, then the difference between 'f(x)' and 'L' is less than Epsilon. This relationship is crucial as it mathematically formalizes the concept of getting infinitely close to a value without reaching it, which is the essence of a limit.
📘 Understanding the Limit Definition in Practice
The final paragraph reinforces the understanding of limits by relating the formal definition to practical applications. It emphasizes that the definition of a limit is a framework that allows for approaching a point 'a' from both the left and right, and ensuring that as x gets infinitely close to 'a', the function 'f(x)' gets infinitely close to the limit 'L'. The paragraph assures that grasping this concept is fundamental to understanding more complex theorems in calculus, setting the stage for further exploration of limit laws and rules in subsequent sections.
Mindmap
Keywords
💡Limit
💡Function
💡Continuous Function
💡Delta (Δ)
💡Epsilon (ε)
💡Graphical Representation
💡Approaching
💡Formal Definition
💡Proof
💡Theorem
Highlights
The limit of a function f(x) as x approaches a number a is defined as a value L that the function gets infinitely close to without actually reaching a.
For a smooth and continuous function, the limit as x approaches a can be found by simply substituting the value of a into the function.
The concept of a limit involves getting closer and closer to a value without ever actually reaching it, which is represented graphically by approaching a from both directions.
The formal definition of a limit introduces the idea of a proof in mathematics, requiring a rigorous framework to establish the existence of limits.
The Greek letter Delta (δ) is used to represent a small number that allows x to approach a without actually reaching it.
Epsilon (ε) is another small number representing how close the function's value is to the limit L as x approaches a.
The definition of a limit states that for every small positive Epsilon, there exists a corresponding Delta such that if x is within Delta of a, then f(x) is within Epsilon of L.
Graphically, as Delta becomes smaller, the function f(x) gets closer and closer to the limit L, illustrating the concept of approaching a value infinitely.
The absolute value in the definition of a limit accounts for approaching a from both the left and right sides.
The definition of a limit is a fundamental concept in calculus that establishes a rigorous mathematical framework for understanding how functions behave as they approach certain values.
The limit definition provided in textbooks can be difficult to understand without the visual aid of a graph, which helps to illustrate the concept more clearly.
Understanding the definition of a limit is crucial for grasping more complex theorems and concepts in calculus.
The limit definition involves the idea of infinitesimally small numbers, which is key to comprehending how functions approach limits.
The function f(x) can get as close as desired to the limit L by taking x closer and closer to a, without x ever equaling a.
The concept of limits is not just theoretical; it has practical applications in various areas of mathematics, including calculus.
Learning the formal definition of a limit helps in understanding the methodology used to find limits of functions in calculus.
The video aims to clarify the often confusing definition of a limit found in textbooks by providing a step-by-step graphical explanation.
Transcripts
Browse More Related Video

Epsilon-delta limit definition 1 | Limits | Differential Calculus | Khan Academy

Precise Definition of a Limit - Example 1 Linear Function

Formal definition of limits Part 1: intuition review | AP Calculus AB | Khan Academy

A Tale of Three Functions | Intro to Limits Part II

Math 11 - Sections 1.1-1.2
Epsilon-Delta Definition of Functional Limits | Real Analysis
5.0 / 5 (0 votes)
Thanks for rating: