Derivatives of Trigonometric Functions

Professor Dave Explains
11 Mar 201807:57
EducationalLearning
32 Likes 10 Comments

TLDRThis video explains how to take the derivatives of trigonometric functions like sine, cosine, tangent, and their inverses. It starts by visually showing how to find the derivative of sine from its graph, realizing it is cosine. Similarly for cosine, its derivative is negative sine. Using rules like the quotient rule, the derivatives of tangent, cotangent, secant, and cosecant are derived as well. Some examples are then shown applying these rules with the product and quotient rules. Finally, the derivatives of the six inverse trig functions are simply stated to memorize before the video concludes.

Takeaways
  • 😀 The derivatives of the 6 trig functions are: sin(x) = cos(x), cos(x) = -sin(x), tan(x) = sec^2(x), cot(x) = -csc^2(x), sec(x) = sec(x)tan(x), csc(x) = -csc(x)cot(x)
  • 😎 To find derivatives of trig functions, analyze slopes at key points and relate to basic trig function graphs
  • 📈 Use product rule, quotient rule and pythagorean identities to find derivatives of more complex trig expressions
  • 🎯 The derivatives of inverse trig functions have 1/sqrt(x^2 ± a^2) type formats
  • 🤓 Memorize derivatives of 6 trig functions & rules to tackle trig differentiation problems
  • 😊 Maxima/minima on sin(x)/cos(x) graphs = horizontal tangents = 0 derivative
  • 🔍 Increasing/decreasing sections on sin(x)/cos(x) match positive/negative derivative signs
  • 🧠 Derivative of tan(x) involves quotient rule, pythagorean identity and cosecant definition
  • 📒 Example problems: derive (x^2)*sin(x) and x/cos(x)
  • ❗Trig differentiation gets trickier, but mastering these basics will help tremendously
Q & A

  • What is the derivative of sine x equal to?

    -The derivative of sine x is equal to cosine x.

  • How can you find the derivative of other trigonometric functions like tangent, using the derivatives of sine and cosine?

    -You can use rules like the quotient rule. For example, to find the derivative of tangent x, you take cosine x times the derivative of sine x, minus sine x times the derivative of cosine x, over cosine squared x.

  • What is the derivative of secant x equal to?

    -The derivative of secant x is equal to secant x times tangent x.

  • What is the significance of maxima, minima, and inflection points when thinking about derivatives of trigonometric functions?

    -At maxima and minima, the tangent line is horizontal so the slope and derivative is 0. Inflection points mark the transition from increasing to decreasing slope.

  • What is the derivative of x squared times sine x?

    -Using the product rule: (x squared)(cosine x) + (2x)(sine x)

  • What is the derivative of the quotient x over cosine x?

    -Using the quotient rule: (cosine x) + (x sine x) over (cosine x) squared

  • What are some key derivatives to memorize?

    -Memorize the derivatives of the 6 basic trig functions: sine x, cosine x, tangent x, cotangent x, secant x, and cosecant x. Also know the derivatives of their inverses.

  • How can the pythagorean identity help in simplifying some trig derivatives?

    -The pythagorean identity states that sine squared x + cosine squared x = 1. This can help simplify expressions like the derivative of tangent x.

  • What rules are important for differentiating trig functions?

    -Know how to apply rules like the product rule, quotient rule, and chain rule when differentiating expressions with trig functions.

  • What other types of functions have trickier derivatives?

    -Exponential and logarithmic functions can have trickier derivatives. Also derivatives get more complex for partial derivatives and higher order derivatives.

Outlines
00:00
😀 Deriving Trig Function Derivatives

This paragraph introduces the topic of derivatives of trigonometric functions. It mentions taking derivatives of polynomials and special functions, then specifies the trig functions: sine, cosine, tangent, cosecant, secant, and cotangent. It refers back to a previous trigonometry video for clarity if needed, then proceeds with the assumption of understanding what these trig functions mean in order to learn how to take their derivatives.

05:01
😃 Using Graphs to Find Trig Derivative Patterns

This paragraph uses the graphical interpretation of derivatives as slopes of tangent lines to reason about and sketch the derivatives of sine and cosine. It identifies points where the derivatives are 0, ±1 and pieces together the full graphs, recognizing cosine as the derivative of sine, and -sine as the derivative of cosine. It then uses rules like the quotient rule to find the derivatives of the other trig functions like tangent, cotangent, cosecant, and secant.

Mindmap
Keywords
💡derivatives
The derivatives refer to a mathematical concept where the rate of change of a function is calculated. In the context of this video, derivatives help determine the slope or tangent of trigonometric functions like sine, cosine, etc. at any point. Understanding derivatives is key as Professor Dave aims to teach the audience how to calculate derivatives of trig functions.
💡trigonometric functions
Trigonometric functions like sine, cosine, tangent, secant, cosecant and cotangent are the main focus of this video. Professor Dave talks about their graphs and how to visualize and calculate the derivatives of these periodic functions. Knowing trig functions is core to following the logic shown in the video.
💡slope
The slope refers to the steepness of a line or curve at any point. Slope is used interchangeably with derivative in the video when explaining tangents on the sine and cosine graphs. Analyzing the changing slope helps determine what the derivative graphs should look like.
💡product rule
The product rule is a technique used to find the derivative of a function multiplied by another function. Professor Dave uses the product rule to find the derivative of x^2(sine(x)) as an example problem, applying the rule correctly.
💡quotient rule
Similar to the product rule, the quotient rule is used to determine the derivative when one function is divided by another function. The video shows proper usage of the quotient rule in the example derivative of x/cosine(x).
💡pythagorean identity
The pythagorean identity states that sine(x)^2 + cosine(x)^2 = 1. This trigonometric concept is key in simplifying the derivative of tangent(x) to secant(x)^2 in one of the examples.
💡inverse trig functions
While not derived in the video, the derivatives of inverse trigonometric functions like arcsine(x), arccosine(x) etc. are listed to familiarize viewers, as they are useful for solving more advanced problems.
💡periodic function
Sine, cosine and other trig functions are periodic, meaning they repeat their values over regular intervals. Understanding this repeating nature of their graphs helps envision what their corresponding derivative graphs should look like.
💡maxima and minima
The highest and lowest points on periodic trig graphs are the maxima and minima. Professor Dave uses the fact that slope equals 0 at these points to determine places where the trig function derivatives must also equal 0.
💡memorize
Memorizing the derivatives of basic trig functions is emphasized so that more complex derivatives can be tackled via the product, quotient and other rules. Avoiding the need to re-derive simplifies the problem-solving process.
Highlights

The derivative of sine x is cosine x.

The derivative of cosine x is negative sine x.

The derivative of tangent x is secant squared x.

The derivative of cotangent x is negative cosecant squared x.

The derivative of cosecant x is negative cosecant cotangent x.

The derivative of secant x is secant tangent x.

Memorize the derivatives of the six trigonometric functions.

Use the product rule and quotient rule to differentiate functions with trigonometric terms.

The derivatives of the six inverse trigonometric functions are in the form of one over some expression involving x squared.

Example of differentiating (x^2)(sin x) using product rule.

Example of differentiating x/cos x using quotient rule.

The derivative turns sine x into cosine x.

The derivative turns cosine x into negative sine x.

Use rules already known to find derivatives of other trig functions from sine and cosine.

Check comprehension on finding derivatives of trig functions.

Transcripts

Browse More Related Video

Evaluating Integrals With Trigonometric Functions

Derivatives of Trigonometric Functions - Product Rule Quotient & Chain Rule - Calculus Tutorial

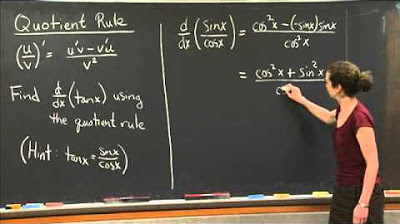
Quotient Rule | MIT 18.01SC Single Variable Calculus, Fall 2010

Derivatives of Trig Functions - Calculus | MCV4U

Derivatives of Trig Functions (Sin, Cos, Tan) in Calculus - [1-4]

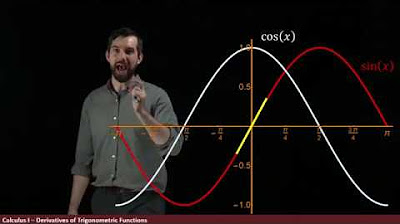
The derivative of Trigonometric Functions

Rate This

5.0 / 5 (0 votes)

Thanks for rating: