Introduction to Limits (NancyPi)
TLDRIn this informative video, Nancy introduces the concept of limits in mathematics, explaining how they predict the value that a function approaches as the input (x-value) gets closer to a certain point. She clarifies that limits are always y-values and emphasizes the importance of checking both sides of a point to find a limit. Nancy illustrates this with examples, including cases where the function has a hole or is not defined at a specific x-value. She also discusses one-sided limits and how they differ from overall limits. The video touches on limits at infinity, showing how to determine the y-values as x approaches very large positive or negative numbers. Nancy's engaging explanation demystifies the concept of limits, making it accessible to viewers and encouraging further exploration of the topic.
Takeaways
- 📚 A limit is a prediction of the y-value a function approaches as x gets closer to a certain point.
- 🔍 The notation for a limit is read as 'the limit, as x approaches a certain value, of f(x) equals some number'.
- 📈 To find a limit, you must check the behavior of the function from both the left and the right of the point in question.
- 🔄 The concept of limits is important for understanding the behavior of functions at points where the function may not be defined.
- ➡️ For a limit to exist, the left-sided limit and the right-sided limit must be equal.
- 🔴 If the left-sided and right-sided limits are not equal, the overall limit does not exist (DNE).
- 🔵 The limit answer is always a y-value, even if the function is not defined at the exact point x is approaching.
- 🔑 The equal sign in limit notation does not imply the function equals that value at the point, but rather what the function is approaching.
- 🌐 For limits at infinity, you evaluate the behavior of the function as x becomes very large in the positive or negative direction.
- 📉 When a function has a hole or is not defined at a certain point, the limit can still be determined by the behavior of the function around that point.
- 📈📉 In cases where the function is continuous and equals the limit value at the point, the limit concept still focuses on the approach from both sides, not the value at the point itself.
Q & A
What is the basic concept of a limit in mathematics?
-A limit is a prediction of what the y-value appears to be as the input x approaches a certain point, even if the function is not defined at that exact point.
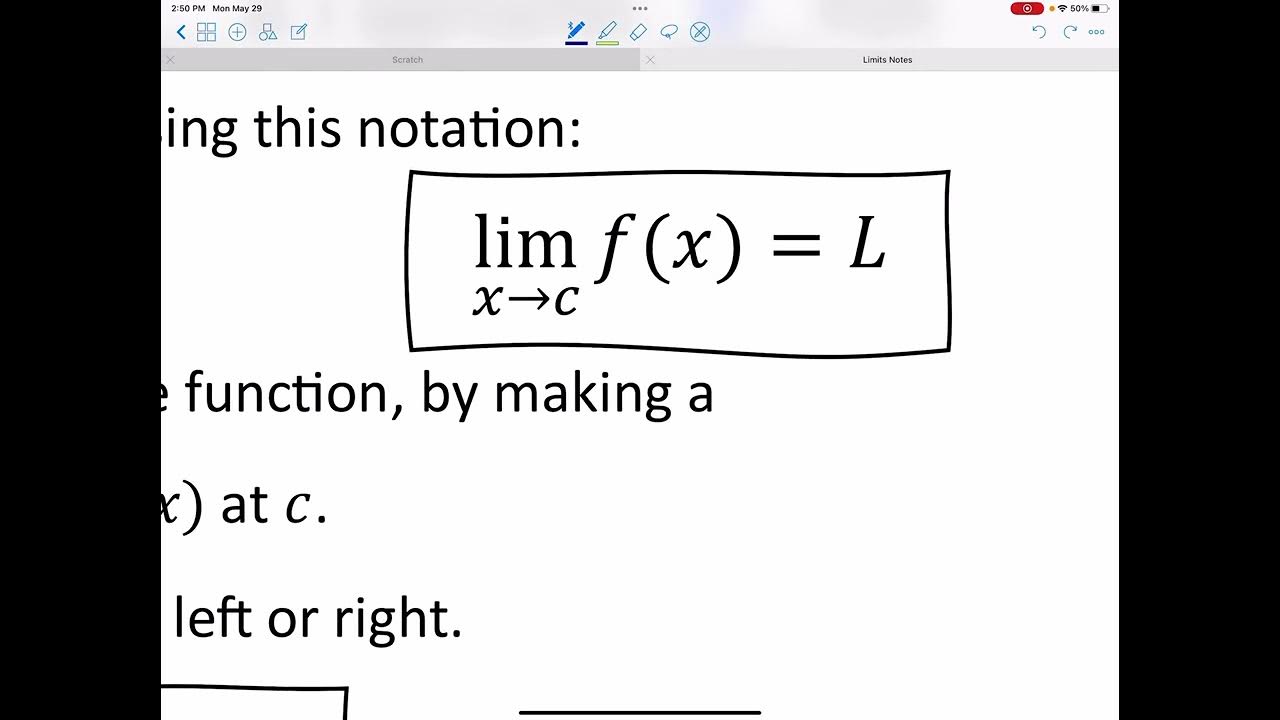
How is the limit notation read when you see 'lim (x -> a) f(x) = L'?
-It is read as 'the limit as x approaches a, of f(x), equals L', which means y is getting really close to L as x gets really close to a.
Why do we need to check both sides of a point when finding a limit?
-We check both sides because the limit implies the behavior of the function around a point, not necessarily at the point itself. This ensures we capture the function's trend as it approaches from both directions.
What does it mean when the left-sided limit and the right-sided limit of a function at a point are not the same?
-If the left-sided limit and the right-sided limit are not the same, it indicates that the overall limit does not exist at that point because the function does not approach a single value as x approaches from both sides.
How can a function have a defined point at a certain x-value but still have an undefined limit at that point?
-A function can have a defined point at an x-value, but the limit at that point may be undefined if the behavior of the function as it approaches that x-value from both sides does not lead to a consistent y-value.
What is the significance of the equal sign in limit notation?
-The equal sign in limit notation signifies that the function is approaching the value on the right as x approaches the value on the left, not that the function is exactly equal to that value at the point.
What is a one-sided limit and how is it represented?
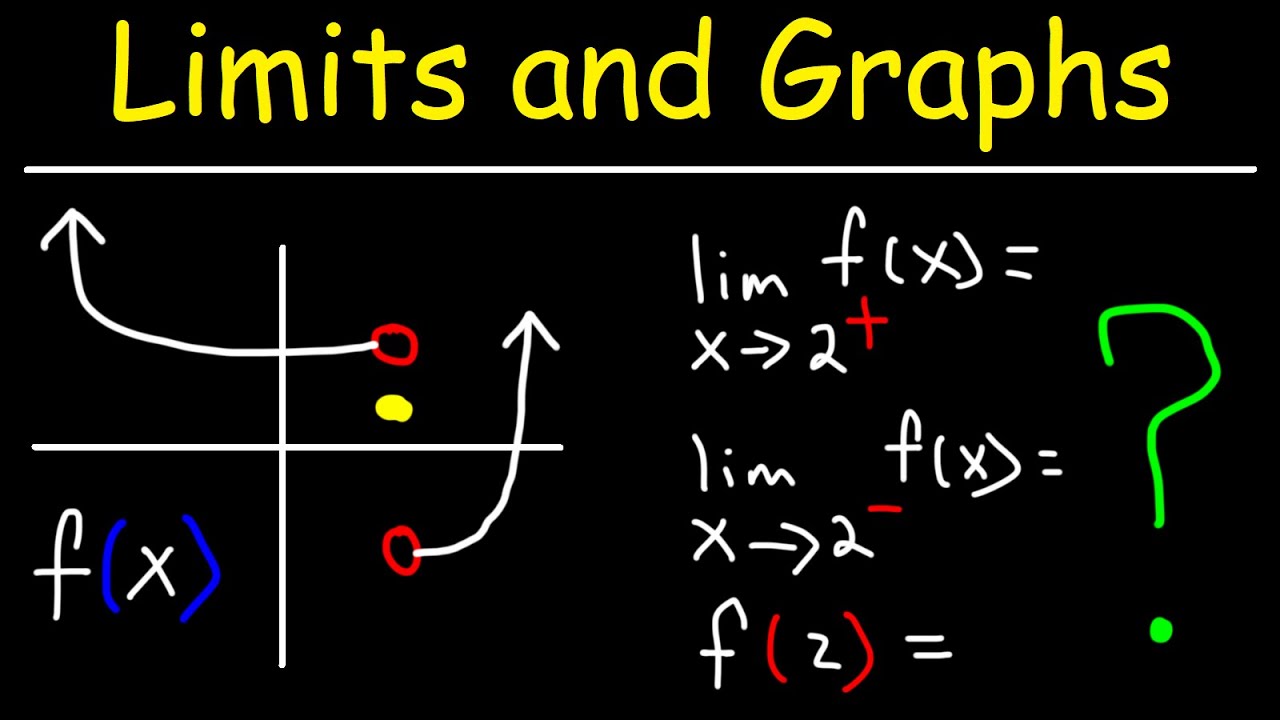
-A one-sided limit is the behavior of a function as x approaches a certain point from either the left or the right side. It is represented as 'lim (x -> a⁻) f(x)' for the left-sided limit and 'lim (x -> a⁺) f(x)' for the right-sided limit.
How do you find the limit as x approaches infinity?
-To find the limit as x approaches infinity, you trace the graph towards larger and larger positive x-values to see how the y-values behave, looking for any trends or asymptotic behavior.
What is the difference between a limit at a point and a limit at infinity?
-A limit at a point refers to the behavior of the function as x approaches a specific finite value, whereas a limit at infinity refers to the behavior of the function as x becomes very large, either in the positive or negative direction.
Why might the limit of a function at a certain point be different from the value of the function at that point?
-The limit of a function at a point is concerned with the trend or approach of the function's output as the input approaches that point, regardless of the actual value of the function at that point. A function may be undefined or discontinuous at a point but still have a limit there.
What does it mean if the limit from the left and the limit from the right are equal?
-If the limit from the left and the limit from the right are equal, it means that the function approaches the same y-value from both sides as x approaches the given point, suggesting a consistent trend and thus an existing overall limit.
How can you tell if a function has a hole or a gap at a certain point on its graph?
-A function has a hole or a gap at a certain point if it is not defined at that point or if there is a discontinuity. This can be observed on the graph as a break or missing section in the function's curve at that specific x-value.
Outlines
📈 Introduction to Limits and Their Notation
Nancy introduces the concept of limits in mathematics, explaining that a limit is a prediction of the y-value as x approaches a certain point. She clarifies that limits are represented by a specific notation, and their answers are always y-values. Nancy also emphasizes the importance of checking both sides of a point to find a limit, using the example of a graph with a hole at x=1. She illustrates how to find the limit from the left and right, and how they should be the same for the limit to exist.
🔍 Understanding Limits at a Point and at Infinity
The second paragraph delves into the nuances of limits at a specific point, such as x=1, and how the function's value at that point does not affect the limit. Nancy shows that even if a function has a hole or is later defined at that point, the limit is determined by the behavior of the function around, not at, the point. She also explores limits as x approaches infinity or negative infinity, using the concept of one-sided limits and explaining how to interpret the behavior of the function as it extends to these extremes.
🌌 Limits at Infinity and Their Practical Significance
In the final paragraph, Nancy addresses the concept of limits as x approaches infinity or negative infinity. She explains that while we can never actually reach these points, we can observe the behavior of the function as it trends towards them. Using graphical examples, she demonstrates how to determine the y-values that the function approaches at these extremes. Nancy concludes with encouragement and provides additional resources for further understanding of limits, inviting viewers to like, subscribe, and utilize her channel for study support.
Mindmap
Keywords
💡Limit
💡Notation
💡Graph
💡Approaching
💡One-Sided Limits
💡Continuous Function
💡Hole
💡Asymptote
💡Infinity
💡Indeterminate
💡Does Not Exist (DNE)
Highlights
Introduction to limits, explaining what a limit is and how to interpret its notation.
A limit is a prediction of the y-value as x approaches a certain point.
The limit notation is read as 'the limit, as x approaches a value, of f(x) equals some number'.
The concept of a function having a hole or gap, and how limits operate around such discontinuities.
Explanation of checking the limit from both sides of a point (left and right).
Demonstration of how to find the limit from the left and right sides of x = 1 on a graph.
Clarification that the limit is always a y-value, despite the function's behavior at the exact x-value.
The use of equal sign in limit notation to indicate what the function is approaching, not necessarily its exact value.
Illustration of how to handle a function defined at a point of interest, emphasizing that the limit is unaffected by the function's value at that point.
Procedure for finding the limit as x approaches a normal looking function, focusing on the behavior around the point, not the point itself.
Introduction to one-sided limits, showing how to calculate left-sided and right-sided limits separately.
Explanation of when the overall limit does not exist due to left-sided and right-sided limits being different.
Approach to finding limits as x goes to infinity or negative infinity, focusing on the behavior as x becomes very large.
Visualization of a function leveling off towards an asymptote as x approaches infinity.
Method for determining the limit as x approaches negative infinity by observing the y-value's trend.
Emphasis on the conceptual understanding of limits, rather than just computational techniques.
Encouragement to 'Like' the video and subscribe to the channel for further assistance with limits.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: