Hyperbolic Functions: Definitions, Identities, Derivatives, and Inverses

Professor Dave Explains
17 Aug 201807:33
EducationalLearning
32 Likes 10 Comments

TLDRThe script explains hyperbolic functions, relating them to the hyperbola graph instead of the circle like trig functions. Hyperbolic sine and cosine are defined in terms of exponential functions that form a hyperbola when graphed. Their graphs, derivatives, inverses and more are explored. Identities and derivatives mirror those of trig functions. Inverse hyperbolic functions involve logarithms and quadratic equations. The key ideas around definitions, graphs, identities and applications of these advanced mathematical functions are covered.

Takeaways
  • 😀 Hyperbolic functions are related to the hyperbola, similar to how trig functions relate to the unit circle.
  • 😇 Hyperbolic sine is expressed using exponential terms and forms a hyperbola when graphed.
  • 🧠 Hyperbolic cosine looks similar but crosses the x-axis at 1 instead of 0.
  • 👩‍🏫 Hyperbolic tangent is hyperbolic sine over hyperbolic cosine.
  • 📝 Hyperbolic functions have familiar identities and derivatives like trig functions.
  • 🔢 There are inverse hyperbolic functions that can be derived from the main ones.
  • ⚗️ The derivatives of inverse hyperbolic functions can be found using the chain rule.
  • 🎓 Hyperbolic functions have horizontal and vertical asymptotes.
  • ✏️ The domain and range vary for different hyperbolic functions.
  • 🏁 Understanding hyperbolic functions elucidates mysterious calculator buttons.
Q & A

  • What is the relationship between hyperbolic functions and the hyperbola?

    -Hyperbolic functions are related to the hyperbola in the same way that trigonometric functions are related to the unit circle. The graphs and equations of hyperbolic functions involve the hyperbola.

  • How is the graph of hyperbolic sine derived?

    -The graph of hyperbolic sine is derived by adding the graphs of (1/2)*e^x and -(1/2)*e^(-x). This sums to a function that crosses the origin and approaches the asymptotes of the hyperbola.

  • What is the domain and range of hyperbolic sine?

    -The domain and range of hyperbolic sine are both all real numbers.

  • What is the difference between the graphs of hyperbolic sine and hyperbolic cosine?

    -Hyperbolic cosine is derived by changing the minus sign between the two exponential terms to a plus sign. This reflects the lower portion of the hyperbola above the x-axis to create the graph of hyperbolic cosine.

  • What identities and properties do hyperbolic functions share with trigonometric functions?

    -Hyperbolic functions share identities and properties with trigonometric functions such as squared function formulas and sum formulas. They also have very similar derivatives with mostly just differences in signs.

  • How are inverse hyperbolic functions graphed?

    -Inverse hyperbolic functions are graphed by reflecting the regular hyperbolic functions across the line y=x.

  • How can you express inverse hyperbolic sine in terms of x?

    -Inverse hyperbolic sine can be expressed as ln(x + √(x^2+1)) by manipulating the exponential terms and applying the quadratic formula and natural logarithm.

  • What is the derivative of inverse hyperbolic tangent?

    -The derivative of inverse hyperbolic tangent is 1/(1-x^2).

  • What are some uses of hyperbolic functions?

    -Hyperbolic functions are used in areas like differential equations, special relativity, fluid dynamics, electromagnetic fields, image processing, and more.

  • What calculator buttons represent hyperbolic functions?

    -On some scientific calculators, hyperbolic functions are represented by the normal trig function symbols (sin, cos, tan) combined with an H, such as sinh, cosh, and tanh.

Outlines
00:00
😊 Introducing Hyperbolic Functions and Their Relationship to the Hyperbola

This paragraph introduces hyperbolic functions, explaining that they are similar to trigonometric functions but relate to the hyperbola rather than the unit circle. It shows how the graph of the hyperbola arises from the exponential definition of the hyperbolic sine function. It also defines the hyperbolic cosine and tangent functions similarly.

05:01
😃 Inverse Hyperbolic Functions and Derivatives

This paragraph derives expressions for the inverse hyperbolic functions using algebra. It takes the derivatives of the inverse hyperbolic sine function as an example, using the chain rule. It presents the derivatives of all three inverse hyperbolic functions.

Mindmap
Keywords
💡hyperbola
A hyperbola is one of the conic sections formed by the intersection of a cone with a plane. It has two separate curved branches that get closer to two asymptotes but never touch. Hyperbolas are related to hyperbolic functions in mathematics.
💡exponential
An exponential function has the general form y = a^x where a is a constant and x is the independent variable. Exponentials appear in the definitions of hyperbolic sine and cosine as e^x and e^-x which form the two branches of a hyperbola.
💡trigonometric
Trigonometric functions like sine, cosine and tangent are periodic functions defined on the unit circle. They contrast with hyperbolic functions which are defined on a hyperbola and capture exponential growth rather than cyclic behavior.
💡derivative
The derivative of a function captures its rate of change. The video shows how to take derivatives of hyperbolic functions and inverse hyperbolic functions using rules like the chain rule. The derivatives resemble those of trigonometric functions.
💡identity
An identity is an equality between two mathematical expressions that holds true for all values of the variables. Hyperbolic functions have identities relating squared and summed terms that parallel trigonometric identities.
💡asymptotic
An asymptotic line is one that a curve gets closer and closer to but never reaches. The video shows how the branches of the hyperbola representing hyperbolic tangent have horizontal asymptotes at -1 and 1.
💡inverse
The inverse function of a mathematical function flips it around the line y=x. Inverse hyperbolic functions can be derived from the originals and their graphs and derivatives are also covered.
💡domain
The domain of a function is the set of permissible inputs. Hyperbolic sine and tangent have domains of all real numbers while hyperbolic cosine requires restriction to positive values to make its inverse a valid function.
💡range
The range of a function tells what set of output values it can produce. The video contrasts the ranges of hyperbolic sine, cosine and tangent, showing how they differ.
💡conic section
A conic section is the intersection of a cone with a plane. The four conic sections are circles, ellipses, parabolas and hyperbolas. The video explores the connection between one such section - the hyperbola - and hyperbolic functions in math.
Highlights

Hyperbolic functions are related to the hyperbola, similar to how trigonometric functions relate to the unit circle.

Hyperbolic sine is defined using exponential functions, which form a hyperbola when graphed separately and added together.

Hyperbolic cosine is similar to hyperbolic sine, with a key difference in the sign of one of the exponential terms.

Hyperbolic tangent is defined as hyperbolic sine divided by hyperbolic cosine.

There are hyperbolic versions of cosecant, secant and cotangent as well.

Hyperbolic functions have similar identities and derivatives as trigonometric functions.

The derivatives of hyperbolic functions can be understood by examining the derivative of the exponential definition of hyperbolic sine.

There are inverse hyperbolic functions, obtained by reflecting the graphs of the regular hyperbolic functions.

The inverse hyperbolic functions can be expressed in terms of the natural log and exponential functions.

The derivatives of the inverse hyperbolic functions can be found using the chain rule.

Hyperbolic functions relate to the hyperbola in a way analogous to how trigonometric functions relate to the unit circle.

The exponential definition provides insight into derivatives and identities of hyperbolic functions.

Inverse hyperbolic functions extend the hyperbolic function family.

Derivatives of inverse hyperbolics can be found with the chain rule, similar to inverse trigonometric derivatives.

Understanding hyperbolic functions builds on and mirrors knowledge of trigonometric functions.

Transcripts

Browse More Related Video

Hyperbolic trig functions | MIT 18.01SC Single Variable Calculus, Fall 2010

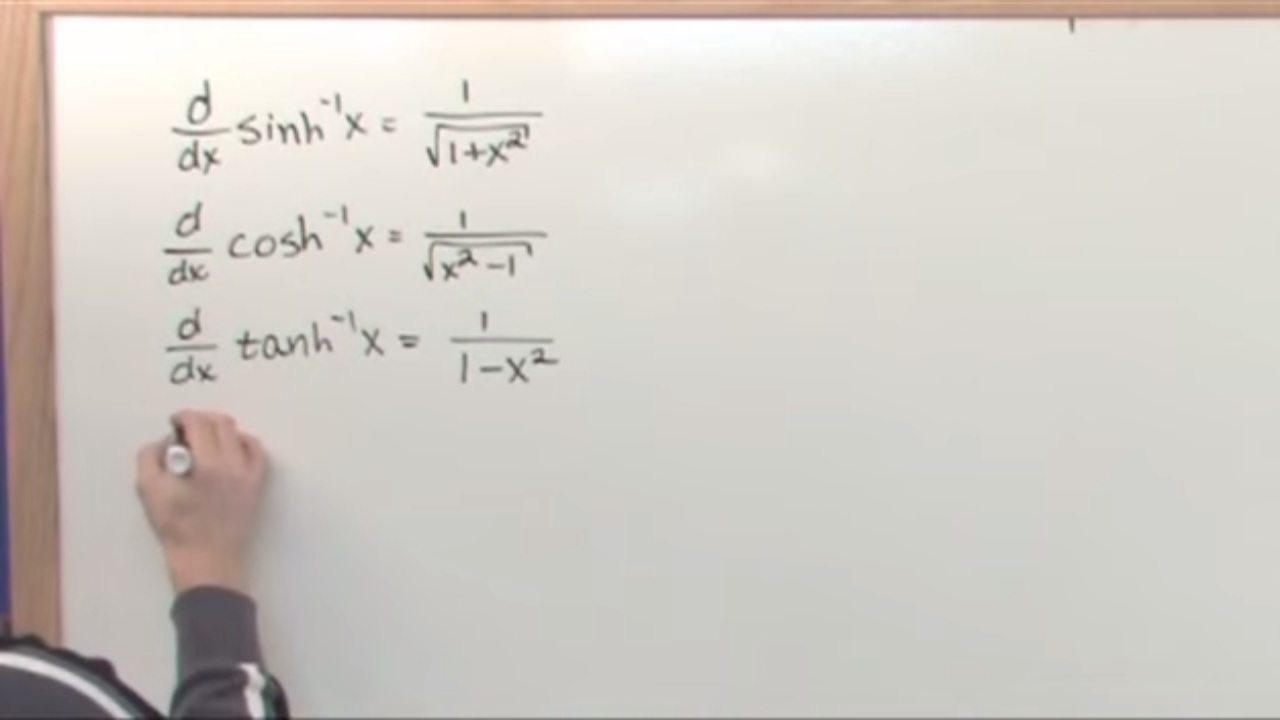
Lesson 4 - Inverse Hyperbolic Functions (Calculus 2 Tutor)

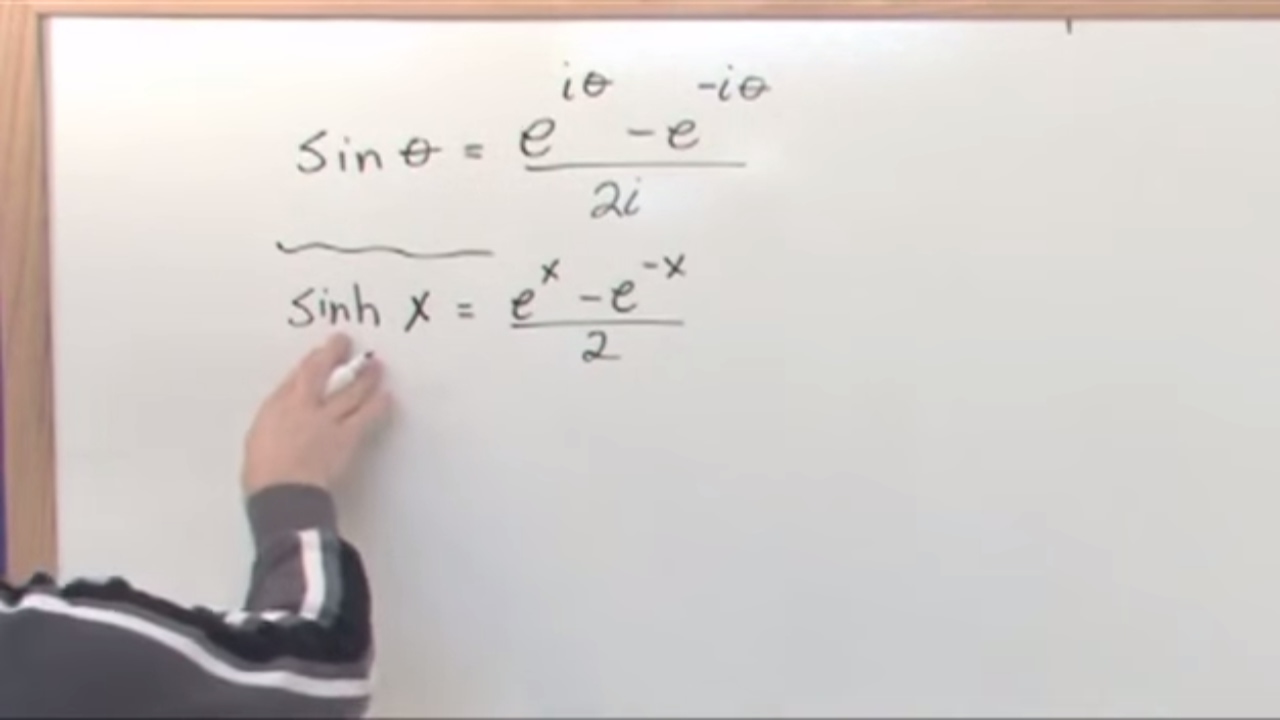
Lesson 3 - Hyperbolic Functions (Calculus 2 Tutor)

Calculus 2 Lecture 6.6: A Discussion of Hyperbolic Functions

Why hyperbolic functions are actually really nice

DERIVATIVES that keep getting HARDER 🤯 | jensenmath.ca

Rate This

5.0 / 5 (0 votes)

Thanks for rating: