Triple integrals 2 | Double and triple integrals | Multivariable Calculus | Khan Academy
TLDRThe video script discusses the concept of calculating the mass of a volume with variable density using triple integrals. It explains that while basic geometry can be used for constant density, the mass of a variably dense volume requires integrating the product of the density function and the volume differential over the domain. The example involves a rectangular domain with given boundaries and a density function dependent on x, y, and z. The process of integrating with respect to z, then y, and finally x is demonstrated, leading to the calculation of the mass in kilograms. The video emphasizes the complexity of triple integrals and the importance of setting them up correctly, even when analytical evaluation is challenging.
Takeaways
- 📏 The concept of volume calculation using integrals was discussed, specifically for a rectangle using triple integrals.
- 🔄 The video highlighted the transition from basic geometry to more complex integral calculus for volume calculation.
- 🎯 The importance of considering variable density in calculating mass, not just volume, was emphasized.
- 📌 The script introduced the density function, rho, which varies with respect to the position in space.
- 🔢 The relationship between mass, density, and volume was clarified: mass equals density times volume.
- 🌀 The process of setting up a triple integral to calculate the mass of a variably dense volume was demonstrated.
- 📈 The integration was performed in a specific order: dz first, then dy, and finally dx, with respective boundaries for each.
- 📊 The antiderivatives for each integral step were calculated, leading to the final expression for mass.
- 📚 The example used rectangular coordinates and assumed the density was in kilograms per meter cubed.
- 🔍 The video script served as an educational tool for understanding triple integrals and their applications.
- 🚀 The next video will cover more complex triple integrals and demonstrate how to switch the order of integration.
Q & A
What was the main topic of the video?
-The main topic of the video was the calculation of the mass of a volume with a non-constant density using triple integrals.
How was the volume of the rectangle initially calculated?
-The volume of the rectangle was initially calculated using a triple integral with respect to z, after recognizing it as a constant-valued function.
What is a density function?
-A density function, denoted as rho, is a function that gives the density at any given point within a volume, which can vary depending on the location within the volume.
How is mass related to density and volume?
-Mass is related to density and volume by the equation: mass = density × volume. Alternatively, density can be seen as mass divided by volume.
What are the units used for the x, y, z coordinates and density in the example provided?
-In the example, the x, y, z coordinates are in meters, and the density is in kilograms per meter cubed (kg/m³).
What was the order of integration used to calculate the mass of the variably dense volume?
-The order of integration used was dz first, then dy, and finally dx, with the respective boundaries for z being 0 to 2, y being 0 to 4, and x being 0 to 3.
What was the antiderivative of xyz with respect to z?
-The antiderivative of xyz with respect to z is xyz²/2.
What was the result of integrating the antiderivative of xyz with respect to z from 0 to 2?
-The result of integrating the antiderivative from 0 to 2 was 2xy, with the specific evaluation yielding 2xy - 0, which simplifies to 2xy.
How was the remaining double integral evaluated after integrating with respect to z?
-The remaining double integral was evaluated by first taking the antiderivative of 2xy with respect to y, resulting in y²/2 * 2x, and then integrating with respect to x from 0 to 3, yielding 8x² evaluated from 0 to 3, which results in 72.
What was the final mass of the volume calculated in the video?
-The final mass of the volume, which had a volume of 24 meters cubed, was calculated to be 72 kilograms.
What is the main challenge when dealing with triple integrals, according to the video?
-The main challenge with triple integrals is the complexity and difficulty in evaluating them analytically, especially when dealing with complicated boundaries or density functions.
What was emphasized in the video regarding the expectation on calculus exams?
-On calculus exams, it is often expected that students can set up triple integrals correctly. Sometimes, the exams may require students to switch the order of integration as a more advanced task.
Outlines
📐 Understanding Volume and Mass Calculation with Densities
This paragraph discusses the process of calculating the volume of a rectangle using basic geometry and then extends the concept to calculate the mass of a volume with non-constant density. The introduction of a density function is explained, which assigns a density value to every point within the volume. The mass is then determined by multiplying the density with the volume. The paragraph also introduces the concept of differential mass and explains how to set up an integral to calculate the mass of a variably dense volume, using rectangular coordinates and integrating with respect to z first.
🧮 Completing the Mass Calculation with Triple Integrals
The paragraph continues the discussion on calculating the mass of a variably dense volume using triple integrals. It details the steps of integrating the density function with respect to z, y, and x, explaining the antiderivative process and the boundaries for each integral. The calculation leads to the evaluation of the integral with respect to y, resulting in a function of x. Finally, the integral with respect to x is evaluated, yielding the total mass of the figure. The paragraph concludes by reflecting on the complexity of triple integrals and their evaluation, emphasizing the importance of setting up the integral correctly, which is often the focus in educational settings.
Mindmap
Keywords
💡triple integral
💡constant-valued function
💡density function
💡mass
💡volume differential
💡antiderivative
💡integration
💡Si units
💡scalar field
💡analytically evaluate
💡switch the order
Highlights
The video discusses the use of triple integrals to calculate the volume of a rectangular solid.
The method of using basic geometry to find volume is contrasted with the use of integrals.
The concept of a constant-valued function is introduced in relation to the volume calculation.
The video presents a scenario where the goal shifts from finding volume to determining the mass of a volume with non-constant density.
A density function, denoted by rho, is defined to represent the density at any given point within the volume.
The relationship between mass, density, and volume is explained, emphasizing that mass is density times volume.
The differential of mass, d mass, is described as the product of density at a point and the volume differential around that point.
The use of rectangular coordinates to express the volume differential is detailed.
The process of integrating with respect to z first is outlined, including the boundaries for z, y, and x.
The antiderivative of xyz with respect to z is calculated and evaluated from 2 to 0.
The remaining double integral involving y and x is discussed, with the boundaries for these variables.
The antiderivative of 2xy with respect to y is found, and the resulting integral is evaluated.
The final integral with respect to x is set up, and the evaluation process is described.
The mass of the volume is determined to be 72 kilograms, given the density in kilograms per meter cubed.
The concept of a scalar field in three dimensions is introduced, relating to the density function.
The video concludes by noting the complexity of evaluating triple integrals with more complicated boundaries or density functions.
The importance of setting up triple integrals and the ability to switch the order of integration is emphasized.
Transcripts
Browse More Related Video

Triple integrals 3 | Double and triple integrals | Multivariable Calculus | Khan Academy
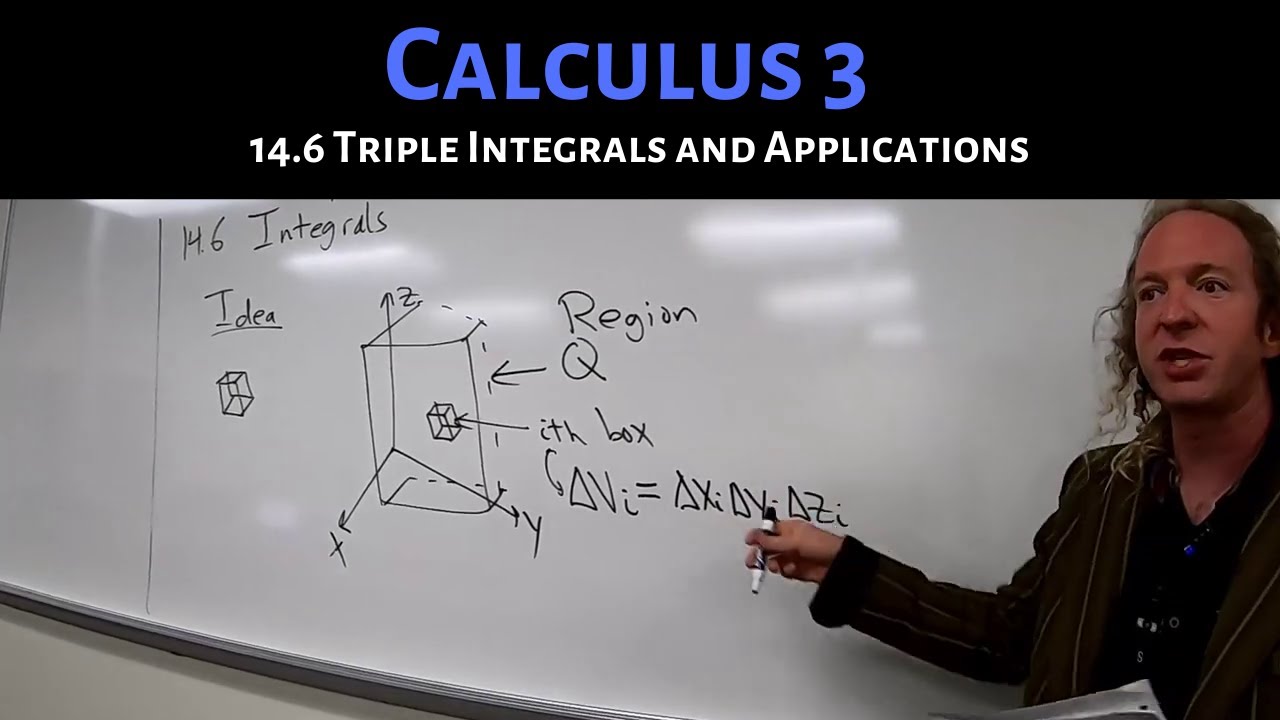
Calculus 3: Lecture 14.6 Triple Integrals and Applications

Triple integrals 1 | Double and triple integrals | Multivariable Calculus | Khan Academy

Calculating Density
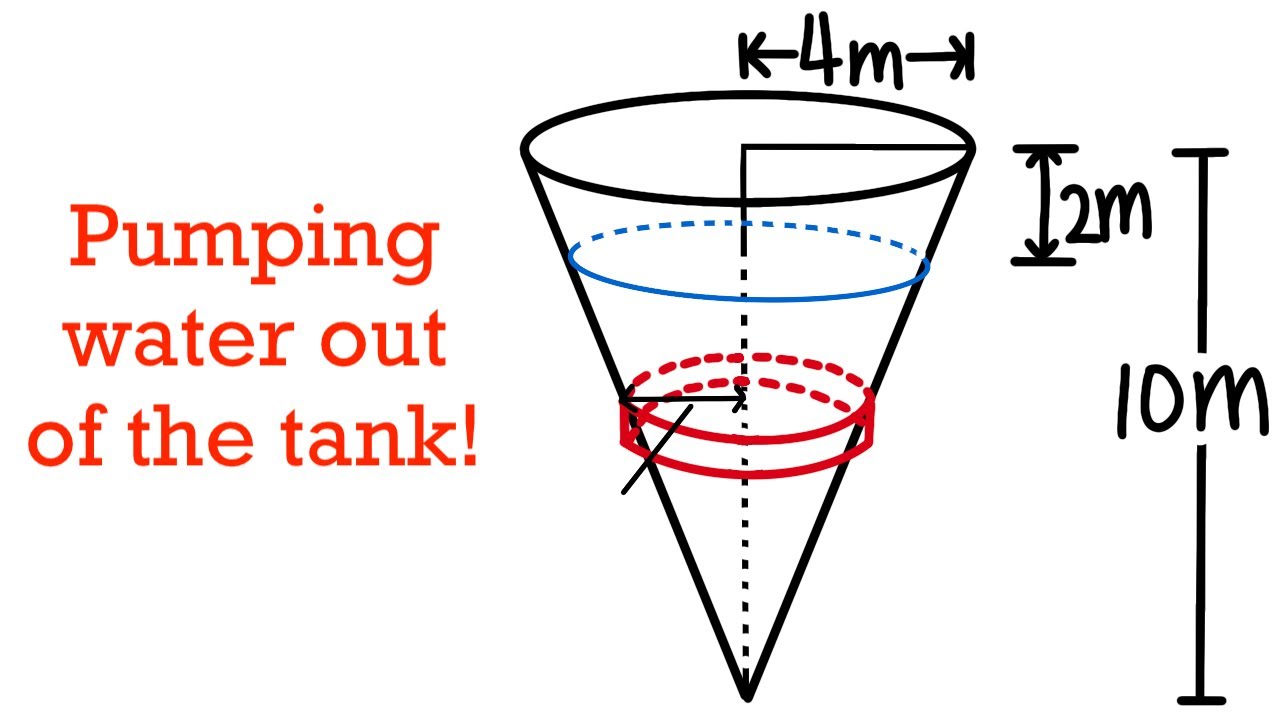
calculating work by using integral, pumping water out of a tank, calculus 2 tutorial
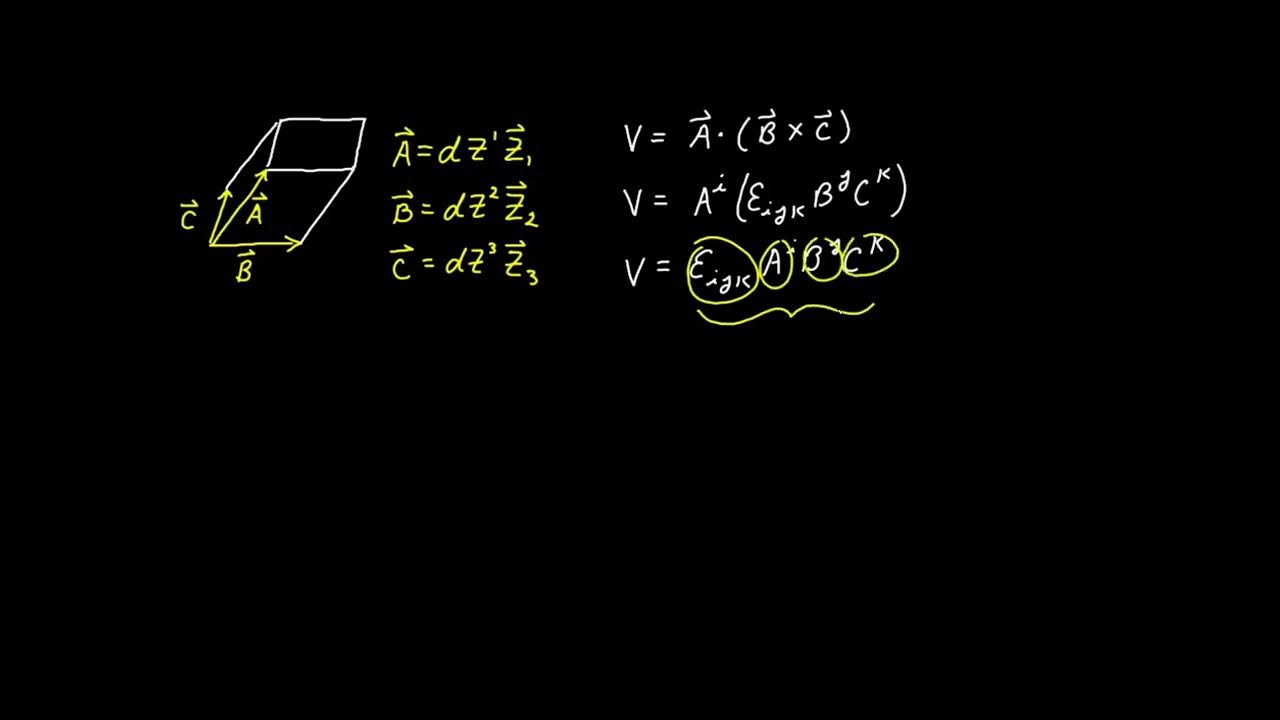
Video 54 - Integration
5.0 / 5 (0 votes)
Thanks for rating: