Triple integrals 1 | Double and triple integrals | Multivariable Calculus | Khan Academy

Khan Academy
24 Aug 200810:38
EducationalLearning
32 Likes 10 Comments

TLDRThe video script introduces the concept of triple integrals, using the example of calculating the volume of a cube with specified bounds for each dimension. It explains the process of breaking down the volume into infinitesimally small cubes and summing their volumes. The script then transitions to discussing the practical application of triple integrals in determining the mass of an object with variable density, emphasizing the importance of correctly integrating over the spatial dimensions.

Takeaways
  • πŸ“ˆ The volume of a cube can be calculated using the formula V = width * height * depth.
  • 🌟 This example is used to familiarize with the concept of triple integrals and their relation to double integrals.
  • πŸ“Š The problem is set with specific bounds for each dimension: x (0 to 3), y (0 to 4), and z (0 to 2).
  • πŸ” The process of finding the volume involves breaking it down into smaller volumes (dv) and then integrating over the respective bounds.
  • 🧩 The volume of a small cube within the larger volume is represented as dv = dx * dy * dz.
  • πŸ”„ Integration starts with the z-axis (from 0 to 2), then y-axis (from 0 to 4), and finally x-axis (from 0 to 3).
  • πŸ“š The integral with respect to z gives a constant value for a column, which is 2 * dy * dx.
  • πŸ“ˆ The double integral resulting from the z-axis integration is similar to those discussed in previous videos on double integrals.
  • πŸ€” The practical application of triple integrals extends beyond volume to calculating the mass of an object with variable density.
  • 🌐 The density function, if variable, can be represented as a function of x, y, and z (e.g., ρ = xyz).
  • πŸ’‘ The differential mass (dm) of a small volume is the product of the density at that point and the differential volume (dm = ρ * dv).
  • πŸŽ₯ The next video will demonstrate how to calculate the mass of the figure by integrating the density function over the volume.
Q & A

  • What is the purpose of calculating the volume of a cube using a triple integral?

    -The purpose of calculating the volume of a cube using a triple integral is to understand the concept and process of integrating in three dimensions, which is an extension of double integrals. This method becomes particularly useful when dealing with variable boundaries, surfaces, or curves as boundaries.

  • How does the value range of x, y, and z affect the volume calculation?

    -The value ranges for x, y, and z (0 ≀ x ≀ 3, 0 ≀ y ≀ 4, 0 ≀ z ≀ 2) define the boundaries of the cube. These ranges are used to set the limits for the integrals, ensuring that the volume calculation corresponds to the specific region of interest within the coordinate system.

  • What is the differential volume element dv mentioned in the script?

    -The differential volume element dv represents an infinitesimally small volume within the larger volume under consideration. It is expressed as dv = dx dy dz, which represents the product of the infinitesimal width (dx), height (dy), and depth (dz) of the small volume element.

  • How does the integral help in calculating the volume of a column within the cube?

    -The integral helps in calculating the volume of a column by summing up the infinitesimally small volumes (dv) along the z-axis from z=0 to z=2. This process finds the total volume of the column for a given x and y value, which is a constant value across the entire cube in this case.

  • What is the significance of switching the order of integration?

    -Switching the order of integration is significant because it allows for the most convenient evaluation of the integral based on the specific problem. The commutative property of multiplication ensures that the order in which the variables are integrated does not affect the final result.

  • How does the concept of density come into play when calculating the mass of a figure?

    -The concept of density becomes crucial when the mass of a figure is to be calculated, especially when the density is not uniform across the volume. The mass of a small volume element is found by multiplying the density at that point (which can be a function of x, y, and z) by the differential volume dv.

  • What is the antiderivative of 1 with respect to z, and how is it used in the volume calculation?

    -The antiderivative of 1 with respect to z is z, because the derivative of z is 1. This antiderivative is used to evaluate the integral with respect to z from 0 to 2, which results in 2 - 0, leaving us with a value of 2 for further integration with respect to y and x.

  • How does the volume of a sheet parallel to the zy plane get calculated?

    -The volume of a sheet parallel to the zy plane is calculated by integrating the volume of the columns (which is a constant 2 dy dx for this problem) with respect to y from 0 to 4. This results in the volume of the sheet being 8 dy, which is then integrated with respect to x from 0 to 3.

  • What is the final result of the volume calculation for the cube?

    -The final result of the volume calculation for the cube is 24 cubic units. This is obtained by integrating the volume of the sheets (8x from 0 to 3) with respect to x, which yields 24 when x is evaluated from 0 to 3.

  • Why might one need to calculate the mass of a figure instead of just its volume?

    -Calculating the mass of a figure is necessary when the density of the material within the volume is not uniform. The mass can be determined by multiplying the variable density (which can be a function of x, y, and z) by the differential volume dv and then integrating over the entire volume.

  • What is the significance of the triple integral in practical applications?

    -The triple integral is significant in practical applications where the properties of the volume under consideration vary with position, such as density in a physical mass or concentration in a chemical volume. It allows for the precise calculation of quantities like mass or total charge when these properties are not uniformly distributed.

Outlines
00:00
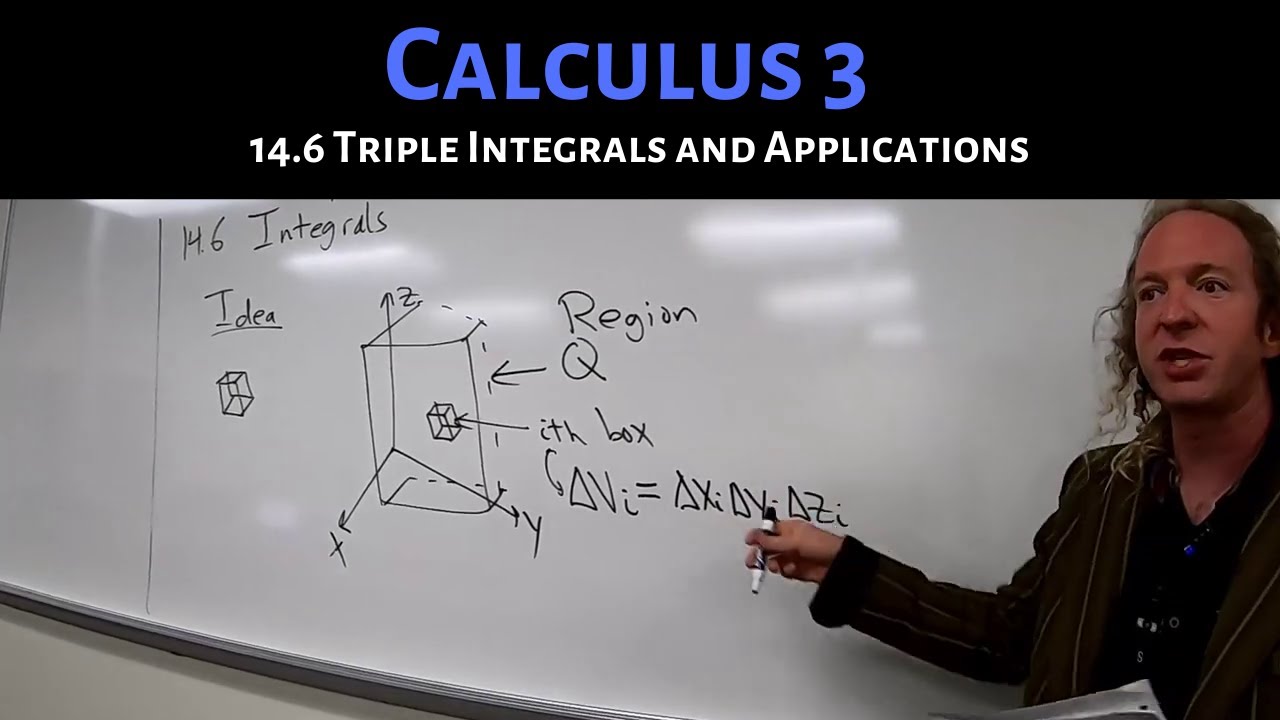
πŸ“ Introduction to Triple Integrals and Volume Calculation

This paragraph introduces the concept of triple integrals through the example of calculating the volume of a cube with specified bounds for each dimension. The cube is defined with x ranging from 0 to 3, y from 0 to 4, and z from 0 to 2. The speaker explains how to visualize this volume and the process of calculating its volume using basic geometry. The paragraph sets the stage for a deeper exploration of triple integrals by comparing them to double integrals and highlighting their utility in more complex scenarios, such as when dealing with variable boundaries and surfaces.

05:04
πŸ“Š Understanding the Process of Calculating Volume through Triple Integrals

In this paragraph, the speaker delves deeper into the process of calculating the volume of the described cube using triple integrals. The explanation begins with breaking down the volume into smaller cubes, calculating the volume of these smaller cubes, and then using integrals to sum up these volumes. The concept of volume differential (dv) is introduced as dx times dy times dz. The speaker then explains how to set up and evaluate the integrals step by step, first with respect to z, then y, and finally x, to arrive at the total volume of the cube. This process is shown to be an extension of the methods used in double integrals, but with an additional dimension of integration.

10:07
πŸ”¬ Application of Triple Integrals in Calculating Mass with Variable Density

The final paragraph shifts the focus from volume to mass, especially in scenarios where the density is not uniform throughout the volume. The speaker introduces the concept of density as a function of x, y, and z (denoted as xyz in this example), and explains how the mass of a small volume element (dmass) can be calculated by multiplying the density function by the differential volume (dv). The paragraph emphasizes the importance of triple integrals in such cases, where the density varies, and the mass cannot be simply calculated by multiplying the uniform density by the volume. The speaker concludes by noting that the next video will demonstrate how to calculate the mass of the figure using the triple integral of the density function.

Mindmap
Keywords
πŸ’‘volume
In the context of the video, 'volume' refers to the amount of space an object occupies, which is calculated by multiplying its dimensionsβ€”length, width, and height. The main theme of the video revolves around finding the volume of a cube with specified bounds for each dimension. The volume is used as a foundational concept to further explore more complex ideas such as triple integrals and mass calculations in three-dimensional space.
πŸ’‘triple integral
A 'triple integral' is a mathematical concept used to calculate the volume of a solid object in three-dimensional space, especially when the boundaries are not straightforward. In the video, the presenter uses the example of a cube with variable bounds (0 ≀ x ≀ 3, 0 ≀ y ≀ 4, 0 ≀ z ≀ 2) to illustrate how to set up and compute a triple integral. This concept is essential for solving more complex problems in calculus and physics.
πŸ’‘geometry
Geometry is a branch of mathematics concerned with the properties and relations of points, lines, surfaces, and solids. In the video, basic geometric principles are mentioned as a way to initially approach the problem of finding the volume of the cube. The presenter, however, transitions from basic geometry to more advanced concepts like triple integrals to provide a deeper understanding of the volume calculation.
πŸ’‘integration
Integration is a fundamental concept in calculus that involves finding the accumulated quantity, such as volume or area, under a curve. In the video, integration is used to calculate the volume of the cube by summing up infinitesimally small volumes (dv) along the x, y, and z axes. This process is demonstrated through the step-by-step evaluation of a triple integral.
πŸ’‘antiderivative
An antiderivative, also known as an indefinite integral, is a function that represents the reverse process of differentiation. In the context of the video, the presenter uses antiderivatives to evaluate the integrals and find the volume of the cube. The antiderivative is a crucial tool in integration, allowing the calculation of volumes and other quantities from given functions.
πŸ’‘density
Density is a property of matter that represents the mass per unit volume. In the video, the concept of density is introduced to explain how the mass of an object can be calculated by multiplying the density function, which varies with position (x, y, z), by the differential volume (dv). This concept is essential for understanding how mass can be determined in more complex scenarios where density is not uniform throughout the object.
πŸ’‘differential volume (dv)
The term 'differential volume' or 'dv' refers to an infinitesimally small volume element in three-dimensional space, used in integration to find the total volume of an object. In the video, dv is represented as the product of differentials in each dimension (dx, dy, dz), and it is the building block for calculating the volume through triple integration.
πŸ’‘coordinates
Coordinates are a set of numerical values that determine the position of a point in space. In the video, rectangular (Cartesian) coordinates are used to define the bounds of the cube (x, y, z) and to set up the integrals for calculating the volume. Understanding coordinates is crucial for visualizing and calculating volumes and other properties in multi-dimensional space.
πŸ’‘variable boundaries
Variable boundaries refer to the changing or non-uniform limits of an integral's region of integration. In the video, the variable boundaries are the conditions given for x, y, and z (0 ≀ x ≀ 3, 0 ≀ y ≀ 4, 0 ≀ z ≀ 2), which make the calculation of the volume more complex than with constant boundaries. The presenter uses these variable boundaries to demonstrate the process of setting up and evaluating a triple integral.
πŸ’‘mass
Mass is a measure of the amount of matter in an object, typically measured in kilograms. In the video, the concept of mass is introduced to discuss how the mass of an object with variable density can be calculated using triple integrals. The presenter suggests that if the density (mass per unit volume) varies with position, then the mass of the object can be found by integrating the product of the density function and the differential volume over the entire region.
Highlights

Exploring the concept of triple integrals and their relation to double integrals.

Describing the process of calculating the volume of a cube with given constraints on x, y, and z axes.

Using basic geometry to multiply width, height, and depth to find the volume.

Introducing the concept of a triple integral as the sum of infinitesimally small volumes.

Defining the volume differential (dv) as the product of infinitesimal widths (dy), heights (dz), and depths (dx).

Explaining the process of integrating in the z-direction to find the volume of a column.

Expressing the volume of a column as a function of x and y, which simplifies to a constant value due to the constants in the problem.

Integrating in the y-direction to find the volume of a sheet parallel to the zy plane.

Summing up sheets in the x-direction to obtain the total volume of the figure.

Evaluating the triple integral by first integrating with respect to z, yielding a double integral.

Demonstrating that the triple integral results in a double integral when dealing with a constant function of x and y.

Discussing the practical application of triple integrals in calculating the mass of a figure with non-uniform density.

Introducing the concept of density as a variable function of x, y, and z within a volume.

Describing how to calculate the mass by multiplying the differential mass (dv) with the density function xyz.

Explaining that the mass calculation involves integrating the product of the density function and the differential volume.

Highlighting the importance of careful computation and the use of antiderivatives in evaluating triple integrals.

Promising a follow-up video that will demonstrate the calculation of mass for a figure with variable density.

Transcripts

Browse More Related Video

Triple integrals 2 | Double and triple integrals | Multivariable Calculus | Khan Academy

Double integrals 4 | Double and triple integrals | Multivariable Calculus | Khan Academy

Calculus 3: Lecture 14.6 Triple Integrals and Applications

Triple Integrals in Rectangular Coordinates

Triple Integrals in Cylindrical Coordinates

The Divergence Theorem

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsTriple IntegralsVolume CalculationMass EstimationVariable DensityGeometryCube PropertiesIntegration TechniquesEducational ContentMath Tutorial