The Fundamental Theorem of Calculus. Part 2
TLDRThe video script offers a clear explanation of the Fundamental Theorem of Calculus Part 2, emphasizing its significance in calculating definite integrals. It illustrates the process through a step-by-step example, where finding the antiderivative of a given function and evaluating it at the limits of integration is the key. The example demonstrates integrating (x + 2) from 1 to 3, resulting in a final answer of 8, highlighting the theorem's practical application.
Takeaways
- 📚 The Fundamental Theorem of Calculus Part 2 is introduced as a crucial concept for calculating definite integrals.
- 🧮 To find a definite integral from A to B of a function f(x), one must first identify the antiderivative F(x) of f(x).
- 📈 The notation F(x) with a bar on top signifies the evaluation of the antiderivative from A to B.
- 🔢 The process of evaluating the antiderivative involves calculating F(B) - F(A), where B and A are the upper and lower limits of integration, respectively.
- 🌟 The antiderivative F(x) is found by integrating the function f(x), often requiring the use of integration techniques.
- 📌 For a polynomial function, the antiderivative can be found by increasing the exponent by 1 and dividing by the new exponent.
- 🔍 Verifying an antiderivative can be done by differentiating it back to see if the original function is obtained.
- 👉 An example given in the script demonstrates the calculation of the definite integral of x + 2 from 1 to 3.
- 🧮 The example shows the step-by-step process of substituting the upper limit (3) and lower limit (1) into the antiderivative and performing the necessary arithmetic.
- 🎯 The final result of the example is 8, illustrating the value of the definite integral from 1 to 3 for the function x + 2.
- 📝 The script emphasizes the simplicity and importance of the Fundamental Theorem of Calculus Part 2 in evaluating definite integrals.
Q & A
What is the main topic of the video?
-The main topic of the video is the Fundamental Theorem of Calculus Part 2, which explains how to calculate a definite integral.
What does the Fundamental Theorem of Calculus Part 2 state?
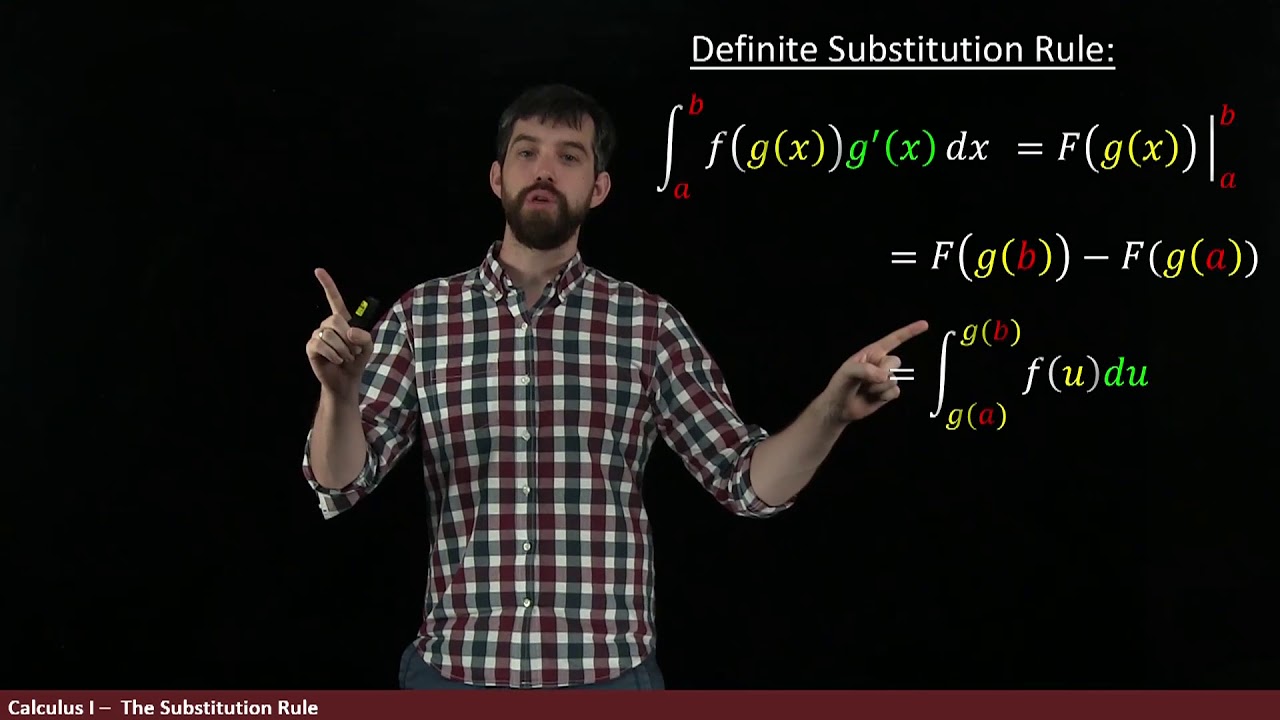
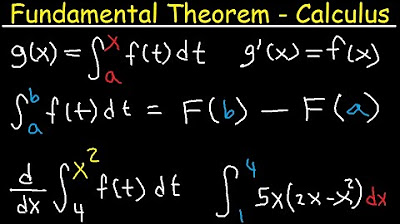
-The Fundamental Theorem of Calculus Part 2 states that to calculate a definite integral from A to B of a function, one must find the antiderivative (capital F of X) and evaluate it from B to A, effectively computing F of B minus F of A.
How does one find the antiderivative of a function?
-To find the antiderivative of a function, one must find a function that, when differentiated, yields the original function. For example, the antiderivative of X to the power of n is (X to the power of (n+1))/(n+1).
What is the significance of the antiderivative in the context of the Fundamental Theorem of Calculus Part 2?
-The antiderivative is significant because it is used to evaluate definite integrals. By finding the antiderivative of the integrand, one can apply the Fundamental Theorem to calculate the definite integral by evaluating the antiderivative at the upper and lower limits of integration.
How can you verify if a function is the correct antiderivative of another function?
-To verify if a function is the correct antiderivative, one should differentiate the suspected antiderivative and check if it results in the original function.
What is the example problem solved in the video?
-The example problem solved in the video is to calculate the definite integral from 1 to 3 of the function X + 2.
How does the process of calculating the definite integral of X + 2 from 1 to 3 work?
-The process involves finding the antiderivative of X + 2, which is (1/2)X^2 + 2X. Then, the antiderivative is evaluated at the upper limit, 3, and at the lower limit, 1, and the results are subtracted from each other to find the value of the definite integral.
What is the result of the example problem in the video?
-The result of the example problem is 8, which is the value of the definite integral from 1 to 3 of the function X + 2.
What is the role of the upper and lower limits of integration in the calculation?
-The upper and lower limits of integration are crucial as they define the interval over which the integration is performed. The definite integral is calculated by evaluating the antiderivative at the upper limit and subtracting the evaluation at the lower limit.
Why is the Fundamental Theorem of Calculus Part 2 important for calculus?
-The Fundamental Theorem of Calculus Part 2 is important because it provides a practical method for calculating definite integrals, which are essential in various applications of calculus, including determining areas under curves and solving problems in physics and engineering.
How does the process of evaluating the antiderivative at the limits of integration give the area under the curve?
-Evaluating the antiderivative at the limits of integration and subtracting the lower limit evaluation from the upper limit evaluation effectively calculates the signed area under the curve between the two limits, which is the basis for many applications of definite integrals.
Outlines
📚 Introduction to Fundamental Theorem of Calculus Part 2
This paragraph introduces the topic of the video, which is the Fundamental Theorem of Calculus Part 2. It explains that this theorem is essential for calculating a definite integral and is a concept that many people use, even if they may not recall its specific name. The theorem states that to find the definite integral from A to B of a function f(x)dx, one must find the antiderivative F(x) and evaluate it from B to A, using the notation F(B) - F(A). The paragraph emphasizes the importance of understanding antiderivatives and provides a basic example to illustrate the process.
Mindmap
Keywords
💡Fundamental Theorem of Calculus Part 2
💡Definite Integral
💡Antiderivative
💡Limits of Integration
💡Evaluation
💡Function
💡Derivative
💡Area under the curve
💡Arithmetic
💡Power Rule
💡Constant
Highlights
The video discusses the Fundamental Theorem of Calculus Part 2, a key concept in calculus.
The theorem explains how to calculate a definite integral, which is a fundamental operation in calculus.
To find a definite integral, one must first identify the antiderivative of the function.
The process involves evaluating the antiderivative at the upper limit of integration and subtracting the evaluation at the lower limit.
The antiderivative is represented by capital F of X in the theorem's explanation.
The video provides a practical example of calculating the definite integral from 1 to 3 of X + 2.
For a function like X to the power of 1, the antiderivative is X to the power of 2 divided by 2.
The antiderivative of a constant, like 2, is simply 2X.
To verify an antiderivative, one can take its derivative to retrieve the original function.
The video demonstrates the substitution of the upper limit (3) into the antiderivative to find F of 3.
Similarly, the lower limit (1) is substituted into the antiderivative to find F of 1.
The final result is obtained by performing the arithmetic of the calculated values from the upper and lower limits.
The video concludes that the definite integral of the given function from 1 to 3 is 8.
The Fundamental Theorem of Calculus Part 2 is emphasized as crucial for calculating definite integrals.
The process of finding a definite integral involves finding the antiderivative, substituting the limits, and simplifying the result.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: