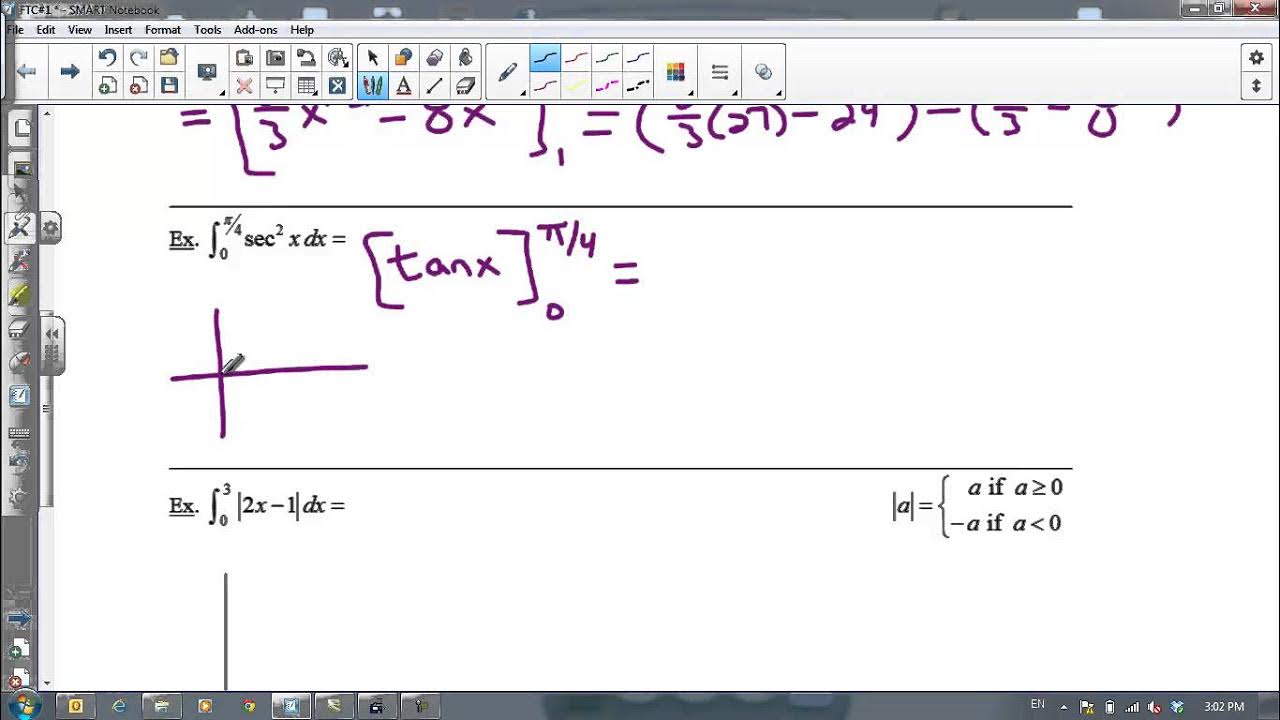
Definite Integral
TLDRThis video script delves into the distinction between indefinite and definite integrals, emphasizing the role of limits of integration in definite integrals. It illustrates the process of evaluating definite integrals through examples, highlighting the use of the Fundamental Theorem of Calculus Part 2. The script guides viewers through finding anti-derivatives and calculating specific values of integrals, demonstrating how constants cancel out in the process. The examples provided cover a range of scenarios, from straightforward calculations to more complex integrals involving natural logs and power rules.
Takeaways
- 📌 The difference between indefinite and definite integrals is that the definite integral includes lower and upper limits of integration (a and b), while the indefinite integral does not.
- 📈 Definite integrals provide a specific numerical value, whereas indefinite integrals yield a function in terms of x, with an arbitrary constant (c).
- 🌟 The fundamental theorem of calculus, Part 2, states that the definite integral of a function f from a to b is equal to F(b) - F(a), where F is the anti-derivative of f.
- 🔢 To evaluate a definite integral, find the anti-derivative of the integrand, substitute the upper limit into the anti-derivative to get F(b), and then substitute the lower limit to get F(a), and finally subtract F(a) from F(b).
- 📚 When dealing with definite integrals, the constant of integration (c) is not needed and will cancel out when calculating the difference F(b) - F(a).
- 🧮 Example problem: To evaluate ∫ from 2 to 5 of 8 dx, first find the anti-derivative (8x), then evaluate from x=5 (F(b) = 40) and x=2 (F(a) = 16), and the result is 40 - 16 = 24.
- 📝 For the integral of 5x - 4, use the power rule to find the anti-derivatives (5x^2/2 and -4x), and evaluate from x=1 to x=4 to get the result 25.5 (or 51/2 as an improper fraction).
- 🤔 When integrating a function like 8x^(-3), rewrite the expression to work with positive exponents (2x^2) and evaluate from x=-3 to x=4 to get the result 7/36.
- 🌐 For the integral of 5/x from 1 to e, use the natural logarithm (ln x) as the anti-derivative, evaluate from ln(1) to ln(e), and the result is 5, since ln(e) = 1 and ln(1) = 0.
- 🛠️ When integrating a function like √x (x^(1/2)), rewrite it as x^(-1/2), find the anti-derivative (2x^(1/2)), and evaluate from x=4 to x=9 to get the result 2.
Q & A
What is the main difference between an indefinite integral and a definite integral?
-A definite integral includes the lower limit 'a' and the upper limit 'b', representing the limits of integration, whereas an indefinite integral does not have these limits and results in a function in terms of 'x' plus a constant 'C'.
What is the integrand in the context of a definite integral?
-The integrand, denoted as 'f(x)', is the function that is being integrated with respect to the variable 'x' in a definite integral.
What does the integral sign represent?
-The integral sign represents the concept of a limit of sums, which is the foundation of the process of integration.
What is the Fundamental Theorem of Calculus, Part 2, and how does it relate to definite integrals?
-The Fundamental Theorem of Calculus, Part 2, states that the definite integral of a function 'f' from 'a' to 'b' is equal to the antiderivative of 'f' evaluated at 'b' minus the antiderivative evaluated at 'a', denoted as F(b) - F(a), where F is the antiderivative of f.
Why is the constant of integration 'C' not included in the result of a definite integral?
-The constant of integration 'C' cancels out when evaluating the difference between the antiderivative at the upper and lower limits, thus it is not needed in the final result of a definite integral.
How do you evaluate the definite integral of 8 dx from 2 to 5?
-The antiderivative of 8 dx is 8x. Evaluating this from 2 to 5 gives us 8*5 - 8*2, which simplifies to 40 - 16, resulting in a final answer of 24.
What is the antiderivative of 5x - 4 using the power rule?
-The antiderivative of 5x is (5x^2)/2, and the antiderivative of -4x is -4x. So, the antiderivative of 5x - 4 is (5x^2)/2 - 4x.
What is the final result of evaluating the definite integral of 5x - 4 from 1 to 2?
-After finding the antiderivative and applying the limits, the result is ((5*2^2)/2 - 4*2) - ((5*1^2)/2 - 4*1), which simplifies to (20 - 8) - (5 - 4), resulting in a final answer of 24 - 1, which is 23.
How do you simplify the expression 8x^(-3) for integration purposes?
-By using the power rule, the expression simplifies to -4/x^2, where negative 3 plus 1 becomes negative 2, and we divide by 2.
What is the result of evaluating the definite integral of 8x^(-3) from -3 to 4?
-The antiderivative is -4/x^2. Evaluating from -3 to 4 gives us (-4/4^2) - (-4/(-3)^2), which simplifies to (-1/4) - (4/9), and the final result is 7/36.
What is the antiderivative of 1/x?
-The antiderivative of 1/x is the natural logarithm of|x|, denoted as ln|x|.
What is the final answer when evaluating the definite integral of 5/x from 1 to e?
-The antiderivative is 5ln|x|. Evaluating from 1 to e gives us 5ln(e) - 5ln(1), which simplifies to 5 - 0, resulting in a final answer of 5.
How do you evaluate the definite integral of the square root of x from 4 to 9?
-The antiderivative is 2x^(1/2). Evaluating from 4 to 9 gives us 2*sqrt(9) - 2*sqrt(4), which simplifies to 6 - 4, resulting in a final answer of 2.
Outlines
📚 Understanding the Difference Between Indefinite and Definite Integrals
This paragraph introduces the concepts of indefinite and definite integrals, highlighting their differences. It explains that a definite integral includes limits of integration (lower limit a and upper limit b), while an indefinite integral does not. The paragraph also describes the process of integrating, where an indefinite integral results in a function (anti-derivative) plus a constant (F(x) + C), and a definite integral yields a specific numerical value. The fundamental theorem of calculus, particularly its second part, is introduced as a key tool for evaluating definite integrals.
🧮 Evaluating Definite Integrals with Examples
The paragraph delves into the practical application of evaluating definite integrals through two examples. The first example demonstrates the process of finding the anti-derivative of a function and then using the limits of integration to find the definite integral's value. The second example involves using the power rule to find the anti-derivative and then applying the limits of integration to obtain the result. The paragraph emphasizes the cancellation of the constant of integration in definite integrals and provides a step-by-step breakdown of the calculations involved.
📈 Solving More Definite Integral Problems
This paragraph continues with the theme of evaluating definite integrals, presenting additional examples with varying complexity. It covers the integration of functions with negative exponents, the use of the natural logarithm, and the integration of functions involving square roots. The explanations include the necessary steps of rewriting expressions, applying power rules, and evaluating anti-derivatives at the given limits. The paragraph concludes with the final answers for each example, showcasing the process of simplifying and solving definite integral problems.
Mindmap
Keywords
💡Definite Integral
💡Indefinite Integral
💡Limits of Integration
💡Antiderivative
💡Fundamental Theorem of Calculus Part 2
💡Integrand
💡Variable of Integration
💡Power Rule
💡Constant of Integration
💡Integration by Substitution
💡Natural Logarithm
Highlights
The difference between an indefinite integral and a definite integral is explained, with the definite integral including lower limit a and upper limit b.
A definite integral provides a specific numerical value, whereas an indefinite integral yields a function in terms of x.
The integrand f(x) is the function being integrated, and dx represents the variable of integration.
The fundamental theorem of calculus, part two, is associated with definite integrals and states that the definite integral of f from a to b is equal to the anti-derivative of f evaluated at b minus the same evaluated at a.
The process of evaluating a definite integral is demonstrated with a simple example of ∫8 dx from 2 to 5, resulting in the answer 24.
When finding the anti-derivative of an expression, the constant c appears; however, it cancels out in definite integrals, making it unnecessary.
The anti-derivative of 5x - 4 is calculated using the power rule, resulting in (5x^2)/2 - 4x.
The evaluation of the definite integral of (5x - 4) from 1 to 4 results in a final answer of 25.5 or 51/2.
The anti-derivative of 8x^(-3) is found by applying the power rule, resulting in -4/x^2.
The definite integral of 8x^(-3) from -3 to 4 is evaluated, yielding a final answer of 7/36.
The anti-derivative of 1/x is the natural logarithm of x, ln(x), which is used to evaluate the definite integral from 1 to e of 5/x dx, resulting in the answer 5.
The definite integral of the square root of x, or x^(-1/2), from 4 to 9 is evaluated, with the final answer being 2.
The video provides a clear and detailed explanation of the difference between indefinite and definite integrals, making complex calculus concepts accessible.
The use of the fundamental theorem of calculus is demonstrated to simplify the process of evaluating definite integrals.
The importance of understanding the power rule and the constant of integration when finding anti-derivatives is emphasized.
The video walks through multiple examples of evaluating definite integrals, showcasing the application of calculus concepts in practice.
The process of simplifying and rewriting expressions before integrating is highlighted as a crucial step in solving integral problems.
The video concludes with a summary of the key points, reinforcing the understanding of definite integrals and their evaluation.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: