Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (10 of 92) What is Normalization? Ex. 1

Michel van Biezen

6 Feb 201706:55

EducationalLearning

32 Likes 10 Comments

TLDRIn this video, the concept of normalization in quantum mechanics is explored to determine the probability of finding a particle at a specific location. The script explains that the probability density function, or wave function, must be normalized so that its integral over all possible locations equals 1, representing a 100% probability. The process involves finding the correct constant to normalize the wave function, ensuring that the probability density accurately reflects the true likelihood of the particle's presence at any given point within a defined range.

Takeaways

📈 The concept of a probability density function (PDF) is introduced to describe the likelihood of finding a particle at a specific location.
🌐 Normalization of the PDF is necessary to ensure that the integral of the function over all possible locations equals 1, representing a 100% probability.
📌 The amplitude of the PDF indicates the relative likelihood of finding a particle at a particular location, but it is not an absolute probability.
🔄 The product of a wave function and its complex conjugate results in a real number, which is crucial for normalization.
🧬 For a particle confined between points A and B, the wave function must be normalized such that the integral from A to B equals 1.
🔢 The constant 'a' in the wave function is determined by the condition that the integral from A to B equals 1, leading to 'a' being equal to 1 over the square root of (B - A).
🌊 The normalized wave function for a single particle in one dimension with no forces acting on it and constant potential energy is given by 1 over the square root of (B - A) times e to the (±IKX - ΩT).
🎲 The normalization process ensures that the probability density function accurately represents the true probability of finding a particle at any given location.
📊 The area under the curve of the PDF represents the probability of finding the particle within a certain range of positions.
🔍 By selecting a value for X, one can determine the exact probability of finding the particle at that specific location.
📈 Normalization is a fundamental step in quantum mechanics to ensure that the wave function represents a physically meaningful probability distribution.

Q & A

What is the purpose of normalizing the probability density function?
-The purpose of normalizing the probability density function is to ensure that the integral of the function over the entire possible range of values equals 1, which represents a 100% probability of finding the particle within that range.
What does a large amplitude in the probability density function indicate?
-A large amplitude in the probability density function indicates a higher likelihood of finding the particle at that particular location.
What does a small amplitude in the probability density function indicate?
-A small amplitude in the probability density function indicates a lower likelihood of finding the particle at that particular location.
What is the condition that must be satisfied for a function to be considered a valid probability function?
-The condition that must be satisfied for a function to be considered a valid probability function is that the integral of the product of the wave function and its complex conjugate over all space must equal one.

Outlines

00:00

🌟 Introduction to Probability Density Function

This paragraph introduces the concept of a probability density function (PDF) and its importance in quantum mechanics. It explains that the PDF, denoted as P(X, T), describes the likelihood of finding a particle at a specific location 'X'. The amplitude of the PDF indicates the relative probability, with larger amplitudes suggesting higher likelihood and vice versa. However, it emphasizes that the probability is relative and not an absolute value, as the area under the curve of the PDF must equal 1, representing a 100% probability over its defined range. The paragraph also sets up a hypothetical scenario where a particle is confined between 'a' and 'B', and discusses the need to normalize the wave function to ensure the total probability is 1 within this range.

05:03

📏 Normalization of the Wave Function

This paragraph delves into the process of normalizing the wave function to obtain a probability density function. It explains that normalization involves finding the correct constant 'a' such that the integral of the product of the wave function and its complex conjugate over the entire possible range of the particle's location equals 1. This ensures that the probability density function accurately represents the true probability of finding the particle at any given location. The paragraph provides a mathematical example of normalizing a wave function for a single particle in one dimension with no forces acting on it and a constant potential energy. The resulting normalized wave function is given as 1/√(B - a) times e^(i(kx - ωt)), highlighting the significance of the normalization process in quantum mechanics.

Mindmap

Keywords

💡Normalization

Normalization in the context of the video refers to the process of adjusting a probability distribution or wave function so that its integral over the entire possible range of values equals 1. This ensures that the function accurately represents a probability distribution, where the area under the curve represents the total probability, which must equal 100%. In the video, normalization is crucial for transforming the probability density function into a true probability function, allowing for the accurate calculation of the likelihood of finding a particle at a specific location within a given range.

💡Probability Density Function (PDF)

The Probability Density Function (PDF) is a mathematical function that describes the relative likelihood of a particle being found at a particular location. Unlike a probability function, which gives the absolute probability, the PDF provides a relative likelihood, with higher values indicating areas where the particle is more likely to be found. The integral of the PDF over the entire space must equal 1 for it to be a valid probability distribution.

💡Wave Function

In quantum mechanics, the wave function is a mathematical description of the quantum state of a particle or system of particles. It contains all the information about the system and is used to calculate probabilities. The wave function is complex-valued, and its modulus squared represents the probability density. The script discusses a specific wave function that describes a single particle in one dimension with no forces acting on it and a constant potential energy.

💡Complex Conjugate

The complex conjugate of a complex number is obtained by changing the sign of the imaginary part while keeping the real part the same. In the context of wave functions, the product of a wave function and its complex conjugate results in a real number, which is essential for normalization and calculating probability densities.

💡Integral

In mathematics, an integral represents the accumulation of a quantity over a range or region. In the context of the video, the integral is used to calculate the area under the probability density function, which must equal 1 for the function to be normalized and represent a valid probability distribution.

💡Amplitude

Amplitude in a wave function context refers to the magnitude or height of the wave at a particular point. In the video, it is used to describe the relative likelihood of finding a particle at a certain location; a higher amplitude indicates a higher probability, while a lower amplitude indicates a lower probability.

💡Quantum Mechanics

Quantum mechanics is a fundamental theory in physics that describes the behavior of matter and energy at very small scales, such as atomic and subatomic particles. It introduces concepts like wave functions, probability densities, and quantum states, which are essential for understanding the behavior of particles at the quantum level.

💡Potential Energy

Potential energy is the stored energy an object has due to its position in a force field or as a result of its configuration. In the context of the video, the potential energy is mentioned as being constant, which implies that there are no forces acting on the particle, and it is in a stable state.

💡One-Dimensional

One-dimensional refers to a system or scenario that has only one independent variable or degree of freedom. In the video, the wave function represents a single particle in one dimension, meaning the particle's position and the wave function's effects are only considered along a single line.

💡Constant Potential Energy

Constant potential energy implies that the energy associated with the position of a particle in a force field does not change with position. This is significant in the video because it allows for the simplification of the wave function and the illustration of quantum mechanical principles without the complexity of varying forces.

💡Particle

In the context of the video, a particle refers to a small, often subatomic, entity that is subject to the principles of quantum mechanics. The behavior of particles is described by wave functions, and the probabilities of finding them in certain locations are calculated using probability density functions.

Highlights

The importance of normalizing the probability density function to ensure the total probability equals 1.

The concept of probability density and how it relates to finding a particle at a particular location.

The equation P(X,T) as the probability distribution function for a particle's location.

The condition that the integral of the wave function and its complex conjugate over all space must equal 1.

The hypothetical scenario of a particle being more likely to be found in the middle than close to the edges (between points A and B).

The mathematical process of normalizing the wave function by integrating from A to B and ensuring the result equals 1.

The product of the wave function and its complex conjugate resulting in a squared term, which simplifies to 1.

The method for finding the constant 'a' by integrating from A to B and setting the result equal to 1/(B-A).

The final normalized wave function for a single particle with no forces acting on it and constant potential energy.

The significance of the constant 'a' being equal to 1/√(B-A) for the wave function to be normalized.

The practical application of the normalized wave function in determining the true probability of finding a particle at a specific location.

The process of integrating the product of the wave function and its complex conjugate over a defined range.

The role of the complex conjugate in the normalization process of the wave function.

The concept that the area under the probability density function curve represents the probability of finding a particle within a certain range.

The explanation of how the wave function's constant is determined to ensure the probability density function is normalized.

The discussion of how the normalized wave function allows for the direct reading of the probability of finding a particle at a specific location.

Transcripts

Browse More Related Video

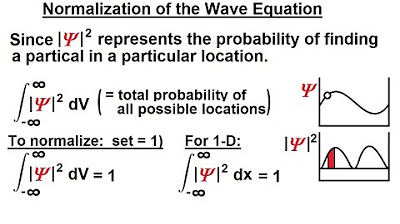
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (2 of 92) Normalization of the Wave Equation

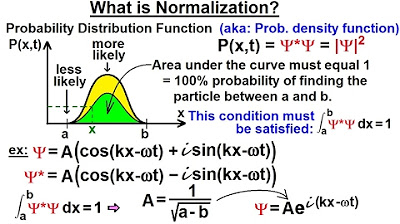
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (11 of 92) What is Normalization? Ex. 2

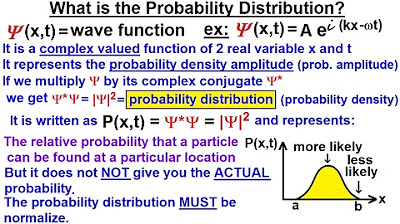
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (9 of 92) What is the Prob Distribution?

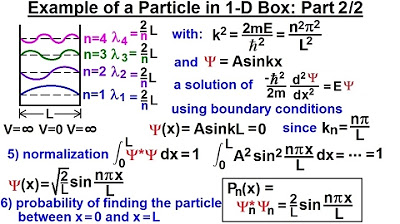
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (20 of 92) Particle in 1-D Box: Example 2/2

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (25 of 92) Prob. of a Particle 1-D Box n=1

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (18 of 92) Particle in 1-D Box: Gen. Appr.