Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (9 of 92) What is the Prob Distribution?

Michel van Biezen
5 Feb 201705:39
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the concept of probability distribution in quantum mechanics, explaining how the wave function, a complex-valued function, represents the probability density amplitude of finding a particle at a particular location. It clarifies that the magnitude of the wave function, obtained by multiplying it with its complex conjugate, gives the probability distribution, which is a relative measure rather than an absolute one. The script also touches on the need to normalize the probability distribution to get the actual probability of finding a particle at a specific location, a topic to be explored in the subsequent video.

Takeaways
  • 🌊 The wave function represents the probability density amplitude of a particle, which is complex-valued and not a direct representation of reality.
  • 📈 The magnitude of the wave function is related to the probability of finding a particle at a particular location, with larger magnitudes indicating higher probabilities.
  • 🤝 To obtain a direct connection to the probability of finding a particle, the wave function must be multiplied by its complex conjugate, resulting in a real number that represents the probability distribution.
  • 🔄 The complex conjugate of a wave function involves changing the sign of the imaginary part, which is crucial for eliminating the imaginary component from the probability distribution.
  • 📊 The probability distribution, also known as the probability density, is represented by the square of the wave function (Ψ^2) and indicates the likelihood of finding a particle at various locations.
  • 📈 The probability density function (PDF) is a graph that visually demonstrates how the likelihood of finding a particle varies with position, with higher amplitudes corresponding to higher probabilities.
  • 🔄 The probability distribution is relative, not absolute, meaning it shows the likelihood of finding a particle at one location compared to another, rather than an exact percentage chance.
  • 🌐 Normalization of the probability distribution is necessary to convert it into an actual probability function, which will be explained in a subsequent video.
  • 🔗 The wave equation and the Schrödinger equation are connected, with the wave function satisfying the Schrödinger equation and being used to determine the probability of finding a particle at a specific location.
  • 📚 The video series aims to provide a deeper understanding of the relationship between wave equations, particle behavior, and probability distributions in quantum mechanics.
Q & A

  • What is the significance of the wave function in quantum mechanics?

    -The wave function in quantum mechanics represents the probability density amplitude, which is related to the likelihood of finding a particle at a particular location.

  • Why is the wave function considered a complex-valued function?

    -The wave function is complex-valued because it contains both real and imaginary parts, which are necessary to describe the quantum state of a particle fully.

  • How does the magnitude of the wave function relate to the probability of finding a particle?

    -The magnitude of the wave function (its absolute value) is related to the probability of finding a particle at a specific location; a larger magnitude indicates a higher probability.

  • What is the complex conjugate of a wave function?

    -The complex conjugate of a wave function is obtained by changing the sign of the imaginary part of the function, which results in a real-valued function.

  • What is the probability distribution in the context of quantum mechanics?

    -The probability distribution, also known as the probability density, is the real part obtained by multiplying the wave function by its complex conjugate, which represents the likelihood of finding a particle at a certain location.

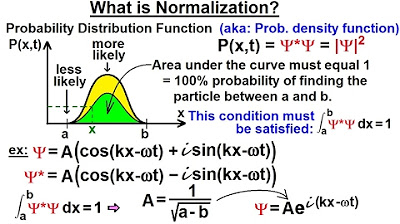
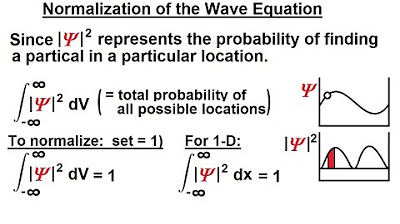
  • Why is it necessary to normalize the probability distribution function?

    -Normalization of the probability distribution function is required to ensure that the total probability of finding the particle somewhere in all possible locations sums up to 1, which is a fundamental principle in probability theory.

  • How does the probability density function (PDF) differ from the actual probability?

    -The probability density function gives the relative likelihood of finding a particle at different locations, but it is not the actual probability. To obtain the actual probability, the PDF must be normalized.

  • What does the horizontal axis represent in a probability density function graph?

    -In a probability density function graph, the horizontal axis represents the position or location along which the likelihood of finding a particle is plotted.

  • What does the vertical axis represent in a probability density function graph?

    -In a probability density function graph, the vertical axis represents the probability distribution or the likelihood of finding a particle at various positions.

  • How does the shape of the probability density function graph relate to particle location?

    -The shape of the probability density function graph indicates where particles are more likely to be found; peaks in the graph correspond to higher probabilities, while troughs correspond to lower probabilities.

  • What is the connection between the Schrödinger equation, the wave equation, and the probability of finding a particle?

    -The Schrödinger equation is a fundamental equation in quantum mechanics that describes how the wave function evolves over time. The wave equation represents the quantum state of a particle, and by solving it, one can determine the probability distribution, which gives the likelihood of finding the particle at specific locations.

Outlines
00:00
🌟 Understanding Probability Distribution in Quantum Mechanics

This paragraph introduces the concept of probability distribution in the context of quantum mechanics. It explains that the wave function, which is complex-valued, represents the probability density amplitude of finding a particle at a particular location. The magnitude of the wave function is related to the probability of finding the particle, but a direct connection is established by multiplying the wave function by its complex conjugate. This process results in the probability distribution, which is a real function that describes the likelihood of finding a particle at a certain place relative to other places. The paragraph emphasizes that the probability distribution is relative, not absolute, and that further steps are needed to obtain the actual probability of finding a particle at a specific location.

05:01
🚀 Normalization of Probability Distribution in Quantum Physics

The second paragraph delves into the necessity of normalizing the probability distribution to obtain the actual probability of finding a particle at a particular location. It mentions that the relative probability function derived from the wave function and its complex conjugate does not provide the actual probability values. The video script teases that the next video will explain how to normalize this function and connect it with the Schrödinger equation to extract meaningful information about the probability of locating a particle. The speaker also expresses excitement about the upcoming content, hinting at the depth of the topic and its implications in quantum physics.

Mindmap
Keywords
💡probability distribution
The probability distribution refers to the likelihood of finding a particle at a particular location. In the context of the video, it is derived from the wave function by multiplying it by its complex conjugate, which results in a real-valued function that represents the probability density. This distribution is crucial for understanding the quantum behavior of particles and is used to predict where particles are most likely to be found.
💡wave function
The wave function is a mathematical description used in quantum mechanics to represent the quantum state of a particle. It is a complex-valued function that encodes information about the probability amplitude of the particle's position. The magnitude of the wave function, not the function itself, is related to the probability of finding the particle at a certain location. The wave function is fundamental to the understanding of quantum mechanics and the behavior of particles at the quantum level.
💡complex conjugate
The complex conjugate of a complex number is obtained by changing the sign of its imaginary part. In the context of the video, taking the complex conjugate of the wave function is necessary to convert it into a real-valued function, which can then be used to determine the probability distribution of a particle. This process is essential for removing the imaginary part of the wave function and focusing on the magnitude, which is directly related to probability.
💡probability density
Probability density, also known as the probability density function, is a measure that describes the relative likelihood of finding a particle at a specific point in space. It is derived from the square of the wave function (or the product of the wave function and its complex conjugate) and represents the probability distribution. The probability density is not an absolute measure but a relative one, indicating where particles are more or less likely to be found compared to other locations.
💡particle
In the context of the video, a particle refers to a physical entity that is being described by the wave function in quantum mechanics. The particle could be an electron, a photon, or any other subatomic particle whose behavior is governed by the principles of quantum physics. The video discusses how the wave function and its associated probability distribution relate to the likelihood of finding this particle at a particular location.
💡quantum mechanics
Quantum mechanics is a fundamental theory in physics that describes the behavior and interactions of particles at the atomic and subatomic scale. It is the foundation upon which the concepts of wave functions, probability distributions, and complex conjugates are built. The video's discussion of wave functions and probability densities is rooted in the principles of quantum mechanics, which provide a framework for understanding the probabilistic nature of particles' locations and behaviors.
💡probability amplitude
Probability amplitude, also referred to as the wave function, is a term used in quantum mechanics to describe the likelihood of a particle's presence at a certain point in space. It is a complex number, and its magnitude (absolute value) is related to the probability of finding the particle at that point. The probability amplitude is a crucial concept because it allows for the prediction of where particles are most likely to be found, which is central to the understanding of quantum phenomena.
💡normalization
Normalization in the context of quantum mechanics is the process of adjusting the probability distribution so that the total probability over all possible outcomes sums to one. This is necessary because the probability distribution derived from the wave function does not initially represent an actual probability, but only a relative likelihood. Normalization ensures that the probability distribution is a valid probability measure that can be used to calculate the actual chances of finding a particle in a given state.
💡Schrödinger equation
The Schrödinger equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes with time. It is a partial differential equation that provides a way to calculate the wave function of a particle, which in turn is used to determine the probability distribution of the particle's location. The video script implies that understanding the relationship between the wave equation and the Schrödinger equation is crucial for predicting the behavior of particles in quantum mechanics.
💡one dimension
In the context of the video, one dimension refers to the simplification of the physical scenario where a particle is considered to move or exist along a single line, with only one spatial coordinate being relevant. This simplification is often used in introductory quantum mechanics to illustrate key concepts such as wave functions and probability distributions without the complexity of multiple dimensions.
Highlights

The wave function represents the probability density amplitude, also known as the probability amplitude.

The magnitude of the wave function reflects the probability of finding a particle in a particular place.

To obtain a direct connection to the probability of finding a particle, the wave function must be multiplied by its complex conjugate.

The complex conjugate is found by changing the sign of the imaginary part of a complex number.

The product of the wave function and its complex conjugate is known as the probability distribution.

The probability distribution represents the relative likelihood of finding a particle at a specific location compared to others.

The horizontal axis in the probability distribution graph represents position, while the vertical axis represents the probability density function.

A higher amplitude on the probability density graph indicates a higher likelihood of finding the particle at that location.

The probability distribution must be normalized to give the actual probability of finding a particle at a specific location.

Normalization of the probability distribution will be explained in the next video.

The wave equation is central to understanding the behavior of particles in quantum mechanics.

The relationship between the Schrödinger equation, wave equation, and probability of finding a particle will be further explored in the next video.

The concept of probability distribution is crucial for predicting the outcomes of quantum events.

The probability density function is a key tool in quantum mechanics for understanding the distribution of particles.

The video provides a foundational understanding of how to interpret and use the wave function in quantum mechanics.

The explanation clarifies the transition from the complex wave function to a practical probability distribution that can be used for predictions.

The discussion emphasizes the importance of the magnitude of the wave function in determining the probability of particle location.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (11 of 92) What is Normalization? Ex. 2

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (10 of 92) What is Normalization? Ex. 1

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (2 of 92) Normalization of the Wave Equation

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (20 of 92) Particle in 1-D Box: Example 2/2

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (27 of 92) Expectation Value=? 1-D Box n=1

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (25 of 92) Prob. of a Particle 1-D Box n=1

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsWaveFunctionProbabilityDensityComplexConjugateNormalizationParticleLocationPhysicsEducationElectronBehaviorOneDimensionSchrodingerEquation