Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (2 of 92) Normalization of the Wave Equation

Michel van Biezen
17 Nov 201603:11
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the concept of normalization in the context of the wave equation, emphasizing its importance in quantum mechanics. It explains that the wave function alone lacks physical meaning, but when squared, it represents the probability of locating a particle at a specific point. To ensure the total probability across all possible locations does not exceed 100%, normalization is applied by dividing the sum of probabilities by itself, resulting in a value of one. The script further clarifies that the wave function must be continuous, single-valued, and its derivative must also possess these properties for successful normalization. Additionally, the integral of the probability density function over all space must yield a finite, constant value for the function to be valid and representable as a probability distribution.

Takeaways
  • 🌊 The wave function alone lacks physical meaning, but its square represents the probability of finding a particle at a specific location.
  • πŸ”’ To ensure the total probability of finding a particle across all locations does not exceed 100%, the wave function must be normalized.
  • πŸ’‘ Normalization involves dividing the summed probabilities by themselves to equal one, making it a valid probability representation.
  • πŸ“ˆ The integral of the probability density function over all space is set to one to achieve normalization.
  • 🚫 Integration does not always span from negative to positive infinity; it can be limited by the physical boundaries of the particle's possible locations.
  • πŸ“Œ A wave function must be continuous and single-valued, akin to algebraic functions where one input (X) corresponds to one output (Y).
  • πŸ”„ The derivative of the wave function also needs to be continuous and single-valued for the function to be normalized.
  • 🌟 The probability density function, when integrated over all space, must yield a finite, constant value for the wave function to be normalizable.
  • πŸ“Š A normalized wave function is essential for representing the probability of finding a particle at any point in space.
  • πŸ” The process of normalization ensures that the wave function adheres to the principles of quantum mechanics and probability theory.
  • πŸ”‘ Normalization is a fundamental aspect of the wave function, critical for its validity as a probability density function.
Q & A

  • What is the significance of the wave equation in quantum mechanics?

    -The wave equation is fundamental in quantum mechanics as it describes the behavior of quantum systems, particularly the probability of finding a particle at a particular location in space.

  • Why is it necessary to normalize the wave function?

    -Normalization of the wave function is necessary to ensure that the total probability of finding a particle in all possible locations sums up to 100%, which is the maximum probability in quantum mechanics.

  • How is the probability of finding a particle represented in quantum mechanics?

    -The probability of finding a particle is represented by the wave function squared (ψ^2), which gives the probability density of the particle being at a specific location.

  • What does it mean for a wave function to be continuous and single-valued?

    -A wave function being continuous and single-valued means that it has no breaks or jumps, and for every specific value of the position (X), there is exactly one corresponding value of the wave function (Y).

  • What is the condition for the derivative of the wave function?

    -The derivative of the wave function must also be continuous and single-valued, ensuring smooth transitions and no discontinuities in the rate of change of the wave function's values.

  • How do you perform normalization of the wave function?

    -Normalization is performed by taking the integral of the probability density function over all space and setting it equal to one. This ensures that the total probability sums up to 1, which is the requirement for a valid probability distribution.

  • What is the implication of the wave function having a finite integral over all space?

    -A finite integral over all space indicates that the wave function is integrable and can be normalized. If the integral were infinite, the wave function could not represent a valid probability density function.

  • What happens when the total probability from all possible locations is greater than one?

    -When the total probability is greater than one, it indicates that the wave function has not been properly normalized. The wave function must be divided by this total probability to ensure that the sum of all probabilities equals one.

  • How does the normalization process affect the probability of finding a particle in a specific region?

    -Normalization ensures that the probability of finding a particle in a specific region is a small fraction of one. This means that the probability is expressed as a part of the total probability, allowing for accurate predictions of where a particle might be found.

  • Why is it important that the wave function adheres to certain conditions like continuity and single-valuedness?

    -These conditions are important because they ensure that the wave function can be properly normalized and that it provides a valid representation of the quantum state, which is necessary for making accurate predictions and interpretations in quantum mechanics.

  • What is the role of the probability density function in quantum mechanics?

    -The probability density function, derived from the wave function, is crucial in quantum mechanics as it provides the means to calculate the probability of finding a particle in a specific location, which is essential for understanding and predicting quantum phenomena.

Outlines
00:00
πŸ“ Understanding Wave Function Normalization

This paragraph delves into the concept of wave function normalization in quantum mechanics. It explains that the wave function itself lacks physical meaning, but when squared, it represents the probability of finding a particle at a specific location. The process of summing these probabilities across all possible locations should not exceed 100%, as probability cannot be greater than certain. To ensure this, the probabilities are normalized by dividing by the sum of probabilities, resulting in a total probability of one. The paragraph also touches on the conditions for wave functions, such as continuity, single-valuedness, and the requirement for the integral of the probability density function to be a finite constant to allow for normalization.

Mindmap
Keywords
πŸ’‘Normalization
Normalization is the process of adjusting a mathematical function so that its integral over a certain domain equals one. In the context of the video, it is essential for the wave function to be normalized to represent a valid probability density function. This ensures that the total probability of finding a particle across all possible locations sums up to 100%. For instance, the script mentions dividing the sum of probabilities by itself to achieve this normalization.
πŸ’‘Wave Function
A wave function is a mathematical description in quantum mechanics that represents the probability amplitude of a particle's location. It is a fundamental concept in the video, as it forms the basis for discussing the need for normalization. The wave function itself does not have a direct physical interpretation, but when squared, it gives the probability density.
πŸ’‘Probability Density
Probability density refers to the likelihood of finding a particle within a specific region of space. It is derived from the square of the wave function and is integral to the concept of normalization. The video emphasizes that the integral of the probability density function over all space must equal one for the wave function to be normalized properly.
πŸ’‘Particle
In the context of the video, a particle refers to a physical entity that is subject to the principles of quantum mechanics. The wave function is used to describe the probability of finding this particle at various locations in space, and normalization ensures that the total probability of finding the particle anywhere is 100%.
πŸ’‘Continuous Function
A continuous function is one where there are no breaks, jumps, or gaps in its graph. In quantum mechanics, the wave function must be continuous to ensure that the probability density function, derived from it, is well-defined and physically meaningful. The video highlights this as a necessary condition for the wave function.
πŸ’‘Single Valued
A single-valued function is one that assigns exactly one output value to each input value. In the context of the video, the wave function must be single-valued to ensure that for every position in space, there is a unique probability associated with finding the particle. This is crucial for the physical interpretation of the wave function.
πŸ’‘Derivative
In mathematics, a derivative represents the rate of change of a function with respect to its independent variable. For the wave function in quantum mechanics, the derivative must also be continuous and single-valued, ensuring that the rate of change of the probability distribution is well-defined and physically interpretable.
πŸ’‘Integral
The integral of a function over a certain domain represents the accumulation or summation of the function's values over that domain. In the context of the video, the integral of the probability density function over all space must equal one for the wave function to be normalized.
πŸ’‘Quantum Mechanics
Quantum mechanics is a fundamental theory in physics that describes the behavior of matter and energy at very small scales, such as atomic and subatomic particles. The wave function and the concept of normalization discussed in the video are key components of quantum mechanics, as they relate to the probabilistic nature of particles' locations.
πŸ’‘Physical Limits
Physical limits refer to the boundaries or constraints that are imposed by the laws of physics or the nature of the system being studied. In the context of the video, physical limits might define the range over which a particle can exist, affecting how the wave function is integrated over space.
πŸ’‘Finite Constant
A finite constant is a value that does not change and is not infinite in magnitude. In the video, the requirement for the integral of the probability density function to be a finite constant ensures that the wave function can be normalized, meaning the total probability across all space is a fixed, non-infinite number.
Highlights

The importance of normalizing the wave equation is discussed.

The wave function itself has no physical meaning.

Squaring the wave function represents the probability of finding a particle at a specific location.

The total probability of finding a particle across all locations should never exceed one.

Normalization is the process of dividing the probability sum by itself to equal one.

The probability density function is integrated over all space to set it equal to one.

Physical limits may restrict integration to a specific dimension rather than infinity.

The wave function must be continuous and single-valued.

The derivative of the wave function must also be continuous and single-valued.

Normalization is a crucial aspect of the probability density function.

The integral of the probability density function over all space must be a finite constant.

Normalization ensures a valid probability density function for representing the probability of finding a particle in space.

The concept of normalization ensures that the probability of finding a particle is a fraction of one.

The process of normalization involves integrating the probability density function and setting it to one.

The wave function's normalization ensures that the sum of probabilities across all possible locations equals one.

The wave function's properties, including continuity and single-valued nature, are essential for normalization.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (10 of 92) What is Normalization? Ex. 1

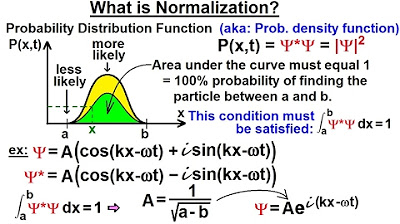
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (11 of 92) What is Normalization? Ex. 2

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (14 of 92) Time & Position Dependencies 3/3

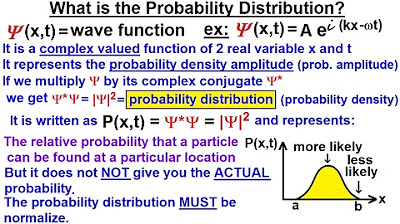
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (9 of 92) What is the Prob Distribution?

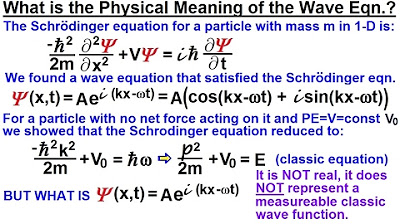
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (8 of 92) Meaning of the Wave Eqn.

21. Quantum Mechanics III

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsWaveEquationsNormalizationProcessProbabilityDensityPhysicsLecturesMathematicalConceptsParticleLocalizationContinuousFunctionsEducationalContentScientificPrinciples