Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (20 of 92) Particle in 1-D Box: Example 2/2
TLDRIn this video, we delve into the quantum mechanics of a particle in a one-dimensional box, exploring the wave equation and probability function for various energy levels. By applying the Schrödinger equation, we determine the wave number and normalize the wave function to find the constant 'a'. The resulting wave equation is then used to calculate the probability function, which varies with energy levels and is integral to understanding the particle's distribution within the box. The video concludes with a clear explanation of how to find the probability of finding the particle within a specific range.
Takeaways
- 🌊 The wave equation for a particle in a one-dimensional box is derived from the Schrödinger equation, specifically the time-independent version.
- 📈 The wave number square (k^2) is defined as twice the mass times the kinetic energy divided by ħ^2, which is also equal to n^2π^2/L^2, where n is the quantum number and L is the box's length.
- 🚫 The wave function cannot be expressed as eight times the cosine of (kx) because it does not satisfy the boundary conditions.
- 🔄 The valid wave function is a sine function with k = nπ/L, which meets the boundary conditions of being zero at x=0 and x=L.
- 🌀 The general wave equation is a times sine(kx), and the boundary conditions lead to the normalization of the wave function.
- 📐 The normalization process involves integrating the product of the wave function and its complex conjugate over the space (from 0 to L), which must equal one.
- 🤹 The constant 'a' is determined to be the square root of 2 divided by L, ensuring the integral of the probability function equals one.
- 📊 The probability function is the square of the wave function, which for a particle in a one-dimensional box is 2/L times the sine squared of (nπx/L).
- 🔢 The probability of finding the particle at a specific energy level depends on the quantum number n.
- 🌟 For different energy levels, there are different probability functions, which describe the likelihood of finding the particle within the box.
- 🛠️ The probability of finding the particle between two points a and b is calculated by integrating the probability function from a to b.
Q & A
What is the main topic of the video?
-The main topic of the video is determining the wave equation and the probability function of a particle in a one-dimensional box for various energy levels.
How is the wave number square defined in the context of the problem?
-The wave number square is defined as twice the mass of the particle times the kinetic energy, divided by H bar squared.
What is the relationship between the wave number and the quantum number n?
-The wave number is equal to n times PI over L, where n is the quantum number describing the energy level.
Why is the potential inside the box considered to be zero?
-The potential inside the box is considered to be zero because the box represents a region without any external forces or potential energy barriers acting on the particle.
What is the significance of the boundary conditions in this problem?
-Boundary conditions are crucial for determining the valid wave functions that satisfy the physical constraints of the system, ensuring that the wave function is zero at the boundaries of the box (x=0 and x=L).
How is the wave function normalized?
-The wave function is normalized by integrating the product of the complex conjugate of the wave function and the wave function over all space (between 0 and L) and ensuring that the integral equals one.
What is the final form of the wave equation for a particle in a one-dimensional box?
-The final form of the wave equation is a sine function with the argument n PI x over L, where n is the quantum number and L is the length of the box.
How does the probability function depend on the energy level?
-The probability function depends on the energy level through the quantum number n, which affects the shape of the wave function and thus the distribution of the probability density across the box.
What is the process for calculating the probability of finding the particle between two points a and b?
-To calculate the probability of finding the particle between points a and b, one takes the integral of the probability function from a to b, which involves integrating 2 over L times the sine squared of the angle from a to b.
What is the significance of the constant 'a' in the wave equation?
-The constant 'a' is determined by the normalization condition and is equal to the square root of 2 divided by L. It ensures that the wave function represents a valid quantum state with a probability amplitude that integrates to one over the entire box.
Outlines
📝 Quantum Mechanics: Wave Equation and Probability Function
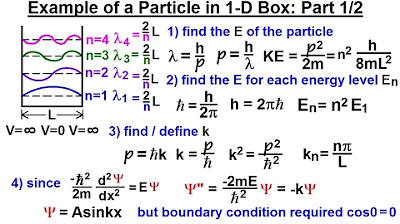
This paragraph delves into the quantum mechanics of a particle within a one-dimensional box, focusing on the derivation of the wave equation and the probability function for different energy levels. It begins by revisiting the previous video's conclusion that the square of the wave number is defined by the equation (2mKE)/ħ² = n²π²/L², where m is the mass of the particle, KE is the kinetic energy, ħ is the reduced Planck's constant, n is the quantum number, and L is the length of the box. The wave function is then derived as a sine function, satisfying the boundary conditions and the Schrödinger equation without a potential term, since the potential inside the box is zero. The normalization of the wave function is also discussed, leading to the determination of the constant 'a' as √(2/L), and the final wave equation is presented. The probability function is introduced as the product of the wave function and its complex conjugate, simplified to sine squared due to the absence of an imaginary term, and is used to calculate the likelihood of finding the particle within a specific range on the x-axis.
🧠 Probability Calculation for Particle Position
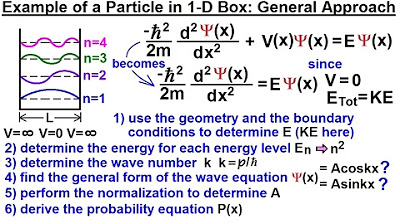
The second paragraph continues the discussion on quantum mechanics, specifically focusing on how to calculate the probability of finding a particle within a certain range of positions. It explains that the probability can be found by integrating the probability function from point A to point B. The integration process is outlined, with the example of finding the particle between two points a and b, using the integral of (2/L) * sin²(πx/L) from A to B. The paragraph emphasizes the dependency of the probability function on the energy level and concludes by summarizing the six steps required to utilize the Schrödinger equation and boundary conditions to derive the wave equation and probability function for a single particle in a box.
Mindmap
Keywords
💡Wave Equation
💡Probability Function
💡Quantum Number (n)
💡Boundary Conditions
💡Normalization
💡Schrödinger Equation
💡One-Dimensional Box
💡Potential Energy
💡Wave Number (K)
💡Sine Function
💡Cosine Function
Highlights
The wave equation for a particle in a one-dimensional box is derived using the Schrödinger equation.
The wave number square is defined as twice the mass of the particle times the kinetic energy divided by H bar squared.
The wave number square is also equal to n square PI square over L square, where L is the length of the box and n is the quantum number.
The general wave equation is solved using boundary conditions, showing that the cosine function solution is not possible.
The sine function solution meets the boundary conditions and is valid for a particle in a box.
The wave equation is normalized to find the constant in front of the sine function.
The integral over all space equals one, which is a requirement for the normalization of the wave function.
The constant a is determined to be the square root of 2 divided by L.
The final wave equation for a particle in a one-dimensional box is given, with n representing the quantum number and L the box length.
The probability function is defined as the product of the wave function and its complex conjugate.
The probability function depends on the energy level, and each level has a different function.
The probability of finding the particle between points a and b is calculated using an integral of the probability function over that range.
The process completes the six steps of utilizing the Schrödinger equation to calculate the wave and probability functions for a single particle in a box.
The potential inside the box is considered to be zero, simplifying the time-independent Schrödinger equation.
The sine function solution, sine of n PI x over L, is used to describe the particle's wave behavior within the box.
The normalization process leads to the integral of the sine squared function, which simplifies to the requirement that L divided by 2n pi equals one.
The probability function, 2 over L times sine squared, gives the likelihood of finding the particle at any point within the box.
The video provides a step-by-step guide on solving quantum mechanics problems involving particles in a one-dimensional box.
Transcripts
Browse More Related Video
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (19 of 92) Particle in 1-D Box: Example 1/2
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (18 of 92) Particle in 1-D Box: Gen. Appr.

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (10 of 92) What is Normalization? Ex. 1

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (25 of 92) Prob. of a Particle 1-D Box n=1

22. Quantum mechanics IV: Measurement theory, states of definite energy

23. Quantum Mechanics V: Particle in a Box
5.0 / 5 (0 votes)
Thanks for rating: