Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (11 of 92) What is Normalization? Ex. 2
TLDRThe video script delves into the concept of normalizing a probability distribution function, crucial for determining the exact probability of finding a particle at a specific location. It explains the process of converting the wave function and its complex conjugate into a sum of sines and cosines, and how to normalize it to ensure the integral over all possible locations equals 1. The script provides a hypothetical example to illustrate the normalization process, emphasizing that while the example is not directly applicable, it serves to demonstrate the general approach to finding the exact probability distribution in quantum mechanics.
Takeaways
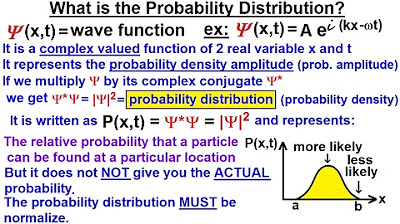
- 📈 The script discusses the concept of normalizing a function, specifically the probability distribution function denoted by P(X).
- 🌐 In the one-dimensional case, the probability distribution is the product of the wave function and its complex conjugate.
- 📊 Graphing the function yields a relative distribution where the height of the curve indicates the likelihood of finding a particle at a specific location.
- 🎯 The exact probability is not given by the relative distribution; instead, it requires normalization to find specific probabilities like 5% or 10%.
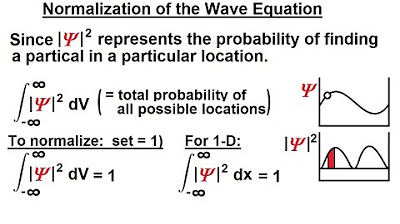
- 🔄 Normalization ensures that the area under the curve equals 1, representing a 100% probability of finding the particle within a given range.
- 🌀 The script introduces an alternative method to express the wave function as a sum of sines and cosines, which simplifies the normalization process.
- 📌 The complex conjugate of the wave function is derived and used to find the product that will be integrated over all possible particle locations.
- 🧮 The integral of the product of the wave function and its complex conjugate from location A to B must equal 1 for the function to be normalized.
- 🔢 The constant 'a' in the wave function is determined by the normalization condition, leading to a specific formula for the normalized function.
- 💡 The normalized function provides the exact probability of finding a particle at a particular location, which is crucial for quantum mechanics.
- 🚫 The speaker clarifies that the example given is hypothetical and does not directly relate to the specific equation discussed, serving only as an illustrative model.
Q & A
Why is it necessary to normalize a function in quantum mechanics?
-Normalization is necessary in quantum mechanics to ensure that the probability distribution function sums up to 1, representing a 100% chance of finding the particle within a given range. This process allows for the calculation of exact probabilities, such as 5% or 10%, rather than just a relative distribution.
What does the unnormalized probability distribution function represent?
-The unnormalized probability distribution function, represented by the product of the wave function and its complex conjugate, gives a relative distribution of where a particle is likely to be found. A higher curve indicates a higher likelihood, while a lower curve indicates a lower likelihood, but it does not provide the exact probability values.
How is the probability distribution function normalized?
-To normalize the probability distribution function, one must find a constant that, when multiplied by the unnormalized wave function, results in the integral of the product of the wave function and its complex conjugate over all possible locations equaling 1. This ensures that the area under the curve of the normalized function is 100%.
What is the significance of the integral of the normalized probability distribution function equaling 1?
-The integral equaling 1 signifies that there is a 100% probability of finding the particle within the range of all possible locations, which is a requirement for a valid probability distribution in quantum mechanics.
How does the script transition from the complex wave function to a sum of sines and cosines?
-The script rewrites the wave function as a sum of sines and cosines by expressing it in terms of a times the cosine of KX minus Omega T plus I times the sine of KX, and then finding its complex conjugate. When these are multiplied together, the middle terms cancel out, leading to an expression that can be written in terms of sine and cosine squared, which always equals 1.
What is the role of the complex conjugate in the normalization process?
-The complex conjugate is used to ensure that the product of the wave function and its complex conjugate results in a real-valued function, which is necessary for the normalization process. It helps in obtaining the correct form of the probability distribution function.
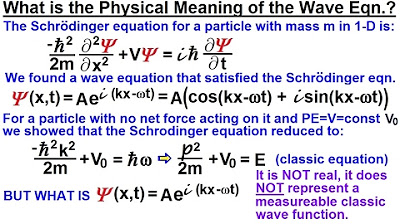
What is the final form of the normalized function for a single particle in a constant potential?
-The normalized function for a single particle in a constant potential is given by 1 over the square root of B minus A times e to the I times KX minus Omega T. This function allows for the calculation of the exact probability of finding the particle at a particular location.
How does the value of 'a' change after normalization?
-After normalization, the value of 'a' becomes 1 over the square root of B minus A. This new value ensures that the integral of the squared normalized wave function from A to B equals 1, satisfying the normalization condition.
What is the significance of the product of the wave function and its complex conjugate?
-The product of the wave function and its complex conjugate is significant because it represents the probability density of finding the particle at a particular location. This product is what needs to be integrated and normalized to obtain the probability distribution function.
Why is it important to distinguish between the unnormalized and normalized functions?
-It is important to distinguish between the unnormalized and normalized functions because the unnormalized function only provides a relative likelihood, while the normalized function provides the exact probabilities needed for making precise predictions in quantum mechanics.
How does the normalization process relate to the interpretation of quantum states?
-The normalization process is crucial for the interpretation of quantum states because it ensures that the probabilities derived from the wave function are physically meaningful and consistent with the principles of probability theory. Without normalization, the probabilities would not sum up to 1, which is required for a valid probability distribution.
Outlines
📚 Understanding Probability Distribution and Normalization
This paragraph introduces the concept of normalizing a probability distribution function, denoted as P(X), to ensure the area under the curve equals 1, representing a 100% probability of finding a particle within a given range. It explains the process of normalizing the product of the wave function and its complex conjugate to find the exact probability of locating a particle at a specific position. The paragraph then transitions into discussing a method of rewriting the function as a sum of sines and cosines, leading to the derivation of the normalized wave function for a particle in a constant potential with no forces acting on it.
🧮 Deriving the Normalized Function and Its Implications
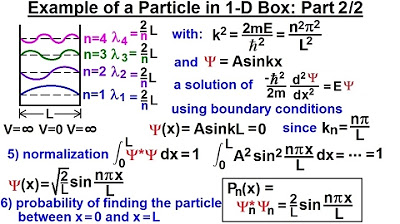
The second paragraph delves into the mathematical process of normalizing the wave function. It describes how to find the constant 'a' by integrating the squared wave function over a given range and setting the integral equal to 1. The resulting normalized function is then provided, which allows for the calculation of the exact probability of finding a particle at a specific location. The paragraph concludes with a cautionary note that the derived function is a hypothetical model and may not directly apply to specific examples, but serves to illustrate the general approach to working with realistic functions in quantum mechanics.
Mindmap
Keywords
💡normalize
💡probability distribution function
💡wave function
💡complex conjugate
💡integral
💡sines and cosines
💡KX and Omega T
💡constant potential
💡exact probability
💡hypothetical model
Highlights
The importance of normalizing a function, particularly in the context of probability distribution functions.
The one-dimensional case of the probability distribution function is the product of the wave function and its complex conjugate.
Graphing the probability distribution function results in a relative distribution, indicating likelihood of finding a particle at a particular location.
Normalization is required to find the exact probability, such as 5% or 10%, rather than just a relative likelihood.
The integral of the product of the wave function and its complex conjugate over all locations must equal 1 for proper normalization.
The method of rewriting the wave function as a sum of sines and cosines is introduced as a different approach to normalization.
The complex conjugate of the wave function is derived and its relationship with the original wave function is explained.
The product of the wave function and its complex conjugate results in a term involving sine and cosine functions.
The middle term in the product cancels out, leaving a final term that is key to normalization.
The realization that the cosine square plus the sine square of any angle equals one simplifies the normalization process.
The integral from A to B of the normalized wave function squared equals 1, which is a fundamental condition for normalization.
The constant 'a' is determined to be 1 over the square root of B minus A, which is crucial for the normalized function.
The normalized function for a single particle with no forces acting on it in a constant potential is derived.
The normalized function allows for the exact calculation of the probability of finding a particle at a specific location.
A warning is given that the example provided is hypothetical and does not directly relate to the specific equation discussed.
The significance of understanding the normalization process is emphasized for working with more realistic functions.
Transcripts
Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (10 of 92) What is Normalization? Ex. 1
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (9 of 92) What is the Prob Distribution?
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (2 of 92) Normalization of the Wave Equation
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (8 of 92) Meaning of the Wave Eqn.

What is the Heisenberg Uncertainty Principle? A wave packet approach
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (20 of 92) Particle in 1-D Box: Example 2/2
5.0 / 5 (0 votes)
Thanks for rating: