Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (18 of 92) Particle in 1-D Box: Gen. Appr.

Michel van Biezen
14 Feb 201704:48
EducationalLearning
32 Likes 10 Comments

TLDRThis script introduces the concept of calculating the wave equation for a particle in a one-dimensional box with infinite potential wells. It emphasizes the importance of using the box's geometry and boundary conditions to determine the particle's kinetic energy and the quantized energy states. The video outlines the steps to find the wave number, the general form of the wave equation, and ultimately the probability function that describes the likelihood of finding the particle at any given point within the box. The process is methodical, leading to a deeper understanding of quantum mechanics and particle behavior in defined physical scenarios.

Takeaways
  • 📌 The one-dimensional infinite potential well is used as an example to illustrate the calculation of the wave equation for a particle.
  • 🔄 The particle's total energy in the box equals its kinetic energy, as there is no potential energy within the well.
  • 🌐 The Schrödinger equation simplifies to a second-order differential equation for a particle in an infinite potential well.
  • 🏁 Boundary conditions, such as the box's length and the requirement that the particle cannot exceed the boundary, are crucial in determining the kinetic energy.
  • 🔢 Energy levels in the box are quantized, with each level's energy being proportional to n^2, where n is a positive integer.
  • 🌊 The wave number (K) for the particle is derived from the concept that for a photon, K equals momentum divided by h-bar.
  • 📈 The general form of the wave equation for a particle in a one-dimensional box is either a sine or cosine function of KX.
  • ✅ The boundary conditions will determine which form of the general wave equation (sine or cosine) is applicable.
  • 📊 Normalization of the wave equation is necessary to derive the constant factor in the equation.
  • 🎯 The probability equation, derived from the normalized wave equation, describes the likelihood of finding the particle at a specific location.
  • 🚀 Understanding the methodical approach to these calculations makes it easier to apply the process to various physical situations.
Q & A

  • What physical model is used as an example in the script?

    -The script uses the one-dimensional infinite potential well as an example to demonstrate how to calculate the wave equation for a particle in such a physical situation.

  • What is the significance of the potential well in the example?

    -The potential well has infinite potential on both sides and zero potential in the center, which simplifies the Schrödinger equation to a form that can be solved to find the wave equation for a particle within the well.

  • How does the potential well affect the total energy of the particle?

    -In the potential well, the total energy of the particle is equal to its kinetic energy since there is no potential energy within the well.

  • What are the steps to find the wave equation for a particle in a physical situation?

    -The steps include using the geometry and boundary conditions of the situation, determining the kinetic energy that meets those conditions, calculating the energy for each quantized energy level, determining the wave number, finding the general form of the wave equation, and using the normalization process to derive the probability equation.

  • What is the relationship between the energy levels in the one-dimensional box model?

    -The energy at each level depends on n squared, where n is a quantized number, and each successive energy level is a multiple of the previous level (e.g., the second energy level is 4 times the first, the third is 9 times, and so on).

  • How is the wave number (K) determined for a photon?

    -For a photon, the wave number K is equal to the momentum divided by h-bar (the reduced Planck constant).

  • What is the general form of the wave equation for a particle in a one-dimensional box?

    -The general form of the wave equation for a particle in a one-dimensional box is either a sine or cosine function of KX, where K is the wave number and X is the position.

  • How are the boundary conditions used in the calculation?

    -Boundary conditions are used to determine which form of the general wave equation (sine or cosine) is applicable and to find the constants in the equation through the normalization process.

  • What is the purpose of the normalization process in the context of the wave equation?

    -The normalization process is used to determine the constant in front of the equation, which is necessary to derive the probability function that describes the likelihood of finding the particle at a particular location.

  • What does the probability equation reveal about the particle in the one-dimensional box?

    -The probability equation reveals the probability of finding the particle at any point X between two specified locations within the one-dimensional box.

  • How does understanding the method make the process of finding the wave equation and probability function easier?

    -Understanding the method provides a clear and specific set of steps to follow, making it easier to apply the process to various physical situations and to discover how to use the Schrödinger equation to find the wave equation and probability function for a particle in a specific physical situation.

Outlines
00:00
📝 Introduction to Electron in a Box

This paragraph introduces the concept of an electron in an infinite potential well, also known as a one-dimensional box. It explains the simplification of the Schrödinger equation for this scenario, where the potential is zero within the box and infinite outside. The total energy of the particle is equal to its kinetic energy, as there is no potential energy. The paragraph outlines the procedure for finding the wave equation and the probability of finding a particle in such a physical situation, emphasizing the importance of using the geometry of the situation and boundary conditions.

Mindmap
Keywords
💡Electron Line
The term 'Electron Line' seems to be the name of the series or the course from which this transcript is taken. It is likely a program or a set of lessons dedicated to explaining concepts related to electrons and their behavior in various physical scenarios.
💡Infinite Potential Well
An infinite potential well, also known as an infinite square well, is a theoretical model in quantum mechanics where a particle is confined within a region of space where the potential energy is zero, surrounded by a region of infinite potential energy that the particle cannot cross.
💡Wave Equation
The wave equation is a fundamental equation in quantum mechanics that describes how the probability amplitude of a quantum state (or wave function) evolves over time and space. It is used to determine the quantum states of particles, such as electrons in atoms or molecules.
💡Schrödinger Equation
The Schrödinger equation is a partial differential equation that describes how the quantum state of a physical system changes with time. It is named after Erwin Schrödinger and is fundamental to quantum mechanics, forming the basis for describing the behavior of quantum systems.
💡Quantum States
Quantum states are the various conditions that a quantum system can be in, each characterized by a distinct wave function. These states are discrete and quantized, meaning they can only take on certain specific values, which is a key concept in quantum mechanics.
💡Boundary Conditions
Boundary conditions are specifications of a function's values at the edges of its domain, or the limits of the problem space. In the context of quantum mechanics, they are essential for solving differential equations like the Schrödinger equation, as they define the behavior of the wave function at the limits of the potential well.
💡Kinetic Energy
Kinetic energy is the energy that a body possesses due to its motion. In classical mechanics, it is given by the equation KE = 1/2 mv^2, where m is the mass of the object and v is its velocity. In quantum mechanics, the concept is related to the energy associated with the particle's wave function.
💡Energy Levels
Energy levels in quantum mechanics refer to the quantized values that the energy of a quantum system can take. These levels are discrete and correspond to the different possible states of the system. The energy of each level is determined by the system's wave function and the boundary conditions.
💡Wave Number (K)
The wave number, often denoted by K, is a measure of the spatial frequency of a wave. In quantum mechanics, it is related to the momentum of a particle and is used to describe the behavior of wave functions in different physical situations.
💡Normalization
Normalization in the context of quantum mechanics refers to the process of adjusting the wave function so that its integral over all space equals 1. This ensures that the total probability of finding the particle anywhere in the possible space is 100%, which is a fundamental requirement in probability theory.
💡Probability Distribution
The probability distribution in quantum mechanics describes the likelihood of finding a particle in a particular state or at a specific location. It is derived from the square of the wave function and gives the probability density as a function of position or other variables.
Highlights

Introduction to the one-dimensional box model for illustrating wave equation calculations.

Explaining the infinite potential well with zero potential in the center as a starting example.

The serving equation simplifies to a form where the total energy equals kinetic energy due to zero potential energy.

Procedure for finding the wave equation and the probability of finding a particle in a physical situation.

Use of the box's geometry and boundary conditions to determine kinetic energy.

Quantized energy states and the particle's inability to exceed the box's boundaries.

Energy level quantization and its dependence on n squared, with higher levels being multiples of the first level's energy.

Determination of the wave number K, starting with the concept of K for a photon being momentum divided by h-bar.

Finding the general form of the wave equation that fits the conditions of the situation.

The wave equation likely taking the form of a sine or cosine function of KX.

Selection of the correct wave equation form based on boundary conditions.

Normalization process to determine the constant in the wave equation.

Derivation of the probability equation from the normalized wave equation.

The probability of finding a particle at any point X between two locations.

A specific set of steps to apply to every physical situation for finding wave equations and probability functions.

The methodical approach to using the Schrödinger equation to find wave equations and probability functions.

The importance of understanding the method to the madness when dealing with quantum mechanics problems.

Transcripts

Browse More Related Video

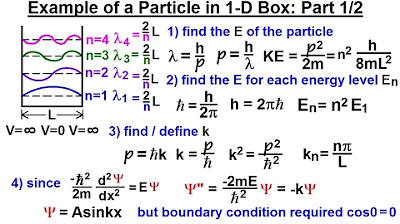
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (19 of 92) Particle in 1-D Box: Example 1/2

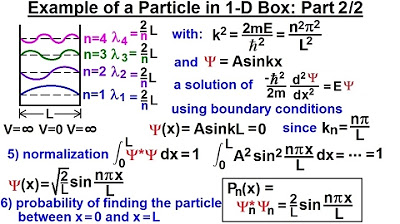
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (20 of 92) Particle in 1-D Box: Example 2/2

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (25 of 92) Prob. of a Particle 1-D Box n=1

EASIEST question on the International Physics Olympiad?

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (17 of 92) How to Use Schrod. Eqn: 2

22. Quantum mechanics IV: Measurement theory, states of definite energy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumPhysicsWaveEquationsParticleProbabilityOneDimensionalBoxKineticEnergyQuantizedStatesBoundaryConditionsMomentumPreservationProbabilityDistributionEducationalContent