Calculus Grade 12 Tangent
TLDRThis educational script explains how to find the equation of a line perpendicular to the tangent of a curve at a given point 'A'. It clarifies the concept with a graph, emphasizing the importance of the first derivative as the gradient of the curve and the tangent at point 'A'. The script guides through the process of calculating the gradient of the tangent, determining the perpendicular line's gradient by using the fact that the product of gradients of perpendicular lines is -1, and finally finding the equation of the perpendicular line using the point 'A' and the calculated gradient.
Takeaways
- 📝 The problem involves finding the equation of a line perpendicular to the tangent at a specific point on a curve.
- 📈 A tangent is a line that touches a curve at exactly one point.
- 🎯 The goal is to determine the equation of the perpendicular line, which forms a 90-degree angle with the tangent.
- 🧮 The gradient of the tangent is the same as the gradient of the curve at the point of tangency.
- 🔄 The gradient of two perpendicular lines multiplied together equals -1.
- 📐 The first derivative of the curve's equation represents the gradient of the tangent.
- ➕ To find the gradient of the perpendicular line, divide -1 by the gradient of the tangent.
- 🔍 The equation of the perpendicular line is in the form y = mx + c, where m is the gradient of the perpendicular line.
- 🖊️ To find the value of c, substitute the x and y coordinates of the point into the equation.
- ✅ The final equation of the perpendicular line is determined by combining the calculated gradient and the y-intercept.
Q & A
What is the main goal of the problem discussed in the script?
-The main goal is to determine the equation of a line that is perpendicular to the tangent of a curve at a given point A.
What is the significance of the tangent in this problem?
-The tangent is a line that touches the curve at exactly one point, and its gradient at that point is the same as the gradient of the curve at that point.
How is the gradient of the tangent related to the gradient of the curve?
-The gradient of the tangent is the same as the gradient of the curve at the point where the tangent touches the curve.
What is the relationship between the gradients of two perpendicular lines?
-If two lines are perpendicular, the product of their gradients is equal to -1.
How do you find the gradient of the curve at a specific point?
-The gradient of the curve at a specific point can be found by taking the first derivative of the curve's equation and substituting the x-value of that point into the derivative.
Why is it important to use correct notation when taking the derivative?
-Using the correct notation, such as dy/dx, is important to avoid losing marks in a test, as it correctly represents the derivative of a function when y is used instead of f(x).
What is the gradient of the tangent at point A in the problem?
-The gradient of the tangent at point A is found to be -2 after substituting the x-value of 2 into the first derivative of the curve's equation.
How do you determine the gradient of the line perpendicular to the tangent?
-The gradient of the line perpendicular to the tangent is determined by setting the product of the gradient of the tangent and the gradient of the perpendicular line equal to -1, resulting in a gradient of 1/2 for the perpendicular line.
What additional information is needed to find the equation of the perpendicular line?
-To find the equation of the perpendicular line, you need the gradient (which is 1/2) and a point on the line. The point can be found by substituting the x-value of 2 into the original curve equation to find the corresponding y-value, which is 8.
What is the final equation of the line that is perpendicular to the tangent at point A?
-The final equation of the line that is perpendicular to the tangent at point A is y = 1/2x + 7.
Outlines
📈 Understanding Perpendicular Lines on a Graph
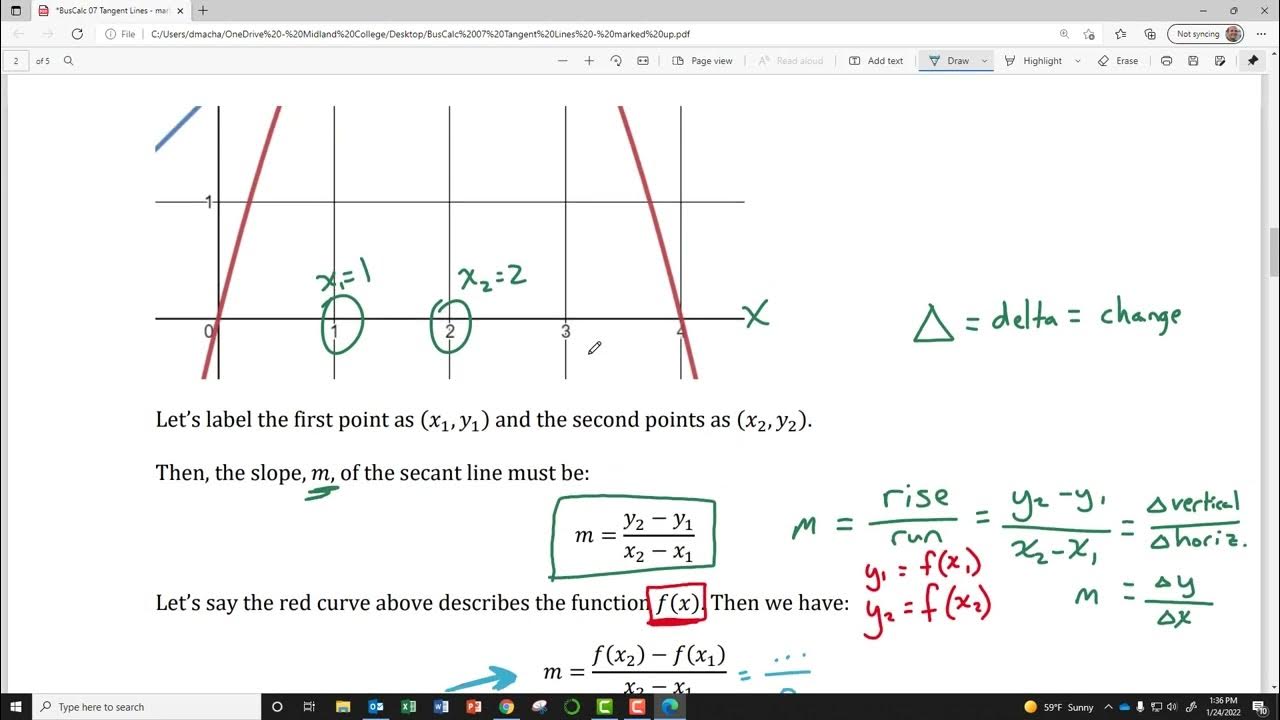
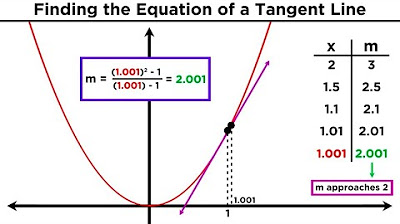
The script begins by introducing a mathematical problem: determining the equation of a line perpendicular to the tangent at a given point on a curve. The speaker acknowledges the complexity of the problem and simplifies it by drawing a graph to visually explain the concept. The explanation includes drawing the curve, identifying the point of tangency, and illustrating the tangent line. The goal is to find the equation of the line perpendicular to the tangent, which requires understanding the relationship between the gradients of the tangent and the curve at the point of interest.
🧮 Calculating the Gradient Using First Derivative
This section delves into the mathematical process of finding the gradient of the curve using calculus. The speaker explains the importance of correctly using notation when taking the first derivative, especially when working with different representations of the function (e.g., f(x) vs. y). The derivative is calculated to find the gradient of the curve at the specific point, which is identified as -2. This gradient is crucial as it equals the gradient of the tangent at that point, setting the stage for finding the perpendicular line's gradient.
🔄 Deriving the Equation of the Perpendicular Line
Building on the previous steps, the speaker explains how to find the gradient of the perpendicular line. By applying the mathematical rule that the product of the gradients of perpendicular lines equals -1, the gradient of the perpendicular line is found to be 1/2. The equation of the perpendicular line is then formed as y = 1/2x + c. To find the y-intercept (c), the speaker substitutes the known x and y values of the point into the equation, leading to the final equation: y = 1/2x + 7.
Mindmap
Keywords
💡Curve
💡Tangent
💡Perpendicular
💡Gradient
💡First Derivative
💡Calculus
💡Equation
💡Graph
💡Point 'a'
💡Perpendicular Line
Highlights
Introduction of the problem: Determining the equation of a line that is perpendicular to the tangent at a specific point on a curve.
Visualizing the problem: Drawing a graph and labeling the point A where the tangent touches the curve.
Explanation of a tangent: A tangent is a line that touches a curve at only one point.
Concept of perpendicular lines: Understanding that the line perpendicular to the tangent forms a 90-degree angle with the tangent.
Key point: The gradient of the tangent is the same as the gradient of the graph at the point of tangency.
Important concept: For two lines to be perpendicular, their gradients must multiply to give negative one.
Introduction of calculus: Using the first derivative to find the gradient of the graph.
Technical detail: The first derivative represents the gradient of the graph.
Procedure: Taking the first derivative of the equation and evaluating it at the point x = 2.
Calculation result: The gradient of the tangent (and the graph) at x = 2 is -2.
Finding the perpendicular gradient: Calculating that the gradient of the line perpendicular to the tangent is 1/2.
Setting up the equation: Formulating the equation of the perpendicular line as y = (1/2)x + c.
Finding the y-value: Plugging x = 2 into the original equation to find the y-value at point A.
Substituting to find c: Using the point (2, 8) to determine that c = 7.
Final answer: The equation of the line perpendicular to the tangent at point A is y = (1/2)x + 7.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: