Equation of a tangent line [IB Maths AI SL/HL]

OSC
26 Feb 202112:50
EducationalLearning
32 Likes 10 Comments

TLDRThe video script provides a detailed walkthrough on finding the equation of a tangent line to a given graph. The process begins by selecting a specific point on the graph and then determining the derivative of the function at that point, which gives the slope (m) of the tangent line. The next step involves identifying the x and y coordinates of the chosen point, which are then used to solve for the y-intercept (c) in the line equation y = mx + c. The script also demonstrates how to use a calculator to graph the function, find the derivative, and draw the tangent line. It concludes with a practical example, illustrating the calculation of the tangent line for y = x^2 at x = 2, and how to find the value of a constant (k) in a more complex function using the tangent line's gradient. The summary emphasizes the importance of understanding the derivative as a gradient and the significance of the chosen point for the tangent line's equation.

Takeaways
  • 📌 To find the equation of a tangent line, first identify a specific point on the curve where the tangent line is desired.
  • 🔍 The equation of a tangent line is in the form y = mx + c, where m is the slope (gradient) and c is the y-intercept.
  • 🧮 Calculate the derivative of the function (f'(x)) to find the slope of the tangent line at a given point.
  • 📐 The slope (m) of the tangent line at a specific point is found by evaluating the derivative at that point.
  • 📈 The value of the derivative at a point gives the gradient of the tangent line at that point.
  • 📍 Knowing the x and y coordinates of the point, along with the slope (m), allows you to determine the y-intercept (c) and thus the equation of the tangent line.
  • 🤔 The choice of the point where the tangent line is drawn is crucial, as it affects the slope and the equation of the tangent line.
  • 💡 If the question provides the equation of the tangent line and a point on the curve, you can work backwards to find unknown parameters of the curve.
  • 📊 Use a graphing calculator to graph the function, find the derivative, and draw the tangent line at a specific point if needed.
  • ✅ Confirm the correctness of your work by substituting the found values back into the original equation and checking the tangent line equation.
  • 📚 Understanding the concept of derivatives as gradients is key to solving problems involving tangent lines to curves.
  • 😅 The speaker uses humor to engage the audience, demonstrating that learning can be both informative and enjoyable.
Q & A

  • What is the general equation of a straight line?

    -The general equation of a straight line is y = mx + c, where m is the slope (gradient) and c is the y-intercept.

  • Why is it important to choose a specific point when finding the equation of a tangent line?

    -The equation of a tangent line changes depending on the point of tangency. The specific point determines the slope (gradient) and the y-intercept of the tangent line.

  • What is the first step in finding the equation of a tangent line?

    -The first step is to find the derivative of the function at the specific point of interest, which gives you the slope (m) of the tangent line at that point.

  • What is the derivative of y = x^2?

    -The derivative of y = x^2 with respect to x is f'(x) = 2x.

  • How do you find the y-intercept (c) of the tangent line once you have the slope (m) and the point of tangency (x, y)?

    -You can find the y-intercept (c) by plugging in the x and y values of the point of tangency into the equation y = mx + c and solving for c.

  • What is the equation of the tangent line to y = x^2 at the point where x = 2?

    -The equation of the tangent line at x = 2 is y = 4x - 4, since the slope (m) is 4 and the point of tangency is (2, 4).

  • How can you use a graphing calculator to find the equation of a tangent line?

    -You can graph the function, identify the point of tangency, use the calculator to find the derivative at that point to get the slope (m), and then use the geometry function to draw the tangent line and find its equation.

  • Why is it necessary to know both the x and y coordinates of the point of tangency when finding the equation of a tangent line?

    -Knowing both coordinates allows you to find the y-intercept (c) by substituting the values into the equation y = mx + c, which is essential for determining the complete equation of the tangent line.

  • How does the position of the point of tangency affect the equation of the tangent line?

    -The position of the point of tangency affects both the slope (m) and the y-intercept (c) of the tangent line, resulting in a different equation for each point on the curve.

  • What is the significance of the derivative in the context of finding the equation of a tangent line?

    -The derivative of a function at a specific point gives the slope (gradient) of the tangent line at that point, which is a crucial component of the tangent line's equation.

  • Can you find the equation of a tangent line without knowing the function's derivative?

    -It is more challenging to find the equation of a tangent line without knowing the derivative, as the derivative provides the slope at the point of tangency, which is essential for determining the tangent line's equation.

  • How does the process of finding the equation of a tangent line apply to a function like f(x) = kx^2 + x + 3, where k is an unknown constant?

    -You can use the given point on the curve and the known equation of the tangent line to find the value of k by equating the derivative of the function at the given point to the slope of the tangent line.

Outlines
00:00
📐 Understanding Tangent Lines and Derivatives

This paragraph introduces the concept of finding the equation of a tangent line on a graph. The importance of selecting a specific point on the graph to determine the tangent line is emphasized. The process involves finding the derivative at that point, which gives the gradient (m) of the tangent line. The equation of a straight line is then used (y = mx + c), where m is the gradient and c is the y-intercept. The coordinates of the chosen point are used to solve for c, thus finding the equation of the tangent line. An example is given using the function y = x^2 to illustrate the steps.

05:01
📱 Using a Calculator to Find Tangent Lines

The second paragraph demonstrates how to use a calculator, specifically a TI-84, to find the equation of a tangent line. It shows the process of graphing the function, identifying a specific point, and using the calculator's derivative function to find the gradient at that point. The calculator can then be used to draw the tangent line, and the equation of the tangent line is shown on the screen. The paragraph also discusses how the equation of the tangent line changes with the point of tangency and how to handle more complex functions where the equation might not be immediately apparent.

10:03
🔍 Solving for a Variable in a Tangent Line Equation

The final paragraph deals with a more complex scenario where the function is given as f(x) = kx^2 + x + 3, and a point P(1,5) is provided. It outlines how to find the value of k by using the known gradient of the tangent line at x = 1, which is given as 3. By equating this gradient with the derivative of the function, the value of k is solved to be 1. The paragraph concludes by verifying the solution by substituting k back into the function and checking the derivative at x = 1, confirming that the process was done correctly.

Mindmap
Keywords
💡Tangent Line
A tangent line is a straight line that touches a curve at a single point without crossing it. It is a fundamental concept in calculus and geometry. In the video, finding the equation of a tangent line is the main objective, which is used to understand the rate of change at a specific point on a curve. The script illustrates this by showing how to calculate the tangent line for a curve represented by the function y = x^2.
💡Derivative
The derivative of a function at a certain point gives the slope of the tangent line to the curve of the function at that point. It is a key concept in calculus that measures the rate at which a quantity changes with respect to another quantity. In the script, the derivative is calculated for the function y = x^2 to find the gradient (slope) of the tangent line at the point x = 2.
💡Gradient
Gradient refers to the slope of a line, which in the context of calculus, is synonymous with the derivative. It indicates the rate of change of a function at a given point. The script uses the term 'gradient' to describe the slope of the tangent line, which is found by evaluating the derivative of the function at a specific point.
💡Equation
An equation is a statement that expresses the equality of two mathematical expressions and can be used to describe a relationship between different quantities. In the video, the equation of a tangent line is sought, which is typically in the form y = mx + c, where m is the gradient and c is the y-intercept. The script demonstrates how to derive this equation using the function y = x^2.
💡Y-Intercept
The y-intercept is the point where a line crosses the y-axis and is represented by the 'c' in the equation y = mx + c. It is a key component in determining the full equation of a tangent line. In the script, the y-intercept is calculated by plugging the point and the gradient into the line equation to solve for 'c'.
💡X and Y Coordinates
Coordinates are pairs of numbers that define a point's location on a two-dimensional plane, with 'x' representing the horizontal axis and 'y' representing the vertical axis. In the context of the video, x and y coordinates are used to identify a specific point on the curve and to determine the equation of the tangent line at that point. The script emphasizes the importance of knowing the coordinates of the point where the tangent line is to be found.
💡Graph
A graph is a visual representation of data in which the relationship between two or more quantities is depicted. In the video, the graph of the function y = x^2 is used to visualize the curve and the tangent line. The script describes the process of graphing the function and then drawing the tangent line on the graph using a calculator.
💡Calculator
A calculator is an electronic device used for performing arithmetic operations and in this context, for graphing and finding derivatives. The script demonstrates the use of a calculator, specifically a TI-84, to graph the function, find the derivative, and draw the tangent line, illustrating an alternative method to manual calculations.
💡Quadratic Function
A quadratic function is a polynomial function of degree two, often represented by the standard form ax^2 + bx + c. In the video, y = x^2 is an example of a quadratic function, which is used to demonstrate the process of finding the equation of a tangent line. The script explains how to find the derivative of this function and use it to calculate the tangent line.
💡Rate of Change
The rate of change refers to how much one quantity changes relative to another quantity. In calculus, the rate of change is often discussed in terms of the instantaneous rate of change at a particular point, which is what the derivative represents. The video uses the concept of rate of change to explain why the derivative (gradient) is essential in finding the equation of the tangent line.
💡Point of Tangency
The point of tangency is the exact location on the curve where the tangent line touches. It is defined by specific x and y coordinates. In the script, the point of tangency is (2, 4) for the function y = x^2, and this point is crucial for determining the equation of the tangent line at that location.
Highlights

The process of finding the equation of a tangent line involves selecting a specific point on the graph and understanding that the tangent line's equation changes depending on this point.

The equation of a straight line is given by y = mx + c, where m is the gradient (slope) and c is the y-intercept.

To find the gradient (m) of the tangent line, one must first find the derivative of the function at the chosen point.

The derivative of a function at a specific point gives the gradient of the tangent line at that point.

Once the gradient (m) is known, the coordinates of the point (x, y) are used to solve for the y-intercept (c).

The importance of choosing the correct point on the graph for the tangent line calculation is emphasized, as it affects the resulting equation.

An example is given using the function y = x^2 to demonstrate the process of finding the equation of the tangent line at x = 2.

The derivative of y = x^2 is found to be f'(x) = 2x, which simplifies to f'(2) = 4 at the specific point x = 2.

The gradient (m) for the tangent line at x = 2 is determined to be 4, and the y-intercept (c) is calculated to be -4.

The final equation of the tangent line for y = x^2 at x = 2 is given as y = 4x - 4.

The use of a calculator, specifically the TI-84, is demonstrated to graph the function, find the derivative, and draw the tangent line.

The calculator automatically provides the equation of the tangent line when the specific point is selected.

The concept is further illustrated with a more complex function, f(x) = kx^2 + x + 3, where the value of k is unknown.

By knowing the equation of the tangent line and the point it passes through, the value of k can be solved for.

The derivative is used to find the value of k, which is determined to be 1 in the given example.

The process of verifying the solution by substituting the value of k back into the original function and checking the derivative at the specific point is explained.

The importance of understanding the relationship between the derivative, the gradient, and the equation of the tangent line is emphasized for solving calculus problems.

Transcripts

Browse More Related Video

Equation of a normal line [IB Maths AI SL/HL]

Equation of Tangent Calculus Grade 12

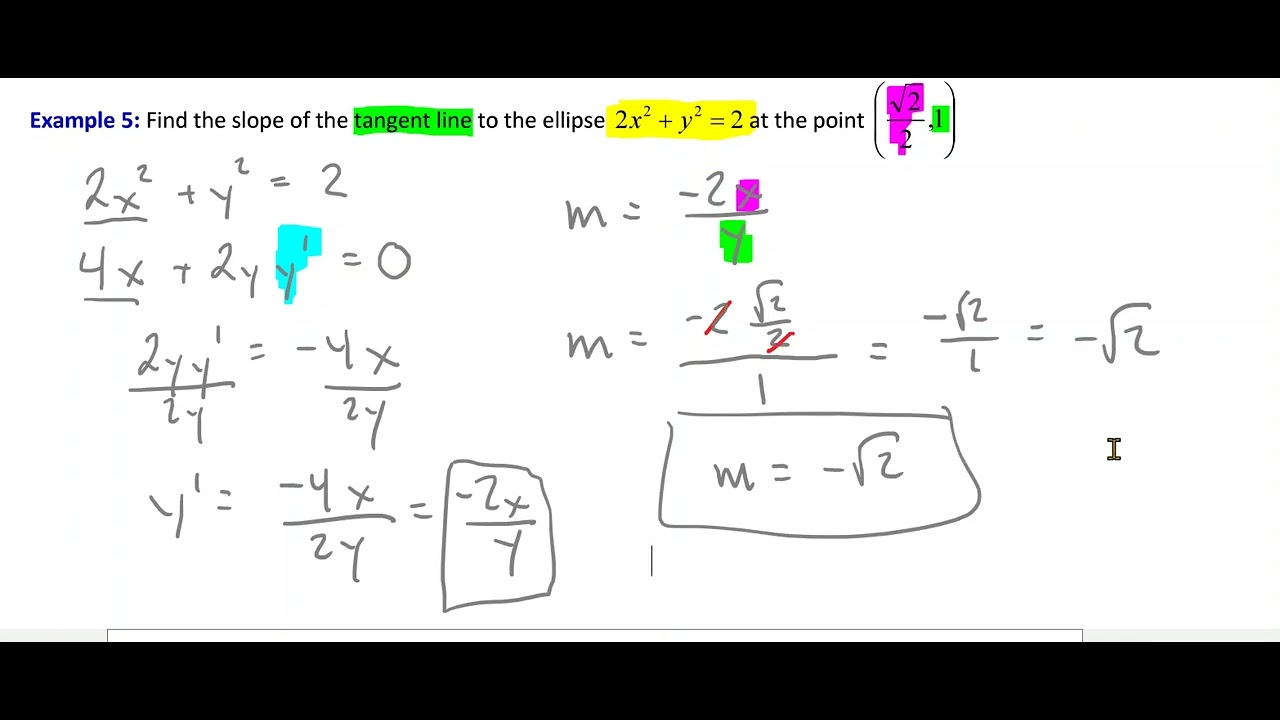
Implicit Differentiation - Finding Equation of Tangent Line

Tangent Line to a Polynomial | MIT 18.01SC Single Variable Calculus, Fall 2010

Calculus Grade 12: Tangent

2011 Calculus AB free response #3 (a & b) | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusTangent LineDerivativeGraphingEquationGeometryMathematicsEducationalProblem SolvingX-SquaredGradient