BusCalc 07 Tangent Lines

Drew Macha

24 Jan 202247:19

EducationalLearning

32 Likes 10 Comments

TLDRThis video script is an educational walkthrough on the concept of tangent lines, specifically in relation to circles and curves. The presenter explains that a tangent line intersects a circle at exactly one point and is perpendicular to the radius at that point. Using Desmos, a mathematical graphing tool, the script illustrates how tangent lines can be visualized for any point on a curve, with an emphasis on the uniqueness of the tangent line at any given point. The video also delves into the formal definition of a tangent line by contrasting it with a secant line, which passes through two points on a curve. The concept of a derivative is introduced as a tool to find the slope of the tangent line, with the derivative of a function at a point being the limit of the slope of the secant line as the two points defining it approach each other. The script provides detailed examples of finding the derivative and the equation of the tangent line for given functions, such as the square root and reciprocal functions. It concludes with a discussion on the existence of derivatives, noting that they do not always exist, especially at points where the function is undefined or appears 'pointy'. The presenter also demonstrates how to approximate derivatives numerically using small values for h, showcasing the process through an example in Microsoft Excel.

Takeaways

📐 A tangent line to a circle is a line that touches the circle at exactly one point and is perpendicular to the radius at the point of contact.
📈 The tangent line to a curve at any given point is unique and represents the limit of the secant line as the points defining the secant come closer together.
✏️ The slope of a secant line passing through two points (x1, y1) and (x2, y2) on a curve is given by (y2 - y1) / (x2 - x1).
📉 For a function f(x), the slope of the secant line through points x1 and x2 is the difference in function values at these points divided by the difference in x values, i.e., (f(x2) - f(x1)) / (x2 - x1).
🔍 The derivative of a function, denoted as f'(x), is the limit of the slope of the secant line as x2 approaches x1, which is also the slope of the tangent line at point x.
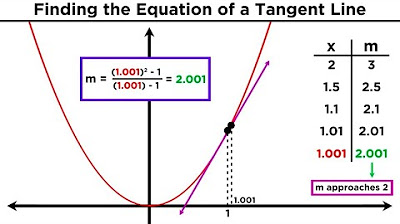
🧮 The derivative can be approximated numerically by choosing a very small value for h in the limit expression, which is useful when an exact derivative is difficult to find.
🚫 Derivatives do not always exist, such as at points where the function is undefined or where the function's graph has a sharp point (like the absolute value function at x = 0).
🔑 The point-slope form of a line is y - y1 = m(x - x1), where m is the slope and (x1, y1) is a point on the line.
📉 The derivative of a function at a specific point gives the slope of the tangent line at that point, which can be used with the point-slope form to find the equation of the tangent line.
🤔 Understanding the concept of limits is crucial for grasping the definition of a derivative and how it relates to the slope of the tangent line.
📊 Desmos is a powerful tool for visualizing tangent lines and understanding the behavior of functions and their derivatives through animations and graphical representations.

Q & A

What is a tangent line to a circle?
-A tangent line to a circle is a line that intersects the circle at exactly one point and is perpendicular to the radius at the point of intersection.
How does a tangent line relate to a curve at a given point?
-At any given point on a curve, there is exactly one tangent line. This line intersects the curve at that point only once and can be thought of as touching the curve without crossing it.
What is a secant line?
-A secant line is a line that passes through any two points along a curve. It intersects the curve at those two points and is used to help understand the concept of a tangent line.
How is the slope of a secant line calculated?
-The slope of a secant line (denoted as 'm') is calculated using the formula (y2 - y1) / (x2 - x1), where (x1, y1) and (x2, y2) are the coordinates of the two points the secant line passes through.
What is the connection between the slope of a secant line and the derivative of a function?
-The slope of a secant line is an approximation of the derivative of a function at a point. As the points defining the secant line get closer together, the slope of the secant line approaches the value of the derivative at that point.
How do you find the formula for the tangent line to a function at a specific point?
-To find the formula for the tangent line, first calculate the derivative of the function to find the slope of the tangent line at the specific point. Then use the point-slope form of a line, y - y1 = m(x - x1), where m is the slope and (x1, y1) is the point of tangency.
What is the significance of the derivative in calculus?
-The derivative in calculus is a measure of the rate at which a function is changing at a certain point. It is equivalent to the slope of the tangent line to the curve of the function at that point and is a fundamental concept in understanding the behavior of functions.
Why might a derivative not exist at a certain point on a function?
-A derivative might not exist at a certain point if the function is not defined at that point, if the function has a sharp corner or cusp at that point, or if the limit used to define the derivative does not converge to a single value.
How can derivatives be approximated numerically?
-Derivatives can be approximated numerically by using a small non-zero value for 'h' in the limit definition and calculating the difference quotient. As 'h' gets smaller, the approximation gets closer to the actual derivative.
What is the geometric interpretation of the derivative of a function at a point?
-The geometric interpretation of the derivative of a function at a point is the slope of the tangent line to the graph of the function at that point. It represents the instantaneous rate of change of the function at that point.
What is the role of the Greek letter delta (Δ) in the context of derivatives and limits?
-The Greek letter delta (Δ) represents a change in a quantity. In the context of derivatives and limits, Δy represents a change in the vertical direction (rise), and Δx represents a change in the horizontal direction (run). The ratio Δy/Δx gives the slope of a line, which is a key concept in finding derivatives.

Outlines

00:00

📐 Introduction to Tangent Lines and Circles

The video begins with an introduction to tangent lines in the context of circles. A tangent line is defined as a line intersecting the circle at exactly one point and is perpendicular to the radius at the point of intersection. The presenter uses a visual representation to illustrate this concept, highlighting the unique property of a tangent line to touch the circle at a single point without crossing it. The video also mentions the use of Desmos, a mathematical graphing tool, to demonstrate the concept further.

05:01

📈 Understanding Tangent Lines on Curves

Moving beyond circles, the video explains that a tangent line to any curve is a line that intersects the curve at exactly one point. The presenter clarifies that while there may be many tangents to a curve, there is only one unique tangent at any given point on the curve. An animation is suggested as a means to better visualize the concept of a tangent line. The video also introduces the concept of secant lines, which are lines passing through two points on a curve, and discusses the slope of secant lines as a precursor to understanding tangent lines.

10:03

🔍 The Limit of Secant Lines Defines Tangent Lines

The video delves into the mathematical definition of a tangent line by considering the limit of secant lines as the points defining the secant line get closer together. The presenter uses the concept of limits and introduces the notation for the difference in input values (h) to define the slope of the secant line. It is shown that as the points coincide, the secant line's slope approaches the slope of the tangent line, providing a rigorous definition of a tangent line.

15:05

🧮 Derivatives and the Slope of Tangent Lines

The concept of the derivative is introduced as a tool to find the slope of the tangent line to a curve at a given point. The presenter explains that the derivative of a function at a point is the same as the slope of the tangent line to the graph of the function at that point. The video demonstrates how to calculate the derivative of a function, using the limit definition and providing a worked example involving the square root function.

20:07

🔢 Calculating the Tangent Line for a Specific Function

The video provides a step-by-step example of finding the formula for the tangent line to a specific function (the square root function) at a given point (x = 4). It involves calculating the derivative of the function, evaluating it at the point of interest, and then using the point-slope form of a line to find the equation of the tangent line. The presenter also uses Desmos to verify the result visually.

25:08

📉 The Tangent Line to the Reciprocal Function

The presenter continues with another example, this time finding the tangent line to the reciprocal function (1/x) at the point x = 3. The derivative of the reciprocal function is calculated, and the slope of the tangent line at the specified point is determined. Using the point-slope form, the equation of the tangent line is found and then verified using Desmos.

30:10

🚫 Instances Where Derivatives Do Not Exist

The video highlights that derivatives do not always exist, particularly at points where the function is undefined or where the function's graph has a sharp point (like the absolute value function at x = 0). The presenter demonstrates this using the absolute value function and explains that the limit defining the derivative does not exist in such cases, resulting in an undefined derivative.

35:12

📊 Approximating Derivatives Numerically

The final part of the video discusses the numerical approximation of derivatives. An example is given where the derivative of a function is approximated at a specific point (x = 5) by using a small value for h in the difference quotient. The presenter uses Microsoft Excel to demonstrate how decreasing the value of h increases the precision of the approximation, providing a practical method for estimating derivatives when exact solutions are difficult to obtain.

Mindmap

Keywords

💡Tangent Line

A tangent line to a curve at a given point is the straight line that just touches the curve at that point. In the context of the video, tangent lines are described in relation to circles and other curves. For circles, the tangent is perpendicular to the radius at the point of contact, illustrating the geometric relationship between the line and the shape. The video extends this concept to other curves, highlighting that at any point on a curve, there can be exactly one tangent line, which helps in understanding the slope and direction of the curve at that point.

💡Secant Line

A secant line intersects a curve at two distinct points, providing a way to approximate the slope of a tangent line. The video discusses secant lines as a precursor to understanding tangent lines and derivatives. By measuring the slope between two points on a curve and gradually bringing these points closer, the concept of a limit is introduced, transitioning to the slope of a tangent line as the points converge.

💡Derivative

In calculus, the derivative of a function at a point measures the rate at which the function's value changes as its input changes. The video uses derivatives to find the slope of the tangent line to a curve at any point, describing it as the limit of the slope of the secant lines as they become increasingly close. This concept is crucial for understanding how functions change and is used to solve problems in rates of change and motion.

💡Limit

A limit in mathematics refers to the value that a function or sequence 'approaches' as the input or index approaches some value. In the video, limits are used to define derivatives and understand the behavior of functions as inputs become very close to a specific value, which is fundamental in the analysis of tangent lines and their slopes.

💡Function

A function in mathematics is described as a relation that uniquely associates members of one set with members of another set. The video frequently references functions to demonstrate how tangent and secant lines can be analyzed and calculated, especially when plotting curves graphically using tools like Desmos.

💡Slope

The slope of a line is a measure of its steepness, usually calculated as the change in the vertical direction divided by the change in the horizontal direction (rise over run). The video elaborates on calculating the slope of secant lines and extends this concept to the slope of tangent lines through the derivative, demonstrating how these concepts interlink in the study of calculus.

💡Desmos

Desmos is referenced in the video as a graphical tool used to illustrate mathematical concepts such as curves, tangent lines, and derivatives. It helps visually demonstrate how changing the conditions or parameters of a function affects its graph and the corresponding tangent lines, making abstract concepts more tangible.

💡Algebra

Algebra involves the study of symbols and the rules for manipulating these symbols; it is a unifying thread of almost all of mathematics. The video touches on algebraic methods frequently, such as solving for slopes or rearranging equations to find derivatives, emphasizing its critical role in understanding and applying calculus concepts.

💡Curve

A curve in the context of the video is a graphical representation of a function, which can be linear or nonlinear. Discussing tangent lines and derivatives inherently involves the analysis of points on these curves, understanding their properties, and how they relate to real-world applications like motion paths and rates of change.

💡Point-Slope Form

Point-slope form is a method for writing the equation of a line when you know the slope and a point on the line. This form is used in the video to find equations of tangent lines once the slope at a specific point is determined through derivatives. This form is fundamental in linear algebra and helps bridge the gap between graphical analysis and algebraic representation.

Highlights

A tangent line to a circle is a line that intersects the circle at exactly one point and is perpendicular to the radius at that point.

The purpose of a tangent line is to closely approximate the curve at a given point without actually touching it more than once.

For any curve, a tangent line intersects the curve at exactly one point, and there is only one unique tangent line at any given point on the curve.

Secant lines are lines that pass through any two points along a curve and are used to understand the concept of tangent lines.

The slope of a secant line is calculated using the formula (y2 - y1) / (x2 - x1), where (x1, y1) and (x2, y2) are points on the curve.

The limit of the slope of a secant line as the points x1 and x2 approach each other defines the slope of the tangent line.

The derivative of a function at a point is the limit of the average rate of change as the interval size approaches zero, which is also the slope of the tangent line.

The derivative can be represented as f'(x) = lim(h→0) [f(x+h) - f(x)] / h, which is a fundamental concept in calculus.

For the function g(x) = √x, the derivative g'(x) is found to be 1 / (2√x) by evaluating the limit as h approaches 0.

The point-slope form of a line is y - y0 = m(x - x0), where m is the slope and (x0, y0) is a point on the line.

The slope-intercept form of the tangent line to the function g(x) = √x at x = 4 is y = (1/4)x + 1, verified using Desmos.

Derivatives do not always exist, such as at points where the function is undefined or where the function has a sharp point (like the absolute value function at x = 0).

The concept of a limit is crucial for understanding when a derivative does not exist, as it involves the behavior of the function as it approaches a certain point.

An approximation of the derivative can be obtained numerically by using a small value for h in the derivative formula and evaluating the function at a specific point.

Microsoft Excel can be used to approximate derivatives numerically by calculating the derivative for increasingly smaller values of h.

For engineering, business, or other real-life applications, an approximate value of the derivative can often be sufficient, as exact values are not always necessary.

The video provides a comprehensive understanding of tangent lines, secant lines, and the concept of derivatives, including their practical applications and limitations.

Transcripts

Browse More Related Video

Definition of the Derivative

Definition of the Derivative | Part I

1.4 - Differentiation Using Limits of Difference Quotients

Linear Approximations | Using Tangent Lines to Approximate Functions

Understanding Differentiation Part 1: The Slope of a Tangent Line

The Tangent Line and the Derivative (Calculus)

Related Tags

Tangent Lines Derivatives Calculus Desmos Mathematics Limits Slope Absolute Value Numerical Approximation Educational Content Algebra Geometry