Mean value theorem | MIT 18.01SC Single Variable Calculus, Fall 2010

MIT OpenCourseWare
7 Jan 201103:22
EducationalLearning
32 Likes 10 Comments

TLDRIn this recitation video, the professor challenges students to prove that for a continuous and differentiable function f, if its derivative f' is never zero and a ≠ b, then f(a) ≠ f(b). The solution utilizes the Mean Value Theorem, asserting that the difference f(b) - f(a) is nonzero, given that the derivative is always non-zero and the inputs are distinct. This confirms that different inputs yield different outputs, a principle reminiscent of determining a function's monotonicity based on the sign of its derivative.

Takeaways
  • 📚 The video is a recitation focused on a mathematical problem involving a continuous and differentiable function.
  • 🔍 The problem statement asks to prove that if the derivative of a function is never zero and two inputs are different, the outputs must also be different.
  • 🤔 The professor encourages students to think about the problem before revealing the solution.
  • 📉 The problem is related to the behavior of a function when its derivative has a constant sign, indicating monotonic increase or decrease.
  • 📚 The Mean Value Theorem is the key tool used to solve the problem, applicable to any interval between two different points a and b.
  • 📝 The Mean Value Theorem states that for a continuous function on a closed interval, there exists at least one point c where the derivative equals the average rate of change over the interval.
  • 🔢 The formula derived from the theorem is \( \frac{f(b) - f(a)}{b - a} = f'(c) \) for some c between a and b.
  • 🚫 Given that \( f' \) is never zero, it is impossible for \( f(b) - f(a) \) to be zero, as the product of two non-zero numbers cannot be zero.
  • 📈 The conclusion is that if the inputs are different (a ≠ b), the outputs must also be different (f(a) ≠ f(b)) due to the non-zero derivative.
  • 🔄 The proof exploits the properties of the derivative and the Mean Value Theorem to establish the uniqueness of the function's output for different inputs.
  • 📚 The lesson reinforces the understanding of the relationship between the derivative's sign and the function's monotonicity, as well as the application of the Mean Value Theorem.
Q & A

  • What is the main objective of the video script?

    -The main objective is to demonstrate that if a function f is continuous, differentiable, and its derivative is never zero, then for two distinct values a and b, f(a) is not equal to f(b).

  • What property of the function's derivative is assumed in the problem?

    -The property assumed is that the derivative of the function, denoted as f', is never zero.

  • Why can we apply the Mean Value Theorem to the function f in this context?

    -We can apply the Mean Value Theorem because the function f is continuous on the interval [a, b] and differentiable on the open interval (a, b), which are the necessary conditions for the theorem to hold.

  • What does the Mean Value Theorem state in the context of this script?

    -The Mean Value Theorem states that for a continuous function on [a, b] and differentiable on (a, b), there exists at least one c in (a, b) such that (f(b) - f(a)) / (b - a) = f'(c).

  • What is the significance of the condition that a is not equal to b?

    -The condition that a is not equal to b ensures that we are dealing with two distinct input values for the function, which is necessary to show that the outputs f(a) and f(b) are different.

  • How does the script use the fact that f' is never zero to prove the result?

    -The script multiplies the expression (f(b) - f(a)) / (b - a) by (b - a) to isolate f'(c), and since f'(c) is never zero and (b - a) is not zero, the product f'(c) * (b - a) is also not zero, implying that f(b) - f(a) cannot be zero.

  • What conclusion can we draw from the fact that f(b) - f(a) is not zero?

    -We can conclude that f(a) is not equal to f(b), which means the function f is not constant and its outputs are different for different inputs.

  • How is this problem related to the concept of a function being increasing or decreasing?

    -The problem is related to the concept of monotonicity because if the derivative of a function has a consistent sign (positive or negative), it indicates that the function is either always increasing or always decreasing, respectively.

  • What does the script suggest about the relationship between the sign of the derivative and the behavior of the function?

    -The script suggests that if the derivative of the function has a consistent sign, it can be used to determine whether the function is increasing or decreasing, which is a key aspect of the function's behavior.

  • What is the role of the Mean Value Theorem in proving that f(a) ≠ f(b)?

    -The Mean Value Theorem provides a mathematical framework to establish the existence of a point c in the interval (a, b) where the rate of change (f'(c)) can be used to show that the difference in function values (f(b) - f(a)) cannot be zero, thus proving that f(a) ≠ f(b).

  • Why is it important to consider the sign of the derivative in similar problems?

    -Considering the sign of the derivative is important because it helps in determining the monotonicity of the function, which in turn can be used to analyze the behavior of the function and prove properties such as the one discussed in the script.

Outlines
00:00
📚 Introduction to the Problem

The professor begins the recitation by introducing a mathematical problem involving a continuous and differentiable function 'f' with a non-zero derivative. The challenge is to prove that for two distinct values 'a' and 'b', the function values 'f(a)' and 'f(b)' are not equal. The professor encourages students to ponder this before providing a detailed explanation, hinting at the relevance of the mean value theorem and the behavior of the function's derivative.

Mindmap
Keywords
💡Continuous function
A continuous function is one where the function's values form an unbroken line with no breaks or jumps. In the context of the video, the professor is discussing a function 'f' that is both continuous and differentiable, meaning it has a derivative at every point in its domain. The continuity of 'f' is a prerequisite for applying the Mean Value Theorem, which is central to the argument being made.
💡Differentiable function
A differentiable function is one that has a derivative at every point in its domain. The derivative represents the rate at which the function changes at any given point. In the video, the differentiability of 'f' is crucial because it allows us to consider the behavior of its derivative, which is said to never be zero.
💡Derivative
The derivative of a function measures the sensitivity to change of the function's output with respect to its input. In the script, the professor mentions that the derivative of 'f' (denoted as f') is never zero, indicating that the function is always changing at a non-zero rate, which is key to the argument about the function's output values being distinct for different inputs.
💡Mean Value Theorem
The Mean Value Theorem is a fundamental theorem in calculus that states if a function is continuous on a closed interval [a, b] and differentiable on the open interval (a, b), then there exists at least one c in the interval (a, b) such that the derivative at c is equal to the average rate of change of the function over [a, b]. The professor uses this theorem to argue that since the derivative of 'f' is never zero, the function's values at 'a' and 'b' must be different.
💡Interval
In mathematics, an interval refers to a set of numbers with a specific order, such as from 'a' to 'b'. The script discusses the function 'f' over an interval from 'a' to 'b', emphasizing that the order of 'a' and 'b' does not affect the application of the Mean Value Theorem.
💡Rate of change
The rate of change is a measure of how quickly a quantity changes with respect to another quantity. In the context of the video, the rate of change is represented by the derivative of the function 'f'. The professor uses the rate of change to argue that since the derivative is never zero, the function must be either always increasing or always decreasing, which implies distinct outputs for different inputs.
💡Increasing function
An increasing function is one where the output values increase as the input values increase. The script suggests that if the derivative of 'f' is positive, then 'f' is an increasing function. This is relevant to the main argument because it implies that different input values 'a' and 'b' will lead to different output values.
💡Decreasing function
A decreasing function is the opposite of an increasing function, where the output values decrease as the input values increase. The script implies that if the derivative of 'f' were negative, 'f' would be a decreasing function. This concept is used to contrast with the given condition that the derivative is never zero, reinforcing the idea that the function's values at different inputs must be distinct.
💡Output values
Output values are the results produced by a function for a given set of input values. The video's main theme revolves around demonstrating that for a continuous and differentiable function with a non-zero derivative, if the input values 'a' and 'b' are different, then the corresponding output values f(a) and f(b) must also be different.
💡Input values
Input values are the quantities that are put into a function to obtain an output. In the script, the professor discusses two different input values 'a' and 'b' and uses the properties of the function 'f' to show that these different inputs cannot result in the same output, which is a direct application of the Mean Value Theorem.
Highlights

Introduction to the problem: proving that if a function is continuous, differentiable, and its derivative is never zero, then for two different inputs a and b, the outputs f(a) and f(b) must also be different.

The importance of the derivative's sign in determining if a function is always increasing or decreasing.

Utilization of the Mean Value Theorem to solve the problem.

Condition that the derivative f'(x) is never zero for the function f(x).

Assumption that a is not equal to b, establishing the premise for different inputs.

Explanation of the Mean Value Theorem's application to the interval from a to b, regardless of their order.

Isolating the expression f(b) - f(a) to show it cannot be zero.

Multiplication of the Mean Value Theorem by (b - a) to emphasize the non-zero nature of the expression.

Understanding that f'(c) is never zero, given the problem's conditions.

Recognition that b - a cannot be zero since a is not equal to b.

Logical deduction that the product of two non-zero numbers cannot be zero.

Conclusion that f(b) - f(a) is not zero, implying f(a) is not equal to f(b).

Connection between the problem and previous concepts of a derivative's sign indicating increasing or decreasing functions.

Final summary emphasizing the use of the Mean Value Theorem to determine the function's behavior.

The problem's resemblance to exercises involving the sign of the derivative to determine function growth.

Highlighting the practical application of the Mean Value Theorem in understanding function properties.

Transcripts

Browse More Related Video

Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.

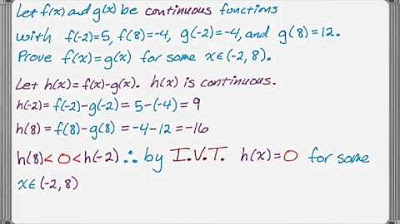
Using the Intermediate Value Theorem Examples

Fundamental theorem of calculus (Part 2) | AP Calculus AB | Khan Academy

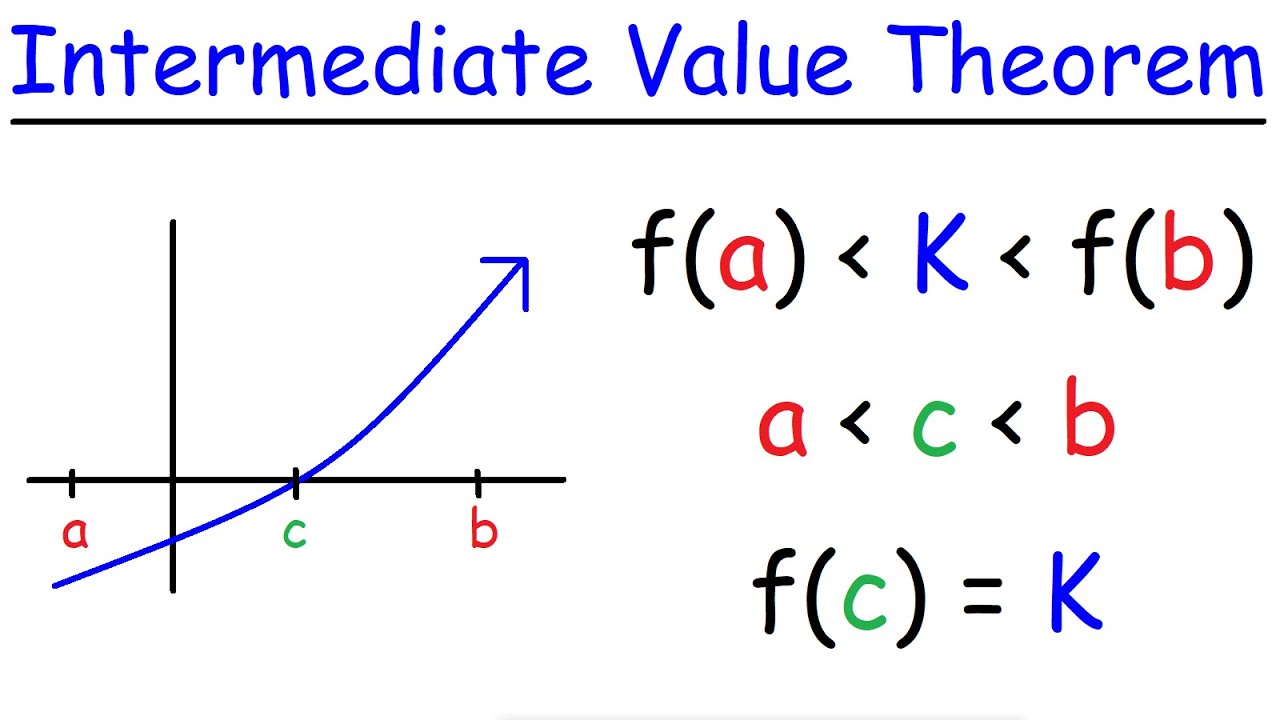
Intermediate Value Theorem

2021 AP Calculus AB Free Response #4 (First Administration)

Intermediate value theorem | Existence theorems | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsMean Value TheoremContinuous FunctionsDifferentiableDerivative AnalysisFunction BehaviorIncreasing FunctionDecreasing FunctionEducational VideoRecitation Guide