2021 AP Calculus AB Free Response #4 (First Administration)
TLDRThe video script discusses the properties of functions and their derivatives in the context of calculus. It begins by introducing a continuous function 'f' defined on a closed interval and represented by line segments on a graph. The focus then shifts to a function 'g', explaining that 'g' is concave up where its second derivative is greater than zero. The script proceeds to calculate the first and second derivatives of 'g', determining the intervals where 'f' has positive slopes. Next, the script defines a function 'p' and computes its derivative at x=3 using the product rule and the values of 'f' and 'g' at that point. The average rate of change of 'g' on a given interval is also calculated. The script concludes by applying L'Hôpital's rule to find a limit and by confirming the applicability of the Mean Value Theorem for 'g' over a specified interval, given that 'g' is both differentiable and continuous.
Takeaways
- 📈 The function f is continuous on the closed interval from -4 to 6 and consists of line segments.
- 📌 To determine if g is concave up, we check if the second derivative is greater than zero, which implies the first derivative of f is positive.
- 🔍 The first derivative of g is found using the Fundamental Theorem of Calculus, which is f(x), and the second derivative is f'(x).
- 📉 The slopes of f are positive from -4 to -2 and from 2 to 6, indicating where g is concave up.
- 🧮 To find p'(3), we use the product rule for differentiation, where p'(x) = g'(x) * f(x) + g(x) * f'(x).
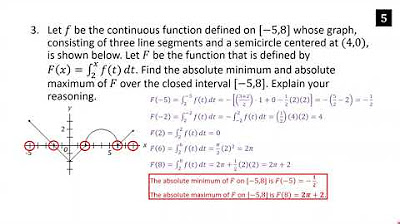
- 📐 f(3) is determined by looking at the graph, and it is -3. g(3) is the area under f from 0 to 3, which is calculated as a combination of a triangle and a trapezoid.
- 🔢 The area under f from 0 to 3 is computed to be -7.5, and f'(3) is the slope of f at x=3, which is +1.
- 🚀 p'(3) is calculated to be 11.5 by substituting the values of f(3), g(3), and f'(3) into the product rule formula.
- 🔬 The limit as x approaches 2 of g(x) * x^2 / 2x is indeterminate, so L'Hôpital's rule is applied, resulting in a limit of -2 as x approaches 2.
- 📊 The average rate of change of g on the interval [-4, 2] is found by calculating the difference in g values at the endpoints and dividing by the difference in x values.
- 🔴 The Mean Value Theorem applies to g on the interval [-4, 2] because g is differentiable on the open interval and continuous on the closed interval.
- 📚 The script provides a comprehensive walkthrough of calculus concepts including derivatives, concavity, limits, and the Mean Value Theorem.
Q & A
What does it mean for a graph to be 'concave up'?
-A graph is 'concave up' when its second derivative is greater than zero, indicating that the function is curving upwards.
How do you find the derivative of a function g defined as an integral?
-The derivative of g, denoted as g', is found by applying the Fundamental Theorem of Calculus, which involves taking the derivative of the integrand (f(x)) and then plugging in the variable x.
What is the condition for the slopes of function f to be greater than zero?
-The slopes of function f are greater than zero where the first derivative of f is positive, which occurs between the intervals of negative four to negative two and two to six in the given script.
How is the function p defined in the script?
-The function p is defined as the product of g'(x) and f(x), which requires the application of the product rule for differentiation.
What is the value of f(3) according to the graph?
-The value of f(3) is -3, as it is the y-coordinate of the point on the graph corresponding to x = 3.
How is g(3) calculated?
-g(3) is calculated as the integral from 0 to 3 of f(t) dt, which involves finding the area under the curve of f from 0 to 3 and summing the areas of the shapes formed (a triangle and a trapezoid in this case).
What is the value of p'(3)?
-The value of p'(3) is 11/2, found by substituting f(3) and g(3) into the expression for p'(x) and evaluating the result.
What is an indeterminate form and how is it resolved?
-An indeterminate form, such as 0/0, occurs when the limit of a function results in a ratio of two quantities that both approach zero or infinity. It is resolved using L'Hôpital's Rule, which involves taking the derivative of the numerator and denominator and re-evaluating the limit.
How do you find the average rate of change of g on the interval from negative 4 to 2?
-The average rate of change is found by calculating the difference in the function values at the endpoints of the interval and dividing by the difference in the x-values. For g on the interval from negative 4 to 2, this involves computing g(2) - g(-4) and (2 - (-4)).
What are the conditions for the Mean Value Theorem to apply to a function g over an interval?
-The Mean Value Theorem applies if the function g is both differentiable on the open interval (a, b) and continuous on the closed interval [a, b].
What does it imply if g'(x) is equal to f(x) for all x in the interval?
-If g'(x) is equal to f(x) for all x in the interval, it implies that g is differentiable over that interval. Since differentiability implies continuity, g is also continuous over the interval.
Outlines
📈 Analysis of Function f and Derivatives
The first paragraph introduces a continuous function f defined on the closed interval from negative four to six, with its graph consisting of foreign line segments. The focus is on determining the intervals where the graph of g, another function, is concave up, which means the second derivative must be greater than zero. To find this, the first and second derivatives of g are computed using the fundamental theorem of calculus. The first derivative of g is found by plugging in x into f(x), and the second derivative is f'(x). The slopes of f are analyzed to determine where they are greater than zero, which are the intervals from negative four to negative two and from two to six. The paragraph also covers finding p'(3) using the product rule and integrating to find g(3), concluding with the calculation of p'(3) as eleven and a half.
🔍 Limit Computation and Mean Value Theorem
The second paragraph deals with calculating the limit of g(x) as x approaches two, which initially results in an indeterminate form of zero over zero. L'Hôpital's rule is applied to resolve this, leading to the computation of g'(x)/2x as x approaches two. The value of f(2) is determined to be negative two, which helps in finding the limit. The average rate of change of g on the interval from negative four to two is then calculated, using the secant line method and integrating f(t) from zero to negative four to find g(-4). The paragraph concludes with a discussion on the Mean Value Theorem's applicability to the interval from negative four to two, verifying that g(x) is differentiable on the open interval and continuous on the closed interval, thus satisfying the conditions of the theorem.
Mindmap
Keywords
💡Continuous function
💡Derivative
💡Concave up
💡Fundamental theorem of calculus
💡Product rule
💡Mean value theorem
💡Average rate of change
💡Indeterminate form
💡L'Hôpital's rule
💡Slope
💡Integral
Highlights
The function f is continuous on the closed interval from negative four to six and consists of foreign line segments.
The function g is defined and its graph is concave up where the second derivative is greater than zero.
The derivative of g is found using the fundamental theorem of calculus by substituting x into f(x).
The second derivative of g is equal to f'(x), indicating where the first derivative of f is greater than zero.
The slopes of f are positive between the intervals negative four to negative two and from two to six.
Function p is defined, and to find p'(3), the product rule is applied using g'(x) and f(x).
The value of f(3) is determined by looking at the y-value on the graph, which is negative three.
g(3) is calculated as the integral from 0 to 3 of f(t) dt, representing the area under f from zero to three.
The area under f from zero to three is computed, resulting in a total area of negative seven halves.
The slope of f at x equals three is determined to be positive one, as it goes up one unit.
p'(3) is calculated to be eleven halves by substituting the values of f(3), g(3), and f'(3) into the equation.
The limit as x approaches two of g(x) * x^2 / 2x is indeterminate, prompting the use of L'Hôpital's rule.
g'(x) is simplified to f(x) / (2x - 2), and the value at x=2 is substituted to find the limit.
The average rate of change of g on the interval negative 4 to 2 is calculated using the secant line value.
g(2) is determined to be zero, and g(-4) is computed by integrating from 0 to negative four of f(t) dt.
The area from negative 4 to 0 is calculated, resulting in a total of negative sixteen.
The average rate of change is found to be eight thirds by dividing the difference in g values by the interval width.
The Mean Value Theorem is confirmed to apply as g(x) is both differentiable and continuous on the interval negative four to two.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: