Arccos | MIT 18.01SC Single Variable Calculus, Fall 2010
TLDRIn this educational video, the instructor, Joel Lewis, delves into the concept of the arccosine function, a topic not covered in the lecture. He explains the graph and properties of the cosine function, then illustrates how to derive the arccosine function by reflecting the cosine curve across the line y=x. The instructor emphasizes the need to restrict the domain to ensure the arccosine is a function, choosing the interval from -1 to 1. He then calculates the derivative of arccosine using implicit differentiation and simplifies it using the Pythagorean identity, revealing its resemblance to the arcsine derivative. The summary concludes with the graph and the derivative formula of the arccosine function.
Takeaways
- 📚 The script discusses the concept of inverse trigonometric functions, focusing on the arccosine function.
- 📈 The graph of y = cosine x is presented, showing its maximum at x = 0, zeros at x = π/2, and trough at x = π.
- 🔄 The cosine function is an even function and periodic with a period of 2π, and is derived from the sine function shifted by π/2.
- 🤔 To graph the arccosine function, the cosine curve is reflected over the line y = x, but this initial reflection does not represent a function due to failing the vertical line test.
- 📉 The arccosine function is defined by selecting a portion of the reflected curve that passes the vertical line test, specifically from x = -1 to x = 1, with y ranging from 0 to π.
- 📝 The notation for the arccosine function is given to reflect its domain [-1, 1] and range [0, π], indicating the output is always between 0 and π.
- 🔑 To find the derivative of the arccosine function, the script uses the relationship cos(y) = x, where y = arccos(x), and applies implicit differentiation.
- 📌 The derivative of arccosine, dy/dx, is derived to be -1 / sin(y), which simplifies to -1 / sqrt(1 - x^2) after substituting y with arccosine of x.
- 📐 The simplification of the derivative uses the Pythagorean theorem in the context of a right triangle where the adjacent side is x and the hypotenuse is 1.
- 📈 The graph of arccosine is a reflection of the arcsine graph, which is why their derivatives are closely related.
- 🔚 The script concludes with a summary of the most important inverse trigonometric functions, including their graphs and derivatives.
Q & A
What is the arccosine function and what is its graph based on?
-The arccosine function, also known as the inverse cosine function, is the inverse of the cosine function. Its graph is derived by reflecting the graph of y = cosine x across the line y = x, but only taking the portion that passes the vertical line test, which is from x = -1 to x = 1.
Why is the full graph of y = cosine x not a function?
-The full graph of y = cosine x is not a function because it fails the vertical line test. The cosine function has multiple values for a single x value, which is not allowed for a function where each x must map to a single y.
What is the domain and range of the arccosine function as discussed in the script?
-The domain of the arccosine function is all real numbers between -1 and 1, inclusive. The range is from 0 to π, as the function maps each x value in its domain to an angle in radians between 0 and π.
How is the derivative of the arccosine function found?
-The derivative of the arccosine function is found using implicit differentiation. By setting y = arccosine(x) and then differentiating both sides with respect to x, we get dy/dx = -1 / sine(y). Substituting y back with arccosine(x) simplifies it to dy/dx = -1 / sqrt(1 - x^2).
What is the relationship between the graphs of arccosine and arcsine?
-The graph of arccosine is a reflection of the graph of arcsine across the line y = x. This is because cosine and sine functions are similar in shape, and their inverses follow a similar pattern.
Why is the sine of arccosine of x equal to the square root of 1 minus x squared?
-The sine of arccosine of x is derived from a right triangle where the adjacent side (x) over the hypotenuse (1) equals x. Using the Pythagorean theorem, the opposite side is the square root of 1 minus x squared, which is the sine of the angle.
What is the significance of the arccosine function in trigonometry?
-The arccosine function is significant as it allows us to find an angle given the cosine of that angle. It is one of the fundamental inverse trigonometric functions used in various mathematical and real-world applications.
How does the periodicity of the cosine function affect the graph of the arccosine function?
-The periodicity of the cosine function, with a period of 2π, means that the cosine function repeats its values every 2π. However, the arccosine function is only defined for a specific interval (-1 to 1) to ensure it is a function, thus avoiding the repetition issue.
What is the maximum value of the arccosine function?
-The maximum value of the arccosine function occurs at x = -1, where the function outputs y = π. This is because the arccosine of -1 is the angle whose cosine is -1, which is π radians.
What is the minimum value of the arccosine function?
-The minimum value of the arccosine function occurs at x = 1, where the function outputs y = 0. This is because the arccosine of 1 is the angle whose cosine is 1, which is 0 radians.
How is the arccosine function related to the unit circle?
-The arccosine function is related to the unit circle as it represents the angle whose cosine value corresponds to a point on the unit circle. The function helps in determining the angle when the x-coordinate (cosine value) is known.
Outlines
📚 Introduction to Arccosine Function
Professor Joel Lewis introduces the arccosine function, also known as the inverse cosine, to the students during a recitation session. He explains that the function is derived from the cosine function, which has a maximum value of 1 at x=0 and a periodicity of 2π. The cosine function's graph is even and can be obtained by shifting the sine function to the left by π/2. The professor then describes the process of creating the arccosine function's graph by reflecting the cosine function's graph across the line y=x, noting that the resulting curve does not pass the vertical line test and thus is not a function. To correct this, a specific segment of the curve that does pass the vertical line test is selected, which is the piece from x=-1 to x=1, with y ranging from π to 0.
🔍 Derivative of Arccosine Function
The second paragraph delves into the process of finding the derivative of the arccosine function. The professor uses the relationship between the cosine and arccosine functions to establish that cos(y) = x, where y is the arccosine of x. Differentiating both sides with respect to x yields the derivative of arccosine, dy/dx, as -1/sin(y). To express this derivative in terms of x, the professor substitutes y with arccosine of x, resulting in a formula that involves the square root of 1 - x^2. He explains that the sine of the arccosine of x is the square root of 1 - x^2, simplifying the derivative to -1/√(1 - x^2). This formula is similar to the derivative of arcsine, reflecting the close relationship between the cosine and sine functions and their inverses.
Mindmap
Keywords
💡Inverse Trigonometric Functions
💡Arccosine
💡Graph
💡Derivative
💡Cosine Function
💡Periodic Function
💡Vertical Line Test
💡Domain and Range
💡Chain Rule
💡Pythagorean Theorem
💡Square Root
Highlights
Introduction to the arccosine function, also known as the inverse cosine function.
Explanation of the graph of y equals cosine x, including its maximum at x equals 0 and periodicity with a period of 2 pi.
Description of the cosine function as an even function with symmetry around the y-axis.
Process of graphing the arccosine function by reflecting the cosine curve across the line y equals x.
Observation that the reflected curve does not pass the vertical line test, indicating it is not a function.
Selection of a specific segment of the curve to define the arccosine function, which passes the vertical line test.
Definition of the domain and range for the arccosine function, taking values between -1 and 1 and outputting values between 0 and pi.
Differentiation of the arccosine function using implicit differentiation and the chain rule.
Derivation of the formula for the derivative of arccosine of x as minus 1 divided by sine y.
Substitution of y with arccosine of x to express the derivative in terms of x.
Use of the Pythagorean theorem to find the sine of the arccosine of x as the square root of 1 minus x squared.
Simplification of the derivative formula to minus 1 over the square root of one minus x squared.
Comparison of the derivative of arccosine with that of arcsine, highlighting their similarity due to the nature of cosine and sine functions.
Reflection on the graph of arccosine as a reflection of the graph of arcsine, explaining the relationship between their derivatives.
Completion of the discussion on the most important inverse trigonometric functions including their graphs and derivatives.
Transcripts
Browse More Related Video
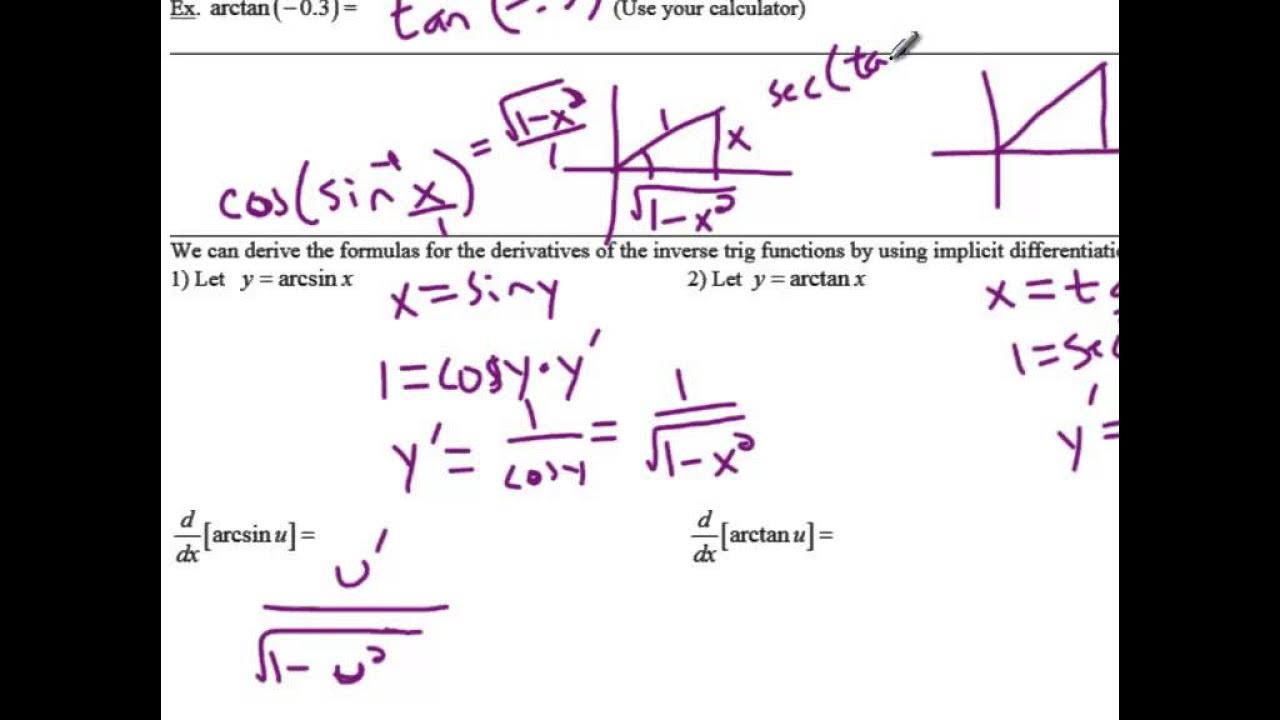
Inverse Trig Functions and Differentiation
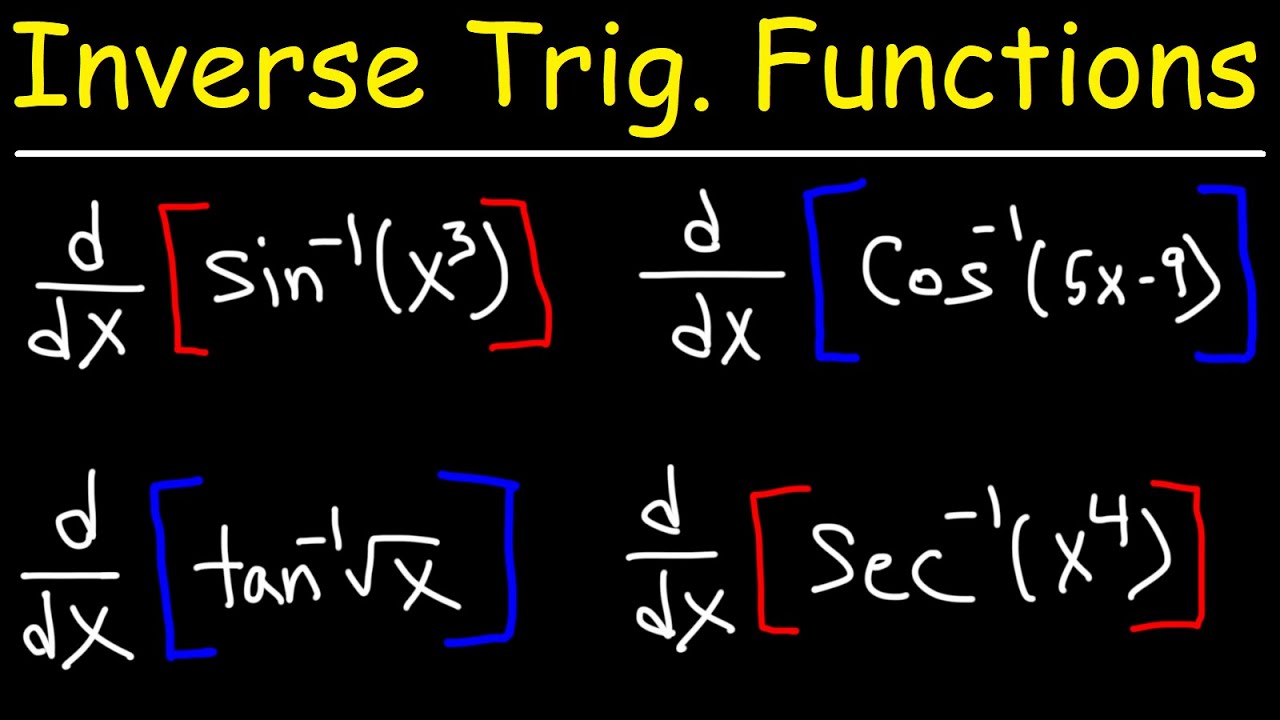
Derivatives of Inverse Trigonometric Functions

Derivative of Inverse Trig Functions via Implicit Differentiation
Tangent Line to a Polynomial | MIT 18.01SC Single Variable Calculus, Fall 2010

Implicit Differentiation | Differentiation when you only have an equation, not an explicit function
Integration of Taylor's Series | MIT 18.01SC Single Variable Calculus, Fall 2010
5.0 / 5 (0 votes)
Thanks for rating: