Derivative of Inverse Trig Functions via Implicit Differentiation

Dr. Trefor Bazett

12 Sept 201704:41

EducationalLearning

32 Likes 10 Comments

TLDRThis transcript details the process of finding the derivative of the arctangent function using implicit differentiation. The video begins by establishing the relationship between the tangent function and its inverse, the arctangent, within a restricted domain. It then proceeds to differentiate both sides of the equation with respect to X, applying the chain rule to handle the composition of functions. The derivative of the tangent function is identified as secant squared, and the process continues by isolating the derivative of Y with respect to X. The video cleverly uses trigonometric identities, specifically the Pythagorean identities, to express the derivative in terms of X, ultimately arriving at the formula \(1/(x^2 + 1)\). The method demonstrated is applicable to finding derivatives of other trigonometric functions such as sine, cosine, and cotangent, providing a comprehensive understanding of the derivative process for inverse trigonometric functions.

Takeaways

📈 The arctangent function is the inverse of the tangent function, restricted to the domain between -π/2 and π/2.
↔️ The relationship between x and y when y is the arctangent of x is that x equals the tangent of y.
🔄 Implicit differentiation is used to find the derivatives of trigonometric functions when y is a function of x.
📌 The derivative of the tangent of y with respect to x, where y is a function of x, is found using the chain rule and is secant squared times dy/dx.
🔢 The derivative of x with respect to x is 1, which is used to isolate dy/dx in the differentiation process.
🔄 To isolate dy/dx, the equation is rearranged to express dy/dx as 1 over secant squared of y.
🔗 The Pythagorean identities are crucial for expressing the derivative in terms of x, with the main identity being sine squared plus cosine squared equals 1.
🔄 By using the Pythagorean identity, 1 over secant squared is replaced by 1 over (sine squared plus 1), which is then expressed in terms of x.
🎓 The final expression for the derivative of arctangent with respect to x is 1 over (x squared plus 1).
📙 The process demonstrated for finding the derivative of arctangent can be applied to find the derivatives of other trigonometric functions like sine, cosine, or cotangent.
🧮 Memorizing the main Pythagorean identity and deducing the others is a useful strategy for solving trigonometric differentiation problems.

Q & A

What is the relationship between the tangent function and its inverse, the arctangent, in the context of the script?
-The tangent function and its inverse, the arctangent, are related such that the tangent of the arctangent of X is equal to X itself. They are inverse functions that undo each other when applied consecutively.
How is implicit differentiation used in the script to find the derivative of the arctangent function?
-Implicit differentiation is used by taking the derivative of both sides of the equation with respect to X, considering Y as a function of X, and applying the chain rule to handle the composition of functions.
What is the derivative of the tangent function with respect to X, according to the chain rule?
-The derivative of the tangent function with respect to X is the secant squared of Y, multiplied by the derivative of Y with respect to X, which is Y (or dy/dx).
How does the script isolate dy/dx to express it solely in terms of X?
-The script isolates dy/dx by dividing both sides of the derived equation by secant squared of Y, resulting in dy/dx = 1 / (secant squared of Y).
What trigonometric identity is primarily used to express the derivative of arctangent in terms of X?
-The primary trigonometric identity used is the Pythagorean identity sine squared plus cosine squared equals 1, which is then manipulated to express secant squared in terms of tangent squared plus 1.
How is the final expression for the derivative of arctangent simplified in terms of X?
-The final expression is simplified by substituting secant squared with (tangent squared of Y + 1) and recognizing that tangent of Y is equivalent to X, leading to the derivative being 1 / (X squared + 1).
What is the final formula for the derivative of arctangent in terms of X?
-The final formula for the derivative of arctangent with respect to X is dy/dx = 1 / (X squared + 1).
How does the script remind us of the relationship between Y and X in the context of the arctangent function?
-The script reminds us that Y is the arctangent of X, which is the same as saying X is the tangent of Y, allowing us to replace tangent of Y with X in our expressions.
What is the significance of using the Pythagorean identities in the process of finding the derivative of arctangent?
-The Pythagorean identities are significant because they allow us to express secant squared, which appears in the derivative, in terms of tangent, which can then be related back to X to find the derivative in terms of X.
Why is it important to express the derivative in terms of X when dealing with the arctangent function?
-It is important to express the derivative in terms of X because the derivative of a function with respect to a variable should ideally be a function of that same variable, making the expression more meaningful and applicable in calculus.
How can the process used in the script to find the derivative of arctangent be generalized for other trigonometric functions?
-The same process of implicit differentiation, applying the chain rule, and using trigonometric identities can be generalized to find the derivatives of other trigonometric functions like sine, cosine, or cotangent.
What is the role of the chain rule in differentiating composite functions such as the tangent of a function of X?
-The chain rule is essential for differentiating composite functions as it allows us to differentiate the outer function (like tangent) with respect to the inner function (like Y, which is a function of X), by multiplying the derivative of the outer function by the derivative of the inner function.

Outlines

00:00

🧮 Deriving the Function of Arctangent

The paragraph begins with an introduction to the arctangent function, explaining its relationship with the tangent function as inverse functions. It discusses the process of finding the derivative of arctangent using implicit differentiation. The explanation involves differentiating both sides of the equation with respect to X, applying the chain rule for the composition of functions, and simplifying the result to isolate dy/dx. The final step involves using trigonometric identities, specifically the Pythagorean identities, to express the derivative solely in terms of X, resulting in the formula 1/(x^2 + 1) for the derivative of arctangent with respect to X.

Mindmap

Keywords

💡arctangent

Arctangent, also known as the inverse tangent function, is a mathematical function that is the inverse of the tangent function. In the context of the video, arctangent is used to describe the relationship between the tangent function and its inverse, where the arctangent of a value x is the angle whose tangent is x. It is central to the discussion of differentiating trigonometric functions.

💡implicit differentiation

Implicit differentiation is a technique used in calculus to find the derivatives of equations that are not explicitly solved for y as a function of x. In the video, implicit differentiation is used to find the derivative of the arctangent function, as the relationship between x and y is not explicitly given in the form y = f(x).

💡trigonometric functions

Trigonometric functions are mathematical functions that relate the angles of a triangle to the lengths of its sides, particularly useful in the study of periodic phenomena. In the video, the focus is on understanding the derivatives of these functions, such as tangent, sine, and cosine, which are essential in calculus.

💡derivative

A derivative in calculus represents the rate at which a function changes with respect to a variable. It is a fundamental concept used to analyze the behavior of functions. In the video, the derivative of the arctangent function is the primary focus, and the process of finding it is explained in detail.

💡Pythagorean identities

Pythagorean identities are mathematical relationships involving trigonometric functions that are derived from the Pythagorean theorem. They are essential in simplifying trigonometric expressions. In the video, these identities are used to express secant squared in terms of tangent, which helps in finding the derivative of the arctangent function.

💡tangent function

The tangent function, often abbreviated as tan, is a trigonometric function that measures the ratio of the opposite side to the adjacent side in a right-angled triangle. In the video, the tangent function is used to establish the relationship between x and y, where y is the arctangent of x, and x is the tangent of y.

💡chain rule

The chain rule is a fundamental theorem in calculus used to compute the derivative of a composite function. It states that the derivative of a function composed of two functions is the product of the derivative of the outer function and the derivative of the inner function. In the video, the chain rule is applied to find the derivative of the tangent of y with respect to x.

💡secant function

The secant function, denoted as sec, is the reciprocal of the cosine function. It is used in trigonometry to find the ratio of the hypotenuse to the adjacent side of a right-angled triangle. In the video, secant squared is used to express the derivative of the tangent function and is later simplified using the Pythagorean identities.

💡differentiation with respect to x

Differentiation with respect to x is the process of finding the rate of change of a function with respect to the variable x. It is a key operation in calculus and is used in the video to find the derivative of the arctangent function by differentiating both sides of the equation involving x and y.

💡trigonometric identities

Trigonometric identities are equations that hold true for all values of the variables under consideration. They are used to simplify and manipulate trigonometric expressions. In the video, the Pythagorean identities are a specific type of trigonometric identities that are used to express secant squared in terms of tangent squared plus one.

💡cotangent function

The cotangent function, often abbreviated as cot, is the reciprocal of the tangent function. It is another trigonometric function that relates the angles of a right-angled triangle to the lengths of its sides. In the video, cotangent is mentioned as part of the Pythagorean identities, which are used to simplify the expression for the derivative of the arctangent function.

Highlights

The graph of the tangent function is explored in relation to the arctangent function.

The concept of inverting the tangent function over the line y=x is introduced.

The restricted domain of the tangent function between -π/2 and π/2 is mentioned.

Implicit differentiation is used to find the derivatives of trigonometric functions.

The definition of Y being the arctangent of X is used to derive the relationship between X and Y.

The chain rule is applied in the process of implicit differentiation.

The derivative of the tangent function is found to be secant squared.

The derivative dy/dx is isolated to find its relationship with secant squared of Y.

The need to express the derivative in terms of X rather than Y is emphasized.

Pythagorean trigonometric identities are used to express secant squared in terms of tangent.

The relationship between X and the tangent of Y is utilized to express the derivative in terms of X.

The final expression for the derivative of arctangent is given as 1/(x^2 + 1).

The process demonstrated can be generalized to find derivatives of other trigonometric functions.

The importance of memorizing the Pythagorean identity sin^2(θ) + cos^2(θ) = 1 is highlighted.

The identities tan^2(θ) + 1 = sec^2(θ) and csc^2(θ) = 1/sin^2(θ) are derived from the main Pythagorean identity.

The final derivative formula of arctangent, dy/dx = 1/(x^2 + 1), is expressed as a function of X.

The transcript provides a comprehensive method for deriving derivatives of inverse trigonometric functions.

The use of trigonometric identities simplifies the expression of derivatives in terms of the original function's variable.

Transcripts

Browse More Related Video

Derivative of inverse tangent | Taking derivatives | Differential Calculus | Khan Academy

Quotient Rule | MIT 18.01SC Single Variable Calculus, Fall 2010

How To Find The Derivative of Sin^2(x), Sin(2x), Sin^2(2x), Tan3x, & Cos4x

Integrals: Trig Substitution 2

The Derivative of ln(x) via Implicit Differentiation

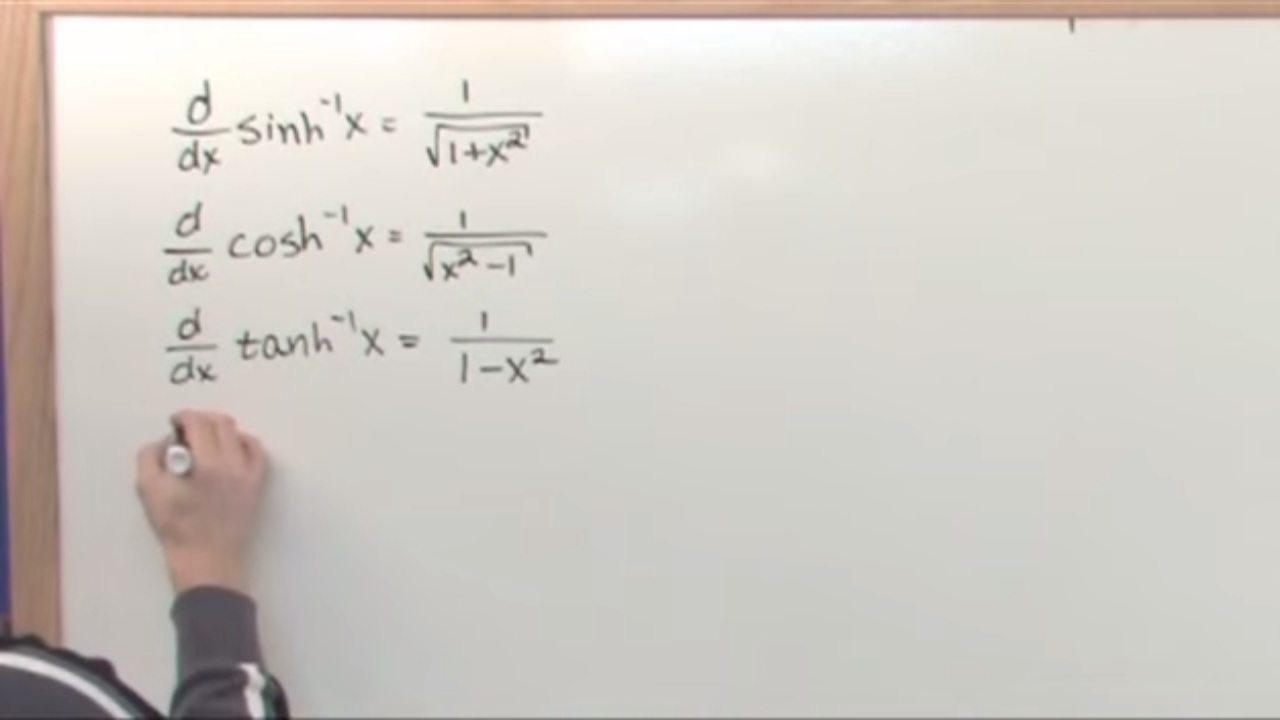
Lesson 4 - Inverse Hyperbolic Functions (Calculus 2 Tutor)

Derivative of Inverse Trig Functions via Implicit Differentiation

Takeaways

Q & A

What is the relationship between the tangent function and its inverse, the arctangent, in the context of the script?

How is implicit differentiation used in the script to find the derivative of the arctangent function?

What is the derivative of the tangent function with respect to X, according to the chain rule?

How does the script isolate dy/dx to express it solely in terms of X?

What trigonometric identity is primarily used to express the derivative of arctangent in terms of X?

How is the final expression for the derivative of arctangent simplified in terms of X?

What is the final formula for the derivative of arctangent in terms of X?

How does the script remind us of the relationship between Y and X in the context of the arctangent function?

What is the significance of using the Pythagorean identities in the process of finding the derivative of arctangent?

Why is it important to express the derivative in terms of X when dealing with the arctangent function?

How can the process used in the script to find the derivative of arctangent be generalized for other trigonometric functions?

What is the role of the chain rule in differentiating composite functions such as the tangent of a function of X?