Derivatives of Inverse Trigonometric Functions

The Organic Chemistry Tutor
26 Feb 201806:19
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script delves into the differentiation of inverse trigonometric functions, providing a comprehensive guide on how to find derivatives of functions such as arcsine, arccosine, and arctangent. The script clearly explains the formulas and processes involved, using specific examples like the derivative of arcsine of x cubed and arccosine of 5x minus 9. It also covers the derivation of more complex functions, including the arctangent of square root x and the arc secant of x to the fourth power. The detailed walkthrough of identifying 'u' and 'u prime', and applying the correct formulas, makes this an informative resource for anyone looking to understand the calculus behind inverse trigonometric functions.

Takeaways
  • 📚 The focus of the lesson is on differentiating inverse trigonometric functions, which is an important concept in calculus.
  • 🌟 The derivative of the arcsine function is given by the formula: (u prime) / √(1 - u^2), where u is the argument of the arcsine function.
  • 🔍 To find the derivative of arcsine(x^3), identify u as x^3 and its derivative u prime as 3x^2, then apply the formula to get the result: 3x^2 / √(1 - x^6).
  • 📈 For the arccosine function, the derivative formula is similar to arcsine but with a negative sign: -(u prime) / √(1 - u^2).
  • 🧠 The derivative of arccosine(5x - 9) is -5 / √(1 - (5x - 9)^2), by identifying u as 5x - 9 and its derivative as 5.
  • 🔧 The derivative of the arctangent function is given by: (u prime) / (1 + u^2), where u is the argument of the arctangent function.
  • 🛠️ To find the derivative of arctangent(√x), identify u as √x (or x^(1/2)), its derivative u prime as 1/(2√x), and then apply the formula to obtain the result: 1/(2√x) / (1 + x).
  • 📊 The derivative of the arcsecant function is derived from the formula: (u prime) / |u|√(u^2 - 1), where u is the argument of the arcsecant function.
  • 👉 For arcsecant(x^4), u is x^4 and its derivative u prime is 4x^3. The derivative is then 4x^3 / |x^4|√(x^8 - 1), which simplifies to 4/x√(x^8 - 1).
  • 📝 Understanding and applying these formulas is crucial for solving problems involving the derivatives of inverse trigonometric functions.
  • 🎓 The process of differentiating inverse trigonometric functions involves identifying the correct u and u prime for each specific function and then applying the appropriate formula.
Q & A

  • What is the main focus of the lesson?

    -The main focus of the lesson is to differentiate inverse trigonometric functions.

  • What is the derivative of the arc sine function of x cubed?

    -The derivative of the arc sine of x cubed is 3x squared divided by the square root of 1 minus x to the sixth power.

  • What is the general formula for the derivative of an arc sine function?

    -The general formula for the derivative of an arc sine function, given as arc sine u, is u prime divided by the square root of 1 minus u squared.

  • What is the derivative of the arc cosine function of 5x minus 9?

    -The derivative of the arc cosine of 5x minus 9 is negative five divided by the square root of 1 minus (5x minus 9) squared.

  • How is the derivative of an arc cosine function different from that of an arc sine function?

    -The derivative of an arc cosine function is similar to that of an arc sine function, but with a negative u prime instead of a positive one.

  • What is the derivative of the arc tangent function of the square root of x?

    -The derivative of the arc tangent of the square root of x is one over two square root x, divided by two x times (1 plus x).

  • What is the general formula for the derivative of an arc tangent function?

    -The general formula for the derivative of an arc tangent function, given as arc tan u, is u prime divided by one plus u squared.

  • What is the derivative of the arc secant function of x to the fourth power?

    -The derivative of the arc secant of x to the fourth power is four over x times the square root of (x to the eight minus one).

  • What is the general formula for the derivative of an arc secant function?

    -The general formula for the derivative of an arc secant function is u prime divided by the absolute value of u times the square root of u squared minus one.

  • How does the absolute value symbol affect the derivative of the arc secant function?

    -The absolute value symbol ensures that the result is always positive, even though in this specific case, the term is always positive, so it can be omitted for simplification.

  • How can the expression for the derivative of the arc tangent function be rationalized?

    -The expression for the derivative of the arc tangent function can be rationalized by multiplying both the numerator and the denominator by the square root of x.

Outlines
00:00
📚 Derivatives of Inverse Trigonometric Functions

This paragraph introduces the concept of derivatives of inverse trigonometric functions, starting with the derivative of arc sine of x cubed. It emphasizes the importance of knowing the formula for such problems, which is u prime divided by the square root of 1 minus u squared. The process involves identifying u (x cubed in this case) and its derivative (3x squared). The final answer is derived by substituting these into the formula, resulting in 3x squared divided by the square root of 1 minus x to the sixth power. The paragraph then proceeds to solve additional examples involving the derivatives of arc cosine and arc tangent of various functions, highlighting the use of specific formulas for each inverse trigonometric function.

05:02
📈 Derivatives of Arc Secant and Simplification

This paragraph focuses on the derivative of arc secant of x to the fourth power. It explains that u is x to the fourth and u prime is four x cubed. The formula for the derivative of arc secant involves u prime divided by the absolute value of u times the square root of u squared minus one. The absolute value is not necessary in this case as the term is always positive. The calculation simplifies to four x cubed divided by x to the fourth, taking the square root of x to the eighth minus one. The final simplification results in four over x times the square root of x to the eighth minus one, providing a clear and comprehensive understanding of the process of deriving and simplifying the expression for the arc secant function.

Mindmap
Keywords
💡Derivative
The derivative is a fundamental concept in calculus that represents the rate of change of a function with respect to its variable. In the context of the video, it is used to find the derivative of inverse trigonometric functions, which is the focus of the lesson. The derivative is calculated using specific formulas and rules, such as the power rule, and is crucial for understanding the behavior of functions and their rates of change at different points.
💡Arc Sine
Arc sine is an inverse trigonometric function that gives the angle whose sine is a given value. In the video, the arc sine function is used with different inputs, such as x cubed, and the goal is to find its derivative. The process involves identifying the 'u' in the function and then applying the derivative formula for the inverse sine function, which is u prime divided by the square root of 1 minus u squared.
💡Arc Cosine
Arc cosine is another inverse trigonometric function that finds the angle whose cosine is a given value. Similar to arc sine, the video discusses finding the derivative of arc cosine functions, with a key difference that the derivative involves a negative sign. The derivative formula for arc cosine is negative u prime divided by the square root of 1 minus u squared.
💡Arc Tangent
Arc tangent is the inverse trigonometric function that finds the angle whose tangent is a given value. The derivative of the arc tangent function is derived using a specific formula, which is u prime divided by one plus u squared. In the video, the arc tangent of square root x is used to illustrate the process of finding the derivative, involving the manipulation of square roots and rationalizing the denominator.
💡Inverse Trigonometric Functions
Inverse trigonometric functions are mathematical functions that 'undo' the trigonometric functions sine, cosine, and tangent, allowing us to find angles based on their trigonometric values. The video's main theme revolves around differentiating these inverse functions, which requires understanding their individual derivative formulas and how to apply them to various problems.
💡Power Rule
The power rule is a fundamental rule in calculus that allows us to find the derivative of a function that is raised to a power. It states that the derivative of x to the n, where n is any real number, is n times x to the (n-1). In the video, the power rule is used when differentiating functions like x to the one half, where the derivative is calculated as one half x to the minus one half.
💡Rationalizing the Denominator
Rationalizing the denominator is a mathematical process used to eliminate the radical (or root) from the denominator of a fraction, resulting in a fraction that has only rational numbers in the denominator. This is often done to simplify expressions or prepare them for further calculations. In the video, it is used when simplifying the derivative of arc tangent of square root x.
💡Absolute Value
Absolute value is a mathematical concept that represents the distance of a number from zero on the number line, regardless of direction. It is denoted by vertical bars and is used in various mathematical expressions and functions, including inverse trigonometric functions. In the video, the absolute value is used in the derivative formula for arc secant, although it is noted that it is not necessary in this specific case as the term is always positive.
💡Arc Secant
Arc secant is the inverse trigonometric function of the secant function, which finds the angle whose secant is a given value. The derivative of the arc secant function is more complex than that of the arc sine or arc cosine and involves the absolute value and square root of terms. In the video, the derivative formula for arc secant is discussed and applied to the function with input x to the fourth power.
💡Trigonometric Functions
Trigonometric functions are mathematical functions that relate angles to the ratios of the lengths of the sides of a right triangle. The three primary trigonometric functions are sine, cosine, and tangent, and their inverse functions are arc sine, arc cosine, and arc tangent. The video focuses on the derivatives of the inverse trigonometric functions, which are calculated based on the original trigonometric functions.
Highlights

The lesson focuses on differentiating inverse trigonometric functions, providing a comprehensive understanding of the topic.

The derivative of the arc sine function is introduced, with a specific example of arc sine of x cubed.

A formula for the derivative of the arc sine function is provided, which is u prime divided by the square root of 1 minus u squared.

The process of identifying u and u prime in the context of the arc sine function is explained.

The derivative of x cubed is calculated as 3x squared, which is a key step in the example problem.

The final answer for the derivative of arc sine x cubed is presented as 3x squared divided by the square root of 1 minus x to the sixth power.

The derivative of the arc cosine function is discussed, with a specific example of arc cosine of 5x minus 9.

The formula for the derivative of the arc cosine function is given, which involves a negative u prime divided by the square root of 1 minus u squared.

The derivative of 5x minus 9 is identified as 5, which is crucial for solving the example problem.

The solution for the derivative of arc cosine 5x minus 9 is presented as negative five divided by the square root of 1 minus (5x minus 9) squared.

The derivative of the arc tangent function is explored, with a focus on the example of arc tangent of square root x.

The formula for the derivative of the arc tangent function is shared, which involves u prime divided by 1 plus u squared.

The derivative of x to the one half is calculated as one over 2x to the positive one half, which is a key intermediate step.

The final expression for the derivative of arc tangent of square root x is simplified to the square root of x divided by two x times (1 plus x).

The derivative of the arc secant function is examined, with an example of arc secant x to the fourth power.

The formula for the derivative of the arc secant function is provided, involving u prime divided by the absolute value of u times the square root of u squared minus one.

The derivative of x to the fourth is calculated as 4x cubed, which is an essential part of solving the example problem.

The final derivative of arc secant x to the fourth power is presented as four over x times the square root of (x to the eight minus one).

Transcripts

Browse More Related Video

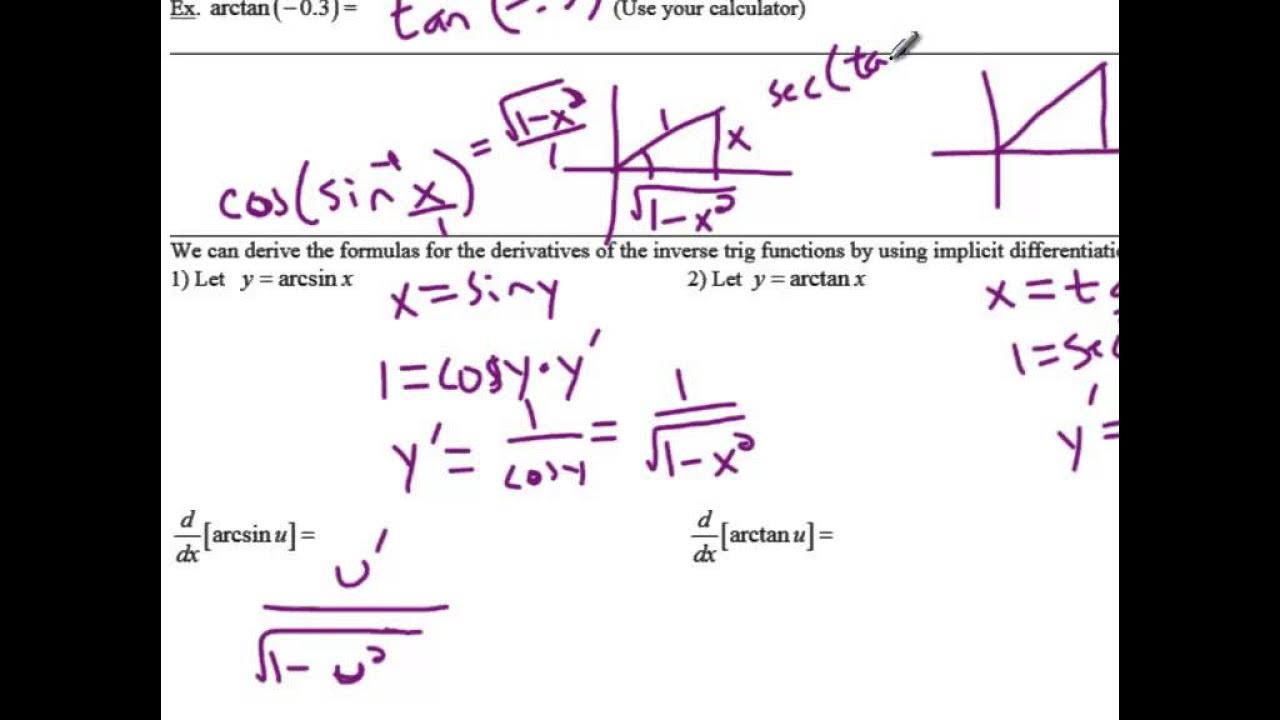
Inverse Trig Functions and Differentiation

An Indepth Look at Using Inverse Trig Functions (Precalculus - Trigonometry 21)

Lesson 2 - Derivatives Of Inverse Trig Functions (Calculus 2 Tutor)

Evaluating Inverse Trigonometric Functions

Take Derivatives of Inverse Trig Functions (ArcSin, ArcCos) - [2]

Integration into Inverse trigonometric functions using Substitution

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Inverse TrigonometryDerivativesMathematicsEducationArcsineArccosineArctangentArcsecantFormulasCalculus