Inverse Trig Functions and Differentiation

Chad Gilliland
4 Dec 201309:38
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video offers a comprehensive review of inverse trigonometric functions, focusing on their derivatives. It begins with an explanation of the restricted ranges for arcsine, arccosine, and arctangent, followed by practical examples of finding angles using these functions. The instructor then delves into deriving the formulas for the derivatives of arcsine and arctangent using implicit differentiation, providing step-by-step guidance. The video also covers the derivative of arccosine, which is the negative of the arcsine derivative. Finally, the script includes examples of applying these derivatives using the chain rule, making it an informative resource for students preparing for exams like the AP Calculus.

Takeaways
  • πŸ“š The video provides a review of inverse trigonometric functions and their derivatives, which are essential topics in pre-calculus.
  • πŸ“ The script explains how to find the value of inverse trigonometric functions like arcsine and arccosine by drawing triangles in the correct quadrants and using the Pythagorean theorem.
  • πŸ” The range for arcsine is from 0 to Ο€, and for arctan, it's from Ο€/2 to -Ο€/2, which is important for understanding the correct quadrant to draw the triangle.
  • πŸ“‰ The script demonstrates how to find the arcsine of -1/2 by drawing a triangle in the fourth quadrant and identifying the angle as -Ο€/6.
  • πŸ“ˆ The video also covers how to find the arccosine of a negative value by drawing a triangle in quadrant 2 and identifying the angle as 3Ο€/4.
  • πŸ”‘ The concept of implicit differentiation is introduced to derive the formula for the derivative of arcsine, which is y' = 1/(cosine(y)).
  • 🧭 To express the derivative of arcsine in terms of x, the cosine of arcsine(x) is found to be √(1 - x^2), leading to the derivative formula y' = 1/√(1 - x^2).
  • πŸ“ The script explains the process of deriving the derivative of arctan using similar methods, resulting in the formula y' = 1/(1 + x^2).
  • πŸ”„ The chain rule is applied when differentiating composite functions involving inverse trigonometric functions, such as arcsine and arctan of a variable.
  • πŸ“š The video emphasizes the importance of memorizing the derivative formulas for arcsine and arctan, especially for exams like the AP exam.
  • πŸ“˜ Finally, the script provides examples of how to apply these derivative formulas to functions like f(x) = arcsin(2x) and f(x) = arctan(3x), using the chain rule when necessary.
Q & A

  • What is the restricted range for the arcsine function?

    -The restricted range for the arcsine function is from 0 to Pi, or in other terms, from 0 to Ο€.

  • What is the range for the arctangent function?

    -The range for the arctangent function is from -Ο€/2 to Ο€/2.

  • How do you find the arcsine of -1/2?

    -To find the arcsine of -1/2, you draw a triangle in the fourth quadrant, where the opposite side is -1 and the hypotenuse is 2, resulting in an angle of -Ο€/6.

  • Why is the range of arctangent not over quadrant 3?

    -The range of arctangent is not over quadrant 3 because the arctangent function does not have a restricted range in that quadrant.

  • What is the derivative of the arcsine function with respect to x?

    -The derivative of the arcsine function with respect to x is 1 / √(1 - x^2).

  • How do you derive the formula for the derivative of arcsine using implicit differentiation?

    -You start by setting y = arcsine(x), rewrite it as x = sin(y), differentiate both sides with respect to x, and solve for dy/dx to get 1 / √(1 - x^2).

  • What is the derivative of the arctangent function with respect to x?

    -The derivative of the arctangent function with respect to x is 1 / (1 + x^2).

  • How do you find the derivative of a function that involves arcsine or arctangent using the chain rule?

    -You multiply the derivative of the inner function (arcsine or arctangent) by the derivative of the outer function (the function inside arcsine or arctangent).

  • What is the derivative of the function f(x) = arcsine(2x)?

    -The derivative of f(x) = arcsine(2x) is 2 / √(1 - (2x)^2).

  • What is the derivative of the function f(x) = arctangent(3x)?

    -The derivative of f(x) = arctangent(3x) is 3 / (1 + (3x)^2).

  • How do you find the derivative of a function that involves the cosine of an arcsine?

    -You use the derivative of the cosine function, which is -sine, and multiply it by the derivative of the inner function (arcsine), which is 3 / √(1 - (3x)^2).

Outlines
00:00
πŸ“š Review of Inverse Trigonometric Functions and Derivatives

This paragraph provides a review of inverse trigonometric functions, focusing on how to determine their derivatives. It starts with a discussion of the restricted ranges for arcsine, arccosine, and arctangent, using the example of finding the arccosecant of -1/2 by drawing a triangle in the correct quadrant and applying the Pythagorean theorem to find the angle. The paragraph also covers the process of finding the arccosine of a value by drawing a triangle in quadrant 2 and rationalizing the denominator to simplify the calculation. The speaker emphasizes the importance of understanding these concepts and the ability to use a calculator for non-special triangles. The paragraph concludes with an introduction to deriving the formula for the derivative of arccosecant using implicit differentiation.

05:03
πŸ” Deriving Derivatives of Inverse Trigonometric Functions

The second paragraph delves into the process of deriving the derivatives of inverse trigonometric functions, specifically focusing on arccosecant and arctangent. The speaker uses implicit differentiation to derive the formula for the derivative of arccosecant, expressing it in terms of the cosine of the angle. The paragraph continues with a similar approach for arctangent, finding its derivative in terms of the secant squared of the angle. The speaker simplifies the expressions by relating them back to the original function's input variable, X. The paragraph also covers the chain rule application when differentiating composite functions involving inverse trigonometric functions, providing formulas for the derivatives of arcsine and arctangent in terms of their inside functions. The speaker concludes with examples of applying these derivatives to functions of the form f(x) = arcsine(2x) and f(x) = arctangent(3x), and a brief mention of the derivative of arccosine as the negative of the arcsine derivative.

Mindmap
Keywords
πŸ’‘Inverse Trigonometric Functions
Inverse trigonometric functions are mathematical operations that 'reverse' the trigonometric functions. They are used to find the angle when given the ratio of the sides of a right triangle. In the video, these functions are reviewed in the context of finding derivatives. Examples include arcsine, arccosine, and arctangent, which are the inverses of sine, cosine, and tangent, respectively.
πŸ’‘Derivatives
Derivatives in calculus represent the rate at which a function changes at a certain point. They are a fundamental concept in the study of rates of change and are essential in understanding the behavior of functions. In the video, the focus is on finding the derivatives of inverse trigonometric functions, which involves using techniques like implicit differentiation.
πŸ’‘Arcsine
Arcsine, denoted as arcsin or sin^-1, is the inverse of the sine function. It is used to find an angle given the sine of that angle. In the video, arcsine is discussed in relation to its restricted range and how to find its derivative using implicit differentiation, which results in the formula dy/dx = 1 / sqrt(1 - x^2).
πŸ’‘Arctangent
Arctangent, denoted as arctan or tan^-1, is the inverse of the tangent function. It is used to find an angle given the tangent of that angle. The video script explains how to find the derivative of arctangent using implicit differentiation, resulting in the formula dy/dx = 1 / (1 + x^2).
πŸ’‘Restricted Range
The restricted range refers to the specific intervals within which an inverse trigonometric function is defined and has a unique output. For example, arcsine has a restricted range from 0 to Ο€, while arctangent has a restricted range from -Ο€/2 to Ο€/2. Understanding these ranges is crucial for correctly applying inverse trigonometric functions.
πŸ’‘Pythagorean Theorem
The Pythagorean theorem is a fundamental principle in geometry that states that in a right-angled triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides. In the video, the theorem is used to find the lengths of the sides of triangles when dealing with inverse trigonometric functions.
πŸ’‘Implicit Differentiation
Implicit differentiation is a method used in calculus to find the derivative of a function that is not explicitly defined in terms of y. It involves differentiating both sides of an equation with respect to x, treating y as a function of x. The video demonstrates how to use implicit differentiation to find the derivatives of inverse trigonometric functions.
πŸ’‘Chain Rule
The chain rule is a fundamental theorem in calculus that allows you to differentiate composite functions. It states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function. In the video, the chain rule is used to find the derivatives of functions involving inverse trigonometric functions.
πŸ’‘Cosine
Cosine is a trigonometric function that relates the angle of a right triangle to the ratio of the adjacent side to the hypotenuse. In the video, the cosine function is discussed in the context of finding the derivative of arccosine, which involves understanding the relationship between the angle and its cosine value.
πŸ’‘Tangent
Tangent is a trigonometric function that relates the angle of a right triangle to the ratio of the opposite side to the adjacent side. In the video, tangent is used to illustrate the process of finding the derivative of arctangent, which involves understanding the relationship between the angle and its tangent value.
πŸ’‘Rationalize the Denominator
Rationalizing the denominator is a mathematical process used to eliminate radicals or fractions from the denominator of a fraction. In the video, the process is mentioned in the context of simplifying expressions involving cosine, which can make it easier to understand and work with the resulting expressions.
Highlights

Introduction to inverse trigonometric functions and their derivatives.

Explaining the restricted range for arccosine and arcsine/arc tangent.

Demonstration of finding the arcsine of -1/2 using a triangle in the fourth quadrant.

Use of the Pythagorean theorem to solve for the sides of the triangle.

Explanation of the negative angle in the context of the fourth quadrant.

Clarification on the non-applicability of arcsine in quadrant 3 despite the negative sine value.

Derivation of the derivative of arcsine using implicit differentiation.

Conversion of the arcsine relationship into a solvable equation for the derivative.

Finding the derivative of arcsine in terms of the original function argument.

Derivation of the derivative of arctan using a similar approach to arcsine.

Explanation of the secant squared in the context of the arctan derivative.

Presentation of the formulas for the derivatives of arcsine and arctan.

Introduction of the derivative formula for arccosine as the negative of arcsine.

Application of the chain rule to find the derivative of a composite function involving arcsine.

Application of the chain rule to find the derivative of a composite function involving arctan.

Example of using the chain rule with the derivative of cosine of arcsine.

Conclusion emphasizing the importance of knowing the derivatives for exams.

Transcripts

Browse More Related Video

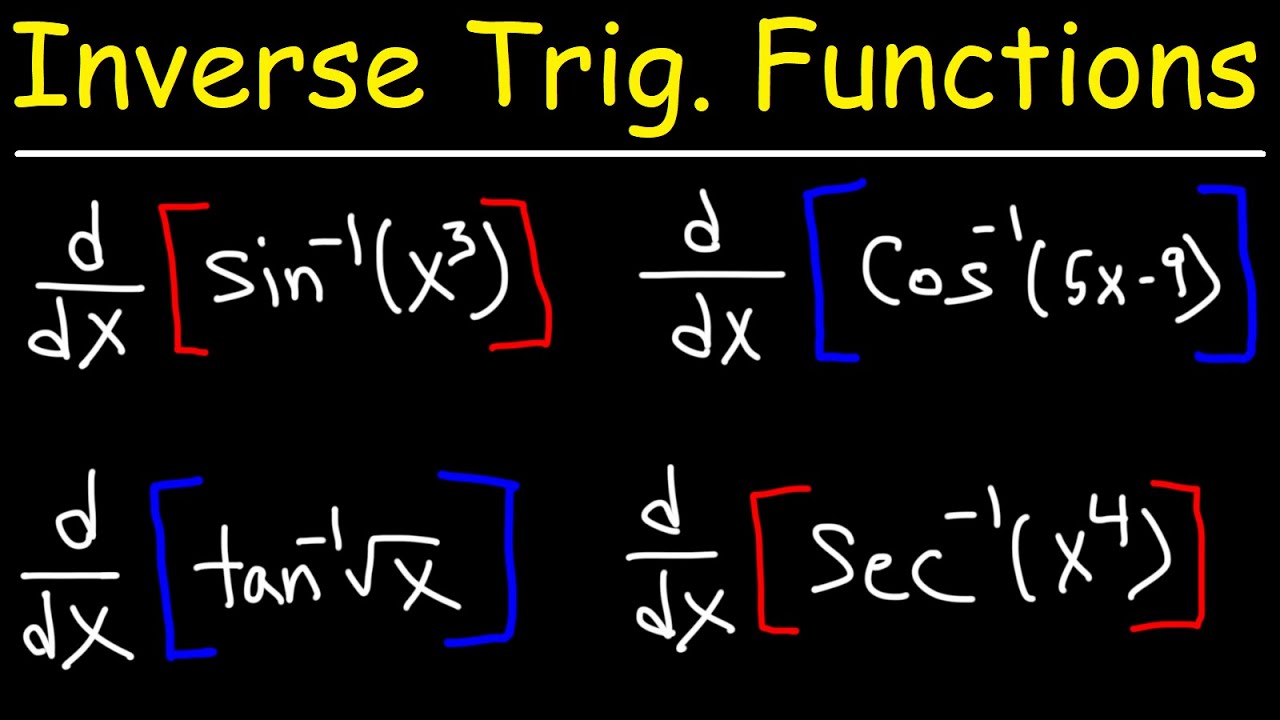
Derivatives of Inverse Trigonometric Functions

Take Derivatives of Inverse Trig Functions (ArcSin, ArcCos) - [2]

Lesson 2 - Derivatives Of Inverse Trig Functions (Calculus 2 Tutor)

Calculus - Find the derivative of inverse trigonometric functions

Calculus AB Homework 3.6 Derivatives of Inverses

An Indepth Look at Using Inverse Trig Functions (Precalculus - Trigonometry 21)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Inverse TrigonometryDerivative CalculationPre-CalculusArcsineArccosineArctangentTrigonometric FunctionsMathematics EducationPythagorean TheoremRationalizationChain Rule